NCERT Solutions for Class 12 Maths Chapter 1 - Relations and Functions - Exercise 1.3

Access Exercises of Class 12 Maths Chapter 1 - Relations and Functions

Exercise 1.1 Solutions : 16 Questions (14 Short Answers, 2 MCQ)

Exercise 1.2 Solutions : 12 Questions (10 Short Answers, 2 MCQ)

Exercise 1.3 Solutions : 14 Questions (12 Short Answers, 2 MCQ)

Exercise 1.4 Solutions : 13 Questions (12 Short Answers, 1 MCQ)

Miscellaneous Exercise Solutions: 19 Questions (7 Long answers, 9 Short Answer Type, 3 MCQs)

Exercise 1.3 :

1. Let f = {1, 3, 4} → {1, 2, 5} and g : {1, 2, 5} → {1, 3} be given by f : {(1, 2), (3, 5), (4, 1)} and g = {(1, 3),
(2, 3), (5, 1)}. Write down gof.

Sol. The functions f : {1, 3, 4} → {1, 2, 5} and g : {1, 2, 5} → {1, 3} are defined as f = {(1, 2), (3, 5), (4, 1)} and g = {(1, 3), (2, 3), (5, 1)}.
∴ gof(1) = g(f(1)) = g(2) = 3
[∴ f(1) = 2 and g(2) = 3] gof(3) = g(f(3)) = g(5) = 1
[∴ f(3) = 5 and g(5) = 1] gof(4) = g(f(4)) = g(1) = 3
[∴ f(4) = 1 and g(1) = 3]
∴ gof = {(1, 3), (3, 1), (4, 3)}

2. Let f, g and h be function from R to R. Show that
(f + g)oh = foh + goh;
(f.g)oh = (foh).(goh)

Sol. To prove (f + g)oh = foh + goh Consider
LHS = ((f + g)oh) (x) = (f + g)(h(x))
= f(h(x)) + g(h(x))
= (foh)(x) + (goh)(x)
= {(foh) + (goh)}(x)
∴ ((f + g)oh)(x) = ((foh) + (goh)}(x) ∀ x ∈ R
Hence, (f + g)oh = foh + goh
To prove (f.g)oh = (foh).(goh) Consider LHS = ((f.g)oh)(x)
= (f.g)(h(x)) = f(h(x)).g(h(x))
= (foh)(x).(goh)(x)
= {(foh).(goh)}(x)
∴ ((f.g)oh(x) = {(foh).(goh)}(x) ∀ x ∈ R
Hence, (f.g)oh = (foh).(goh)

3. Find gof and fog, if
(i) f(x) = |x| and g(x) = |5x – 2|
(ii) f(x) = 8x³ and 8(x) = x^1/3.

Sol. (i) f(x) = |x| and g(x) = |5x – 2|
∴ (gof)(x) = g(f(x)) = g(|x|)
= |5|x| – 2|
and fog(x) = f(g(x)) = f(|5x – 2|)
= ||5x – 2| = |5x – 2|

(ii) f(x) = 8x³ and g(x) = x^1/3
∴ (gof)(x) = g(f(x))
= g(^8x3) = (8x³)^1/3= 2x
and fog(x) = f(g(x)) = f(x^1/3)
= 8(x^1/3)³= 8x

$$\textbf{4. If f( x)}=\frac{(4x+3)}{(6x-4)},x≠\frac{2}{3},\text{show that fof(x) = x, for}\\\text{all}\space x≠\frac{2}{3}\space \text{What is the inverse of f?}$$

$$\textbf{Sol.}\text{It is given that f(x)}=\frac{(4x+3)}{(6x-4)},x≠\frac{2}{3},$$
$$\text{(fof)(x) = f(f(x)) }=f(\frac{(4x+3)}{(6x-4)})\\=\frac{4(\frac{4x+3}{6x-4})+3}{6(\frac{4x+3}{6x-4})-4}\\=\frac{16x+12+18x-12}{24x+18-24x+16}\\=\frac{34x}{34}=x\\\text{Therefore, fof(x) = x, for all}\space x≠\frac{2}{3}\space\text{ fof =I}$$
Hence, the given function f is invertible and the inverse of f is itself.

5. State with reason whether following functions have inverse :
(i) f : {1, 2, 3, 4} → {10} with
f = {(1, 10), (2, 10), (3, 10), (4, 10)}
(ii) g : {5, 6, 7, 8} → {1, 2, 3, 4} with
g = {(5, 4), (6, 3), (7, 4), (8, 2)}
(iii) h : {2, 3, 4, 5} → {7, 9, 11, 13} with
h = {(2, 7), (3, 9), (4, 11), (5, 13)}

Sol. (i) Function f : {1, 2, 3, 4} → {10} defined as f = {(1, 10), (2, 10), (3, 10), (4, 10)} From the given definition of f, we can see that f is a many one.
Function as f(1) = f(2) = f(3) = f(4) = 10.
Therefore, f is not one-one.
Hence, function f does not have an inverse.

(ii) Function g : {5, 6, 7, 8} → {1, 2, 3, 4} defined as g = {(5, 4), (6, 3), (7, 4), (8, 2)} From the given definition of g, it is seen that g is a many one.
Function as g(5) = g(7) = 4. Therefore, g is not one-one.
Hence, function g does not have an inverse.

(iii) Function h : {2, 3, 4, 5} → {7, 9, 11, 13} defined as h = {(2, 7), (3, 9), (4, 11), (5, 13)}
It is seen that all distinct elements of the set {2, 3, 4, 5} have distinct images under h.
Therefore, function h is one-one.
Also, h is onto, since for every element y of the set {7, 9, 11, 13}, there exists an element x in the set {2, 3, 4, 5} have distinct images under h. Thus, h is one-one and onto function. Hence, h has an inverse.

Note : Student should remember that any function has inverse exist only when f is one-one and onto.

$$\textbf{6. Show that f : [– 1, 1] → R, given by f(x)}\\=\frac{x}{(x+2)},$$$$\textbf{is one-one. Find the inverse of the function}$$$$\textbf{f : [– 1, 1] → Range f.}$$

$$\textbf{Sol}.\text{ Show that f : [– 1, 1] → R, given by f(x)}$$$$=\frac{x}{(x+2)},$$
Let f(x) = f(y)
$$⇒ \frac{x}{x+2}=\frac{y}{y+2}⇒ xy + 2x = xy + 2y\\⇒ 2x = 2y ⇒ x = y\\\text{Therefore, f is a one-one function.}\\\text{Let}\space y=\frac{x}{x+2}⇒x = xy + 2y\\⇒x=\frac{2y}{1-y}$$
So, for every y except 1 in the range there exists x in the domain such that f(x) = y. Hence, function f is onto.
Therefore, f : [– 1, 1] → Range f is one-one and onto and therefore, the inverse of the function f : [– 1, 1] → Range f exists.
Let y be an arbitrary element of range f.
Since, f : [– 1, 1] → Range f is onto, we have
y = f(x) for some x → [– 1, 1]
$$⇒y= \frac{x}{x+2}⇒xy+2y=x$$
$$⇒ x(1 – y) = 2y ⇒ x =\frac{2y}{1-y},y≠1$$
Now, let us define g : Range f → [– 1, 1] as
$$g(y) =\frac{2y}{1-y},y≠1\\Now, (gof)(x) = g(f(x)) =g(\frac{x}{x+2})$$
$$=\frac{2(\frac{x}{x+2})}{1-\frac{x}{x+2}}=\frac{2x}{x+2-x}=\frac{2x}{2}=2$$
(fog)(y) = f(g(y))
$$=f(\frac{2y}{1-y})=\frac{(\frac{2y}{1-y})}{\frac{2y}{1-y}+2}\\=\frac{2y}{2y+2-2y}=\frac{2y}{y}=y$$
Therefore, gof = fog = I_R, Therefore, f^–1 = g
$$\text{Therefore,},f^{-1}(y)=\frac{2y}{1-y},y≠1$$

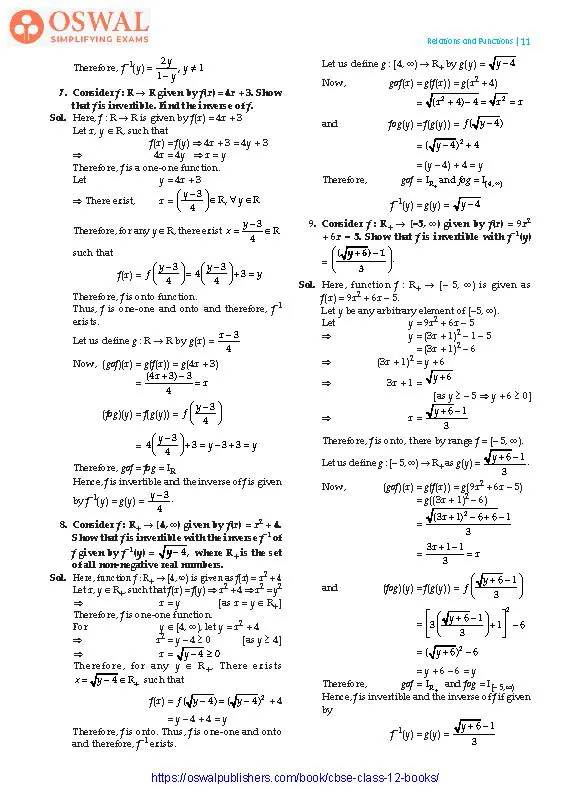
7. Consider f : R → R given by f(x) = 4x + 3. Show that f is invertible. Find the inverse of f.

Sol. Here, f : R → R is given by f(x) = 4x + 3
Let x, y ∈ R, such that
f(x) = f(y) ⇒ 4x + 3 = 4y + 3
⇒ 4x = 4y ⇒ x = y
Therefore, f is a one-one function.
Let y = 4x + 3
$$\text{⇒ There exist,}\space x=(\frac{y-3}{4})\space \varepsilon R,\forall \space y\varepsilon R$$
$$\text{Therefore, for any y ∈ R, there exist x}=\frac{y-3}{4}∈R$$
$$\text{such that}\space f(x)=f(\frac{y-3}{4})=4(\frac{y-3}{4})+3=y$$
Therefore, f is onto function.
Thus, f is one-one and onto and therefore, f^–1
exists.
$$\text{Let us define g : R → R by g(x) =}\frac{x-3}{4}\\Now, (gof)(x) = g(f(x)) = g(4x + 3)\\=\frac{(4x+3)-3}{4}=x\\(fog)(y) = f(g(y)) =f(\frac{y-3}{4})\\=4(\frac{y-3}{4})+3=y-3+3=y$$
Therefore, gof = fog = IR
Hence, f is invertible and the inverse of f is given
$$\text{by} f^{–1}(y) = g(y) =\frac{y-3}{4}.$$

8. Consider f : R₊ → [4, ∞) given by f(x) = x² + 4. Show that f is invertible with the inverse f^–1 of f given by f^–1(y) =√( y − 4 ), where R₊ is the set of all non-negative real numbers.

Sol. Here, function f : R₊ → [4, ∞) is given as f(x) = x² + 4
Let x, y ∈ R₊, such that f(x) = f(y)
⇒ x² + 4 ⇒ x² = y²
⇒ x = y [as x = y ∈ R₊]
Therefore, f is one-one function.
For y ∈ [4, ∞), let y = x² + 4
⇒ x² = y – 4 ≥ 0 [as y ≥ 4]
⇒ x =√ (y − 4) ≥ 0
Therefore, for any y ∈ R₊, There exists
x = √(y − 4) ∈ R₊ such that
f(x) = f (√( y − 4)) = ( √(y − 4))² + 4
= y – 4 + 4 = y
Therefore, f is onto. Thus, f is one-one and onto
and therefore, f^–1 exists.
Let us define g : [4, ∞) → R+ by g(y) =√(y-4)
Now, gof(x) = g(f(x)) = g(x² + 4)
=√(x²+4)-4=√x²=x
and fog(y) = f(g(y)) =f(√(y-4))
=(√(y-4))²+4
= (y – 4) + 4 = y
Therefore, gof = I_R₊ and I + fog =I [4, ∞)
f^–1(y) = g(y) =√(y-4)

9. Consider f : R+ → [–5, ∞) given by f(x) = 9x²+ 6x – 5. Show that f is invertible with f^–1(y)
$$=(\frac{(\sqrt{y+6})-1}{3})$$

Sol. Here, function f : R+ → [– 5, ∞) is given as
f(x) = 9x² + 6x – 5.
Let y be any arbitrary element of [–5, ∞).
Let y = 9x² + 6x – 5
⇒ y = (3x + 1)² – 1 – 5
= (3x + 1)² – 6
⇒ (3x + 1)² = y + 6
⇒ 3x + 1 = √(y + 6)
[as y ≥ – 5 ⇒ y + 6 ≥ 0]
$$⇒x=\frac{\sqrt{y+6}-1}{3}$$
Therefore, f is onto, there by range f = [– 5, ∞).
$$\text{Let us define g : [– 5, ∞) → R+ as g(y)}=\frac{\sqrt{y+6}-1}{3}$$
Now, (gof)(x) = g(f(x)) = g(9x² + 6x – 5)
= g((3x + 1)² – 6)
$$=\frac{\sqrt{(3x+1)^2-6+6}-1}{3}\\\frac{3x+1-1}{3}=x\\\text{and (fog)(y) = f(g(y))}\\=f(\frac{\sqrt{(y+6)-1}}{3})\\=[3(\frac{\sqrt{(y+6)-1}}{3})+1]^2-6\\=(\sqrt{y+6})^2-6\\= y + 6 – 6 = y$$
Therefore, gof = I_R+ and fog = I_{[– 5, ∞)}
Hence, f is invertible and the inverse of f if given
by
$$f^{–1}(y) = g(y) =\frac{\sqrt{y+6}-1}{3}$$

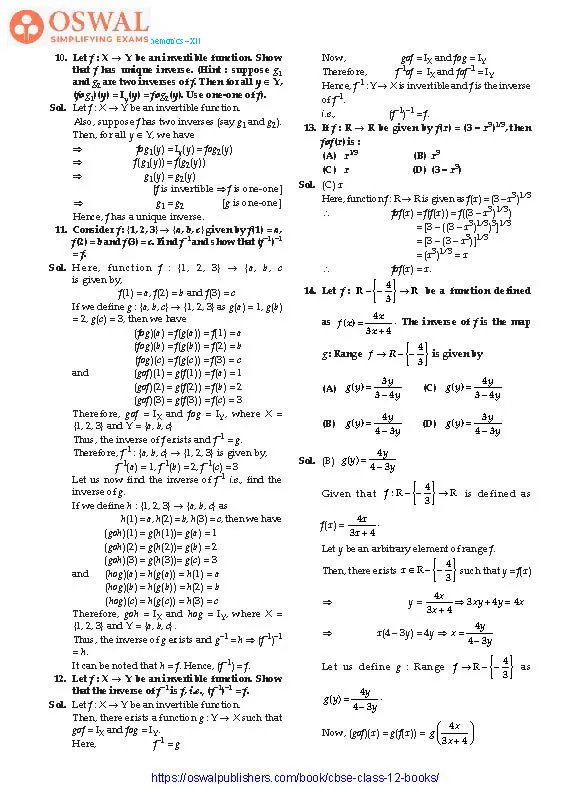
10. Let f : X → Y be an invertible function. Show that f has unique inverse. (Hint : suppose g₁ and g₂ are two inverses of f. Then for all y ∈ Y, (fog₁)(y) = Iy(y) = fog₂(y). Use one-one of f).

Sol. Let f : X → Y be an invertible function.
Also, suppose f has two inverses (say g₁ and g₂).
Then, for all y ∈ Y, we have
⇒ fog₁(y) = Iy(y) = fog₂(y)
⇒ f(g₁(y)) = f(g₂(y))
⇒ g₁(y) = g₂(y)
[f is invertible ⇒ f is one-one]
⇒ g₁ = g₂ [g is one-one]
Hence, f has a unique inverse.

11. Consider f : {1, 2, 3} → {a, b, c} given by f(1) = a, f(2) = b and f(3) = c. Find f^–1 and show that (f^–1)^–1= f.

Sol. Here, function f : {1, 2, 3} → {a, b, c
is given by,
f(1) = a, f(2) = b and f(3) = c
If we define g : {a, b, c} → {1, 2, 3} as g(a) = 1, g(b)
= 2, g(c) = 3, then we have
(fog)(a) = f(g(a)) = f(1) = a
(fog)(b) = f(g(b)) = f(2) = b
(fog)(c) = f(g(c)) = f(3) = c
and (gof)(1) = g(f(1)) = f(a) = 1
(gof)(2) = g(f(2)) = f(b) = 2
(gof)(3) = g(f(3)) = f(c) = 3
Therefore, gof = I_X and fog = I_Y, where X =
{1, 2, 3} and Y = {a, b, c}
Thus, the inverse of f exists and f^–1 = g.
Therefore, f^–1 : {a, b, c} → {1, 2, 3} is given by,
f^–1(a) = 1, f^–1(b) = 2, f^–1(c) = 3
Let us now find the inverse of f^–1 i.e., find the
inverse of g.
If we define h : {1, 2, 3} → {a, b, c} as
h(1) = a, h(2) = b, h(3) = c, then we have
(goh)(1) = g(h(1))= g(a) = 1
(goh)(2) = g(h(2))= g(b) = 2
(goh)(3) = g(h(3))= g(c) = 3
and (hog)(a) = h(g(a)) = h(1) = a
(hog)(b) = h(g(b)) = h(2) = b
(hog)(c) = h(g(c)) = h(3) = c
Therefore, goh = IX and hog = IY, where X = {1, 2, 3} and Y = {a, b, c}.
Thus, the inverse of g exists and g–1 = h ⇒ (f^–1)^–1= h.
It can be noted that h = f. Hence, (f^–1) = f.

12. Let f : X → Y be an invertible function. Show that the inverse of f^–1 is f, i.e., (f^–1)^–1 = f.

Sol. Let f : X → Y be an invertible function.

Then, there exists a function g : Y → X such that gof = I_X and fog = I_Y.

Here, f^–1 = g

Now, gof = I_X and fog = I_Y

Therefore, f^–1of = I_X and fof^–1 = I_Y

Hence, f^–1 : Y → X is invertible and f is the inverseof f^–1.

i.e., (f^–1)^–1 = f.

$$\textbf{13.\space If f} \textbf{: R}\xrightarrow{}\textbf{R}\space\textbf{be given by}\\\textbf{f(x) = (3-x}^{\textbf{3}})^{\frac{\textbf{1}}{\textbf{3}}}\textbf{,}\space \textbf{then fof(x) is :}\\\textbf{(A)\space x}^{\frac{\textbf{1}}{\textbf{3}}}\\\textbf{(B)\space x}^{\textbf{3}}\\\textbf{(C)\space} \textbf{x}\\\textbf{(D)\space}\textbf{(3-x}^{\textbf{3}})$$

Sol. (C) x

$$\text{Here, function}\space\text{f}: \text{R}\xrightarrow{}\text{R}\space\\\text{is given as f(x) = (3-x}^{3})^{\frac{1}{3}}\\\therefore\space\text{fof(x) = f(f(x)) = f((3-x}^{3})^{\frac{1}{3}})\\=\lbrack 3 - ((3-x^{3})^{\frac{1}{3}})^{3}\rbrack^{\frac{1}{3}}\\=\lbrack 3 - (3 - x^{3})\rbrack^{\frac{1}{3}}\\=(x^{3})^{\frac{1}{3}} = x$$

∴ fof(x) = x.

$$\textbf{14.\space Let}\space \textbf{f : R} - \begin{Bmatrix}-\frac{\textbf{4}}{\textbf{3}}\end{Bmatrix}\xrightarrow{}\textbf{R}\space$$

be a function defined as

$$\textbf{f(x)} =\frac{\textbf{4x}}{\textbf{3x+4}}\textbf{.}\space\textbf{The inverse of }\space\\\textbf{f is the map g : Range}\\\textbf{f}\xrightarrow{}\textbf{R} -\begin{Bmatrix}-\frac{\textbf{4}}{\textbf{3}}\end{Bmatrix}\space\textbf{is given by}\\\textbf{(A)\space g(y) =}\frac{\textbf{3y}}{\textbf{3-4y}}\\\textbf{(B)\space}\textbf{g(y) =} \frac{\textbf{4y}}{\textbf{4-3y}}\\\textbf{(C)\space}\textbf{g(y) = }\frac{\textbf{4y}}{\textbf{3-4y}}\\\textbf{(D)\space g(y) =}\frac{\textbf{3y}}{\textbf{4-3y}}\\\textbf{Sol.\space}\text{(B)} \space g(y) =\frac{4y}{4-3y} $$

$$\text{Given that}\space \text{f : R-}\begin{Bmatrix}-\frac{4}{3}\end{Bmatrix}\xrightarrow{}\text{R}\space$$

is defined as

$$\text{f(x) = }\frac{4x}{3x + 4}.$$

Let y be an arbitrary element of range f.

$$\text{Then, there exists x} \epsilon \text{R −}\begin{Bmatrix}-\frac{4}{3}\end{Bmatrix}$$

such that y = f(x)

$$\Rarr\space y =\frac{4x}{3x+4}\space\\\Rarr\space 3xy + 4y = 4x\\\Rarr\space x(4-3y) = 4y\\\Rarr\space x = \frac{4y}{4-3y}$$

Let us define g : Range

$$f\xrightarrow{} \text{R} -\begin{Bmatrix}-\frac{4}{3}\end{Bmatrix}\space\text{as}\space \text{g(y)} =\frac{4y}{4-3y}.$$

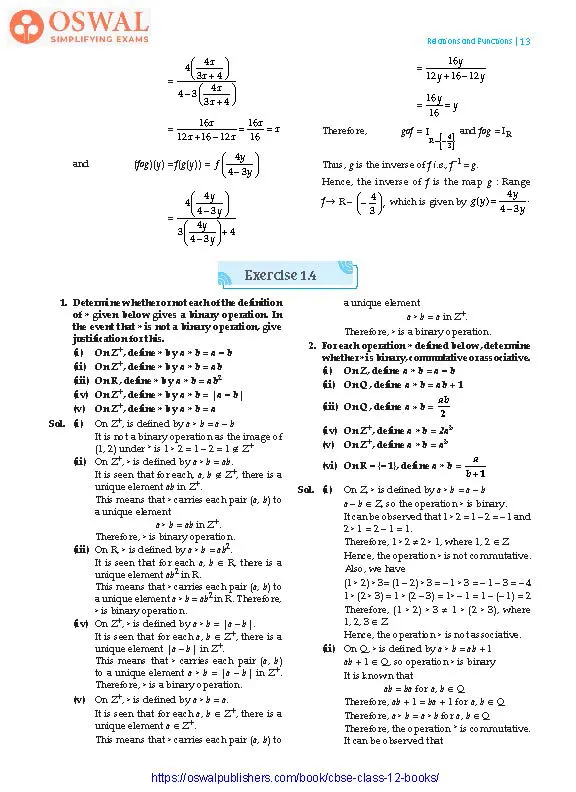
$$\text{Now, (gof)(x) = g(f(x)) =}\\g\bigg(\frac{4x}{3x+4}\bigg)\\=\frac{4\bigg(\frac{4x}{3x+4}\bigg)}{4-3\bigg(\frac{4x}{3x+4}\bigg)}\\=\frac{16x}{12x + 16 - 12 x} =\frac{16x}{16} = x$$

$$\text{and (fog)(y) = f(g(y))} =\\ f\bigg(\frac{4y}{4-3y}\bigg)\\=\frac{4\bigg(\frac{4y}{4-3y}\bigg)}{3\bigg(\frac{4y}{4-3y}\bigg) + 4}\\=\frac{16y}{12y +16- 12y}\\=\frac{16y}{16} = y$$

$$\text{Therefore,}\space\text{gof = I}_{\text{R -}\begin{Bmatrix}-\frac{4}{3}\end{Bmatrix}}\\\text{and fog = I}_{R}$$

Thus, g is the inverse of f i.e., f^–1 = g.

Hence, the inverse of f is the map g : Range

$$f\xrightarrow{}\text{R} -\bigg(-\frac{4}{3}\bigg),\space\\\text{which is given by g(y)} =\frac{4y}{4-3y}.$$