NCERT Solutions for Class 12 Maths Chapter 4 - Determinants
NCERT Solutions for Class 12 Mathematics Chapter 4 Free PDF Download
Please Click on Free PDF Download link to Download the NCERT Solutions for Class 12 Mathematics Chapter 4 Determinants
The dot mark ◉ field are mandatory, So please fill them in carefully
To download the complete Syllabus (PDF File), Please fill & submit the form below.
To download the complete Syllabus (PDF File), Please fill & submit the form below.
https://drive.google.com/file/d/1lOGMqP4EmwhMwAQtdP3KP-6J0jZCk38x/view?usp=sharing
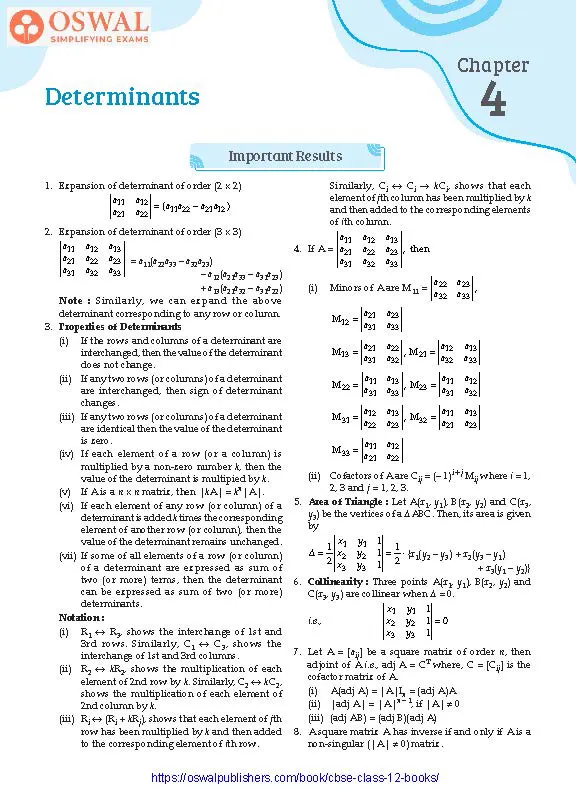
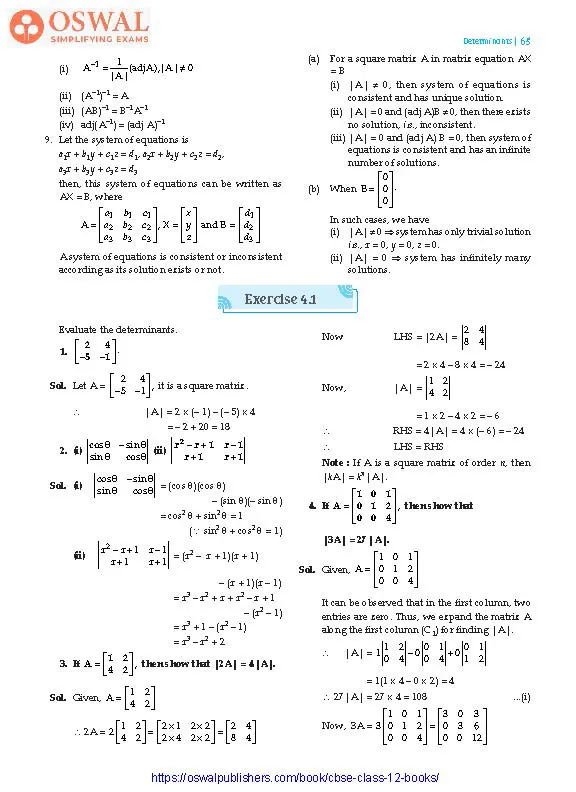
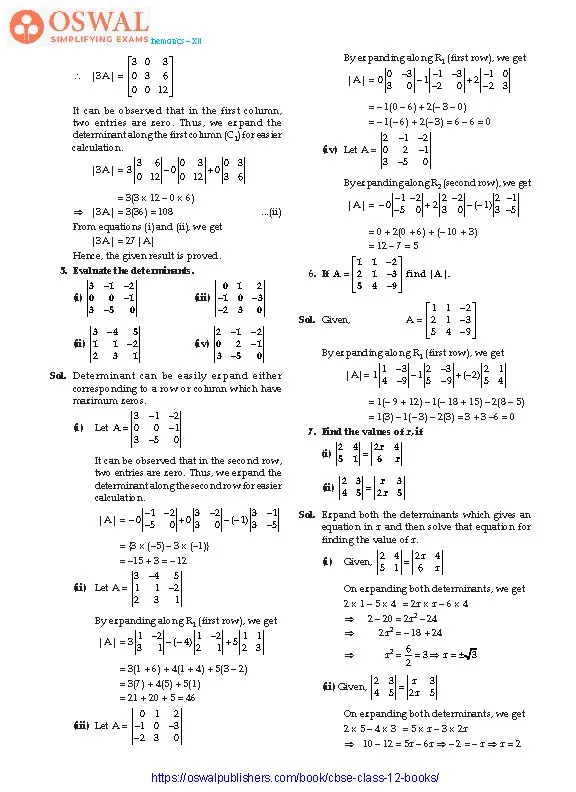
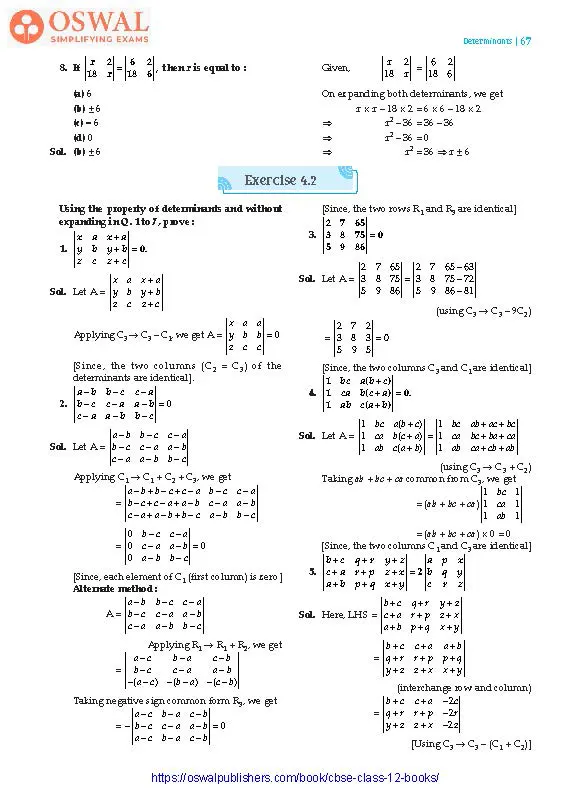
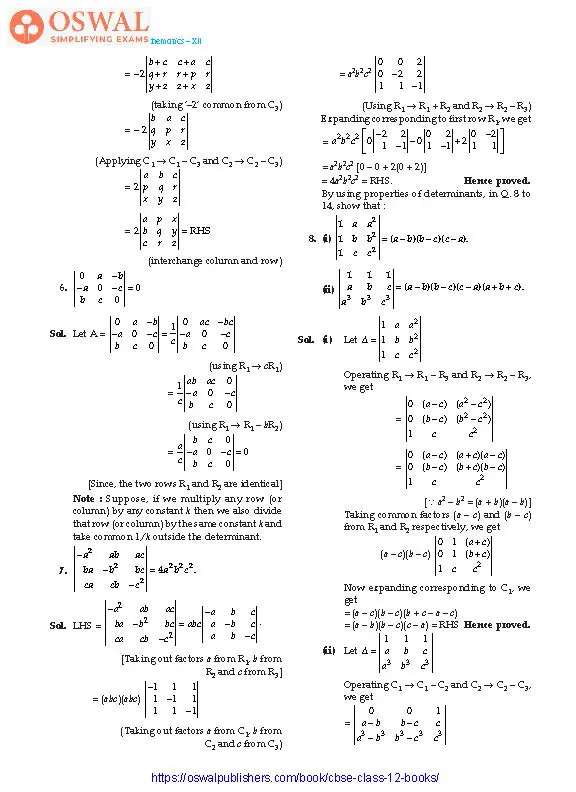
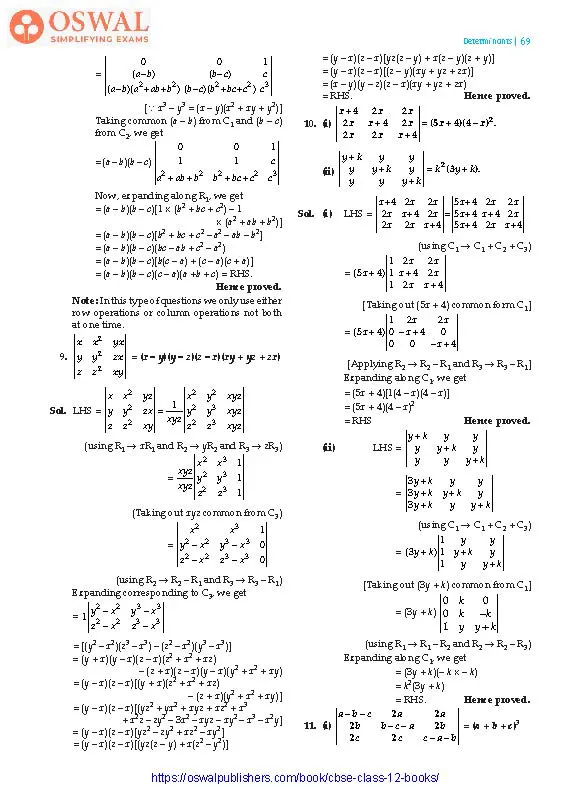
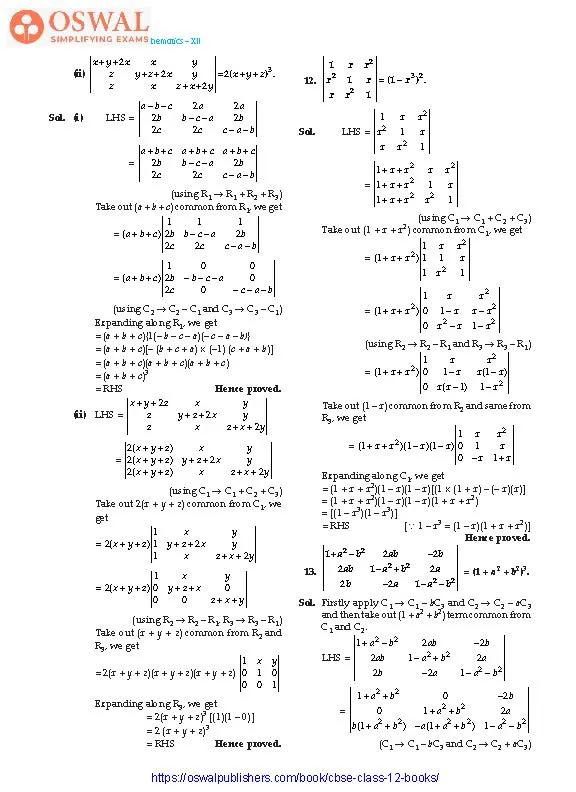
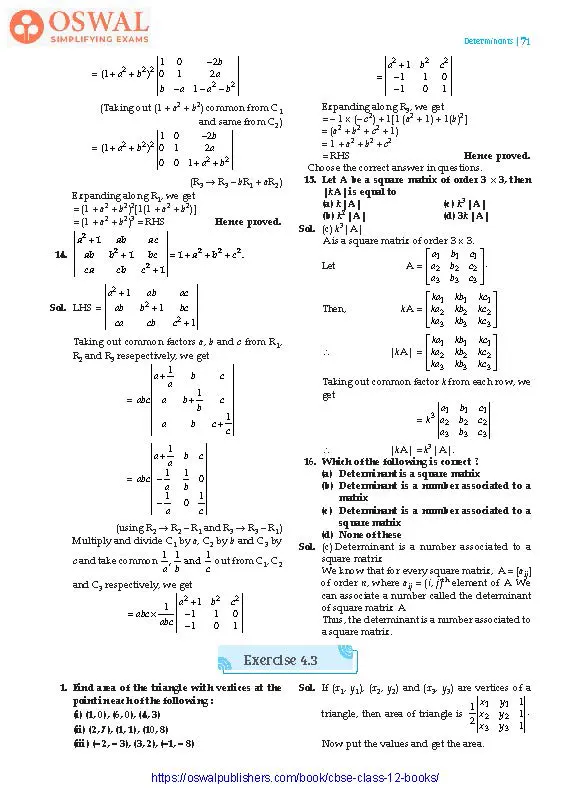

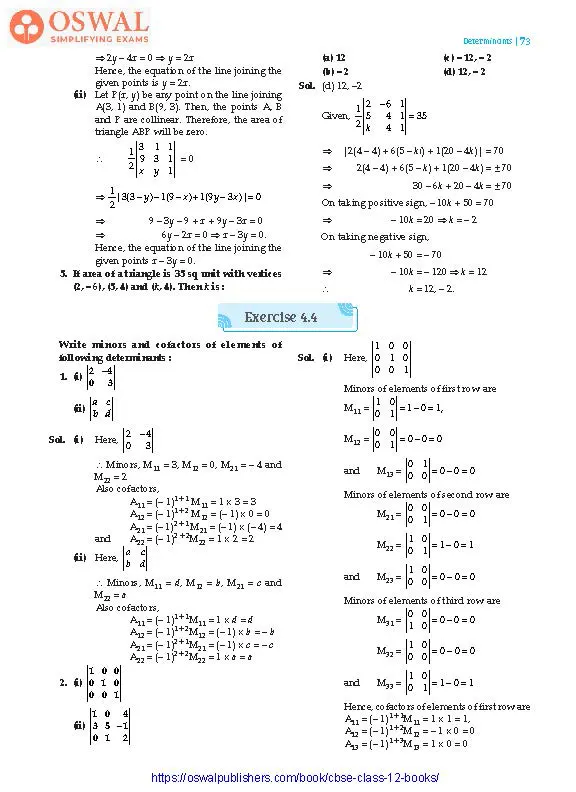
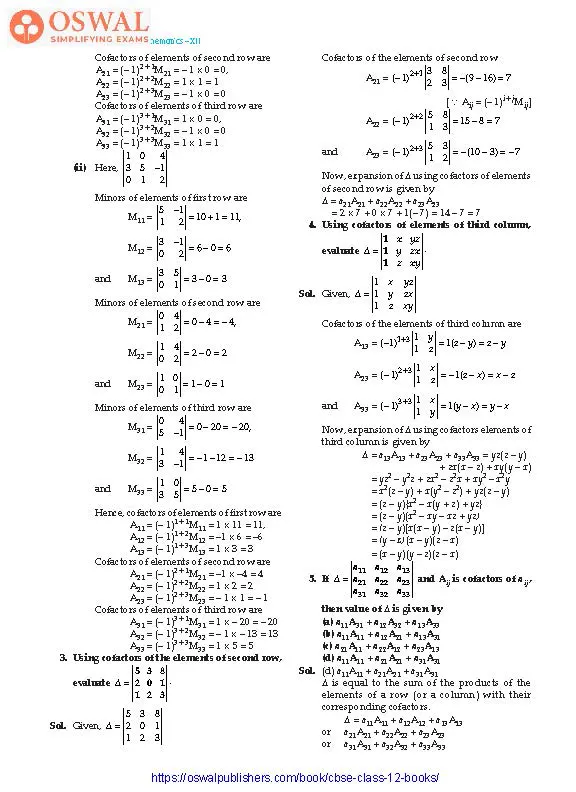

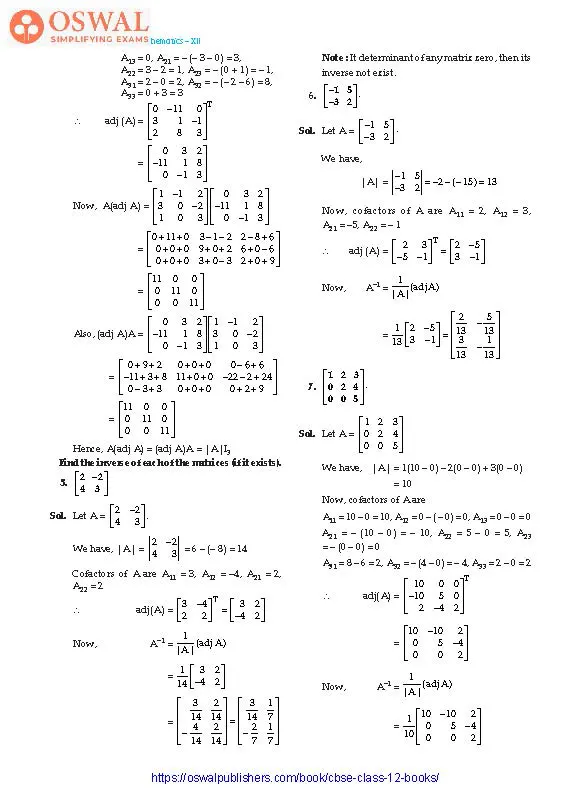
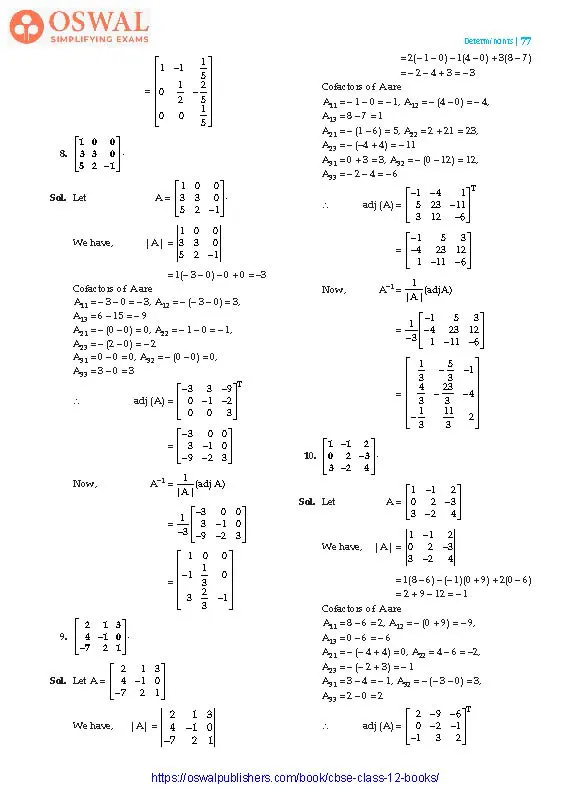
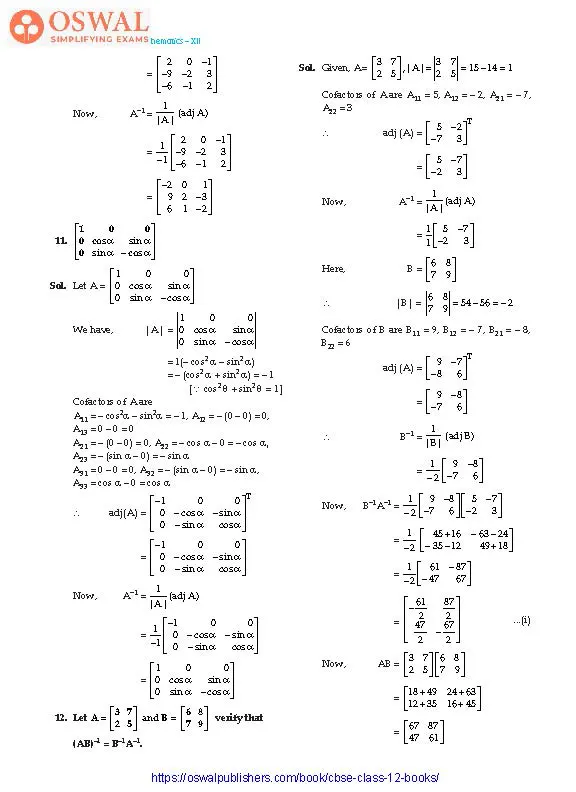


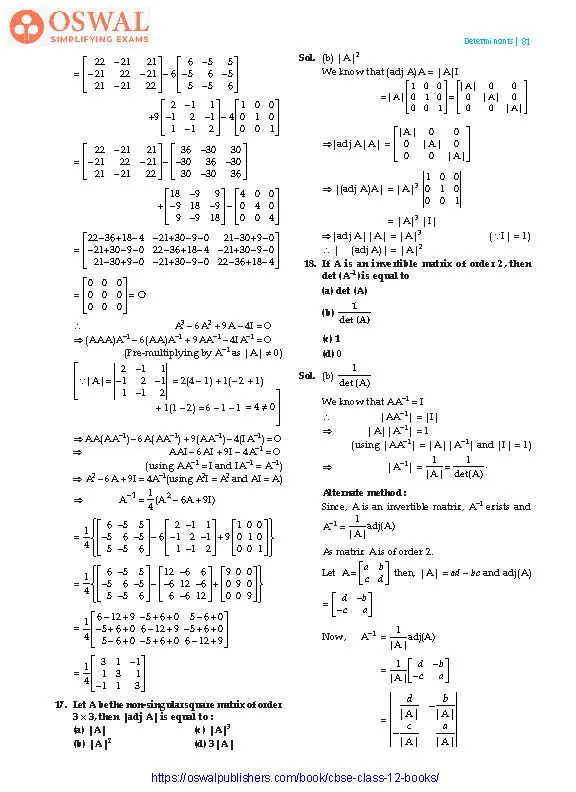




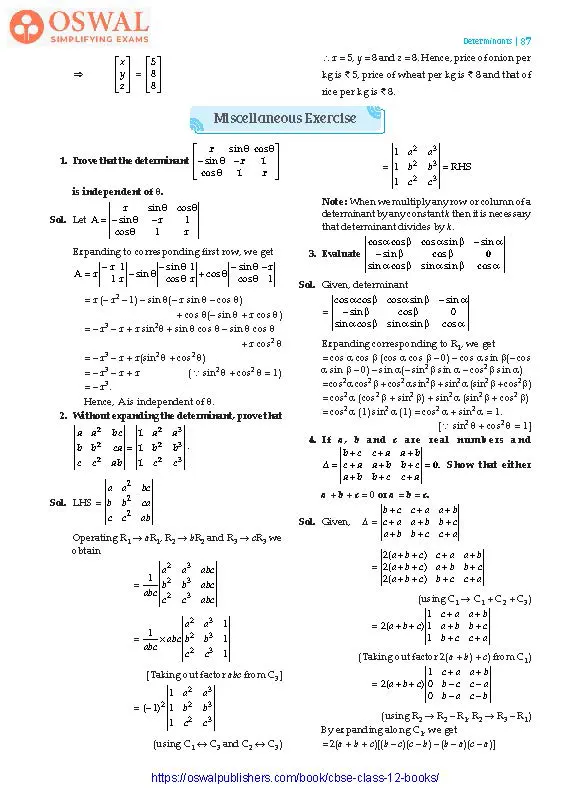

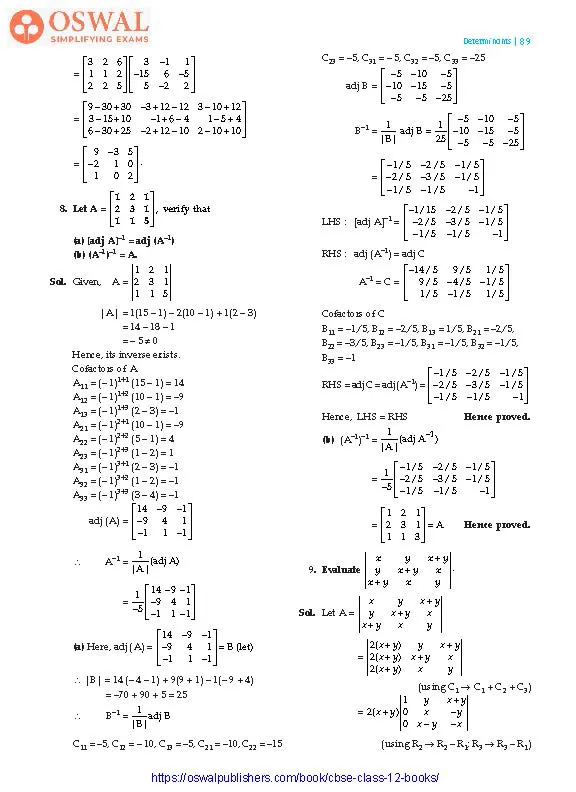
Access Exercises of Class 12 Maths Chapter 4 –Determinants
Exercise 4.1 Solutions: 8 Questions (2 Long, 5 Short Answers, 1 MCQ)
Exercise 4.2 Solutions: 16 Questions(7 Long, 7 Short, 2 MCQs)
Exercise 4.3 Solutions: 5 Questions ( 4 Short Answers, 1 MCQ)
Exercise 4.4 Solutions: 5 Questions (4 Long, 1 MCQ)
Exercise 4.5 Solutions: 18 Questions (11 Long, 5 Short, 2 MCQs)
Exercise 4.6 Solutions: 16 Questions (13 Long, 3 Short)
Miscellaneous Exercise Solutions: 19 Questions (15 Long, 1 Short, 3 MCQs)
