NCERT Solutions for Class 12 Maths Chapter 4 Determinants - Exercise 4.6




Access Exercises of Class 12 Maths Chapter 4 –Determinants
Exercise 4.1 Solutions: 8 Questions (2 Long, 5 Short Answers, 1 MCQ)
Exercise 4.2 Solutions: 16 Questions(7 Long, 7 Short, 2 MCQs)
Exercise 4.3 Solutions: 5 Questions ( 4 Short Answers, 1 MCQ)
Exercise 4.4 Solutions: 5 Questions (4 Long, 1 MCQ)
Exercise 4.5 Solutions: 18 Questions (11 Long, 5 Short, 2 MCQs)
Exercise 4.6 Solutions: 16 Questions (13 Long, 3 Short)
Miscellaneous Exercise Solutions: 19 Questions (15 Long, 1 Short, 3 MCQs)
Exercise 4.6
Examine the consistency of the system of equations in Q. 1 to 6.
1. x + 2y = 2, 2x + 3y = 3.
Sol. The given system can be written as AX = B, where
$$\text{A =}\begin{bmatrix}1 &2\\2 &3\end{bmatrix},\space\text{X} =\begin{bmatrix}x\\y\end{bmatrix}\\\text{and B} = \begin{bmatrix}2 \\3\end{bmatrix}\\|\text{A}| =\begin{vmatrix}1 &2\\2 &3\end{vmatrix}$$
= 1(3) − 2(2) = 3 − 4 = −1 ≠ 0
∴ A is non-singular. Therefore, A–1 exists.
Hence, the given system of equations is consistent.
2. 2x – y = 5, x + y = 4.
Sol. The given system can be written as AX = B, where
$$\text{|A|} =\begin{bmatrix}2 &\normalsize-1\\1 &1\end{bmatrix},\text{X} =\begin{bmatrix}x\\y\end{bmatrix}\\\text{and B = }\begin{bmatrix}5\\4\end{bmatrix}\\\text{Here, |A|} =\begin{vmatrix}2 &\normalsize-1\\1 &1\end{vmatrix}$$
=2(1)-(-1)(1) = 2+1 =3 ≠ 0
∴ A is non-singular. Therefore, A–1 exists.
Hence, the given system of equations is consistent.
3. x + 3y = 5, 2x + 6y = 8.
Sol. The given system can be written as AX = B, where
$$\text{A} = \begin{bmatrix}1 &3\\2 &6\end{bmatrix}\\\text{X} =\begin{bmatrix}x\\y\end{bmatrix}\text{B} =\begin{bmatrix}5\\8\end{bmatrix}\\\text{Here,\space}|\text{A}| =\begin{vmatrix}1 &3\\2 &6\end{vmatrix}$$
= 1(6) − 3(2) = 6 − 6 = 0
∴ A is singular matrix.
Nothing can be said about consistency as yet. We compute
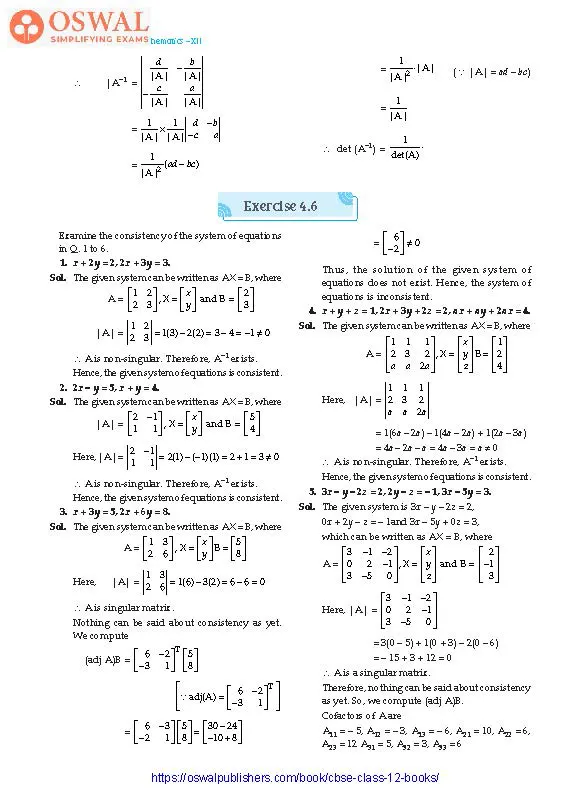
$$(\text{adj A})\text{B} =\begin{bmatrix}6 &-2\\-3 &1\end{bmatrix}^{\text{T}}\begin{bmatrix}5\\8\end{bmatrix}\\\begin{bmatrix}\because\space\text{adj A =}\begin{bmatrix}6 &\normalsize-2\\\normalsize-3 &1\end{bmatrix}^{\text{T}}\end{bmatrix}\\=\begin{bmatrix}6 &\normalsize-3\\\normalsize-2 &1\end{bmatrix}\begin{bmatrix}5\\8\end{bmatrix}\\=\begin{bmatrix}30-24\\-10+28\end{bmatrix}\\=\begin{bmatrix}6\\\normalsize-2\end{bmatrix}\neq 0$$
Thus, the solution of the given system of equations does not exist. Hence, the system of equations is inconsistent.
4. x + y + z = 1, 2x + 3y + 2z = 2, ax + ay + 2ax = 4.
Sol. The given system can be written as AX = B, where
$$\text{A} = \begin{bmatrix}1 &1 &1\\2 &3 &2\\a &a &2a\end{bmatrix},\\\text{X} =\begin{bmatrix}x\\y\\z\end{bmatrix}\space\text{B} = \begin{bmatrix}1 \\2 \\4\end{bmatrix}\\\text{Here, |A| =}\begin{vmatrix}1 &1 &1\\2 &3 &2\\a &a &2a\end{vmatrix}$$
= 1(6a – 2a) – 1(4a – 2a) + 1(2a – 3a)
= 4a – 2a – a = 4a – 3a = a ≠ 0
∴ A is non-singular. Therefore, A–1 exists.
Hence, the given system of equations is consistent.
5. 3x – y – 2z = 2, 2y – z = – 1, 3x – 5y = 3.
Sol. The given system is 3x – y – 2z = 2,
0x + 2y – z = – 1and 3x – 5y + 0z = 3,
which can be written as AX = B, where
$$\text{A} = \begin{bmatrix}3 &\normalsize-1 &\normalsize-2\\0 &2 &\normalsize-1\\3 &\normalsize-5 &0\end{bmatrix},\\\text{X} =\begin{bmatrix}x\\y\\z\end{bmatrix}\space\text{and B = }\begin{bmatrix}2 \\\normalsize-1\\3\end{bmatrix}\\\text{Here}\space|\text{A}| = \begin{bmatrix}3 &\normalsize-1 &\normalsize-2\\0 &2 &\normalsize-1\\3 &\normalsize-5 &\normalsize0\end{bmatrix}$$
= 3(0 – 5) + 1(0 + 3) – 2(0 – 6)
= – 15 + 3 + 12 = 0
∴ A is a singular matrix.
Therefore, nothing can be said about consistency as yet. So, we compute (adj A)B.
Cofactors of A are
A11 = – 5, A12 = – 3, A13 = – 6, A21 = 10, A22 = 6, A23 = 12 A31 = 5, A32 = 3, A33 = 6
$$\text{adj(A) =}\space\begin{bmatrix}-5 &-3 &-6\\10 &6 &12\\5 &3 &6\end{bmatrix}^{\text{T}}\\=\begin{bmatrix}\normalsize-5 &10 &5\\\normalsize-3 &6 &3\\\normalsize-6 &12 &6\end{bmatrix}\\\therefore\space\text{(adj A)B} =\begin{bmatrix}\normalsize-5 &10 &5\\\normalsize-3 &6 &3\\-6 &12 &6\end{bmatrix}\begin{bmatrix}2\\\normalsize-1\\3\end{bmatrix}\\=\begin{bmatrix}-10-10+15\\-6-6+9\\-12-12+18\end{bmatrix}=\begin{bmatrix}\normalsize-5\\\normalsize-3\\\normalsize-6\end{bmatrix}\neq 0$$
Thus, the solution of the given system of equations does not exist.
Hence, the system of equations is inconsistent.
6. 5x – y + 4z = 5, 2x + 3y + 5z = 2, 5x – 2y + 6z = –1.
Sol. The given system can be written as AX = B, where
$$\text{A =}\begin{bmatrix}5 &\normalsize-1 &\normalsize4\\2 &3 &5\\5 &\normalsize-2 &\normalsize-6\end{bmatrix},\\\text{X} =\begin{bmatrix}x\\y\\z\end{bmatrix}\space\text{and B =}\begin{bmatrix}5\\2\\\normalsize-1\end{bmatrix}\\\text{Here, |A| = }\begin{vmatrix}5 &\normalsize-1 &\normalsize4\\2 &3 &5\\5 &\normalsize-2 &6\end{vmatrix}$$
= 5(18 + 10) – (– 1)(12 – 25) + 4(– 4 – 15)
= 140 – 13 – 76 = 51 ≠ 0
∴ A is non-singular. Therefore, A–1 exists.
Hence, the given system of equations is consistent.
Solve the following system of linear equations, using matrix method in Q. 7 to 14.
7. 5x + 2y = 4, 7x + 3y = 5.
Sol. The given system can be written as AX = B, where
$$\text{A} = \begin{bmatrix}5 &2\\7 &3\end{bmatrix},\text{X} =\begin{bmatrix}x\\y\end{bmatrix}\\\text{and B} =\begin{bmatrix}4 \\5\end{bmatrix}\\\text{Here,\space |A| = }\begin{vmatrix}5 &2\\7 &3\end{vmatrix}\\=15-14=1\neq 0$$
Thus, A is non-singular. Therefore, its inverse exists.
Therefore, the given system is consistent and has a unique solution given by
A–1(AX) = A–1B ⇒ X = A–1B
Cofactors of A are, A11 = 3, A12 = – 7, A21 = – 2, A22 = 5
$$\therefore\space\text{adj (A)} =\begin{bmatrix}3 &\normalsize-7\\\normalsize-2 &5\end{bmatrix}^{\text T}\\=\begin{bmatrix}3 &\normalsize-2\\\normalsize-7 &5\end{bmatrix}\\\text{Now, A}^{\normalsize-1}=\frac{1}{|\text{A}|}(\text{adj A})\\=\frac{1}{1}\begin{bmatrix}3 &\normalsize-2\\\normalsize-7 &5\end{bmatrix}\\=\begin{bmatrix}3 &\normalsize-2\\\normalsize-7 &5\end{bmatrix},\\\text{X} =\text{A}^{\normalsize-1}\text{B} \\=\begin{bmatrix}3 &\normalsize-2\\\normalsize-7 &5\end{bmatrix}\begin{bmatrix}4\\5\end{bmatrix}$$
$$=\begin{bmatrix}3×4+(\normalsize-2)×5\\-7×4+5 ×5\end{bmatrix} =\begin{bmatrix}2 \\\normalsize-3\end{bmatrix}$$
$$\Rarr\space\begin{bmatrix}2 \\\normalsize-3\end{bmatrix} = \begin{bmatrix}x\\ y\end{bmatrix}$$
Hence, x = 2 and y = – 3.
8. 2x – y = –2, 3x + 4y = 3.
Sol. The given system can be written as AX = B, where
$$\text{A} =\begin{bmatrix}2 &\normalsize-1\\3 &4\end{bmatrix},\text{X} =\begin{bmatrix}x\\y\end{bmatrix},\\\text{B = }\begin{bmatrix}\normalsize-2\\3\end{bmatrix}\\\text{Here,\space |A| =}\begin{vmatrix}2 &\normalsize-1\\3 &4\end{vmatrix}$$
= 2 × 4-(-3) = 11 ≠ 0
Thus, A is non-singular. Therefore, its inverse
exists.
Therefore, the given system of consistent and has
a unique solution given by
X = A–1B
Cofactors of A are A11 = 4, A12 = – 3, A21 = 1, A22 = 2
$$\text{adj\space(A) = }\begin{bmatrix}4 &\normalsize-3\\1 &2\end{bmatrix}^{\text{T}}\\=\begin{bmatrix}4 &1\\\normalsize-3 &2\end{bmatrix}\\\therefore\space\text{A}^{\normalsize-1}=\frac{1}{|\text{A}|}(\text{adj A}) \\=\frac{1}{11}\begin{bmatrix}4 &1\\\normalsize-3 &2\end{bmatrix}$$
Now, X = A−1 B
$$=\frac{1}{11}\begin{bmatrix}4 &1\\\normalsize-3 &2\end{bmatrix}\begin{bmatrix}\normalsize-2\\3\end{bmatrix}\\=\frac{1}{11}\begin{bmatrix}-8+3\\6+6\end{bmatrix}\\=\frac{1}{11}\begin{bmatrix}-5\\12\end{bmatrix}\\=\begin{bmatrix}-\frac{5}{11}\\\frac{12}{11}\end{bmatrix}\\\Rarr\space\begin{bmatrix}x\\y\end{bmatrix} =\begin{bmatrix}-\frac{5}{11}\\\frac{12}{11}\end{bmatrix}\\\text{Hence, x =}\frac{\normalsize-5}{11}\space\text{and y} =\frac{12}{11}.$$
9. 4x – 3y = 3, 3x – 5y = 7.
Sol. The given system can be written as AX = B, where
$$\text{A} =\begin{bmatrix}4 &\normalsize-3\\3 &\normalsize-5\end{bmatrix},\\\text{X} =\begin{bmatrix}x\\y\end{bmatrix},\space\text{B} =\begin{bmatrix}3\\7\end{bmatrix}\\\text{Here, |A| =}\begin{vmatrix}4 &\normalsize-3\\3 &\normalsize-5\end{vmatrix}\\= 4(\normalsize-5)-3(\normalsize-3)$$
= – 20 + 9 = – 11 ≠ 0
Thus, A is non-singular. Therefore, its inverse exists.
Therefore, the given system is consistent and has a unique solution given by X = A–1B.
Cofactors of A are
A11 = – 5, A12 = – 3, A21 = 3, A22 = 4
$$\text{adj(A)} =\begin{bmatrix}\normalsize-5 &\normalsize-3\\3 &4\end{bmatrix}^{\text{T}}\\=\begin{bmatrix}\normalsize-5 &3\\\normalsize-3 &4\end{bmatrix}\\\therefore\space\text{A}^{\normalsize-1}=\frac{1}{|\text{A}|}(\text{adj A}) \\=\frac{1}{\normalsize-11}\begin{bmatrix}\normalsize-5 &3\\\normalsize-3 &4\end{bmatrix}$$
Now, X = A−1B
$$=\frac{1}{\normalsize-11}\begin{bmatrix}\normalsize-5 &3\\\normalsize-3 &4\end{bmatrix}\begin{bmatrix}3 \\7\end{bmatrix}\\=\frac{1}{-11}\begin{bmatrix}-15+21\\-9+28\end{bmatrix}\\=\frac{1}{\normalsize-11}\begin{bmatrix}6\\9\end{bmatrix}\\\Rarr\space\begin{bmatrix}x\\y\end{bmatrix} =\begin{bmatrix}\frac{-6}{11}\\\frac{-19}{11}\end{bmatrix}.\\\text{Hence, x = -}\frac{6}{11}\space\text{and y}=-\frac{19}{11}.$$
10. 5x + 2y = 3, 3x + 2y = 5.
Sol. The given system can be written as AX = B, where
$$\text{A} =\begin{bmatrix}5 &2\\3 &2\end{bmatrix},\space\text{X} =\begin{bmatrix}x\\y\end{bmatrix}\\\text{and B} = \begin{bmatrix}3 \\5\end{bmatrix}\\\text{Here,\space |A| =}\begin{vmatrix}5 &2\\3 &2\end{vmatrix}$$
= 10 − 6 = 4 ≠ 0.
Thus, A is non-singular. Therefore, its inverse exists.
Therefore, the given system is consistent and has a unique solution given by X = A–1B.
Cofactors of A are,
A11 = 2, A12 = – 3, A21 = –2, A22 = 5
$$\text{adj\space(A)} =\begin{bmatrix}2 &\normalsize-3\\\normalsize-2 &5\end{bmatrix}^{\text{T}}\\=\begin{bmatrix}2 &\normalsize-2\\\normalsize-3 &5\end{bmatrix}\\\therefore\space\text{A}^{\normalsize-1}=\frac{1}{|\text{A}|}\space\text{(adj A)}\\=\frac{1}{4}\begin{bmatrix}2 &\normalsize-2\\\normalsize-3 &5\end{bmatrix}$$
Now, X = A–1B
$$=\frac{1}{4}\begin{bmatrix}2 &\normalsize-2\\\normalsize-3 &5\end{bmatrix}\begin{bmatrix}3\\5\end{bmatrix}\\=\frac{1}{4}\begin{bmatrix}6-10\\-9+25\end{bmatrix}\\=\frac{1}{4}\begin{bmatrix}\normalsize-4\\16\end{bmatrix} =\begin{bmatrix}\normalsize-1 \\4\end{bmatrix}\\\Rarr\space\begin{bmatrix}x\\y\end{bmatrix}= \begin{bmatrix}\normalsize-1\\4\end{bmatrix}.$$
Hence, x = – 1 and y = 4.
$$\textbf{11.}\space \textbf{2x + y + z = 1,}\\\textbf{x − 2y − z}\textbf{=}\frac{\textbf{3}}{\textbf{2}},\\\textbf{3y - 5z = 9} $$
Sol. The given system can be written as AX = B, where
$$\text{A =}\begin{bmatrix}2 &1 &1\\2 &\normalsize-4 &\normalsize-2\\0 &3 &\normalsize-5\end{bmatrix},\\\text{X} =\begin{bmatrix}x\\y\\z\end{bmatrix}\space\text{and B =}\begin{bmatrix}1\\3\\9\end{bmatrix}\\\text{Here,\space}|\text{A}| =\begin{bmatrix}2 &1 &1\\2 &\normalsize-4 &\normalsize-2\\0 &3 &\normalsize-5\end{bmatrix}$$
= 2(20 + 6) – 1(– 10 – 0) + 1(6 – 0)
= 52 + 10 + 6 = 68 ≠ 0
Thus, A is non-singular. Therefore, its inverse exists.
Therefore, the given system is consistent and has a unique solution given by X = A–1B.
Cofactors of A are
A11 = 20 + 6 = 26, A12 = – (– 10 + 0) = 10,
A13 = 6 + 0 = 6
A21 = – (– 5 – 3) = 8, A22 = – 10 – 0 = – 10,
A23 = – (6 – 0) = – 6
A31 = (– 2 + 4) = 2, A32 = – (– 4 – 2) = 6,
A33 = – 8 – 2 = – 10
$$\text{adj}\space(\text{A}) = \begin{bmatrix}26 &10 &6\\8 &\normalsize-10 &\normalsize-6\\2 &6 &\normalsize-10\end{bmatrix}^{\text{T}}\\=\begin{bmatrix}26 &8 &2\\10 &\normalsize-10 &6\\6 &\normalsize-6 &\normalsize-10\end{bmatrix}\\\therefore\space\text{A}^{\normalsize-1}=\frac{1}{|\text{A}|}(\text{adj A})\\=\frac{1}{68}\begin{bmatrix}26 &8 &2\\10 &\normalsize-10 &6\\6 &\normalsize-6 &10\end{bmatrix}$$
Now, X = A–1B
$$\Rarr\space\begin{bmatrix}x\\y\\z\end{bmatrix}=\frac{1}{68}\\\begin{bmatrix}26 &8 &2\\10 &\normalsize-10 &6\\6 &\normalsize-6 &10\end{bmatrix}\begin{bmatrix}1 \\3\\9\end{bmatrix}\\\Rarr\space\begin{bmatrix}x\\y\\z\end{bmatrix}=\frac{1}{68}\begin{bmatrix}26+24+18\\10-30+54\\6-18-90\end{bmatrix}\\=\frac{1}{68}\begin{bmatrix}68\\34\\\normalsize-102\end{bmatrix}\\\Rarr\space\begin{bmatrix}x\\y\\z\end{bmatrix}=\begin{bmatrix}1\\\frac{1}{2}\\\frac{\normalsize-3}{2}\end{bmatrix}$$
$$\text{Hence, x=1, y=}\frac{1}{2}\\\text{and z}=\frac{\normalsize-3}{2}.$$
12. x – y + z = 4, 2x + y – 3z = 0, x + y + z = 2.
Sol. The given system can be written as AX = B, where
$$\text{A = }\begin{bmatrix}1 &\normalsize-1 &1\\2 &1 &\normalsize-3\\1 &1 &1\end{bmatrix},\\\text{X} =\begin{bmatrix}x\\y\\z\end{bmatrix}\space\text{and B = }\begin{bmatrix}4\\0\\2\end{bmatrix}\\\text{Here,\space |\text{A}| =}\begin{bmatrix}1 &\normalsize-1 &1\\2 &1 &\normalsize-3\\1 &1 &1\end{bmatrix}$$
= 1(1 + 3) – (– 1)(2 + 3) + 1(2 – 1)
= 4 + 5 + 1 = 10 ≠ 0
Thus, A is non-singular. Therefore, its inverse exists.
Therefore, the given system of consistent and has a unique solution given by X = A–1B.
Cofactors of A are
A11 = 1 + 3= 4, A12 = – (2 + 3) = – 5, A13 = 2 – 1 = 1,
A21 = – (– 1 – 1) = 2, A22 = 1 – 1 = 0,A23 = – (1 + 1) = – 2,
A31 = 3 – 1 = 2, A32 = – (– 3 – 2) = 5, A33 = 1 + 2 = 3
$$\therefore\space\text{adj(A)} = \begin{bmatrix}4 &-5 &1\\2 &0 &-2\\2 &5 &3\end{bmatrix}^{\text{T}}\\=\begin{bmatrix}4 &2 &2\\\normalsize-5 &0 &5\\1 &\normalsize-2 &3\end{bmatrix}$$
$$\therefore\space\text{A}^{\normalsize-1}=\frac{1}{|\text{A}|}\space\text{(adj A)}\\=\frac{1}{10}\space\begin{bmatrix}4 &2 &2\\\normalsize-5 &0 &5\\1 &\normalsize-2 &3\end{bmatrix}$$
Now, X = A–1B
$$\Rarr\space\begin{bmatrix}x\\y\\z\end{bmatrix} =\frac{1}{10}\\\begin{bmatrix}4 &2 &2\\\normalsize-5 &0 &5\\1 &\normalsize-2 &3\end{bmatrix}\begin{bmatrix}4 \\0 \\2\end{bmatrix}\\\Rarr\space\begin{bmatrix}x\\y \\z\end{bmatrix} = \frac{1}{10}\begin{bmatrix}16+0+4\\-20+0+10\\4+0+6\end{bmatrix}\\\Rarr\space\begin{bmatrix}x\\y \\z\end{bmatrix}=\frac{1}{10}\begin{bmatrix}20\\\normalsize-10\\10\end{bmatrix} = \begin{bmatrix}2 \\\normalsize-1\\1\end{bmatrix}$$
Hence, x = 2, y = – 1 and z = 1.
13. 2x + 3y + 3z = 5, x – 2y + z = – 4, 3x – y – 2z = 3.
Sol. The given system can be written as AX = B, where
$$\text{A = }\begin{bmatrix}2 &3 &3\\1 &\normalsize-2 &1\\3 &\normalsize-1 &\normalsize-2\end{bmatrix},\\\text{X} =\begin{bmatrix}x\\y\\z\end{bmatrix}\space\text{and B} =\begin{bmatrix}5\\\normalsize 4\\3\end{bmatrix}\\\text{Here,}\space|\text{A}| =\begin{bmatrix}2 &3 &3\\1 &\normalsize-2 &1\\3 &\normalsize-1 &\normalsize-2\end{bmatrix}$$
= 2 (4 + 1) – 3(– 2 – 3) + 3(– 1 + 6)
= 10 + 15 + 15 = 40 ≠ 0
Thus, A is non-singular. Therefore, its inverse exists. Therefore, the given system is consistent and has a unique solution given by X = A–1B.
Cofactors of A are
A11 = 4 + 1 = 5, A12 = – (– 2 – 3) = 5, A13 = (– 1 + 6) = 5,
A21 = – (– 6 + 3) = 3, A22 = (– 4 – 9) = – 13, A23 = – (– 2 – 9) = 11,
A31 = 3 + 6 = 9, A32 = – (2 – 3) = 1, A33 = – 4 – 3 = – 7
$$\text{adj(A) = }\begin{bmatrix}5 &5 &5\\3 &\normalsize-13 &\normalsize-11\\9 &1 &\normalsize-7\end{bmatrix}^{\text{T}}\\=\begin{bmatrix}5 &3 &9\\5 &\normalsize-13 &1\\5 &11 &\normalsize-7\end{bmatrix}\\\therefore\space\text{A}^{\normalsize-1} =\frac{1}{|\text{A}|}(\text{adj A})\\=\frac{1}{40}\begin{bmatrix}5 &3 &9\\5 &\normalsize-13 &1\\5 &11 &\normalsize-7\end{bmatrix}$$
Now, X = A–1B
$$\Rarr\space\begin{bmatrix}x\\y\\z\end{bmatrix} =\frac{1}{40}\\\begin{bmatrix}5 &3 &9\\5 &-13 &1\\5 &11 &-7\end{bmatrix}\begin{bmatrix}5 \\\normalsize-4 \\3\end{bmatrix}\\=\frac{1}{40}\begin{bmatrix}25-12+27\\25+52+3\\25-44-21\end{bmatrix}\\=\frac{1}{40}\begin{bmatrix}40\\80\\\normalsize-40\end{bmatrix}=\begin{bmatrix}1 \\2 \\\normalsize-1\end{bmatrix}$$
Hence, x = 1, y = 2 and z = – 1.
14. x – y + 2z = 7, 3x + 4y – 5z = – 5, 2x – y + 3z = 12.
Sol. The given system can be written as AX = B, where
$$\text{A} =\begin{bmatrix}1 &\normalsize-1 &2\\3 &4 &\normalsize-5\\2 &\normalsize-1 &3\end{bmatrix},\space\text{X} =\begin{bmatrix}x\\y\\z\end{bmatrix}\\\text{and B =}\begin{bmatrix}7 \\\normalsize-5\\12\end{bmatrix}\\\text{Here,\space |\text{A}|} =\begin{vmatrix}1 &\normalsize-1 &2\\3 &4 &\normalsize-5\\2 &\normalsize-1 &3\end{vmatrix}$$
= 1(12 – 5) – (– 1)(9 + 10) + 2(– 3 – 8)
= 7 + 19 – 22 = 4 ≠ 0
Thus, A is non-singular. Therefore, its inverse exists.
Therefore, the given system is consistent and has a unique solution given by X = A–1B.
Cofactors of A are
A11 = 12 – 5 = 7, A12 = – (9 + 10) = – 19, A13 = – 3 – 8 = – 11,
A21 = – (– 3 + 2) = 1, A22 = 3 – 4 = – 1, A23 = – (– 1 + 2) = – 1
A31 = 5 – 8 = – 3, A32 = – (– 5 – 6) = 11, A33 = 4 + 3 = 7
$$\text{(adj A)} =\begin{bmatrix}7 &-19 &-11\\1 &\normalsize-1 &\normalsize-1\\\normalsize-3 &11 &7\end{bmatrix}^{\text{T}}\\=\begin{bmatrix}7 &1 &\normalsize-3\\\normalsize-19 &\normalsize-1 &11\\\normalsize-11 &\normalsize-1 &7\end{bmatrix}\\\therefore\space\text{A}^{\normalsize-1}=\frac{1}{|\text A|}(\text{adj}\space\text{A})\\=\frac{1}{4}\begin{bmatrix}7 &1 &\normalsize-3\\\normalsize-19 &\normalsize-1 &11\\-11 &\normalsize-1 &7\end{bmatrix}$$
Now, X = A–1B
$$\Rarr\space\begin{bmatrix}x \\y \\z\end{bmatrix} =\frac{1}{4}\\\begin{bmatrix}7 &1 &\normalsize-3\\\normalsize-19 &\normalsize-1 &11\\\normalsize-11 &\normalsize-1 &7\end{bmatrix}\begin{bmatrix}7\\\normalsize-5\\12\end{bmatrix}\\=\frac{1}{4}\begin{bmatrix}49-5-36\\-133+5+132\\-77+5+84\end{bmatrix}\\\Rarr\space\begin{bmatrix}x\\y\\z\end{bmatrix} =\frac{1}{4}\begin{bmatrix}8\\4\\12\end{bmatrix}\\=\begin{bmatrix}2 \\1 \\3\end{bmatrix}.$$
Hence, x = 2, y = 1 and z = 3.
$$\textbf{15. If A =}\begin{bmatrix}\textbf{2} &\textbf{\normalsize-3} &\textbf{5}\\\textbf{3} &\textbf{2} &\textbf{\normalsize-4}\\\textbf{1} &\textbf{1} &\textbf{\normalsize-2}\end{bmatrix}\textbf{,}$$
find A–1. Using A–1 solve the system of equations 2x – 3y + 5z = 11, 3x + 2y – 4z = – 5, x + y – 2x = –3.
Sol. The given system can be written as AX = B, where
$$\text{A} =\begin{bmatrix}2 &\normalsize-3 &5\\3 &2 &\normalsize-4\\1 &1 &-2\end{bmatrix}\textbf{,}\\\text{X} =\begin{bmatrix}x\\y\\z\end{bmatrix}\space\text{and B} =\begin{bmatrix}11 \\\normalsize-5\\\normalsize-3\end{bmatrix}\\\therefore\space|\text{A}| =\begin{bmatrix}2 &\normalsize-3 &5\\3 &2 &\normalsize-4\\1 &1 &\normalsize-2\end{bmatrix}$$
= 2(– 4 + 4) – (– 3)(– 6 + 4) + 5(3 – 2)
= 0 – 6 + 5 = – 1 ≠ 0
Thus, A is non-singular. Therefore, its inverse exists.
Therefore, the given system is consistent and has a unique solution given by X = A–1B. Cofactors of A are
A11 = – 4 + 4 = 0, A12 = – (– 6 + 4) = 2, A13 = 3 – 2 = 1,
A21 = – ( 6 + 5) = – 1, A22 = – 4 – 5 = – 9, A23 = – (2 + 3) = – 5,
A31 = (12 – 10) = 2, A32 = – (– 8 – 15) = 23, A33 = 4 + 9 = 13
$$\text{adj(A)} =\begin{bmatrix}0 &2 &1\\\normalsize-1 &\normalsize-9 &\normalsize-5\\2 &23 &13\end{bmatrix}^{\text{T}}\\=\begin{bmatrix}0 &\normalsize-1 &2\\2 &\normalsize-9 &23\\1 &\normalsize-5 &13\end{bmatrix}\\\therefore\space\text{A}^{\normalsize-1}=\frac{1}{|\text{A}|}\space\text{(adj A)}\\=\frac{1}{\normalsize-1}\begin{bmatrix}0 &\normalsize-1 &2\\2 &\normalsize-9 &23\\1 &-5 &13\end{bmatrix}\\=\begin{bmatrix}0 &1 &\normalsize-2\\\normalsize-2 &9 &\normalsize-23\\\normalsize-1 &5 &\normalsize-13\end{bmatrix}$$
Now, X = A–1B
$$\Rarr\space\begin{bmatrix}x\\y\\z\end{bmatrix}=\begin{bmatrix}0 &1 &\normalsize-2\\\normalsize-2 &9 &\normalsize-23\\\normalsize-1 &5 &\normalsize-13\end{bmatrix}\begin{bmatrix}11\\\normalsize-5\\\normalsize-3\end{bmatrix}$$
$$\Rarr\space\begin{bmatrix}x\\y\\z\end{bmatrix}=\begin{bmatrix}0-5+6\\-22-45+69\\-11-25+39\end{bmatrix}=\begin{bmatrix}1\\2\\3\end{bmatrix}.$$
Hence, x = 1, y = 2 and z = 3.
16. The cost of 4 kg onion, 3 kg wheat and 2 kg rice is ₹ 60. The cost of 2 kg onion, 4 kg wheat and 6 kg rice is ₹ 90. The cost of 6 kg onion, 2 kg wheat and 3 kg rice is ₹70. Find cost of each item per kg by matrix method.
Sol. Let the prices (per kg) of onion, wheat and rice be ₹x, ₹y and ₹z, respectively then
4x + 3y + 2z = 60, 2x + 4y + 6z = 90, 6x + 2y + 3z = 70
The system of equations can be written as AX = B, where
$$\text{A} =\begin{bmatrix}4 &3 &2\\2 &4 &6\\6 &2 &3\end{bmatrix},\text{X} =\begin{bmatrix}x\\y\\z\end{bmatrix}\\\space\text{and B =}\begin{bmatrix}60\\90\\70\end{bmatrix}\\\text{Here,\space|A|} =\begin{vmatrix}4 &3 &2\\2 &4 &6\\6 &2 &3\end{vmatrix}$$
= 4(12 – 12) – 3(6 – 36) + 2(4 – 24)
= 0 + 90 – 40 = 50 ≠ 0
Thus, A is non-singular. Therefore, its inverse exists. Therefore, the given system is consistent and has a unique solution given by X = A–1B.
Cofactors of A are,
A11 = 12 – 12 = 0, A12 = – (6 – 36) = 30, A13 = 4 – 24 = – 20,
A21 = – (9 – 4)= – 5, A22 = 12 – 12 = 0, A23 = – (8 – 18) = 10,
A31 = (18 – 8) = 10, A32 = – (24 – 4) = – 20, A33 = 16 – 6 = 10
$$\text{adj(A) = }\begin{bmatrix}0 &30 &-20\\\normalsize-5 &0 &10\\10 &\normalsize-20 &10\end{bmatrix}^{\text{T}}\\=\begin{bmatrix}0 &-5 &10\\30 &0 &\normalsize-20\\\normalsize-20 &10 &10\end{bmatrix}\\\therefore\space\text{A}^{\normalsize-1}=\frac{1}{|\text{A}|}\space\text{(adj A)}\\=\frac{1}{\normalsize-1}\begin{bmatrix}0 &\normalsize-1 &2\\2 &\normalsize-9 &23\\1 &\normalsize-5 &13\end{bmatrix}\\=\begin{bmatrix}0 &1 &\normalsize-2\\\normalsize-2 &9 &23\\\normalsize-1 &5 &\normalsize-13\end{bmatrix}$$
Now, X = A–1B
$$\Rarr\space\begin{bmatrix}x\\y\\z\end{bmatrix}=\begin{bmatrix}0 &1 &\normalsize-2\\\normalsize-2 &9 &23\\1 &\normalsize-5 &13\end{bmatrix}\begin{bmatrix}11\\\normalsize-5\\\normalsize-3\end{bmatrix}\\\Rarr\space\begin{bmatrix}x\\y\\z\end{bmatrix} =\begin{bmatrix}0-5+6\\-22-45+69\\-11-25+39\end{bmatrix}=\begin{bmatrix}1 \\2 \\3\end{bmatrix}.$$
Hence, x = 1, y = 2 and z = 3.
$$\Rarr\space\begin{bmatrix}x\\y\\z\end{bmatrix} =\frac{1}{50}\\\begin{bmatrix}0 &-5 &10\\30 &0 &-20\\\normalsize-20 &10 &10\end{bmatrix}\begin{bmatrix}60\\90\\70\end{bmatrix}\\=\frac{1}{30}\begin{bmatrix}0-450+700\\1800+0-1400\\-1200+900+700\end{bmatrix}\\\Rarr\space\begin{bmatrix}x\\y\\z\end{bmatrix}=\frac{1}{50}\begin{bmatrix}250\\400\\400\end{bmatrix}=\begin{bmatrix}5\\8\\9\end{bmatrix}\\\Rarr\space\begin{bmatrix}x\\y\\z\end{bmatrix} =\begin{bmatrix}5\\8\\8\end{bmatrix}$$
∴ x = 5, y = 8 and z = 8. Hence, price of onion per kg is ₹5, price of wheat per kg is ₹8 and that of rice per kg is ₹8.
