NCERT Solutions for Class 12 Maths Chapter 9 - Differential Equations
NCERT Solutions for Class 12 Mathematics Chapter 9 Free PDF Download
Please Click on Free PDF Download link to Download the NCERT Solutions for Class 12 Mathematics Chapter 9 Differential Equations
The dot mark ◉ field are mandatory, So please fill them in carefully
To download the complete Syllabus (PDF File), Please fill & submit the form below.
To download the complete Syllabus (PDF File), Please fill & submit the form below.
https://drive.google.com/file/d/1l9IVV9q1QPy3xo0MtduOTh4ii-dt9ARY/view?usp=sharing






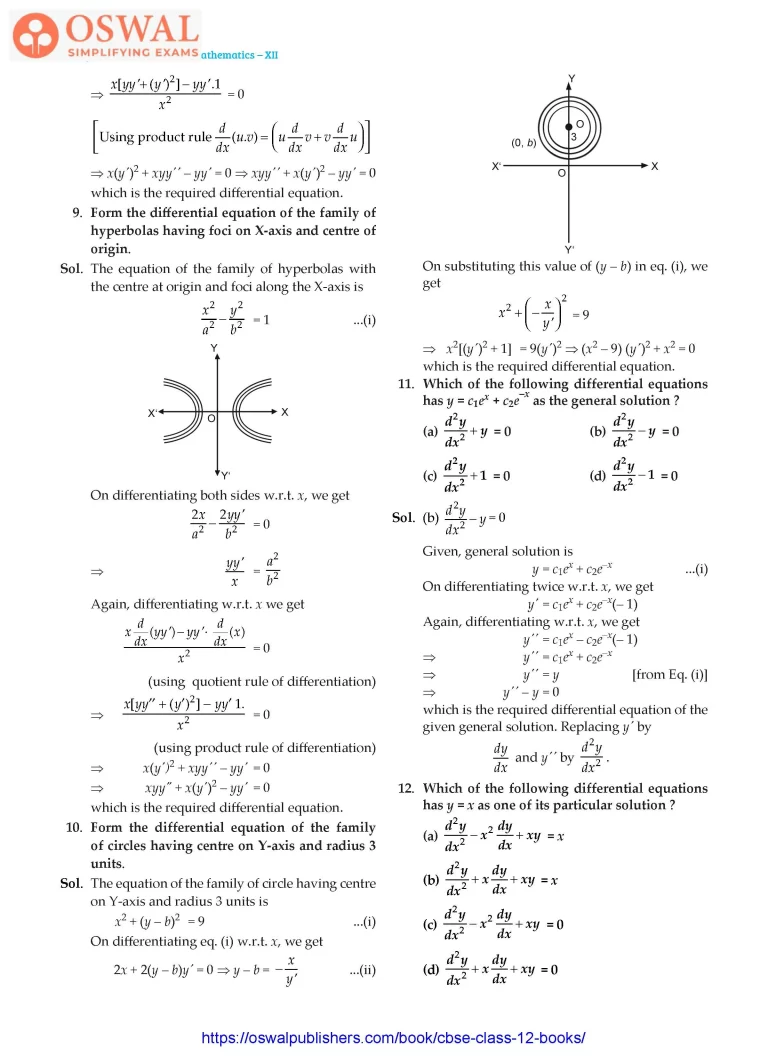




























Access Exercises of Class 12 Maths Chapter 9 – Differential Equations
Exercise 9.1 Solutions 12 Questions
Exercise 9.2 Solutions 12 Questions
Exercise 9.3 Solutions 12 Questions
Exercise 9.4 Solutions 23 Questions
Exercise 9.5 Solutions 17 Questions
Exercise 9.6 Solutions 19 Questions
Miscellaneous Exercise on Chapter 9 Solutions 18 Questions
