NCERT Solutions for Class 12 Maths Chapter 9 - Differential Equations -Exercise 9.3



Access Exercises of Class 12 Maths Chapter 9 – Differential Equations
Exercise 9.1 Solutions 12 Questions
Exercise 9.2 Solutions 12 Questions
Exercise 9.3 Solutions 12 Questions
Exercise 9.4 Solutions 23 Questions
Exercise 9.5 Solutions 17 Questions
Exercise 9.6 Solutions 19 Questions
Miscellaneous Exercise on Chapter 9 Solutions 18 Questions
Exercise 9.3
In each of the question 1 to 5, form a differential equation representing the given family of curves by eliminating arbitrary constants a and b.
$$\textbf{1.\space}\frac{\textbf{x}}{\textbf{a}} + \frac{\textbf{y}}{\textbf{b}} \textbf{= 1.}$$
Sol. Given, equation is
$$\frac{x}{a}+\frac{y}{b}=1\qquad\text{...(i)}$$
On differentiating both sides w.r.t. x, we get
$$\frac{1}{a}+\frac{1}{b}\frac{dy}{dx} = 0.$$
Again differentiating both sides with respect to x, we get
$$0 + \frac{1}{b}\frac{d^{2}y}{dx} = 0\\\Rarr\space y'' =0$$
which is the required differential equation.
Note : Differentiate the given equation as many number of times as the number of arbitrary constants present in the equation.
2. y2 = a(b2 – x2).
Sol. Given equation is
y2 = a(b2 – x2) ...(i)
On differentiating w.r.t. x we get
2yy' = a(0 – 2x)
$$\Rarr\space 2yy'= a(0-2x)\\\Rarr\space yy'=-ax\space\text{...(ii)}$$
Again differentiating w.r.t. x, we get
yy' + (y')2 = – a ...(iii)
(using product rule of differentiation)
Now, for eliminating a, put the value of a from eq. (iii) in eq. (ii), we get
yy' = [yy'' + (y')2]x
$$\Rarr\space yy'= x\lbrack(y')^{2}+yy'\rbrack $$
which is the required differential equation.
3. y = ae3x + be–2x.
Sol. Given, is equation y = ae3x + be– 2x ...(i)
On differentiating w.r.t. x, we get
$$\frac{dy}{dx} = a\frac{d}{dx}(e^{3x}) + b\frac{d}{dx}(e^{-2x})\\\Rarr\space \frac{dy}{dx} = ae^{3x}\frac{d}{dx}(3x) + be^{\normalsize-2x}\frac{d}{dx}(-2x)\\\Rarr\space\frac{dy}{dx} = 3ae^{3x}-2be^{-2x}\space\text{...(ii)}$$
Again differentiating w.r.t. x, we get
$$\frac{d^{2}y}{dx^{2}}=3ae^{3x}\frac{d}{dx}(3x)-2be^{-2x}\frac{d}{dx}(-2x)$$
= 9ae3x + 4be– 2x
$$\Rarr\space\frac{d^{2}y}{dx^{2}}=3(3ae^{3x}) + 4be^{\normalsize-2x}\\\Rarr\space \frac{d^{2}y}{dx^{2}}=3\bigg(\frac{dy}{dx}+2be^{-2x}\bigg) + 4be^{\normalsize-2x}\\\bigg(\because\frac{dy}{dx} + 2be^{-2x} = 3ae^{3x}\bigg)\\\Rarr\space \frac{d^{2}y}{dx^{2}}=3\frac{dy}{dx} + 6be^{-2x} + 4 be^{\normalsize-2x}\space\text{...(iii)}\\\Rarr\space\frac{d^{2}y}{dy^{2}}=3\frac{dy}{dx} + 10be^{\normalsize-2x}$$
On multiplying eq. (i) by 3 and then subtracting from eq. (ii), we get
$$\frac{dy}{dx}-3y = -2be^{\normalsize-2x}-3be^{-2x}\\\Rarr\space 3y-\frac{dy}{dx} = 5be^{\normalsize-2x}\\\Rarr\space b=\frac{3y - \frac{dy}{dx}}{5e^{\normalsize-2x}}\space\text{...(iv)}$$
On putting the value of b in eq. (iii), we get
$$\frac{d^{2}y}{dx^{2}} = 3\frac{dy}{dx} + 10\frac{3y - \frac{dy}{dx}}{5 e^{\normalsize-2x}}.e^{\normalsize-2x}\\\Rarr\space \frac{d^{2}y}{dx^{2}} = 3\frac{dy}{dx} + 2\bigg(3y - \frac{dy}{dx}\bigg)\\\Rarr\space \frac{d^{2}y}{dx^{3}}=3\frac{dy}{dx} + 6y-2\frac{dy}{dx}\\\Rarr\space \frac{d^{2}y}{dx^{2}} = \frac{dy}{dx} + 6y\\\Rarr\space \frac{d^{2}y}{dx^{2}}-\frac{dy}{dx}-6y = 0$$
which is the required differential equation.
4. y = e2x(a + bx).
Sol. Given, y = e2x(a + bx) ...(i)
On differentiating both sides w.r.t. x, we get
$$\frac{dy}{dx} = e^{2x}\frac{d}{dx}(a+bx) + (a + bx)\frac{d}{dx}e^{2x}$$
(using product rule of differentiation)
$$\Rarr\space\frac{dy}{dx} = e^{2x}(b) + 2e^{2x}(a+bx)\\\Rarr\space y' = 2y + be^{2x}\space\\\lbrack\text{using Eq. (i)}\rbrack\text{...(ii)} $$
Again differentiating eq. (ii) w.r.t. x, we get
y'' = 2y' + 2be2x ...(iii)
On multiplying eq. (ii) by 2 and then subtracting form eq. (iii), we get
y'' – 2y' = 2(y' – 2y)
$$\Rarr\space y'' - 2y' = 2y'-4y\\\Rarr\space y''- 4y + 4y = 0$$
Which is the required differential equatinon.
5. y = ex(a cos x + b sin x).
Sol. Given, y = ex(a cos x + b sin x) ...(i)
On dividing both sides by ex,
e–x y = (a cos x + b sin x) ...(ii)
On differentiating both sides w.r.t. x, we get
e–xy' + ye–x (– 1) = – a sin x + b cos x
(Using product rule,
$$\frac{d}{dx}(u.v)=u\frac{d}{dx}v + v\frac{d}{dx}u)$$
Again, differentiating both sides w.r.t. x, we get
$$e^{\normalsize-x}\frac{d}{dx}(y') + y'\frac{d}{dx}(e^{\normalsize-x})-\\\bigg[y\frac{d}{dx}e^{\normalsize-x} + e^{\normalsize-x}\frac{d}{dx}y\bigg]$$
= – a cos x – b sin x
$$\Rarr\space e^{-x}y'' + y'e^{\normalsize-x}(\normalsize-1)-\lbrack y e^{\normalsize-x}(\normalsize 1) + e^{\normalsize-x}y'\rbrack\\=\text{-a cos x - b sin x}\\\Rarr\space e^{\normalsize-x}y''-2y'e^{\normalsize-x} + ye^{\normalsize-x} = -(ye^{\normalsize-x})\\\lbrack\text{using eq. (ii)}\rbrack\\\Rarr\space e^{\normalsize-x}\lbrack y'' - 2y' + 2y\rbrack=0\\\Rarr\space y'' - 2y' + 2y = 0$$
(Dividing by e–x)
which is the required differential equation.
6. Form the differential equation of the family of circles touching the Y-axis at origin.
Sol. The centre of the circle touching the Y-axis at origin lines on the X-axis.
Let (a, 0) be the centre of the circle and its radius is a.
Now, the equation of the family of circle with centre (a, 0) and radius (a) is
(x – a)2 + y2 = a2
$$\Rarr\space x^{2}+y^{2}=2ax\space\text{...(i)}$$

On differentiating eq. (i) w.r.t. x, we get
2x + 2yy' = 2a
$$\Rarr\space x + yy' = a$$
Now, substituting the value of a in eq. (i), we get
x2 + y2 = 2(x + yy')x
$$\Rarr\space x^{2} + y^{2} = 2x^{2} + 2xyy'\\\Rarr\space 2xyy' + x^{2} = y^{2}$$
which is the required differential equation.
7. Form the differential equation of the family of parabolas having vertex at origin and axis along positive Y-axis.
Sol. The equation of the family of parabola having the vertex at origin and the axis along the positive Y. axis is
x2 = 4ay ...(i)

On differentiating eq. (i) w.r.t. x, we get
2x = 4ay' ...(ii)
On dividing eq. (ii) by eq. (i), we get
$$\frac{2x}{x^{2}}=\frac{4ay'}{4ay}\\\Rarr\space \frac{2}{x} = \frac{y'}{y}\\\Rarr\space xy' = 2y\\\Rarr\space xy'-2y=0$$
which is the required differential equation.
8. Form the differential equation of the family of ellipse having foci on Y-axis and centre at origin.
Sol. Equation of family of ellipse is of the form
$$\frac{x^{2}}{b^{2}} + \frac{y^{2}}{a^{2}} = 1\space\text{...(i)}$$
[Since, foci is on Y-axis, so we draw a vertical ellipse]
On differentiating eq. (i) w.r.t. x, we get
$$\frac{d}{dx}\bigg(\frac{x^{2}}{b^{2}}\bigg)+\frac{d}{dx}\bigg(\frac{y^{2}}{a^{2}}\bigg)=\frac{d}{dx}(1)\space\text{...(ii)}\\\Rarr\space \frac{1}{b^{2}}2x + \frac{1}{a^{2}}2yy'= 0\\\frac{yy'}{x}=-\frac{a^{2}}{b^{2}}$$

Again differentiating w.r.t. x, we get
$$\Rarr\space\frac{x\frac{d}{dx}(yy')-yy'\frac{d}{dx}(x)}{x^{2}}=0\\\begin{pmatrix}\text{using quotient rule}\\\frac{d}{dx}\bigg(\frac{u}{v}\bigg) = \frac{v\frac{d}{dx}(u)- u\frac{d}{dx}(v)}{v^{2}}\end{pmatrix}\\\Rarr\space\frac{x \lbrack yy' + (y')^{2}\rbrack - yy'.1}{x^{2}}=0\\\begin{bmatrix}\text{Using product rule}\\\frac{d}{dx}(u.v) =\bigg(u \frac{d}{dx}v + v\frac{d}{dx}u\bigg)\end{bmatrix}\\\Rarr\space x(y')^{2} + xyy' = 0\\\Rarr\space xyy' + x(y')^{2} - yy'=0$$
which is the required differential equation.
9. Form the differential equation of the family of hyperbolas having foci on X-axis and centre of origin.
Sol. The equation of the family of hyperbolas with the centre at origin and foci along the X-axis is
$$\frac{x^{2}}{a} - \frac{y^{2}}{b^{2}}=1\space\text{...(i)}$$

On differentiating both sides w.r.t. x, we get
$$\frac{2x}{a^{2}}-\frac{2yy'}{b^{2}}=0\\\Rarr\space \frac{yy'}{x}=\frac{a^{2}}{b^{2}}$$
Again, differentiating w.r.t. x we get
$$\frac{x\frac{d}{dx}(yy') - yy'.\frac{d}{dx}(x)}{x^{2}}=0$$
(using quotient rule of differentiation)
$$\Rarr\space\frac{x\lbrack yy' + (y')^{2}\rbrack - yy' 1.}{x^{2}}=0\\\text{(using product rule of differentiation)}\\\Rarr\space x(y')^{2} + xyy' - yy'=0\\\Rarr\space xyy" + x(y')^{2} - yy'=0$$
which is the required differential equation.
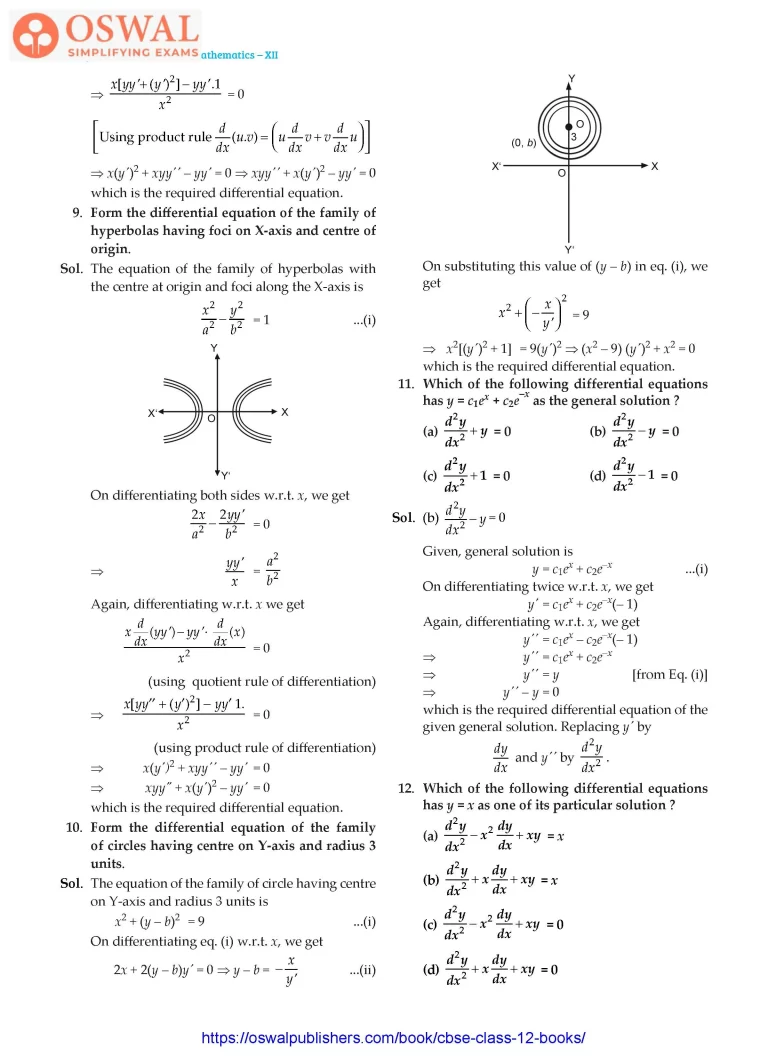
10. Form the differential equation of the family of circles having centre on Y-axis and radius 3 units.
Sol. The equation of the family of circle having centre on Y-axis and radius 3 units is
x2 + (y – b)2 = 9 ...(i)
On differentiating eq. (i) w.r.t. x, we get
2x + 2(y – b)y' = 0
$$\Rarr\space y-b = -\frac{x}{y'}\space\text{...(ii)}$$

On substituting this value of (y – b) in eq. (i), we get
$$x^{2} + \bigg(-\frac{x}{y}\bigg)^{2}=9\\\Rarr\space x^{2}\lbrack(y')^{2}+1\rbrack =9(y')^{2}\\\Rarr\space (x^{2}-9)(y')^{2} + x^{2}=0$$
which is the required differential equation.
11. Which of the following differential equations has y = c1ex + c2e–x as the general solution ?
$$\textbf{(a)\space}\frac{\textbf{d}^{\textbf{2}}\textbf{y}}{\textbf{dx}^{\textbf{2}}}\textbf{\space+ y=0}\\\textbf{(b)\space}\frac{\textbf{d}^{\textbf{2}}\textbf{y}}{\textbf{dx}^{\textbf{2}}}\textbf{-y=0}\textbf{= 0}\\\textbf{(c)\space}\frac{\textbf{d}^{\textbf{2}}\textbf{y}}{\textbf{dx}^{\textbf{2}}}\textbf{+ 1=0}\\\textbf{(d)\space}\frac{\textbf{d}^{\textbf{2}}\textbf{y}}{\textbf{dx}^{\textbf{2}}}\textbf{-1 = 0}\\\textbf{Sol.\space}\text{(b)\space}\frac{d^{2}y}{dx^{2}}-y=0$$
Given, general solution is
y = c1ex + c2e–x ...(i)
On differentiating twice w.r.t. x, we get
y' = c1ex + c2e–x(– 1)
Again, differentiating w.r.t. x, we get
y'' = c1ex – c2e–x(– 1)
$$\Rarr\space y'' = c_1e^{x} + c_2e^{\normalsize-x}\\\Rarr\space y'' = y\space\lbrack\text{from Eq. (i)}\rbrack\\\Rarr\space y'' - y = 0$$
which is the required differential equation of the given general solution. Replacing y' by
$$\frac{dy}{dx}\space\text{and}\space y''\text{by}\frac{d^{2}y}{dx^{2}}.$$
12. Which of the following differential equations has y = x as one of its particular solution ?
$$\textbf{(a)\space}\frac{\textbf{d}^{\textbf{2}}\textbf{y}}{\textbf{dx}^{2}}-\textbf{x}^{\textbf{2}}\frac{\textbf{dy}}{\textbf{dx}}\textbf{ + xy = x}\\\textbf{(b)\space}\frac{\textbf{d}^{\textbf{2}}\textbf{y}}{\textbf{dx}^{\textbf{2}}}\textbf{+ x}\frac{\textbf{dy}}{\textbf{dx}} \textbf{+ xy = x}\\\textbf{(c)\space}\frac{\textbf{d}^{\textbf{2}}\textbf{y}}{\textbf{dx}^{\textbf{2}}}\textbf{- x}^{\textbf{2}}\frac{\textbf{dy}}{\textbf{dx}} \textbf{+ xy = 0}\\\textbf{(d)\space}\frac{\textbf{d}^{\textbf{2}}\textbf{y}}{\textbf{dx}^{\textbf{2}}}\textbf{+x}\frac{\textbf{dy}}{\textbf{dx}}\textbf{ + xy = 0}\\\textbf{Sol.\space}\text{(c)\space}\frac{d^{2}y}{dx^{2}}-x^{2}\frac{dy}{dx} + xy = 0$$
Given solution is
y = x ...(i)
On differentiating w.r.t.x, we get
$$\frac{dy}{dx} = 1\space\text{...(ii)}$$
Again, differentiating w.r.t. x, we get
$$\frac{d^{2}y}{dx^{2}} = 0\space\text{...(iii)}$$
Now, on substituting the values from eqs. (i), (iii) and (ii) in each of the given option, we find that only the differential equation given in option (c) is satisfied by the values of y
$$\frac{dy}{dx}\text{and}\space\frac{d^{2}y}{dx^{2}}.\\\frac{d^{2}y}{dx}-x^{2}\frac{dy}{dx}+xy\\=0 - x^{2}.1 + x.x$$
= – x2 + x2 = 0.
