NCERT Solutions for Class 12 Maths Chapter 1 Relations and Functions - Exercise 1.4
Access Exercises of Class 12 Maths Chapter 1 - Relations and Functions
Exercise 1.1 Solutions : 16 Questions (14 Short Answers, 2 MCQ)
Exercise 1.2 Solutions : 12 Questions (10 Short Answers, 2 MCQ)
Exercise 1.3 Solutions : 14 Questions (12 Short Answers, 2 MCQ)
Exercise 1.4 Solutions : 13 Questions (12 Short Answers, 1 MCQ)
Miscellaneous Exercise Solutions: 19 Questions (7 Long answers, 9 Short Answer Type, 3 MCQs)
Exercise 1.4 :
1. Determine whether or not each of the definition of * given below gives a binary operation. In the event that * is not a binary operation, give justification for this.
(i) On Z+, define * by a * b = a – b
(ii) On Z+, define * by a * b = ab
(iii) On R, define * by a * b = ab2
(iv) On Z+, define * by a * b = |a – b|
(v) On Z+, define * by a * b = a
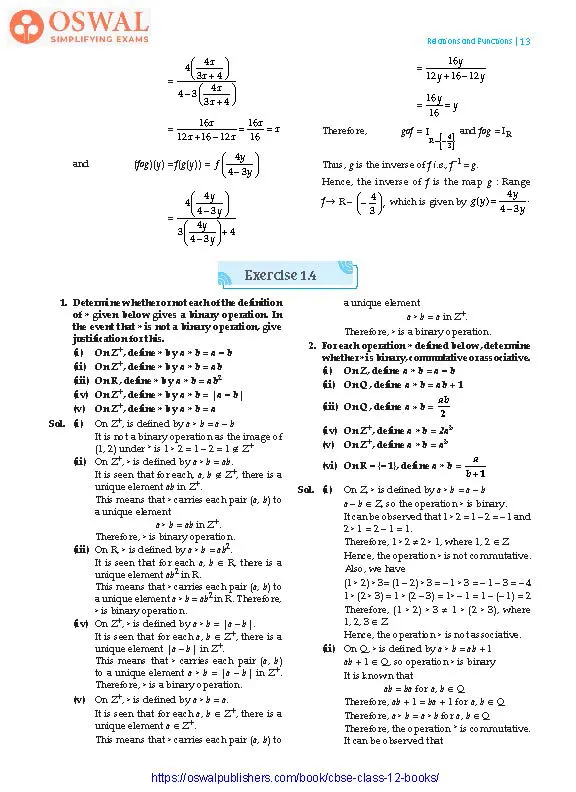
Sol. (i) On Z+, is defined by a * b = a – b
It is not a binary operation as the image of (1, 2) under * is 1 * 2 = 1 – 2 = 1 ∉ Z+
(ii) On Z+, * is defined by a * b = ab.
It is seen that for each, a, b ∉ Z+, there is a unique element ab in Z+.
This means that * carries each pair (a, b) to a unique element
a * b = ab in Z+.
Therefore, * is binary operation.
(iii) On R, * is defined by a * b = ab2.
It is seen that for each a, b ∈ R, there is a
unique element ab2 in R.
This means that * carries each pair (a, b) to a unique element a * b = ab2 in R. Therefore, * is binary operation.
(iv) On Z+, * is defined by a * b = |a – b|.
It is seen that for each a, b ∈ Z+, there is a unique element |a – b| in Z+.
This means that * carries each pair (a, b) to a unique element a * b = |a – b| in Z+.
Therefore, * is a binary operation.
(v) On Z+, * is defined by a * b = a.
It is seen that for each a, b ∈ Z+, there is a unique element a ∈ Z+.
This means that * carries each pair (a, b) to a unique element
a * b = a in Z+.
Therefore, * is a binary operation.
2. For each operation * defined below, determine whether * is binary, commutative or associative.
(i) On Z, define a * b = a – b
(ii) On Q, define a * b = ab + 1
$$\textbf{(iii) On Q, define a * b =}\space\frac{\textbf{ab}}{\textbf{2}}$$
(iv) On Z+, define a * b = 2ab
(v) On Z+, define a * b = ab
$$\textbf{(vi) On R – }\lbrace \textbf{\normalsize– 1}\rbrace\textbf{,}\space\\\textbf{define a * b =}\frac{\textbf{a}}{\textbf{b+1}}$$
Sol. (i) On Z, * is defined by a * b = a – b
a – b ∈ Z, so the operation * is binary.
It can be observed that 1 * 2 = 1 – 2 = – 1 and
2 * 1 = 2 – 1 = 1.
Therefore, 1 * 2 ≠ 2 * 1, where 1, 2 ∈ Z
Hence, the operation * is not commutative.
Also, we have
(1 * 2) * 3 = (1 – 2) * 3 = – 1 * 3 = – 1 – 3 = – 4
1 * (2 * 3) = 1 * (2 – 3) = 1* – 1 = 1 – (– 1) = 2
Therefore, (1 * 2) * 3 ≠ 1 * (2 * 3), where
1, 2, 3 ∈ Z
Hence, the operation * is not associative.
(ii) On Q, * is defined by a * b = ab + 1
ab + 1 ∈ Q, so operation * is binary
It is known that
ab = ba for a, b ∈ Q
Therefore, ab + 1 = ba + 1 for a, b ∈ Q
Therefore, a * b = a * b for a, b ∈ Q
Therefore, the operation * is commutative.
It can be observed that
(1 * 2) * 3 = (1 × 2 + 1) * 3 = 3 * 3 = 3 × 3 + 1 = 10
1 * (2 * 3) = 1 * (2 × 3 + 1) = 1 * 7 = 1 × 7 + 1 = 8
Therefore, (1 * 2) * 3 ≠ 1 * (2 * 3), where 1, 2, 3 ∈ Q
Therefore, the operation * is not associative.
$$\textbf{(iii)} \text{On Q, *is defined by a b a*b =}\frac{ab}{2}.\\\frac{ab}{2}\epsilon\text{Q},\space\text{so the operation * is binary.}$$
It is known that
ab = ba for a, b ∈ Q
$$\text{Therefore,}\space\frac{ab}{2} = \frac{ba}{2}\space\text{for a, b ∈ Q} $$
Therefore, a * b = b * a for a, b ∈ Q
Therefore, the operation * is commutative.
For all a, b, c ∈ Q, we have
$$\text{(a*b)*c} =\bigg(\frac{ab}{2}\bigg)*c\\=\frac{\bigg(\frac{ab}{2}\bigg)c}{2} =\frac{abc}{4}\\ a*(b*c) = a*\bigg(\frac{bc}{2}\bigg) \\=\frac{a\bigg(\frac{bc}{2}\bigg)}{2} =\frac{abc}{4}$$
Therefore, (a * b) * c = a * (b * c)
Therefore, the operation * is associative.
(iv) On Z+, * is defined by a * b = 2ab.
2ab ∈ Z+, so the operation * is binary operation
It is known that
ab = ba for a, b ∈ Z+
Therefore, 2ab = 2ba for a, b ∈ Z+
Therefore, a * b = b * a for a, b ∈ Z+
Therefore, the operation * is commutative.
It can be observed that
(1 * 2) * 3 = 2(1 × 2) * 3 = 4 * 3 = 24 × 3 = 24 × 3 = 212
1 * (2 * 3) = 1 * 22 × 3 = 1 * 26 = 1 * 64 = 264
Therefore, the operation * is not associative.
(v) On Z+, * is defined by a * b = ab,
ab ∈ Z+, so the operation * is binary opertion.
It can be observed that 1 * 2 = 12 = 1 and 2 * 1 = 21 = 2
Therefore, 1 * 2 ≠ 2 * 1 where 1 , 2 ∈ Z+
Therefore, the operation * is not commutative.
It can also be observed that
(2 * 3) * 4 = 23 * 4 = 8 * 4 = 84 = (23)4 = 212
2 * (3 * 4) = 2 * 34 = 2 * 81 = 281
Therefore, (2 * 3 ) * 4 ≠ 2 * (3 * 4); where 2,
3, 4 ∈ Z+
Therefore, the operation * is not associative.
(vi) On R – {– 1}, * is defined by
$$a*b = \frac{a}{b+1}.\\\frac{a}{b+1}\epsilon \text{R}\space\text{for b} ≠ – 1$$
so that operation * is binary.
$$\text{It can be observed that}\\1 * 2 = \frac{1}{2+1}=\frac{1}{3}\space\text{and}\space\\2*1 =\frac{2}{1 + 1} =\frac{2}{2}= 1$$
Therefore, 1 * 2 ≠ 2 * 1 where 1, 2 ∈ R – {– 1} Therefore, the operation * is not commutative,
It can also be observed that
$$(1*2)*3 =\frac{1}{3}*3\\=\frac{\frac{1}{3}}{3+1} =\frac{1}{12}\\1 *(2*3) = 1*\frac{2}{3+1} = 1*\frac{2}{4}\\= 1*\frac{1}{2} =\frac{1}{\frac{1}{2}+1} =\frac{1}{\frac{3}{2}}=\frac{2}{3}$$
Therefore, (1 * 2) * 3 ≠ 1 * (2 * 3) where 1, 2, 3 ∈ R – {– 1}.
Therefore, the operation * is not associative.
3. Consider the binary operation ^ on the set {1, 2, 3, 4, 5} defined by a ^ b = min {a, b}. Write the operation table of the operation.
Sol. The binary operation * on the set {1, 2, 3, 4, 5} is defined as a ^ b = min {a, b} for a, b ∈ {1, 2, 3, 4,
5}.
Thus, the operation table for the given operation ^ can be given as
| ^ | 1 | 2 | 2 | 4 | 5 |
| 1 | 1 | 1 | 1 | 1 | 1 |
| 2 | 1 | 2 | 2 | 2 | 2 |
| 3 | 1 | 2 | 3 | 3 | 3 |
| 4 | 1 | 2 | 3 | 4 | 4 |
| 5 | 1 | 2 | 3 | 4 | 5 |
4. Consider a binary operation * on set {1, 2, 3, 4, 5} given by the following multiplication table:
(i) Compute (2 * 3) * 4 and 2 * (3 * 4)
(ii) Is * commutative?
(iii) Compute (2 * 3) * (4 * 5).
| * | 1 | 2 | 3 | 4 | 5 |
| 1 | 1 | 1 | 1 | 1 | 1 |
| 2 | 1 | 2 | 1 | 2 | 1 |
| 3 | 1 | 1 | 3 | 1 | 1 |
| 4 | 1 | 2 | 1 | 4 | 1 |
| 5 | 1 | 1 | 1 | 1 | 5 |
Sol. (i) We have (2 * 3) * 4 = (1) * 4 = 1
and 2 * (3 * 4) = 2 * 1 = 1
(ii) For every a, b ∈ {1, 2, 3, 4, 5}, we have a * b = b * a.
Therefore, the operation * is commutative.
(iii) We have (2 * 3) = 1 and (4 * 5) = 1
Therefore, (2 * 3) * (4 * 5) = 1 * 1 = 1
5. Let * be the binary operation of the set {1, 2, 3, 4, 5} defined by a * b = HCF of a and b. Is the operation * same as the operation * defined in Q. 4 above? Justify your answer.
Sol. The binary operation *, on the set {1, 2, 3, 4, 5} is defined as a * b = HCF of a and b, the operation table for the operation * can be given as
| * | 1 | 2 | 3 | 4 | 5 |
| 1 | 1 | 1 | 1 | 1 | 1 |
| 2 | 1 | 2 | 1 | 2 | 1 |
| 3 | 1 | 1 | 3 | 1 | 1 |
| 4 | 1 | 2 | 1 | 4 | 1 |
| 5 | 1 | 1 | 1 | 1 | 5 |
We observe that the operation table for the operation * and operation * in Q. 4 are the same. Thus, the operation *, is same as the operation *.
6. Let * be the binary operation on N given by
a * b = LCM of a and b.
(i) 5 * 7, 20 * 16
(ii) Is * commutative?
(iii) Is * associative ?
(iv) Find the identity of * in N
(v) Which elements of N are invertible for the operation * ?
Sol. The binary operation * on N is defined as a * b
= LCM of a and b.
(i) We have 5 * 7 = LCM of 5 and 7 = 35
and 20 * 16 = LCM of 20 and 16 = 80
(ii) It is known that
LCM of a and b = LCM of b and a for a, b ∈ N.
Therefore, a * b = b * a. Thus, the operation * is commutative.
(iii) For a, b, c ∈ N, we have
(a * b) * c = (LCM of a and b) * c = LCM of a, b and c
a * (b * c) = a * (LCM of b and c) = LCM of a, b and c
Therefore, (a * b) * c = a * (b * c). Thus, the operation * is associative.
(iv) It is known that
LCM of a and 1 = a = LCM of 1 and a, a ∈ N
a * 1 = a = 1 * a, a ∈ N
Thus, 1 is the identity of * in N.
(v) An element a in N is invertible with respect to the operation *, if there exists an element b in N such that a * b = e = b * a.
Here, e = 1. This means that
LCM of a and b = 1 = LCM of b and a.
This case is possible only when a and b are equal to 1.
Thus, 1 is the only invertible element of N with respect to the operation *.
7. Is * defined on the set {1, 2, 3, 4, 5} by a * b = LCM of a and b a binary operation? Justify your answer.
Sol. The operation * on the set A = {1, 2, 3, 4, 5} is defined as a * b = LCM of a and b. Now, 2 * 3 = LCM of 2 and 3 = 6. But 6 does not belong to the given set. Hence, the given operation * is not a binary operation.
8. Let * be the binary operation on N defind by a * b = HCF of a and b. Is * commutative?
Is * associative ? Does there exist identity for this binary operation on N?
Sol. The binary operation * on N is defined as a * b = HCF of a and b.
It is known that HCF of a and b = HCF of b and a for a, b ∈ N.
Therefore, a * b = b * a. Thus, the operation * is commutative.
For a, b, c ∈ N, we have (a * b) * c = (HCF of a and b) * c = HCF of a, b and c.
a * (b * c) = a * (HCF of b and c) = HCF of a, b and c
Therefore, (a * b) * c = a * (b * c)
Thus, the operation * is associative.
Now, an element e ∈ N will be the identity for the operation * if a * e = a = e * a, for ∀ a ∈ N. But this relation is not true for any a ∈ N.
Thus, the operation * does not have identity in N.
9. Let * be a binary operation on the set Q of rational number as follows :
(i) a * b = a – b
(ii) a * b = a2 + b2
(iii) a * b = a + ab
(iv) a * b = (a – b)2
$$\textbf{(v)\space}\textbf{a*b =}\frac{\textbf{ab}}{\textbf{4}}$$
(vi) a * b = ab2
Find which of the binary operations are commutative and which are associative.
Sol. (i) On Q, the operation * is defined as a * b = a – b.
It can be observed that for 2, 3, 4 ∈ Q, we have
2 * 3 = 2 – 3 = – 1 and 3 * 2 = 3 – 2 = 1
2 * 3 ≠ 3 * 2
Thus, the operation * is not commutative.
It can also be observed that
(2 * 3) * 4 = (– 1) * 4 = – 1 – 4= – 5
and 2 * (3 * 4) = 2 * (– 1) = 2 – (– 1) = 3
Thus, the operation * is not associative.
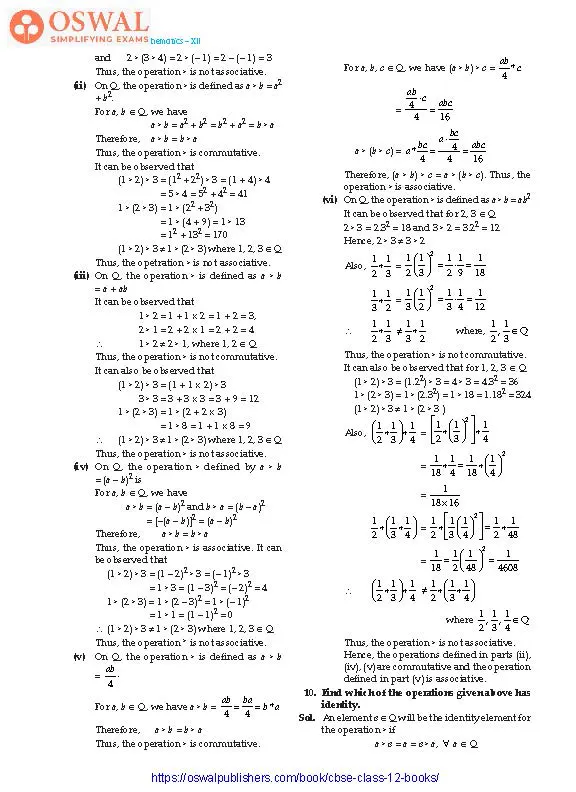
(ii) On Q, the operation * is defined as a * b = a2 + b2.
For a, b ∈ Q, we have
a * b = a2 + b2 = b2 + a2 = b * a
Therefore, a * b = b * a
Thus, the operation * is commutative.
It can be observed that
(1 * 2) * 3 = (12 + 22) * 3 = (1 + 4) * 4
= 5 * 4 = 52 + 42 = 41
1 * (2 * 3) = 1 * (22 + 32)
= 1 * (4 + 9) = 1 * 13
= 12 + 132 = 170
(1 * 2) * 3 ≠ 1 * (2 * 3) where 1, 2, 3 ∈ Q
Thus, the opetration * is not associative.
(iii) On Q, the operation * is defined as a * b
= a + ab
It can be observed that
1 * 2 = 1 + 1 × 2 = 1 + 2 = 3,
2 * 1 = 2 + 2 × 1 = 2 + 2 = 4
∴ 1 * 2 ≠ 2 * 1, where 1, 2 ∈ Q
Thus, the operation * is not commutative.
It can also be observed that
(1 * 2) * 3 = (1 + 1 × 2) * 3
3 * 3 = 3 + 3 × 3 = 3 + 9 = 12
1 * (2 * 3) = 1 * (2 + 2 × 3)
= 1 * 8 = 1 + 1 × 8 = 9
∴ (1 * 2) * 3 ≠ 1 * (2 * 3) where 1, 2, 3 ∈ Q
Thus, the operation * is not associative.
(iv) On Q, the operation * defined by a * b = (a – b)2 is
For a, b ∈ Q, we have
a * b = (a – b)2 and b * a = (b – a)2
= [–(a – b)]2 = (a – b)2
Therefore, a * b = b * a
Thus, the operation * is associative. It can
be observed that
(1 * 2) * 3 = (1 – 2)2 * 3 = (– 1)2 * 3
= 1 * 3 = (1 – 3)2 = (– 2)2 = 4
1 * (2 * 3) = 1 * (2 – 3)2 = 1 * (– 1)2
= 1 * 1 = (1 – 1)2 = 0
∴ (1 * 2) * 3 ≠ 1 * (2 * 3) where 1, 2, 3 ∈ Q
Thus, the operation * is not associative.
(v) On Q, the operation * is defined as a * b
$$=\frac{ab}{4}.\\\text{For a, b ∈ Q, we have a * b}\\=\frac{ab}{4} = \frac{ba}{4} = b*a$$
Therefore, a * b = b * a
Thus, the operation * is commutative.
For a, b, c ∈ Q, we have (a * b) * c =
$$\frac{ab}{4}*c\\=\frac{\frac{ab}{4}.c}{4} =\frac{abc}{16}\\ a*(b*c) = a*\frac{bc}{4}\\=\frac{a.\frac{bc}{4}}{4} =\frac{abc}{16}$$
Therefore, (a * b) * c = a * (b * c). Thus, the
operation * is associative.
(vi) On Q, the operation * is defined as a * b = ab2
It can be observed that for 2, 3 ∈ Q
2 * 3 = 2.32 = 18 and 3 * 2 = 3.22 = 12
Hence, 2 * 3 ≠ 3 * 2
$$\text{Also,\space}\frac{1}{2} + \frac{1}{3} = \frac{1}{2}\bigg(\frac{1}{3}\bigg)^{2}\\=\frac{1}{2}.\frac{1}{9} = \frac{1}{18}\\\frac{1}{3}*\frac{1}{2} =\frac{1}{3}\bigg(\frac{1}{2}\bigg)^{2}\\=\frac{1}{3}.\frac{1}{4} = \frac{1}{12}\\\therefore\space \frac{1}{2}*\frac{1}{3} \neq \frac{1}{3}*\frac{1}{2}\\\text{where,\space}\frac{1}{2},\frac{1}{3}\epsilon \text{Q}$$
Thus, the operation * is not commutative.
It can also be observed that for 1, 2, 3 ∈ Q
(1 * 2) * 3 = (1.22) * 3 = 4 * 3 = 4.32 = 36
1 * (2 * 3) = 1 * (2.32) = 1 * 18 = 1.182 = 324
(1 * 2) * 3 ≠ 1 * (2 * 3 )
$$\text{Also,\space}\bigg(\frac{1}{2}*\frac{1}{3}\bigg)*\frac{1}{4}\\=\bigg[\frac{1}{2}*\bigg(\frac{1}{3}\bigg)^{2}\bigg]*\frac{1}{4}\\= \frac{1}{18}*\frac{1}{4} = \frac{1}{18}*\bigg(\frac{1}{4}\bigg)^{2}\\=\frac{1}{18×16}\\\frac{1}{2}*\bigg(\frac{1}{3}*\frac{1}{4}\bigg) = \frac{1}{2}*\bigg[\frac{1}{3}\bigg(\frac{1}{4}\bigg)^{2}\bigg]\\=\frac{1}{2}*\frac{1}{48}\\=\frac{1}{18}= \frac{1}{2}\bigg(\frac{1}{48}\bigg)^{2} =\frac{1}{4608}$$
$$\therefore\space\bigg(\frac{1}{2}*\frac{1}{3}\bigg)*\frac{1}{4}\neq\frac{1}{2}*\bigg(\frac{1}{3}*\frac{1}{4}\bigg)\\\text{where}\space\frac{1}{4},\frac{1}{4},\frac{1}{4}\epsilon\text{Q}$$
Thus, the operation * is not associative. Hence, the operations defined in parts (ii), (iv), (v) are commutative and the operation defined in part (v) is associative.
10. Find which of the operations given above has identity.
Sol. An element e ∈ Q will be the identity element for the operation * if
a * e = a = e * a, ∀ a ∈ Q
(i) a * b = a – b
If a * e = a, a ≠ 0
⇒ a – e = a, a ≠ 0 ⇒ e = 0
Also, e * a = a
⇒ e – a = a ⇒ e = 2a
∴ e = 0 = 2a, a ≠ 0
But the identity is unique. Hence, this operation has no identity.
(ii) a * b = a2 + b2
If a * e = a, then a2 + e2 = a
For a = –2, (– 2)4 + e2 = 4 + e2 ≠ – 2
Hence, there is no identity element.
(iii) a * b = a + ab
If a * e = a
⇒ a + ae = a
⇒ ae = 0 ⇒ e = 0, a ≠ 0
Also if
⇒ e * a = a ⇒ e + ea = a
$$\Rarr\space e =\frac{a}{1-a}, a\neq1\\\therefore\space e =0=\frac{a}{1-a}, a\neq 0$$
But the identity is unique. Hence this operation has no identity.
(iv) a * b = (a – b)2
If a * e = a, then (a – e)2 = a. A square is always positive, so for
a = – 2, (– 2 – e)2 ≠ – 2
Hence, there is no identity element.
(v) a * b = ab/4
If a * e = a, then ae/4 = a. Hence, e = 4 is the identity element.
∴ a * 4 = 4 * a = 4a/4 = a
(vi) a * b = ab2
If a * e = a ⇒ ae2 = a
⇒ e2 = 1 ⇒ e = ± 1
But identity is unique. Hence this operation has no identity.
Therefore only part (v) has an identity element.
11. Let A = N × N and * be the binary operation on A defined by (a, b) * (c, d) = (a + c, b + d). Show that * is commutative and associative. Find the identity element for * on A, if any.
Sol. Given that A = N × N
and * is a binary operation on A and is defined by
(a, b) * (c, d) = (a + c, b + d)
Let (a, b), (c, d) ∈ A
Then, a, b, c, d ∈ N
we have (a, b) * (c, d) = (a + c, b + d)
and (c, d) * (a, b) = (c + a, d + b)
= (a + c, b + d)
[Addition is commutative in the set of natural numbers]
Therefore, (a, b) * (c, d) = (c, d) * (a, b)
Therefore, the operation * is commutative.
Now, let (a, b), (c, d) (e, f) ∈ A
Then, a, b, c, d, e, f ∈ N
We have ((a, b) * (c, d)) * (e, f) = (a + c, b + d) * (e, f)
= (a + c + e, b + d + f)
and (a, b) * ((c, d) * (e, f)) = (a, b) * (c + e, d + f)
= (a + c + e, b + d + f)
∴ ((a, b) * (c, d)) * (e, f) = (a, b) * ((c, c) * (e, f))
Therefore, the operation * is associative.
An element e = (e1, e2) ∈ A will be an identity element for the operation * if
a * e = a = e * a ∀ a = (a1, a2) i.e.,
(a1 + e1, a2 + e2) = (a1, a2) = (e1 + a1, e2 + a2)
which is not true for any element in A.
Note that a + e = a for e = 0 but 0 does not belong to N.
Therefore, the operation * does not have any identity element.
12. State whether the following statements are true of false. Justify.
(i) For an arbitrary binary operation * on a set N, a * a = a ∀ a ∈ N.
(ii) If * is a commutative binary operation on N, then
a * (b * c) = (c * b) * a
Sol. (i) Define an operation * on N as a * b = a + b ∀ a, b ∈ N
Then, in particular, for b = a = 3, we have
3 * 3 = 3 + 3 = 6 ≠ 3
Therefore, statement (i) is false.
(ii) RHS = (c * b) * a = (b * c) * a
[* is commutative]
= a * (b * c)
[Again, as * is commutative]
= LHS
Therefore, a * (b * c) = (c * b) * a
Therefore, statement (ii) is true.
13. Consider a binary operation * on N defined as a * b = a3 + b3. Choose the correct answer.
(A) Is * both associative and commutative ?
(B) Is * commutative but not associative ?
(C) Is * associative but not commutative ?
(D) Is * neither commutative nor associative ?
Sol. (B) Is * commutative but not associative ?
On N, the operation * is defined as a * b = a3 + b3.
For, a, b ∈ N, we have
a * b = a3 + b3 = b3 + a3 = b * a
[Addition is commutative in N]
Therefore, the operation * is commutative.
It can be observed that
(1 * 2) * 3 = (13 + 23) * 3
= 9 * 3 = 93 + 33
= 729 + 27 = 756
1 * (2 * 3) = 1 * (23 + 33)
= 1 * (8 + 27) = 1 * 35
= 13 + 353
= 1 + (35)3
= 1 + 42875 = 42876
Therefore, (1 * 2) * 3 ≠ 1 * (2 * 3 ) where 1, 2, 3, ∈ N
Therefore, the operation * is not associative.
Hence, the operation * is commutative but not associative.
