NCERT Solutions for Class 12 Maths Chapter 10 - Vectors - Exercise 10.1
Access Exercises of Class 12 Maths Chapter 10 – Vectors
Exercise 10.1 Solutions 5 Questions
Exercise 10.2 Solutions 19 Questions
Exercise 10.3 Solutions 18 Questions
Exercise 10.4 Solutions 12 Questions
Miscellaneous Exercise on Chapter 10 Solutions 19 Questions
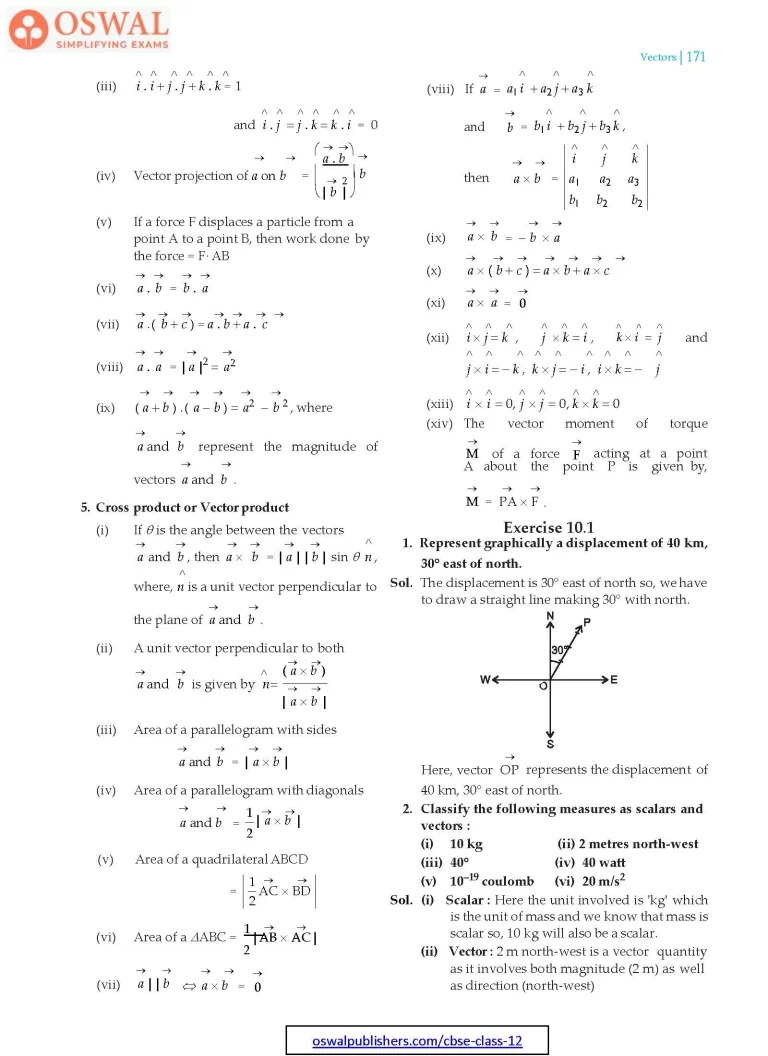
Exercise 10.1
1. Represent graphically a displacement of 40 km, 30° east of north.
Sol. The displacement is 30° east of north so, we have to draw a straight line making 30° with north.
$$\text{Here, vector}\space\vec{\text{OP}}\space\text{represents the}\\\text{displacement of 40 km, 30°}\\\text{east of north.}$$
2. Classify the following measures as scalars and vectors :
(i) 10 kg
(ii) 2 metres north-west
(iii) 40°
(iv) 40 watt
(v) 10–19 coulomb
(vi) 20 m/s2
Sol. (i) Scalar : Here the unit involved is 'kg' which is the unit of mass and we know that mass is scalar so, 10 kg will also be a scalar.
(ii) Vector : 2 m north-west is a vector quantity as it involves both magnitude (2 m) as well as direction (north-west)
(iii) Scalar : 40° represents an angle and we know that angle is a scalar quantity as it involves only magnitude.
(iv) Scalar : Here, the unit involved is watt which is the unit of power which is a scalar quantity. So, 40 W is a scalar quantity as it involves only magnitude.
(v) Scalar : 10–19 C is a scalar quantity as it involves only magnitude.
(vi) Vector : Here, the unit involved is m/s2 which is the unit of acceleration and acceleration is a vector quantity. So, 20 m/s2 is a vector quantity as it involves magnitude as well as direction.
3. Classify the following as scalar and vector quantities :
(i) Time period
(ii) Distance
(iii) Force
(iv) Velocity
(v) Work-done
Sol. (i) Scalar : Time period is a scalar quantity as it involves only magnitude.
(ii) Scalar : Distance is a scalar quantity as it involves only magnitude.
(iii) Vector : Force is a vector quantity as it involves both magnitude as well as direction.
(iv) Vector : Velocity is a vector quantity as it involves both magnitude as well as direction.
(v) Scalar : Work done is a scalar quantity as it involves only magnitude.
4. In figure (a square), identify the following vectors :
(i) Coinitial
(ii) Equal
(iii) Collinear but not equal.
$$\textbf{Sol.\space (i)\space}\text{Vectors}\space\vec{a}\space\text{and}\space\vec{a}\space\text{are coinitial}$$
because they have the same initial point.
$$\textbf{(ii)\space}\text{Vectors}\space\vec{b}\space\text{and}\space \vec{d}\space\text{are equal}$$
because they have the same magnitude and direction.
$$\textbf{(iii)\space}\text{Vectors}\space \vec{a}\space\text{and}\space\vec{c}\space\text{are collinear}$$
but not equal. Although they are parallel, this is because their directions are not same.
5. Answer the following as true or false :
$$\textbf{(i)\space}\vec{\textbf{a}}\space \textbf{and}\space -\vec{\textbf{a}}\space\textbf{are collinear.}$$
(ii) two collinear vectors are always equal in magnitude.
(iii) Two vectors having same magnitude are collinear.
(iv) Two collinear vectors having the same magnitude are equal.
$$\textbf{Sol.\space(i)\space True:}\space\text{Vectors}\space \vec{a}\space\text{and}\space -\vec{a}$$
are parallel to the same line and hence collinear.
(ii) False : Collinear vectors are those vectors that are parallel to the same line but they need not be equal in magnitude.
(iii) False : It is not necessary for two vectors having the same magnitude to be parallel to the same line.
(iv) False : Two vectors are said to be equal, if they have the same magnitude and direction, regardless of the position of their initial points. Hence, two collinear vectors having the same magnitude need not to be equal.
Share page on
