NCERT Solutions for Class 12 Maths Chapter 10 - Vectors - Exercise 10.3

Download PDF

Access Exercises of Class 12 Maths Chapter 10 – Vectors

Exercise 10.1 Solutions 5 Questions

Exercise 10.2 Solutions 19 Questions

Exercise 10.3 Solutions 18 Questions

Exercise 10.4 Solutions 12 Questions

Miscellaneous Exercise on Chapter 10 Solutions 19 Questions

Exercise 10.3

1. Find the angle between two vectors

$$\vec{\textbf{a}}\space\textbf{and}\space\vec{\textbf{b}}\space\textbf{with magnitudes}\space\sqrt{\textbf{3}}\\\textbf{and 2 respectively, having}\\\vec{\textbf{a}}.\vec{\textbf{b}} \textbf{=}\sqrt{\textbf{6}}.$$

Sol. It is given that

$$|\vec{\text{a}}| = \sqrt{3},\space |\vec{\text{b}}|=2\\\text{and}\space\vec{a}.\vec{b}=\sqrt{6}$$

Let θ be the required angle, then

$$\text{cos}\space\theta = \frac{\vec{a}.\vec{b}}{|\vec{a}||\vec{b}|}\\=\frac{\sqrt{6}}{\sqrt{3}×\sqrt{2}}=\frac{1}{\sqrt{2}}$$

$$\Rarr\space\theta = \text{cos}^{\normalsize-1}\bigg(\frac{1}{\sqrt{2}}\bigg)\\=\text{cos}^{\normalsize-1}\bigg(\text{cos}\frac{\pi}{4}\bigg)=\frac{\pi}{4}$$

Hence, the angle between the given vectors

$$\vec{a}\space\text{and}\space\vec{b}\space\text{is}\frac{\pi}{4}.$$

2. Find the angle between the vectors

$$\hat{\textbf{i}} \textbf{= 2}\hat{\textbf{j}}\textbf{+}\hat{\textbf{k}}\space\textbf{and}\space \textbf{3}\hat{\textbf{i}}\textbf{-2}\hat{\textbf{j}}\textbf{+}\hat{\textbf{k}}\textbf{.}$$

$$\textbf{Sol.\space}\text{Let}\space\vec{a} = \hat{i}-2\hat{j}+3\hat{k}\space\text{and}\\\space\vec{b} = 3\hat{i}-2\hat{j}+\hat{k}\\\text{Magnitude of\space}\vec{a},|\\|\vec{a}| = \sqrt{1^{2} + (-2)^{2} +3^{2}}\\=\sqrt{1+4+9} = \sqrt{14}\\\text{Magnitude of}\space\vec{b},\\|b| = \sqrt{3^{2} + (-2)^{2}+1^{2}}\\=\sqrt{9+4+1}=\sqrt{14}\\\text{Now,}\space\vec{a}.\vec{b} = (\hat{i}-2\hat{j}+3\hat{k}).(3\hat{i}-2\hat{j}+\hat{k})$$

= 1.3 + (– 2). (– 2) + 3.1 = 3 + 4 + 3 = 10

(Dot product of two vectors is equal to the sum of the products of their corresponding components.)

$$\text{Let}\space\theta\space\text{be the required angle between}\\\vec{a}\space\text{and}\space\vec{b}\space\text{then}\\\text{cos}\space\theta = \frac{|\vec{a}.\vec{b}|}{|\vec{a}||\vec{b}|}=\frac{10}{\sqrt{14}\sqrt{14}}\\=\frac{10}{14}=\frac{5}{7}\\\Rarr\space\theta = \text{cos}^{\normalsize-1}\bigg(\frac{5}{7}\bigg)$$

3. Find the projection of the vector

$$\hat{\textbf{i}}\space\textbf{-}\space\hat{\textbf{j}}\space\textbf{on the vector}\space\hat{\textbf{i}} +\hat{\textbf{j}}.\\\textbf{Sol.\space}\text{Let}\space\vec{a} = \hat{i} - \hat{j},\vec{b} = \hat{i} + \hat{j}\\\text{Projection of}\space\vec{a}\space\text{on}\space\vec{b}\space\text{is given by}\\\frac{\vec{a}\vec{b}}{|\vec{b}|}=\frac{(\hat{i}-\hat{j}).(\hat{i} + \hat{j})}{\sqrt{1^{2}+1^{2}}}\\=\frac{1×1 + (-1)×1}{\sqrt{2}}$$

= 0

$$\text{Hence, the projection of vector}\\\space\vec{a}\space\text{and}\space\vec{b}\space\text{is 0.}$$

4. Find the projection of the vector

$$\hat{\textbf{i}}\space\textbf{+}\space 3\hat{\textbf{j}} \textbf{+ 7}\hat{\textbf{k}}\space\textbf{on the vector}\space 7\hat{\textbf{i}}\textbf{-}\hat{\textbf{j}} \textbf{+ 8}\hat{\textbf{k}.}\\\textbf{Sol.}\space\text{Let\space}\vec{a} = \hat{i} + 3\hat{j}+7\hat{k}\space\text{and}\\\vec{b} = 7\hat{i}-\hat{j}+8\hat{k},\space\text{then projection of}\space\vec{a}\\\text{is given by}$$

$$\frac{\vec{a}.\vec{b}}{|\vec{b}|} = \bigg(\frac{(\hat{i} + 3\hat{j} +7\hat{k}).(7\hat{i} - \hat{j}+8\hat{k})}{\sqrt{7^{2} + (-1)^{2}+ 8^{2}}}\bigg)\\=\bigg(\frac{1×7+3×(\normalsize-1)+ 7×8}{\sqrt{49 + 1+64}}\bigg)\\=\frac{7-3+56}{\sqrt{114}}\\=\frac{60}{\sqrt{114}}$$

Hence, the projection of vector

$$(\hat{i}+3\hat{j}+7\hat{k})\space\text{on} (7\hat{i} -\hat{j}+8\hat{k})\\\text{is}\space\frac{60}{\sqrt{114}}.$$

5. Show that each of the given three vectors is a unit vector :

$$\frac{\textbf{1}}{\textbf{7}}\textbf{(2}\hat{\textbf{i}}\space\textbf{+}\space \textbf{3}\hat{\textbf{j}}\space\textbf{+}\space 6\hat{\textbf{k}})\textbf{,}\space\frac{\textbf{1}}{\textbf{7}}\textbf{(3}\hat{\text{i}}\space\textbf{- 6}\hat{\textbf{j}}\textbf{ + 2}\hat{\textbf{k}})\textbf{,}\\\frac{\textbf{1}}{\textbf{7}}\textbf{(6}\hat{\textbf{i}}\space\textbf{+}\space \textbf{2}\hat{\textbf{j}}\space\textbf{- 3}\hat{\textbf{k}}\textbf{).}$$

Also, show that they are mutually perpendiclar to each other.

$$\textbf{Sol.\space}\text{Let\space}\vec{\text{a}} = \frac{1}{7}(2\hat{i} + 3\hat{j}+ 6\hat{k})\\=\frac{2}{7}\hat{i}+\frac{3}{7}\hat{j}+\frac{6}{7}\hat{k}\\\vec{b} = \frac{1}{7}(3\hat{\text{i}}-6\hat{\text{j}} + 2\hat{\text{k}}) = \frac{3}{7}\hat{\text{i}}-\frac{6}{7}\hat{\text{j}}+\frac{2}{7}\hat{k}\\\text{and}\space \vec{c} = \frac{1}{7}(6\hat{i}+ 2\hat{j}-3\hat{k})\\=\frac{6}{7}\hat{i}+ \frac{2}{7}\hat{j}-\frac{3}{7}\hat{k}\\\text{Then, magnitude of}\space\vec{a},\\|\vec{a}| =\sqrt{\bigg(\frac{2}{7}\bigg)^{2} + \bigg(\frac{3}{7}\bigg)^{2} + \bigg(\frac{6}{7}\bigg)^{2}}$$

$$ = \sqrt{\frac{49}{49}}=1\\\text{magnitude of}\space\vec{b,}\\|\vec{b}| =\sqrt{\bigg(\frac{3}{7}\bigg)^{2} + \bigg(\frac{-6}{7}\bigg)^{2} + \bigg(\frac{2}{7}\bigg)^{2}}\\=\sqrt{\frac{49}{49}}=1\\\text{and magnitude of}\space\vec{c},\\|\vec{c}| = \sqrt{\bigg(\frac{6}{7}\bigg)^{2} + \bigg(\frac{2}{7}\bigg)^{2} + \bigg(\frac{-3}{7}\bigg)^{2}}\\=\sqrt{\frac{49}{49}}=1$$

Thus, each of the given three vectors is a unit vector.

Now ,

$$\vec{a}.\vec{b} = \bigg(\frac{2}{7}\hat{i} + \frac{3}{7}\hat{j}+ \frac{6}{7}\hat{k}\bigg)\bigg(\frac{3}{7}\hat{\text{i}}-\frac{6}{7}\hat{\text{j}} + \frac{2}{7}\hat{\text{k}}\bigg)\\=\frac{2}{7}×\frac{3}{7}+\frac{3}{7}×\bigg(\frac{\normalsize-6}{7}\bigg) + \frac{6}{7}×\frac{2}{7}\\=\frac{6}{49}- \frac{18}{49}+\frac{12}{49}\\=\frac{6-18+12}{49}=\frac{0}{49}=0\\\text{i.e.,}\vec{\text{a}}\space\text{and}\space\vec{\text{b}}\space\text{are perpendicular}\\\text{to each other.}\\\vec{b}.\vec{c} = \bigg(\frac{3}{7}\hat{i} - \frac{6}{7}\hat{j}+\frac{2}{7}\hat{k}\bigg)\\\bigg(\frac{6}{7}\hat{i}+ \frac{2}{7}\hat{j} -\frac{3}{7}\hat{k}\bigg)$$

$$ = \frac{3}{7}×\frac{6}{7}+\bigg(\frac{-6}{7}\bigg)+\frac{6}{7}×\frac{2}{7}\\=\frac{6}{49}-\frac{18}{49}+\frac{12}{49}\\=\frac{6-18+12}{49}=\frac{0}{49}=0\\\text{i.e.,}\space\vec{a}\space\text{and}\space\vec{b}\space\text{are perpendicular}\\\text{to each other.}\\\vec{b}.\vec{c} =\bigg(\frac{3}{7}\hat{i} -\frac{6}{7}\hat{j}+\frac{2}{7}\hat{k}\bigg)\\\bigg(\frac{6}{7}\hat{i}+\frac{2}{7}\hat{j}-\frac{3}{7}\hat{k}\bigg)$$

$$ = \frac{3}{7}×\frac{6}{7}+\bigg(\frac{-6}{7}\bigg)×\frac{2}{7}+\frac{2}{7}×\bigg(\frac{-3}{7}\bigg)\\=\frac{18}{49}-\frac{12}{49}-\frac{6}{49}\\=\frac{18-12-6}{49}=\frac{0}{49}=0$$

$$\text{i.e.,\space}\vec{b}\space\text{and}\space\vec{c}\space\text{are perpendicular}\\\text{to each other}\\=\vec{c}.\vec{a} = \bigg(\frac{6}{7}\hat{i}+\frac{2}{7}\hat{j}-\frac{3}{7}\hat{k}\bigg)\\\bigg(\frac{2}{7}\hat{i} + \frac{3}{7}\hat{j}+\frac{6}{7}\hat{k}\bigg)\\=\frac{6}{7}×\frac{2}{7}+\frac{2}{7}×\frac{3}{7}+\bigg(\frac{-3}{7}\bigg)×\frac{6}{7}\\=\frac{12}{49}+\frac{6}{49}-\frac{18}{49}\\=\frac{12+6-18}{49}=\frac{0}{49}=0$$

$$\text{i.e.\space}\vec{c}\space\text{and}\space\vec{\text{a}}\space\text{are perpendicular}\\\text{to each other.}$$

Hence, the given three vectors are mutually perpendicular to each other.

$$\textbf{6.\space}\textbf{Find}|\vec{\textbf{a}}|\space\textbf{and}|\vec{\textbf{b}}|\textbf{, if}\space\textbf{(}\vec{\textbf{a}})\space\textbf{and} |\vec{\textbf{b}}|,\\\textbf{if}\space(\vec{\textbf{a}} \textbf{+}\vec{\textbf{b}})\textbf{.(}\vec{\textbf{a}}\textbf{-}\vec{\textbf{b}})\textbf{= 8}\space\textbf{and}|\vec{\textbf{a}}| \textbf{= 8}|\vec{\textbf{b}}|\textbf{.}\\\textbf{Sol.\space}\text{Given,}\space (\vec{a} + \vec{b}).(\vec{a}\space -\space\vec{b}) = 8\space\\\text{and}\space|\vec{a}| = 8|\vec{b}|\\\Rarr\space \vec{a}.\vec{a}-\vec{a}.\vec{b}+\vec{b}.\vec{a}-\vec{b}.\vec{b} = 8\\\Rarr\space |\vec{a}|^{2} -|\vec{b}|^{2} = 8\\(\because\space \vec{a}.\vec{a} = |\vec{a}|^{2}\space\text{and}\space \vec{a}.\vec{b}= \vec{b}.\vec{a})\\\Rarr\space (8|\vec{b}|)^{2} - |\vec{b}|^{2}= 8\\\Rarr\space 63|\vec{b}|^{2} =8\space (\text{Given},|\vec{a}| =8|\vec{b}|)\\\Rarr\space|\vec{b}| = \sqrt{\frac{8}{63}} =\frac{2}{3}\sqrt{\frac{2}{7}}$$

$$\text{Also,\space}|\vec{a}| = 8|\vec{b}| =8\bigg(\frac{2}{3}\sqrt{\frac{2}{7}}\bigg)\\=\frac{16}{3}\sqrt{\frac{2}{7}}.$$

7. Evaluate the product

$$\textbf{(3}\vec{\textbf{a}}\space\textbf{- 5}\vec{\textbf{b}})\textbf{.(2}\vec{\textbf{a}} \textbf{+} 7\vec{\textbf{b}})\textbf{.}$$

$$\textbf{Sol.\space}\text{We have,}\space (3\vec{a} -5\vec{b}).(2\vec{a} + 7\vec{b})\\=(3\vec{a}).(2\vec{a} + 7\vec{b})-(5\vec{b}).(2\vec{a}+ 7\vec{b})\\=6(\vec{a}.\vec{a})+21(\vec{a}.\vec{b})-10(\vec{b}.\vec{a})-35(\vec{b}.\vec{b})\\=6|\vec{a}|^{2} + 11(\vec{a}.\vec{b})-35|\vec{b}|^{2}\\(\because\space \vec{a}.\vec{a} =|\vec{a}|^{2}\text{and}\space \vec{a}.\vec{b} = \vec{b}.\vec{a})$$

8. Find the magnitude of two vectors

$$\vec{\textbf{a}}\space\textbf{and}\space\vec{\textbf{b}}$$having the same magnitude and such that the angle between them is 60° and their scalar product is 1/2.

Sol. Both vectors have same magnitude t.e.,

$$|\vec{a}|=|\vec{b}|$$

and scalar product of vectors,

$$\vec{a}.\vec{b} =\frac{1}{2}\space\text{(Given)}$$

Let θ be the angle between two vectors

$$\vec{a}\space\text{and}\space\vec{b},\text{then}\\\text{cos}\space\theta = \frac{\vec{a}.\vec{b}}{|\vec{a}||\vec{b}|}\\\Rarr\space \text{cos 60\degree} = \frac{\frac{1}{2}}{|\vec{a}||\vec{a}|}\\\lbrack\because |\vec{a}| =|\vec{b}|\rbrack\\\Rarr\space \frac{1}{2} =\frac{1}{2|\vec{a}|^{2}}\\\Rarr\space |\vec{a}|^{2}=1\\\Rarr\space|\vec{a}|=1\\\text{Thus,}\space|\vec{a}|=|\vec{b}|= 1$$

$$\textbf{9. Find}\space|\vec{\textbf{x}}|\textbf{,}\textbf{if for a unit vector}\space\vec{\textbf{a}},\\\textbf{(}\vec{\textbf{x}}\space\textbf{-}\space\vec{\textbf{a}})\textbf{.(}\vec{\textbf{x}} \textbf{+}\vec{\textbf{a}})\textbf{= 12}.\\\textbf{Sol.\space}\text{Given,\space}|\vec{a}|=1\\(\because\space\text{It is a unit vector,}\\\text{magnitude of a unit vector is 1.)}\\\text{and\space}(\vec{x}-\vec{a}).(\vec{x}+\vec{a})=12\\\Rarr\space\vec{x}.\vec{x} + \vec{x}.\vec{a}-\vec{a}.\vec{x}-\vec{a}.\vec{a}=12\\(\because\space \vec{a}.\vec{a} =|\vec{a}|^{2}\space\text{and}\space\vec{x}.\vec{a} = \vec{a}.\vec{x})\\\Rarr\space |\vec{x}|^{2} -|\vec{a}|^{2}=12\\\Rarr\space |\vec{x}|^{2}-1^{2}=12\\\lbrack\because|\vec{a}|=1\space\text{as is a unit vector}\rbrack\\\Rarr\space |\vec{x}|^{2}=13$$

$$\Rarr\space |x| =\sqrt{13}$$

$$\textbf{10.\space If}\space\vec{\textbf{a}} \textbf{= 2}\hat{\textbf{i}}\textbf{+ 2}\textbf{j} + \textbf{3}\hat{\textbf{k}},\vec{\textbf{b}} \textbf{=} \textbf{-}\hat{\textbf{i}} + \textbf{2}\hat{\textbf{j}} + \hat{\textbf{k}}$$

$$\textbf{and}\space\vec{\textbf{c}} \textbf{= 3}\hat{\textbf{i}} + \hat{\textbf{j}}\space\textbf{such that}\space \vec{\textbf{a}} + \lambda\vec{\textbf{b}}\\\textbf{is perpendicular to}\space \vec{\textbf{c},}\\\space\textbf{then find the value of}\space\lambda.$$

Sol. The given vectors are

$$\vec{a} = 2\hat{i} + 2\hat{j} + 3\hat{k},\space \vec{\text{b}} = -\hat{i} + 2\hat{\text{j}} + \hat{k}\\\text{and}\qquad\vec{c} = 3\hat{i}+\hat{j}.\\\text{Now,}\space (\vec{a} + \lambda \vec{b}) \perp c\space\text{(Given)}\\\Rarr\space (\vec{a} + \lambda\vec{b}).\vec{c} = 0$$

(∵ Scalar product of two perpendicular vectors is zero)

$$\Rarr\space \lbrack(2\hat{i} + 2\hat{j} + 3\hat{k}) - \lambda(-\hat{i} + 2\hat{j} +\hat{k})\rbrack\\(3\hat{i}+\hat{j})=0\\\Rarr\space \lbrack(2-\lambda)\hat{i} + (2 + 2\lambda)\hat{j} + (3+\lambda)\hat{k}\rbrack.\\(3\hat{i} +\hat{j}) = 0\\\Rarr\space (2-\lambda)×3 + (2 + 2\lambda)×1 + (3+ \lambda)0 =0\\\Rarr\space 6-3\lambda + 2 + 2\lambda =0\\\Rarr\space 8-\lambda = 0\\\Rarr\space \lambda = 8.$$

Hence, the required value of λ is 8.

$$\textbf{11. Show that}\space|\vec{\textbf{a}}|\vec{\textbf{b}} + \vec{\textbf{b}}\vec{\textbf{a}}\space\\\textbf{is perpendicular to}\\|\vec{\textbf{a}}|\vec{\textbf{b}} \textbf{-} |\vec{\textbf{b}}|\vec{\textbf{a}}\space\textbf{for any two non-zero vectors}\\\vec{\textbf{a}}\space\textbf{and}\space\vec{\textbf{b}.}\\\textbf{Sol.\space}\text{Let}\space\vec{p} = |\vec{a}|\vec{b}+ |\vec{b}|\vec{a}\space\text{and}\space \vec{q} =|\vec{a}|\vec{b} -|\vec{b}|\vec{a}$$

$$\text{Then,}\space\vec{p}.\vec{q}\space=\space(|\vec{a}|\vec{b} \space+\space |\vec{b}|\vec{a}).(|\vec{a}|\vec{b} \space- \space|\vec{b}|\vec{a})\\=|\vec{a}|^{2}(\vec{b}.\vec{b})-|\vec{a}||\vec{b}|(\vec{b}.\vec{a})+\\|\vec{b}||\vec{a}|(\vec{a}.\vec{b}) \space-\space|\vec{b}|^{2}(\vec{a}.\vec{a})\\=|\vec{a}|^{2}|\vec{b}|^{2}- |\vec{a}||\vec{b}|(\vec{a}.\vec{b})+ |\vec{a}| |\vec{b}|(\vec{a}.\vec{b}) \space-\space |\vec{b}|^{2}|\vec{a}|^{2}\\\Rarr\space \vec{a}\perp\vec{b}\\(\because\space \text{If}\space\vec{a}.\vec{b} =0\\\Rarr\space \vec{a}\space\text{is perpendicular to}\space\vec{b})\\\text{Hence,\space}|\vec{a}|\vec{b} + |\vec{b}|\vec{a}\space\text{and}\space |\vec{a}|\vec{b} - |\vec{b}|\vec{a}$$

are perpendicular to each other for any two non-zero vectors

$$\vec{a}\space\text{and}\space\vec{b}.$$

$$\textbf{12\space If}\space \vec{\textbf{a}}.\vec{\textbf{a}}\textbf{ = 0}\space\textbf{and}\space\vec{\textbf{a}}\textbf{.}\vec{\textbf{b}}\textbf{= 0,}\space\\\textbf{then what can be conclude}\\\textbf{about the vector}\space \vec{\textbf{b}}\textbf{?}\\\textbf{Sol.\space}\text{It is given that}\space \vec{a}.\vec{a} = 0\space\\\text{and}\space\vec{a}.\vec{b}=0.\\\text{Now,\space}\vec{a}\vec{a} = 0\\\Rarr\space |\vec{a}|^{2} = 0\\\Rarr\space |\vec{a}| =0\\\therefore\space\vec{a}\space\text{is a zero vector.}\\\text{Hence, vector}\space\vec{b}\space\text{satisfying}\space\vec{a}.\vec{b}=0\\\text{can be any vector.}$$

$$\textbf{13.\space If}\space\vec{\textbf{a}},\vec{\textbf{b}},\vec{\textbf{c}}\space\textbf{are unit vectors such that}\\\vec{\textbf{a}} \textbf{+} \vec{\textbf{b}} \textbf{+}\vec{\textbf{c}} \textbf{= 0,}\space \textbf{find the value of}\\\vec{\textbf{a}}.\vec{\textbf{b}} \space\textbf{+}\space\vec{\textbf{b}}.\vec{\textbf{c}}\space\textbf{+}\space\vec{\textbf{c}}.\vec{\textbf{a}.}\\\textbf{Sol.\space}\text{Given,}\space|\vec{a}| =|\vec{b}| = |\vec{c}|=1\\\text{and}\space\vec{a} + \vec{b} + \vec{c} = 0\\\text{We have}\space (\vec{a} + \vec{b} + \vec{c}).(\vec{a} + \vec{b} + \vec{c}) = 0 \\\Rarr\space \vec{a}.(\vec{a} + \vec{b} + \vec{c}) + \vec{b}.(\vec{a} + \vec{b} +\vec{c})+\\\vec{c}.(\vec{a}+\vec{b}+\vec{c}) = 0\\\Rarr\space \vec{a}.\vec{a} + \vec{a}.\vec{b} + \vec{a}.\vec{c}+\vec{b}.\vec{a} + \vec{b}.\vec{b}+\vec{b}.\vec{c}+\\\vec{c}.\vec{a}+\vec{c}.\vec{b}+\vec{c}.\vec{c} = 0\\\Rarr\space|\vec{a}|^{2} + |\vec{b}|^{2}+|\vec{c}|^{2}+2(\vec{a}.\vec{b}+\vec{b}.\vec{c} + \vec{c.\vec{a}})\\=0$$

$$(\because\space \vec{a}\vec{a} = |\vec{a}|^{2}\space\text{and}\space \vec{a}.\vec{b} = \vec{b}.\vec{a})\\\Rarr\space 1+1+1+2(\vec{a}.\vec{b} + \vec{b}.\vec{c}+\vec{c}.\vec{a})=0\\(\because\space|\vec{a}| = |\vec{b}| = |\vec{c}|=1)\\\Rarr\space 3 + 2(\vec{a}.\vec{b} + \vec{b}.\vec{c}+\vec{c}.\vec{a}) = 0\\\Rarr\space \vec{a}.\vec{b}+\vec{b}.\vec{c}+\vec{c}.\vec{a}=-\frac{3}{2}$$

14. If either$$\vec{\textbf{a}}\space\textbf{= 0}\space\textbf{or}\space \vec{\textbf{b}}\space\textbf{= 0},\textbf{then\space}\vec{\textbf{a}}.\vec{\textbf{b}} \textbf{= 0.}$$

But the converse need not be true. Justify your answer with an example.

$$\textbf{Sol.\space}\text{If}\space\vec{a} = 0 = 0\hat{i} + 0 \hat{j} +0\hat{k}\space\text{and}\space\hat{b}\\\text{is non-zero i.e.,}\\\vec{b} = x\hat{i} + y\hat{j} +z\hat{k}\\\therefore\space \vec{a}\vec{b} = (0\hat{i} + 0\hat{j} + 0\hat{k})(x\hat{i}+y\hat{j}+z\hat{k})\\=(0×x) + (0×y) + (0×z) = 0\\\text{So, if}\space\vec{a} =0\space\text{or}\space\vec{b} = 0,\space \text{then for same}\\\vec{a}.\vec{b}=0$$

To prove that converse need not to be true we have to prove that for two non-zero vectors $$\vec{a}\space\text{and}\space\vec{b}, \vec{a}.\vec{b}\space\text{can be zero.}$$

$$\text{Let}\space \vec{a} = 2\hat{i} + 4\hat{j}+3\hat{k}\space\text{and}\space\vec{b} = 3\hat{i}+3\hat{j}-6\hat{k}$$

$$\text{Then,}\space \vec{a}.\vec{b} = 2.3 +4.3+3.(\normalsize-6) $$

= 6 + 12 – 18 = 0

We now observe that

$$|\vec{a}| =\sqrt{2^{2}+4^{2}+3^{2}}\\=\sqrt{29}\\\therefore\space \vec{a}\neq 0\\|\vec{b}| = \sqrt{3^{2}+3^{2} + (-6)^{2}} = \sqrt{54}\\\therefore\space \vec{b}\neq 0$$

Hence, the converse of the given statement need not be true.

15. If the vertices A, B, C of a triangle ABC are (1, 2, 3), (– 1, 0, 0), (0, 1, 2) respectively then find

$$\angle\textbf{ABC}\textbf{(}\angle \textbf{ABC}\space \textbf{is the angle between}\\\textbf{the vectors}\space \vec{\textbf{BA}}\space\textbf{and}\space\vec{\textbf{BC}}\textbf{).}$$

Sol. Here, we have to find

$$\angle\text{ABC}\space\text{i.e., angle between}\space\vec{\text{BA}}\space\text{and}\space\vec{\text{BC},}$$

so first of all we have to calculate both these vectors after that we can find the angle between them by

$$\text{cos}\space\theta = \frac{(\vec{\text{BA}}).(\vec{\text{BC}})}{|\vec{\text{BA}}||\vec{\text{BC}}|}$$

We are given the points A(1, 2, 3), B(– 1, 0, 0) and C(0, 1, 2).

$$\text{Also, it is given that}\space\angle\text{ABC is the}\\\text{angle between the vectors}\space\vec{\text{BA}}\space\text{and}\space\vec{\text{BC}.}\\\text{Here,}\space \vec{\text{BA}} = \text{P.V. of A - P.V of B}$$

$$=(\hat{i}+ 2\text{j} +3\hat{\text{k}}) - (-\hat{i} + 0\hat{j} + 0\hat{k})\\=\lbrack\hat{i} - (-\hat{i}) +(2\hat{j} -0)+ (3\hat{k} - 0)\rbrack\\= 2\hat{i} + 2\hat{j}+3\hat{k}\\|\vec{\text{BA}}| =\sqrt{(2)^{2} + (2)^{2} + (3)^{2}}\\=\sqrt{4+4+9}\\=\sqrt{17}\\\vec{\text{BC}} = \text{P.V. of C – P.V. of B}$$

$$= (0\hat{i} + 1\hat{j} + 2\hat{k})-(-\hat{i} + 0\hat{j}+ 0\hat{k})\\=\lbrack0-(-\hat{i}) + (1\hat{j}-0) + (2\hat{k}-0)\rbrack\\=\hat{i}+\hat{j}+2\hat{k}\\|\vec{\text{BC}}| =\sqrt{(1)^{2} +(1)^{2} +(2)^{2}}\\=\sqrt{1+1+4} =\sqrt{6}\\\text{Now,\space}\vec{\text{BA}}.\vec{\text{BC}} \\= (2\hat{i} + 2\hat{j}+3\hat{k}).(\hat{i}+\hat{j}+2\hat{k})$$

= 2 × 1 + 2 × 1 + 3 × 2 = 10

$$\text{cos}\space\theta = \frac{\vec{\text{BA}}.\vec{\text{BC}}}{|\vec{\text{BA}}||\vec{\text{BC}}|}\\\Rarr\space\text{cos}(\angle \text{ABC}) = \frac{10}{\sqrt{17}\sqrt{6}}\\\Rarr\space \angle\text{ABC} = \text{cos}^{\normalsize-1}\bigg(\frac{10}{\sqrt{102}}\bigg)$$

16. Show that the points A(1, 2, 7), B(2, 6, 3) and C(3, 10, – 1) are collinear.

Sol. The given points are A(1, 2, 7), B(2, 6, 3) and C(3, 10, – 1).

$$\therefore\space \vec{\text{AB} = }\text{P.V. of B – P.V. of A}\\=(2\hat{i} + 6\hat{j}+3\hat{k})-(1\hat{i}+2\hat{j}+7\hat{k})\\=(2-1)\hat{i} + (6-2)\hat{j} + (3-7)\hat{k}\\=\space\hat{i} + 4\hat{j}-4\hat{k}\\\text{Magnitude of}\space\vec{\text{AB},}\\|\vec{\text{AB}}| = \sqrt{(1)^{2} + (4)^{2} + (-4)^{2}}\\=\sqrt{1+16+16}=\sqrt{33}\\\vec{\text{BC}} = \text{P.V. of C – P.V. of B}\\=(3\hat{i} + 10\hat{j}-1\hat{k}) - (2\hat{i} + 6\hat{j} +3\hat{k})\\=(3-2)\hat{i} + (10-6)\hat{j} + (-1-3)\hat{k}\\ =\hat{i} + 4\hat{j}-4\hat{k}\\\text{Magnitude of}\space\vec{\text{BC}}$$

$$|\vec{\text{BC}}| = \sqrt{(1)^{2} + (4)^{2} + (-4)^{2}}\\=\sqrt{1 + 16 + 16}=\sqrt{33}\\\vec{\text{AC}} = \text{P.V. of C – P.V. of A}\\= (3\hat{i} + 10\hat{j}-1\hat{k}) -(1\hat{i} +2\hat{j} +7\hat{k})\\=(3-1)\hat{i}+ (10-3)\hat{j}+(-1-7)\hat{k}\\ = 2\hat{i} +8\hat{j} -8\hat{k}\\\text{Magnitude of}\space \vec{\text{AC},}\\|\vec{\text{AC}}| =\sqrt{2^{2} + 8^{2} + (-8)^{2}}\\=\sqrt{4+64+64} = \sqrt{132}\\=2\sqrt{33}\\=\sqrt{33} + \sqrt{33}\\\therefore\space |\vec{\text{AC}}| = |\vec{\text{AB}}| + |\vec{\text{BC}}|.$$

Hence, the given points A, B and C are collinear.

17. Show that the vectors

$$\textbf{2}\hat{\textbf{i}}\textbf{-}\hat{\textbf{j}}\textbf{+}\hat{\textbf{k}}\textbf{,} \space \hat{\textbf{i}}\textbf{-3}\hat{\textbf{j}}\textbf{-5}\hat{\textbf{k}}\space\textbf{and}\space \textbf{3}\hat{\textbf{i}}\textbf{-4}\hat{\textbf{j}}\textbf{-4}\hat{\textbf{k}}$$

from the vertices of a right angled triangle.

$$\textbf{Sol.\space}\text{Let A = }2\hat{i} -\hat{j}+\hat{k},\\\text{B = }\hat{i}-3\hat{j}-5\hat{k}\\\text{and}\space\text{C} = 3\hat{i} - 4\hat{j}-4\hat{k}\\\therefore\space\text{Side}\space\vec{\text{AB}} =\text{P.V. of B – P.V. of A}\\=(\hat{i} - 3\hat{j}-5\hat{k})-(2\hat{i} -\hat{j} +\hat{k})\\=\hat{i}-3\hat{j}-5\hat{k}-2\hat{i}+\hat{j}-\hat{k}\\=-\hat{i}-2\hat{j}-6\hat{k}\\|\vec{\text{AB}}| =\sqrt{(-1)^{2} + (-2)^{2} + (-6)^{2}}\\=\sqrt{1+4+36}\\=\sqrt{41}\\\vec{\text{BC}} = \text{P.V. of C – P.V. of B}$$

$$=(3\hat{i}-4\hat{j}-4\hat{k})-(\hat{i}-3\hat{j}-5\hat{k})\\=3\hat{i}-4\hat{j}-4\hat{k}-\hat{i}+3\hat{j}+5\hat{k}\\=2\hat{i}-\hat{j}+\hat{k}\\|\vec{\text{BC}}| =\sqrt{2^{2} + (-1)^{2}+1^{2}}\\=\sqrt{4+1+1}\\=\sqrt{6}\\\text{and}\space\vec{\text{AC}} = \text{P.V. of C – P.V. of A}\\=(3\hat{i}-4\hat{j}-4\hat{k})-(2\hat{i}-\hat{j}+\hat{k})\\=3\hat{i}-4\hat{j}-4\hat{k}-2\hat{i}+\hat{j}-\hat{k}\\=\hat{i}-3\hat{j}-5\hat{k}\\\text{and}\space |\vec{\text{AC}}| = \sqrt{1^{2} + (-3)^{2} + (-5)^{2}}$$

$$\sqrt{1 + 9 + 25}\\=\sqrt{35}\\\text{Now,\space}|\vec{\text{BC}}|^{2} + |\vec{\text{AC}}|^{2}\\= 6+35+41 =|\vec{\text{AB}}|^{2}$$

which shows that ABC is a right angled triangle.

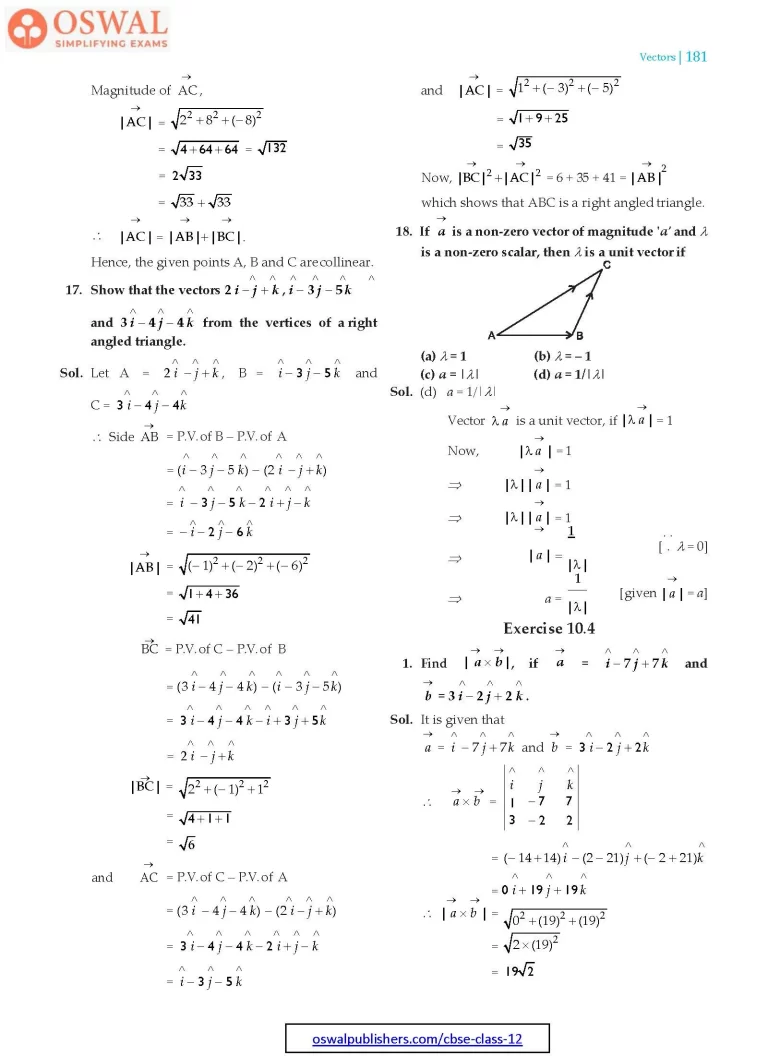
$$\textbf{18.\space If}\space \vec{\textbf{a}}\space\textbf{is a non-zero vector of magnitude}\\\textbf{'a' and}\space\lambda\space\textbf{is a non-zero scalar,}\\\textbf{then}\space\lambda\space\textbf{is a unit vector if}$$

$$\textbf{(a)\space}\lambda\textbf{= 1}\\\textbf{(b)\space}\lambda \textbf{= -1}\\\textbf{(c)\space a = }\textbf{|}\lambda\textbf{|}\\\textbf{(d)\space} \textbf{a =}\frac{\textbf{1}}{\lambda}\\\textbf{Sol.}\space a =\frac{1}{\lambda}\\\text{Vector}\space \lambda\space\vec{a}\text{is a unit vector,}\\\text{if}\space |\lambda \vec{a}|=1\\\text{Now,}\space |\lambda \vec{a}|=1\\\Rarr\space |\lambda||\vec{a}|=1\\\Rarr\space |\lambda||\vec{a}|=1$$

$$\Rarr\space |\vec{a}| =\frac{1}{|\lambda|}\space\lbrack\because\space\lambda =0\rbrack\\\Rarr\space a =\frac{1}{|\lambda|}\\\lbrack\text{given}\space|\vec{a}|= a\rbrack$$

Download PDF