NCERT Solutions for Class 12 Maths Chapter 10 - Vectors - Exercise 10.4

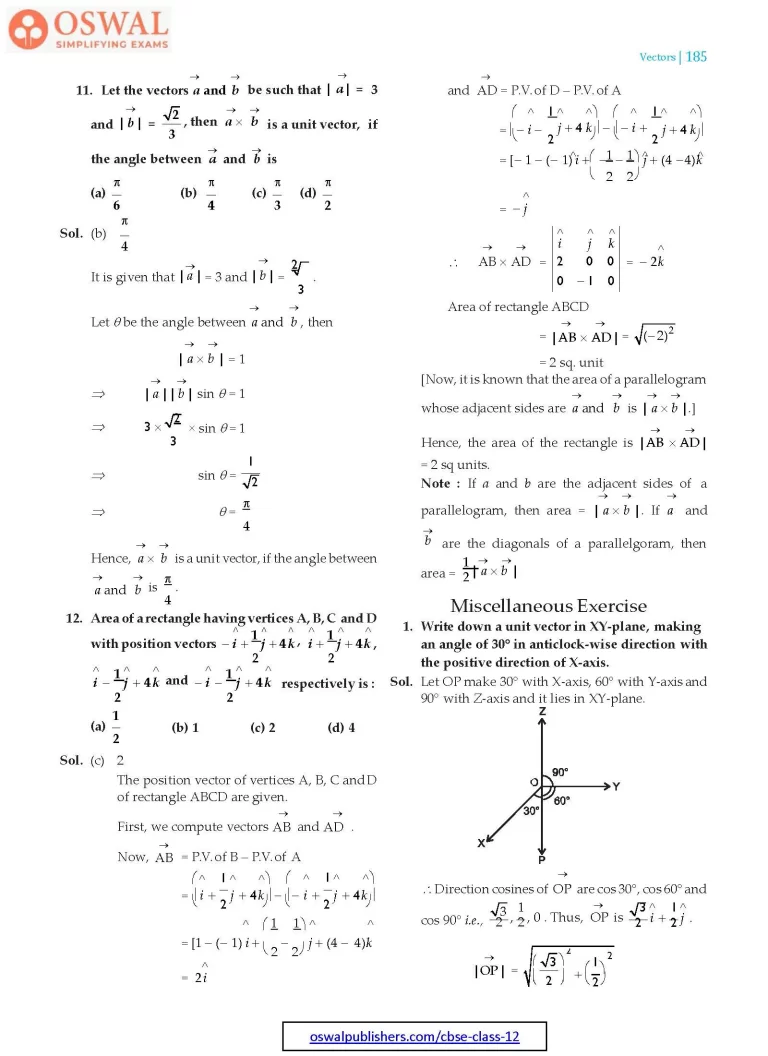
Access Exercises of Class 12 Maths Chapter 10 – Vectors

Miscellaneous Exercise on Chapter 10 Solutions 19 Questions

Exercise 10.4

$$\textbf{1. Find}\space|\vec{\textbf{a}}×\vec{\textbf{b}}|\textbf{, if\space}\vec{\textbf{a}} = \hat{\textbf{i}}\space\textbf{- 7}\hat{\textbf{j}}\space\textbf{+}\space 7\hat{\textbf{k}}\space\textbf{and}\\ \space \vec{\textbf{b}}\textbf{ = 3}\hat{\textbf{i}}\textbf{- 2}\hat{\textbf{j}}\textbf{+ 2}\hat{\textbf{k}.} $$

Sol. It is given that

$$\vec{a} =\hat{i}-7\hat{j}+7\hat{k}\space\text{and}\space \vec{b} = 3\hat{i}-7\hat{j}+7\hat{k}\\\therefore\space \vec{a}×\vec{b} =\begin{vmatrix}\hat{i}&\hat{j}&\hat{k}\\1& -7&7\\ 3&-2&2\end{vmatrix}\\=(-14+14)\hat{i}-2(2 -21)\hat{j} + (2-21)\hat{k}\\= 0\hat{i}+19\hat{j}+19\hat{k}\\\therefore\space |\vec{a}×\vec{b}| =\sqrt{0^{2} +(19)^{2}+(19)^{2}}\\=\sqrt{2×(19)^{2}}\\=19\sqrt{2}$$

2. Find a unit vector perpendicular to each of the vectors

$$\vec{\textbf{a}}\textbf{ +}\vec{\textbf{b}}\space\textbf{and}\space\vec{\textbf{a}}\textbf{-}\vec{\textbf{b}}\textbf{,}\space\textbf{where}\space\\\vec{\textbf{a}} \textbf{=} \textbf{3}\hat{\textbf{i}}\textbf{+ 2}\hat{\textbf{j}}\textbf{+ 2}\hat{\textbf{k}}\textbf{and}\space\vec{\textbf{b}} \textbf{=}\hat{\textbf{i}} \textbf{+2}\hat{\textbf{j}}\textbf{-2}\hat{\textbf{k}}\textbf{.}$$

Sol. Given that

$$\vec{\text{a}}\space\textbf{=}\space 3\hat{i}+2\hat{j}+2\hat{k}\space\text{and}\space\vec{b} =\hat{i}+2\hat{j}-2\hat{k}\\\therefore\space\vec{a}+\vec{b} =(3\hat{i} + 2\hat{j} +2\hat{k}) + (\hat{i} + 2\hat{j}-2\hat{k})\\=4\hat{i} +4\hat{j} + 0\hat{k}\\\text{and}\space \vec{a} -\vec{b} = (3\hat{i}+2\hat{j}+2\hat{k}) -(\hat{i} + 2\hat{j}-2\hat{k})\\=2\hat{i}+4\hat{k}\\\text{Now,} \space(\vec{a} +\vec{b})×(\vec{a} - \vec{b}) =\begin{vmatrix}\hat{i}&\hat{j}&\hat{k}\\4 &4 &0\\ 2& 0 &4\end{vmatrix}\\=\hat{i}(16-0)-\hat{j}(16-0)+\hat{k}(0-8)\\= 16\hat{i} - 16\hat{j}-8\hat{k}\\\Rarr\space |\vec{a} + \vec{b} × (\vec{a} -\vec{b})| = \\\sqrt{(16)^{2} + (-16)^{2}+(-8)^{2}}$$

$$= \sqrt{256 + 256 + 64}\\=\sqrt{576} = 24\\\text{A unit vector, perpendicular to both}\\(\vec{a} +\vec{b})\space\text{and}\space (\vec{a} -\vec{b})\space\text{is}\\\pm\space\frac{(\vec{a} +\vec{b})×(\vec{a} - \vec{b})}{|(\vec{a} + \vec{b})×(\vec{a} - \vec{b})|} \\=\pm\frac{16\hat{i} -16\hat{j} - 8\hat{k}}{24}\\=\pm\frac{8(2\hat{i}- 2\hat{j}-1\hat{k})}{24}\\=\pm\frac{1}{3}(2\hat{i}- 2\hat{j}-\hat{k})$$

∴ Required vector is either

$$\frac{2}{3}\hat{i}-\frac{2}{3}\hat{J} -\frac{2}{3}\hat{k}\space\text{or}\\-\frac{2}{3}\hat{i}+\frac{2}{3}\hat{j} +\frac{1}{3}\hat{k}.$$

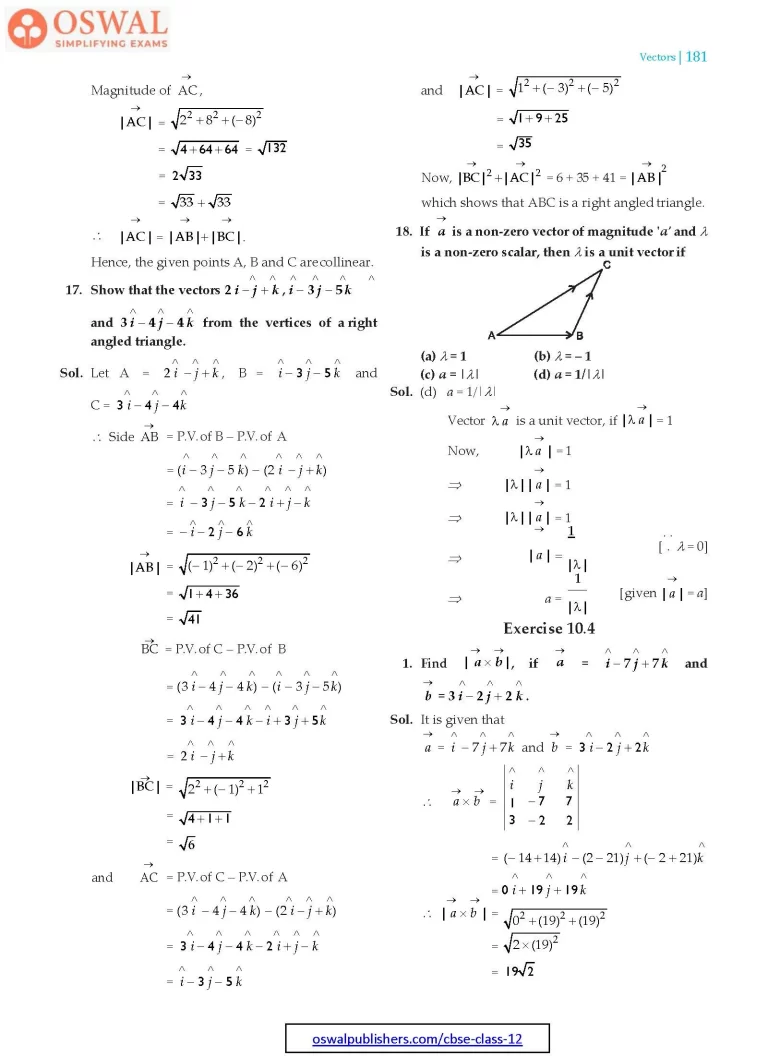
3. If a unit vector

$$\vec{\textbf{a}}\textbf{,}\space\textbf{makes angles}\space\frac{\pi}{\textbf{3}}\space\textbf{with}\space\hat{\textbf{i}},\frac{\pi}{\textbf{4}}\\\space\textbf{with}\space\hat{j}\space\textbf{and an acute angle}\space\theta\space\hat{k}\textbf{,}\\\textbf{then find}\space\theta\space\\\textbf{and hence the components of}\space\vec{\textbf{a}}.$$

Sol. Let unit vector makes angle,

$$\alpha,\beta\space\text{with}\space\gamma\space\text{with}\space\hat{i},\hat{j},\space\text{and}\space\hat{k}\\\space\text{respectively, then}\\\alpha =\frac{\pi}{3},\beta = \frac{\pi}{4}\space\text{and}\space\gamma =\theta\space(\text{Given})\\\therefore\space\text{cos}^{2}\frac{\pi}{3} + \text{cos}^{2}\frac{\pi}{4} + \text{cos}^{2}\theta = 1\\\Rarr\space\bigg(\frac{1}{2}\bigg) ^{2} + \bigg(\frac{1}{\sqrt{2}}\bigg)^{2} + \text{cos}^{2}\theta=1\\\Rarr\space\frac{1}{4} + \frac{1}{2} + \text{cos}^{2}\theta =1\\\therefore\space \text{cos}^{2}\theta = 1-\frac{3}{4}\\\Rarr\space \text{cos}^{2}\theta = \frac{4-3}{4} = \frac{1}{4}$$

$$\Rarr\space \text{cos}\space\theta =\pm \frac{1}{2}\\\text{cos}\space\theta= \frac{1}{2}\\\bigg[\text{cos}\space\theta \neq\frac{1}{2},\therefore\theta\space\text{is an acute angle}\bigg]\\\Rarr\space\theta = \text{cos}^{\normalsize-1}\bigg(\frac{1}{2}\bigg) \\= \text{cos}^{\normalsize-1}\bigg(\text{cos}\frac{\pi}{3}\bigg) = \frac{\pi}{3}\\\text{Also, z = cos}\space\theta = \text{cos}\space60\degree =\frac{1}{2}\\\text{Hence\space x = }\frac{1}{2}, y =\frac{1}{\sqrt{2}}\space\text{and}\space z= \frac{1}{2}$$

$$\therefore\space\text{The required vector}\space\vec{a}\\\space\text{is}\space\frac{1}{2}\hat{i} + \frac{1}{\sqrt{2}}\hat{j} + \frac{1}{2}\hat{k}.\\\text{So, the components of}\\\space\vec{a}\space\text{are} \frac{1}{2},\frac{1}{\sqrt2}\text{and}\frac{1}{2}.$$

4. Show that

$$\textbf{(}\vec{\textbf{a}} \textbf{-}\vec{\textbf{b}})\textbf{×(}\vec{\textbf{a}}\textbf{+}\vec{\textbf{b}}) \textbf{= 2}\textbf{(}\vec{\textbf{a}}\textbf{×}\vec{\textbf{b}}\textbf{).}\\\textbf{Sol.\space}\text{Given that}\\\space (\vec{a}-\vec{b})×(\vec{a} +\vec{b}) = 2(\vec{a}×\vec{b})\\\text{LHS} = (\vec{a} -\vec{b})×(\vec{a} + \vec{b})\\=\vec{a}×\vec{a} + \vec{a}×\vec{b}-\vec{b}×\vec{a}-\vec{b}×\vec{b}\\=\vec{0}+\vec{a}×\vec{b}+\vec{a}×\vec{b}-\vec{0}\\\lbrack\because\space \vec{a}×\vec{a} = \vec{0}\space\text{and}\space \vec{a}×\vec{b} =-\vec{b}×\vec{a}\rbrack\\=2(\vec{a}×\vec{b}) =\text{R.H.S}\space\textbf{Hence Proved.}$$

$$\textbf{5.Find}\space\lambda\textbf{and}\space\mu,\textbf{if}\space\textbf{(2}\hat{\textbf{i}}\textbf{+6}\hat{\textbf{j}}\textbf{+27}\hat{\textbf{k}}\textbf{)×}\\\textbf{(}\hat{\textbf{i}}\textbf{+}\lambda\hat{\textbf{j}}\textbf{+}\mu\hat{\textbf{k}})\textbf{= 0}$$

Sol. Given,

$$(2\hat{i} + 6\hat{j} + 27\hat{k})×(\hat{i} + \lambda\hat{j} +\mu\hat{k}) = 0\\\Rarr\space\begin{vmatrix}\hat{i}&\hat{j} &\hat{k}\\ 2&6 &27\\ 1&\lambda &\mu\end{vmatrix} = 0\\\Rarr\space \hat{i}(6\mu - 27\lambda) -\hat{j}(2\mu- 27) +\hat{k}(2\lambda -6)\\= 0\hat{i} + 0\hat{j}+0\hat{k}$$

On comparing the corresponding componen ts, we have

$$6\mu - 27\lambda = 0\\-2\mu + 27 = 0\\2\lambda - 6 =0\\\Rarr\space 2\mu = -9\lambda\\\mu = \frac{27}{2}\\\text{and}\space \lambda = 3\\\text{Hence,}\space\lambda = 3\space\text{and}\space\mu = \frac{27}{2}.$$

$$\textbf{6.\space Give that\space}\vec{\textbf{a}}.\vec{\textbf{b}}\textbf{= 0}\space\textbf{and}\space \vec{\textbf{a}}\textbf{×}\vec{\textbf{b}}\textbf{= 0}.$$

What can you conclude about the vectors

$$\vec{\textbf{a}}\space\textbf{and}\space\vec{\textbf{b}}\space\textbf{?}\\\textbf{Sol.\space}\text{Given that}\space \vec{a}.\vec{b} = 0\\\text{Then, either}\space |\vec{a}| = 0\space\text{or}\space|\vec{b}| =0\space\text{or}\space \vec{a}\perp\vec{b}\\\text{in case}\space\vec{a}\space\text{and}\space\vec{b}\space\text{are non-zero) and if}\space\vec{a}×\vec{b}=0\\\text{then either}|\vec{a}| =0\space\text{or}|\vec{b}| = 0\space\text{or}\space\vec{a}||\vec{b}\\(\text{In case}\space\vec{a}\text{and}\space\vec{b}\space\text{are non-zero}).\\\text{But\space}\vec{a}\space\text{and}\space\vec{b}\space\text{cannnot be perpendicular}\\\text{ and parallel simultaneously.}\\\text{Hence,}|\vec{a}| =0\space\text{or}\space|\vec{b}|=0.$$

$$\textbf{7. Let the vectors}\space\vec{\textbf{a}},\vec{\textbf{b}},\vec{\textbf{c}}\space\textbf{be given as}\\ \textbf{a}_\textbf{1}\hat{\textbf{i}} \textbf{+ a}_\textbf{2}\hat{\textbf{j}} \textbf{+ a}_\textbf{3}\hat{\textbf{k}},\space \textbf{b}_\textbf{1}\hat{\textbf{i}} \textbf{+ b}_\textbf{2}\textbf{j} \textbf{+ b}_\textbf{3}\hat{\textbf{k}},\\\textbf{c}_1\hat{\textbf{i}} + \textbf{c}_\textbf{2}\hat{\textbf{j}} + \textbf{c}_\textbf{3}\hat{\textbf{k}},\space\textbf{then show that}\space\\\vec{\textbf{a}}\textbf{×(}\vec{\textbf{b}} \textbf{+} \vec{\textbf{c}}) \textbf{=}\space \vec{\textbf{a}}\textbf{×}\vec{\textbf{b}}\textbf{+}\vec{\textbf{a}}\textbf{×}\vec{\textbf{c}}$$

Sol. Given,

$$\vec{a} = a_1\hat{i}+a_2\hat{j} + a_3\hat{k}, \vec{b} = b_1\hat{i}+b_2\hat{j}+b_3\hat{k}\\\text{and}\space \vec{c} = c_1\hat{i} + c_2\hat{j} + c_3\hat{k}\\\text{Now,}\vec{b} +\vec{c} = \\(b_1 + c_1)\hat{i} + (b_2 + c_2)\hat{j} +(b_3 + c_3)\hat{k}\\\therefore\space \vec{a}×(\vec{b} +\vec{c}) = \begin{vmatrix}\hat{i}&\hat{j}&\hat{k}\\ a_1&a_2& a_3\\b_1+c_1 &b_2 +c_2 &b_3 +c_3\end{vmatrix}\\=\begin{vmatrix}\hat{i} &\hat{j} &\hat{k}\\ a_1 &a_2 &a_3\\ b_1 &b_2 &b_3\end{vmatrix} +\begin{vmatrix}\hat{i} &\hat{j} & \hat{k}\\ a_1& a_2 &a_3\\ c_1 & c_2 & c_3\end{vmatrix}\\\text{...(i)}$$

[Using the properties of determinant]

$$\text{Now,\space}\vec{a}×\vec{b} =\begin{vmatrix}\hat{i} &\hat{j} & \hat{k}\\ a_1 &a_2 &a_3\\ b_1 &b_2 &b_3\end{vmatrix}\\\text{and}\space\vec{a}×\vec{c} = \begin{vmatrix}\hat{i} &\hat{j} &\hat{k}\\ a_1 &a_2 &a_3\\ c_1 &c_2 &c_3\end{vmatrix}\\\therefore\space(\vec{a}×\vec{b})+(\vec{a}×\vec{c}) =\\\begin{vmatrix}\hat{i}& \hat{j}& \hat{k}\\ a_1 & a_2 & a_3\\ b_1 & b_2 &b_3\end{vmatrix}+\begin{vmatrix}\hat{i} &\hat{j} &\hat{k}\\ a_1 &a_2 &a_3\\ c_1 &c_2&c_3\end{vmatrix}\\\text{...(iii)}$$

From equations (i) and (ii), we get

$$\vec{a}×(\vec{b}+\vec{c}) =\vec{a}×\vec{b} +\vec{a}×\vec{c}$$

$$\textbf{8. If either}\space\vec{\textbf{a}}\textbf{ = 0}\space\textbf{or}\space\vec{\textbf{b}} \textbf{=0}\textbf{,}\space\textbf{then}\space \vec{\textbf{a}}×\vec{\textbf{b}}\space\textbf{= 0.}$$

Is the converse true? Justify your answer with an example.

$$\textbf{Sol.\space}\text{If}\space\vec{a} = 0\space\text{or}\space\vec{b} =0,\\\text{then surely}\space \vec{a}×\vec{b}=0.$$

However, the converse is not true i.e.

$$\text{if}\space\vec{a}×\vec{b} =0,\space\text{then}\space \vec{a} = 0\space\text{or}\space\vec{b} =0\\\text{may not hold.}\\\text{We know}\space \vec{a}×\vec{b} = 0 =\vec{ab}\space\text{sin}\space\theta,\\\text{if}\space \vec{a}\space\text{and}\space\vec{\text{b}}\space\\\text{are parallel, then q = 0° and sin 0° = 0.}\\\therefore\space \vec{a}×\vec{b} =0\\\text{As an example, consider the vectors}\\\vec{a} =\hat{i}, \vec{b} =\hat{i},\text{then}\space\vec{a}\neq 0\space\text{and also}\\\vec{b}\neq 0.\\\text{But}\space \vec{a}×\vec{b} = \hat{i}×\hat{i}=0\\\textbf{Alternate method}\\\text{Let}\space \vec{a} =\hat{i}+\hat{j}+\hat{k}\space\\\text{and}\space \vec{b} = 4\hat{i} + 4\hat{j}+4\hat{k} $$

$$\therefore\space |\vec{a}| =\sqrt{1^{2}+1^{2}+1^{2}}\\=\sqrt{1+1+1} =\sqrt{3}\neq 0\\|\vec{b}| = \sqrt{4^{2}+4^{2}+4^{2}} \\=\sqrt{16+16+16}\\=\sqrt{48} = 4\sqrt{3}\neq0\\\text{Now,\space} \vec{a}×\vec{b} =\begin{vmatrix}\hat{i}&\hat{j}&\hat{k}\\ 1&1&1 \\4&4&4\end{vmatrix}\\=(4-4)\hat{i} -(4-4)\hat{j} + (4-4)\hat{k} = 0\\\text{Thus}\space \vec{a}×\vec{b}=0\space\text{when}|\vec{a}|\neq 0\space\text{and}|\vec{b}|\neq 0.\\\therefore\space\text{If}\space \vec{a}×\vec{b} = 0,\space\text{then it is not necessary that}\\\text{either}\space|\vec{a}|=0\space\text{or}\space|\vec{b}|=0.$$

9. Find the area of the triangle with vertices A(1, 1, 2), B(2, 3, 5) and C(1, 5, 5).

Sol. To determine the area of the triangle, we will use

$$\text{the formula}\space\Delta =\frac{1}{2}|\vec{\text{AB}}×\vec{\text{AC}}|$$

The vertices of triangle ABC are given as A(1, 1, 2), B(2, 3, 5) and C(1, 5, 5).

$$\text{First we find vectors}\space\vec{\text{AB}}\space\text{and}\space\vec{\text{AC}}.\\\text{Now,\space}\vec{\text{AB}} = \text{P.V. of B – P.V. of A}\\=(2\hat{i} + 3\hat{j}+5\hat{k}) -(\hat{i} + \hat{j} +2\hat{k})\\=(2-1)\hat{i} + (3-1)\hat{j} +(5-2)\hat{k}\\= \hat{i}+2\hat{j}+3\hat{k}\\\text{and}\space\vec{\text{AC}} =\text{P.V. of C – P.V. of A}\\=(\hat{i}+ 5\hat{j} + 5\hat{k}(\hat{i}+\hat{j}+2\hat{k})\\=(1-1)\hat{i}+(5-1)\hat{j}+(5-2)\hat{k}\\= 4\hat{j}+3\hat{k}\\\therefore\space \vec{\text{AB}}×\vec{\text{AC}} =\begin{vmatrix}\hat{i}&\hat{j}&\hat{k}\\ 1&2 & 3\\ 0&4&3\end{vmatrix}\\=\hat{i}(6-12) -\hat{j}(3-0)+\hat{k}(4-0)\\=-6\hat{i}-3\hat{j}+4\hat{k}$$

$$\text{Comparing with}\space\vec{X} = x\hat{i}+y\hat{j}+z\hat{k},\\\text{we get}$$

x = – 6, y = – 3, z = 4

$$\therefore\space|\vec{\text{AB}}×\vec{\text{AC}}| =\sqrt{x^{2}+y^{2}+z^{2}}\\=\sqrt{(-6)^{2}+(-3)^{2}+(4)^{2}}\\=\sqrt{36+9+16}=\sqrt{61}\\\text{Area of}\space\Delta \text{ABC}=\frac{1}{2}|\vec{\text{AB}}×\vec{\text{AC}}|\\=\frac{1}{2}×\sqrt{61}=\frac{\sqrt{61}}{2}\text{sq unit.}\\\text{Hence, the area of}\space\Delta \text{ABC}\space\text{is}\frac{\sqrt{61}}{2}\text{sq unit.}$$

10. Find the area of the parallelogram whose adjacent sides are determined by the vectors

$$\vec{\textbf{a}} \textbf{=}\hat{\textbf{i}}\textbf{-}\hat{\textbf{j}}\textbf{+3}\hat{\textbf{k}}\space\textbf{and}\space\vec{\textbf{b}} \textbf{= 2}\hat{\textbf{i}}\textbf{-7}\hat{\textbf{j}}\textbf{+}\hat{\textbf{k}}\textbf{.}$$

Sol. The area of the parallelogram whose adjacent sides are

$$\vec{\textbf{a}}\space\textbf{and}\space\vec{\text{\textbf{b}}}\space\textbf{is}\space\textbf{|}\vec{\textbf{a}}\textbf{×}\vec{\textbf{b}}\textbf{|.}\\\text{Adjacent sides are given as}\space \vec{a} =\hat{i}-\hat{j}+3\hat{k}\space\text{and}\\\vec{\text{b}} = 2\hat{i}-7\hat{j}+\hat{k}\\\therefore\space \vec{a}×\vec{b} =\begin{vmatrix}\hat{i}&\hat{j}&\hat{k}\\1&-1& 3\\ 2 &-7&1\end{vmatrix}\\=\hat{i}(-1+21)-\hat{j}(1-6)+\hat{k}(-7+2)\\=20\hat{i}+5\hat{j}-5\hat{k}\\\text{Comparing with}\space\vec{\text{X}} = x\hat{i}+y\hat{j}+z\hat{k},\\\text{we get}$$

x = 20, y = 5, z = – 5

∴ Area of the parallelogram

$$\Rarr\space |\vec{a}×\vec{b}| =\sqrt{x^{2}+y^{2}+z^{2}}\\=\sqrt{(20)^{2}+5^{2}+(-5)^{2}}\\=\sqrt{450}\\=\sqrt{225×2}\\=15×\sqrt{2}\space\text{sq. unit}$$

Hence, the area of the given parallelogram is

$$15\sqrt{2}\space\text{sq. unit.}$$

$$\textbf{11. Let the vectors}\space\vec{\textbf{a}}\space\textbf{and}\space\vec{\textbf{b}}\space\textbf{be such that}\\\textbf{|}\vec{\textbf{a}}\textbf{|} \textbf{= 3}\space\textbf{and}\space |\vec{\textbf{b}}| =\frac{\sqrt{\textbf{2}}}{\textbf{3}},\textbf{then}\space\vec{\textbf{a}}×\vec{\textbf{b}}\\\textbf{is a unit vector, if the angle between}\\\vec{\textbf{a}}\space\textbf{and}\space\vec{\textbf{b}}\space\textbf{is :}\\\textbf{(a)\space}\frac{\pi}{\textbf{6}}\\\textbf{(b)}\space\frac{\pi}{4}\\\textbf{(c)}\space\frac{\pi}{\textbf{3}}\\\textbf{(d)}\space\frac{\pi}{\textbf{2}}\\\textbf{Sol.\space}\frac{\pi}{4}$$

$$\text{It is given that}\space|\vec{a}|=3\space\text{and}\space|\vec{b}| = \frac{\sqrt{2}}{3}.\\\text{Let} \theta\space \text{be the angle between}\\\vec{a}\space\text{and}\space\vec{\text{b}},\space\text{then}\\|\vec{a}×\vec{b}|=1\\\Rarr\space |\vec{a}||\vec{b}|\text{sin}\space\theta = 1\\\Rarr\space 3×\frac{\sqrt{2}}{3}×\text{sin}\space\theta=1\\\Rarr\space \text{sin}\space\theta = \frac{1}{\sqrt{2}}\\\Rarr\space \theta =\frac{\pi}{4}\\\text{Hence,}\space \vec{a}×\vec{b}\space\\\text{is a unit vector, if the angle between}\\\vec{a}\text{and}\space\vec{b}\space\text{is}\space\frac{\pi}{4}.$$

12. Area of a rectangle having vertices A, B, C and D with position vectors

$$\textbf{-}\hat{\textbf{i}}\textbf{+}\frac{\textbf{1}}{\textbf{2}}\hat{\textbf{j}}\textbf{+4}\hat{\textbf{k}}\textbf{,}\space \hat{\textbf{i}}\textbf{+}\frac{\textbf{1}}{\textbf{2}}\hat{\textbf{j}}\textbf{+4}\hat{\textbf{k}}\textbf{,}\space\hat{\textbf{i}}-\frac{\textbf{1}}{\textbf{2}}\hat{\textbf{j}}\textbf{+4}\hat{\textbf{k}}\\\textbf{and}\space -\hat{\textbf{i}}-\frac{\textbf{1}}{\textbf{2}}\hat{\textbf{j}}+4\hat{\textbf{k}}\space\textbf{respectively is :}\\\textbf{(a)\space}\frac{\textbf{1}}{\textbf{2}}\\\textbf{(b)}\space\textbf{1}\\\textbf{(c)\space}\textbf{2}\\\textbf{(d)\space}\textbf{4}$$

Sol. (c) 2

The position vector of vertices A, B, C and D of rectangle ABCD are given.

$$\text{First, we compute vectors}\space\vec{\text{AB}}\space\text{and}\space\vec{\text{AD}.}\\\text{Now,}\space \vec{\text{AB}} \text{= P.V. of B – P.V. of A}\\=\bigg(\hat{i}+\frac{1}{2}\hat{j}+4\hat{k}\bigg)-\bigg(-\hat{i}+ \frac{1}{2}\hat{j}+4\hat{k}\bigg)\\=\lbrack1-(-1)\hat{i} + \bigg(\frac{1}{2}-\frac{1}{2}\bigg)\hat{j} + (4-4)\hat{k}\rbrack\\=2\hat{i}\\\text{and}\space\vec{\text{AD}} = \text{P.V. of D – P.V. of A}\\=\bigg(-\hat{i}-\frac{1}{2}\hat{j}+4\hat{k}\bigg) -\bigg(-\hat{i}+\frac{1}{2}\hat{j}+4\hat{k}\bigg)\\=\lbrack-1-(-1)\hat{i} + \bigg(-\frac{1}{2}-\frac{1}{2}\bigg)\hat{j} + (4-4)\hat{k}\rbrack\\= -\hat{j}$$

$$\therefore\space \vec{\text{AB}}×\vec{\text{AD}} = \begin{vmatrix}\hat{i}&\hat{j}&\hat{k}\\ 2 &0 &0\\0 &-1 &0\end{vmatrix} =- 2\hat{k}$$

Area of rectangle ABCD

$$=|\vec{\text{AB}}×\vec{\text{AD}}| =\sqrt{(-2)^{2}}$$

= 2 sq. unit

[Now, it is known that the area of a parallelogram whose adjacent sides are

$$\vec{a}\space\text{and}\space\vec{b}\space\text{is}|\vec{a}×\vec{b}|.\rbrack$$

Hence, the area of the rectangle is

$$|\vec{\text{AB}}×\vec{\text{AD}}|= 2\space\text{sq units.}$$

Note : If a and b are the adjacent sides of a parallelogram, then area =

$$|\vec{a}×\vec{b}|.\space\text{If}\space\vec{a}\space\text{and}\space\vec{b}\space\text{are the diagonals of}\space\\\text{a parallelgoram, then area =}\frac{1}{2}|\vec{a}×\vec{b}|$$

Download PDF