NCERT Solutions for Class 12 Maths Chapter 3 Matrix - Exercise 3.2

Access Exercises of Class 12 Maths Chapter 3 – Matrix

Exercise 3.1 Solutions: 10 Questions (7 Short Answers, 3 MCQs)

Exercise 3.2 Solutions: 22 Questions (14 Long, 6 Short, 2 MCQs)

Exercise 3.3 Solutions: 12 Questions (10 Short Answers, 2 MCQs)

Exercise 3.4 Solutions: 18 Questions (4 Long, 13 Short, 1 MCQ)

Miscellaneous Exercise Solutions: 15 Questions (7 Long, 5 Short, 3 MCQs)

Exercise 3.2

$$\textbf{1. \space Let A} \textbf{=}\begin{bmatrix} \textbf{2} & \textbf{4}\\\textbf{3} &\textbf{2}\end{bmatrix}\textbf{, B} \textbf{=}\begin{bmatrix}\textbf{1}&\textbf{3}\\\textbf{-2} &\textbf{5}\end{bmatrix}\textbf{,}\\\textbf{C} \textbf{=}\begin{bmatrix}\normalsize-\textbf{2} & \textbf{5} \\\normalsize-\textbf{2} &\textbf{5}\end{bmatrix}\textbf{.}$$

Find each of the following :

(i) A + B

(ii) A – B

(iii) 3A – C

(iv) AB

(v) BA

$$\textbf{Sol.\space}\textbf{(i)\space}\text{A + B} =\\\begin{bmatrix}2 &4 \\3 &2 \end{bmatrix}+\begin{bmatrix}1 &3\\-2 &5\end{bmatrix}\\=\begin{bmatrix}2+1 &4+3\\3+(\normalsize-2)&2+5\end{bmatrix}\\=\begin{bmatrix}3 &7\\1 &7\end{bmatrix}\\\textbf{(ii)\space}\text{A - B}=\\ \begin{bmatrix} 2 &4 \\3 &2\end{bmatrix}-\begin{bmatrix}1 &3\\-2 &5\end{bmatrix}\\=\begin{bmatrix} 2-1 &4-3 \\3-(-2) &2-5 \end{bmatrix}\\=\begin{bmatrix}1 & 1 \\5 &-3 \end{bmatrix}$$

$$\textbf{(iii)\space}\text{3A-C} \\= 3\begin{bmatrix}2 & 4 \\3 &2 \end{bmatrix}-\begin{bmatrix}-2 & 5 \\3 &4\end{bmatrix}\\=\begin{bmatrix}6 &12\\9 &6\end{bmatrix} -\begin{bmatrix}-2 &5 \\3 &4 \end{bmatrix}\\=\begin{bmatrix}6-(-12) &12-5\\9-3 &6-4\end{bmatrix}\\=\begin{bmatrix}8 &7\\6 &2\end{bmatrix}$$

$$\textbf{(iv)\space}\text{AB} =\begin{bmatrix}2 &4\\3&2\end{bmatrix}\begin{bmatrix}1 &3\\-2&5\end{bmatrix}\\\begin{bmatrix}2×1+4×(\normalsize-2) &2×3+4×5\\3×1+2×(\normalsize-2) &3×3+2×5\end{bmatrix}\\=\begin{bmatrix}-6 &26\\-1 &19\end{bmatrix}\\\textbf{(v)\space}\text{BA} =\begin{bmatrix}1 &3\\-2 &5\end{bmatrix}\begin{bmatrix}2 &4\\3 &2\end{bmatrix}\\=\begin{bmatrix}1×2 +3×3 &1×4+3×2 \\(\normalsize-2)×2+5×3 &(\normalsize-2)×4+5×2\end{bmatrix}\\=\begin{bmatrix}11 &10\\11& 2\end{bmatrix}$$

2. Compute the following :

$$\textbf{(i)\space}\begin{bmatrix} \textbf{a} &\textbf{b}\\\textbf{\normalsize-b} &\textbf{a}\end{bmatrix} \textbf{+} \begin{bmatrix}\textbf{a} &\textbf{b} \\\textbf{b} &\textbf{a} \end{bmatrix}\\\textbf{(ii)\space}\begin{bmatrix}\textbf{a}^{\textbf{2}}+\textbf{b}^{\textbf{2}} &\textbf{b}^{\textbf{2}}+\textbf{c}^{\textbf{2}}\\\textbf{a}^{\textbf{2}} +\textbf{c}^{\textbf{2}} &\textbf{a}^{\textbf{2}}+\textbf{b}^{\textbf{2}}\end{bmatrix}+\\\begin{bmatrix}\textbf{2ab} &\textbf{2bc}\\\textbf{-2ac} &\textbf{-2ab}\end{bmatrix}\\\textbf{(iii)\space}\begin{bmatrix}\normalsize-\textbf{1} &\textbf{4} &\textbf{-6}\\\textbf{8} &\textbf{5} &\textbf{16}\\\textbf{2} &\textbf{8} &\textbf{5}\end{bmatrix}\textbf{+}\\\begin{bmatrix}\textbf{12} &\textbf{7} &\textbf{6} \\\textbf{8} &\textbf{0} &\textbf{5}\\\textbf{3}&\textbf{2}&\textbf{4}\end{bmatrix}\\\textbf{(iv)\space}\begin{bmatrix}\textbf{cos}^{\textbf{2}}\textbf{x} &\textbf{sin}^{\textbf{2}}\textbf{x}\\\textbf{sin}^{\textbf{2}}\textbf{x}&\textbf{cos}^{\textbf{2}}\textbf{x}\end{bmatrix}\textbf{+}\\\begin{bmatrix}\textbf{sin}^{\textbf{2}}\textbf{x} &\textbf{cos}^{\textbf{2}}\textbf{x}\\\textbf{cos}^{\textbf{2}}\textbf{x} &\textbf{sin}^{\textbf{2}}\textbf{x}\end{bmatrix}$$

$$\textbf{Sol.\space}\textbf{(i)}\space\begin{bmatrix}a & b \\-b &a\end{bmatrix} +\begin{bmatrix}a & b \\b &a\end{bmatrix}\\=\begin{bmatrix}a+a & b+b \\-b+b &a+a\end{bmatrix} =\begin{bmatrix} 2a & 2b \\0 &2a\end{bmatrix}.\\\textbf{(ii)\space}\begin{bmatrix}a^{2} + b^{2} & b^{2}+c^{2}\\a^{2}+c^{2} &a^{2}+b^{2}\end{bmatrix}+\\\begin{bmatrix}2ab &2bc \\-2ac &-2ab\end{bmatrix}\\=\begin{bmatrix}a^{2}+b^{2}+2ab & b^{2}+c^{2}+2bc \\a^{2}+c^{2}-2ac &a^{2}+b^{2}-2ab\end{bmatrix}\\=\begin{bmatrix}(a + b)^{2} &(b+c)^{2}\\(a-c)^{2} &(a-b)^{2}\end{bmatrix}\\\begin{bmatrix}\because\space (a+b)^{2} = a^{2}+ 2ab +b^{2}\\(a-b)^{2} = a^{2}-2ab+b^{2}\end{bmatrix}$$

$$\textbf{(iii)\space}\begin{bmatrix}\normalsize-\text{1} &\text{4}&\text{-6}\\\text{8} &\text{5}&\text{16}\\\text{2}&\text{8}&\text{5}\end{bmatrix}+\begin{bmatrix}\text{12} &\text{7} &\text{6}\\\text{8} &\text{0} &\text{5}\\\text{3} &\text{2} &\text{4}\end{bmatrix}\\=\begin{bmatrix}-1+12 &4+7 &-6+6\\8+8 &5+0 &16+5\\ 2+3 &8+2 &5+4\end{bmatrix}\\=\begin{bmatrix}11 &11 &0 \\16 &5 &21\\ 5 &10 &9\end{bmatrix}$$

$$\textbf{(iv)\space}\begin{bmatrix}\text{cos}^{2}x &\text{sin}^{2}x\\\text{sin}^{2}x &\text{cos}^{2}x\end{bmatrix} +\\\begin{bmatrix}\text{sin}^{2}x & \text{cos}^{2}x \\\text{cos}^{2}x &\text{sin}^{2}x\end{bmatrix}\\=\begin{bmatrix}\text{cos}^{2}x + \text{sin}^{2}x &\text{sin}^{2}x +\text{cos}^{2}x \\\text{sin}^{2}x +\text{cos}^{2}x &\text{cos}^{2}x + \text{sin}^{2}x\end{bmatrix}\\=\begin{bmatrix}1 &1\\1 &1\end{bmatrix}\\\lbrack\because\space\text{sin}^{2}x +\text{cos}^{2}x = 1\rbrack$$

3. Compute the indicated products.

$$\textbf{(i)\space}\begin{bmatrix}\textbf{a} &\textbf{b} \\\normalsize-\textbf{b} &\textbf{a} \end{bmatrix}\begin{bmatrix}\textbf{a} & \textbf{\normalsize-b} \\\textbf{b} &\textbf{a} \end{bmatrix}\\\textbf{(ii)\space}\begin{bmatrix}1 \\2\\ 3\end{bmatrix}\begin{bmatrix}\textbf{2} &\textbf{3} &\textbf{4}\end{bmatrix}\\\textbf{(iii)\space}\begin{bmatrix} \textbf{1} & \textbf{\normalsize-2}\\ \textbf{2} & \textbf{3}\end{bmatrix}\begin{bmatrix}\textbf{1} &\textbf{2} &\textbf{3}\\\textbf{2} &\textbf{3} &\textbf{1}\end{bmatrix}\\\textbf{(iv)\space}\begin{bmatrix}\textbf{2} &\textbf{3} &\textbf{4}\\\textbf{3} &\textbf{4} &\textbf{5}\\ \textbf{4} &\textbf{5} &\textbf{6}\end{bmatrix}\begin{bmatrix}\textbf{1} &\textbf{\normalsize-3}&\textbf{\normalsize5} \\\textbf{\normalsize0} &\textbf{\normalsize2}& \textbf{\normalsize4}\\\textbf{\normalsize3} &\textbf{\normalsize0} &\textbf{\normalsize5}\end{bmatrix}\\\textbf{(v)\space}\begin{bmatrix}\textbf{2} &\textbf{1}\\\textbf{3} &\textbf{2}\\\textbf{\normalsize-1}&\textbf{1}\end{bmatrix}\begin{bmatrix}\textbf{1} &\textbf{0} &\textbf{1}\\\textbf{\normalsize-1} &\textbf{2} &\textbf{1}\end{bmatrix}$$

$$\textbf{(vi)\space}\begin{bmatrix}\textbf{3} &\textbf{\normalsize-1} &\textbf{3}\\\textbf{\normalsize-1} &\textbf{\normalsize0} &\textbf{\normalsize2} \end{bmatrix}\begin{bmatrix} \textbf{2} &\textbf{\normalsize-3} \\\textbf{1} &\textbf{0}\\\textbf{3}&\textbf{1}\end{bmatrix}\\\textbf{Sol.\space}(i)\space \begin{bmatrix} a & b \\ -b &a \end{bmatrix}\begin{bmatrix} a & -b \\b &a \end{bmatrix}\\=\\\begin{bmatrix}a×a + b×b &a×(\normalsize-b) +b×a \\(-b)×a +a×b & (\normalsize-b)×(\normalsize-b)+a×a\end{bmatrix}\\ =\begin{bmatrix}a^{2}+b^{2} &-ab+ab\\-ab+ab &b^{2}+a^{2}\end{bmatrix}\\=\begin{bmatrix} a^{2}+b^{2} &0 \\0 &b^{2}+a^{2}\end{bmatrix}$$

$$\textbf{(ii)\space}\begin{bmatrix}1 \\2\\ 3 \end{bmatrix}_{3×1}\space\text{( 2 3 4)}_{1×3}\\=\begin{bmatrix}1×2 &1×3 &1×4 \\2×2 &2×3 &2×4\\3×2 &3×3 &3×4\end{bmatrix}_{3×3}\\=\begin{bmatrix}2 &3 &4 \\4 &6 &8\\ 6&9 &12\end{bmatrix}\\\textbf{(iii)\space}\begin{bmatrix}1 &-2 \\2 &3\end{bmatrix}\begin{bmatrix}1 &2 &3 \\2 &3 &1\end{bmatrix}\\=\begin{bmatrix}1×1+(\normalsize-2)×2 &1×2+(\normalsize-2)×3 &1×3 +(\normalsize-2)×1 \\2×1+3×2 &2×2+3×3 &2×3+3×1 \end{bmatrix}$$

$$= \begin{bmatrix}-3 &-4 &1 \\8 &13 &9 \end{bmatrix}$$

$$\textbf{(iv)\space}\begin{bmatrix}2 & 3 & 4 \\3 &4 &5\\ 4 &5 &6\end{bmatrix}\begin{bmatrix} 1 &-3 &5\\0 &2 &4\\ 3&0 &5\end{bmatrix}\\=\\\begin{bmatrix} 2+0+12 &-6+6+0 &10+12+20\\3+0+15 &-9+8+0 &15+16+25\\4+0+18 &-12+10+0 &20+20+30\end{bmatrix}\\=\begin{bmatrix} 14 &0 &42 \\18 &\normalsize-1 &56\\ 22 &\normalsize-2 &70\end{bmatrix}$$

$$\textbf{(v)\space}\begin{bmatrix}2 &1 \\3 &2\\\normalsize-1 &1\end{bmatrix}\begin{bmatrix}1 &0 &1\\\normalsize-1 &2 &1 \end{bmatrix}\\=\begin{bmatrix}2-1 &0+2 &2+1\\3-2 &0+4 &3+2\\-1-1 &0+2 &-1+1\end{bmatrix}\\=\begin{bmatrix}1 &2 &3 \\1 &4 &5\\ -2 &2 &0\end{bmatrix}$$

$$\textbf{(vi)}\begin{bmatrix}3 &\normalsize-1 &3 \\\normalsize-1 &0 &2\end{bmatrix}\begin{bmatrix} 2 &-3\\1 &0\\ 3 &1\end{bmatrix}\\=\begin{bmatrix} 6-1+9 &-9+0+3 \\-2+0+6 &3+0+2 \end{bmatrix}\\=\begin{bmatrix}14 &-6 \\4 &5 \end{bmatrix}$$

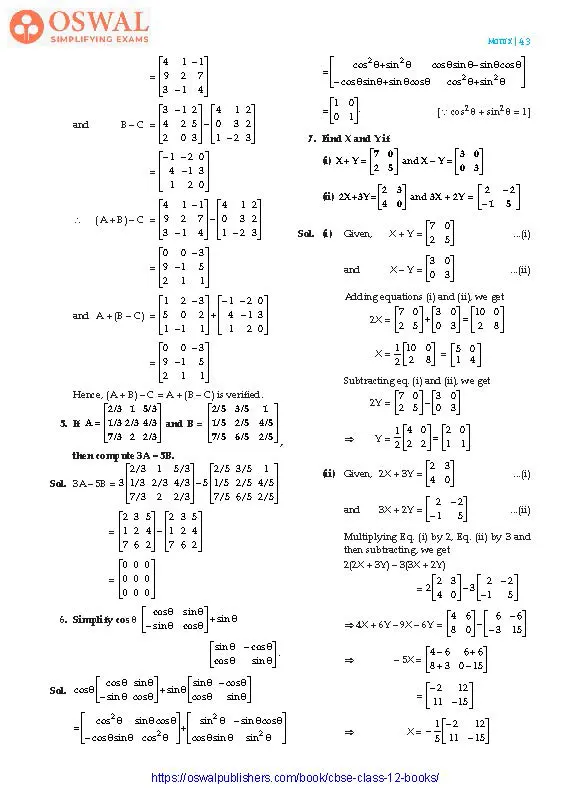
$$\textbf{4.\space If\space A =}\begin{bmatrix} \textbf{1} &\textbf{2} &\textbf{\normalsize-3}\\\textbf{5} &\textbf{0} &\textbf{2}\\ \textbf{1}&\normalsize-\textbf{1} &\textbf{1}\end{bmatrix}\textbf{,}\textbf{B} =\begin{bmatrix}\textbf{3} &\normalsize-\textbf{1} &\textbf{2}\\\textbf{4} &\textbf{2} &\textbf{5}\\ \textbf{2} &\textbf{0} &\textbf{3}\end{bmatrix}\textbf{,}\\\textbf{C} =\begin{bmatrix}\textbf{4} &\textbf{1} &\textbf{2}\\\textbf{0} &\textbf{3} &\textbf{2}\\\textbf{1} &\normalsize-\textbf{2} &\textbf{3}\end{bmatrix} $$

then compute (A + B) and (B – C). Also verify that A + (B – C) = (A + B) – C.

Sol. A + B =

$$\begin{bmatrix} 1 &2 &-3\\5 &0 &2\\ 1 &\normalsize-1 &1\end{bmatrix} + \begin{bmatrix} 3 &\normalsize-1 &2 \\4 &2 &5\\ 2 &0 &3\end{bmatrix}\\=\begin{bmatrix}4 &1 &\normalsize-1\\9 &2 &7\\3 &\normalsize-1 &4\end{bmatrix}\\\text{and}$$

$$\text{B-C} =\\\begin{bmatrix}3 &\normalsize-1 &2\\4 &2 &5\\ 2 &0 &3 \end{bmatrix}-\begin{bmatrix} 4 &1 &2\\0 &3 &2\\ 1 &\normalsize-2 &3\end{bmatrix}\\=\begin{bmatrix}\normalsize-1 &\normalsize-2 &0 \\4 & \normalsize-1 &3\\ 1&2 &0\end{bmatrix}\\\therefore\space\text{(A+B) - C}\\=\\\begin{bmatrix}4 &1 &\normalsize-1\\9 &2 &7\\ 3 &\normalsize-1 &4\end{bmatrix}-\begin{bmatrix} 4 &1 &2\\0 &3 &2\\ 1&\normalsize-2 &3\end{bmatrix}\\=\begin{bmatrix}0 &0 &\normalsize-3\\9 &\normalsize-1 &5\\ 2& 1 & 1\end{bmatrix}$$

Hence, (A + B) – C = A + (B – C) is verified.

$$\textbf{5. If A} = \begin{bmatrix}\frac{\textbf{2}}{\textbf{3}} &\textbf{1} &\frac{\textbf{5}}{\textbf{3}}\\\frac{\textbf{1}}{\textbf{3}} &\frac{\textbf{2}}{\textbf{3}}&\frac{\textbf{4}}{\textbf{3}}\end{bmatrix}\\\textbf{and}\space \textbf{B}\begin{bmatrix}\frac{\textbf{2}}{\textbf{5}} &\frac{\textbf{3}}{\textbf{5}} &\textbf{1} \\\frac{\textbf{1}}{\textbf{5}} &\frac{\textbf{2}}{\textbf{5}} &\frac{\textbf{4}}{\textbf{5}}\\\frac{\textbf{7}}{\textbf{5}} &\frac{\textbf{6}}{\textbf{5}} &\frac{\textbf{2}}{\textbf{5}}\end{bmatrix}$$

then compute 3A – 5B.

Sol. 3A – 5B =

$$3\begin{bmatrix}\frac{2}{3} &1 &\frac{5}{3}\\\frac{1}{3} &\frac{2}{3} &\frac{4}{3}\\\frac{7}{3} &2 &\frac{2}{3}\end{bmatrix}-5\begin{bmatrix}\frac{2}{3} &\frac{3}{5} &1\\\frac{1}{5} &\frac{2}{5} &\frac{4}{5}\\ \frac{7}{5} &\frac{6}{5} &\frac{2}{5}\end{bmatrix}\\=\begin{bmatrix}2 &3 &5\\1 &2 &4\\ 7 &6 &2\end{bmatrix}-\begin{bmatrix}2 &3 &5\\1 &2 &4\\ 7 &6 &2\end{bmatrix}\\=\begin{bmatrix}0 &0 &0 \\0 &0 &0\\0 &0 &0 \end{bmatrix}$$

6. Simplify

$$\textbf{cos}\space\theta\begin{bmatrix}\textbf{cos}\space\theta &\textbf{sin}\space\theta \\\normalsize-\textbf{sin}\theta &\textbf{cos}\theta\end{bmatrix} +\\ \textbf{sin}\space\theta\begin{bmatrix}\textbf{sin}\space\theta &\normalsize-\textbf{cos}\space\theta \\\textbf{cos}\space\theta &\textbf{sin}\space\theta\end{bmatrix}\textbf{.}\\\textbf{Sol.\space}\text{cos}\space\theta\begin{bmatrix} \text{cos}\space\theta &\text{sin}\space\theta \\-\text{sin}\space\theta &\text{cos}\space\theta\end{bmatrix} +\\\text{sin}\space\theta\begin{bmatrix}\text{sin}\space\theta &-\text{cos}\space\theta\\\text{cos}\space\theta &\text{sin}\space\theta\end{bmatrix}$$

$$= \begin{bmatrix}\text{cos}^{2}\theta &\text{sin}\space\theta\text{cos}\theta\\-\text{cos}\theta\text{sin}\theta&\text{cos}^{2}\theta\end{bmatrix}+\\\begin{bmatrix}\text{sin}^{2}\theta &-\text{sin}\theta\text{cos}\theta\\\text{cos}\theta\text{sin}\theta &\text{sin}^{2}\theta \end{bmatrix}\\=\space \begin{bmatrix}\text{cos}^{2}\theta + \text{sin}^{2}\theta &\text{cos}\theta\text{sin}\theta -\text{sin}\theta \text{cos}\theta\\-\text{cos}\theta\text{sin}\theta + \text{sin}\theta\text{cos}\theta &\text{cos}^{2}\theta +\text{sin}^{2}\theta\end{bmatrix}\\=\begin{bmatrix}1 &0 \\0 &1\end{bmatrix}\\\lbrack\because\space \text{cos}^{2}\theta + \text{sin}^{2}\theta =1\rbrack$$

$$\textbf{7. Find X and Y if}\\\textbf{(i)}\space \textbf{X + Y} \textbf{=}\begin{bmatrix}\textbf{7} &\textbf{0} \\\textbf{2} &\textbf{5}\end{bmatrix}\space\textbf{and}\\\textbf{X-Y} \textbf{=}\begin{bmatrix}\textbf{3} &\textbf{0} \\ \textbf{0} &\textbf{3} \end{bmatrix}\\\textbf{(ii)\space} \textbf{2X + 3Y} =\begin{bmatrix}\textbf{2} &\textbf{3} \\\textbf{4} &\textbf{0}\end{bmatrix}\space\textbf{and}\space\\ \textbf{3X + 2Y} \textbf{=}\begin{bmatrix}\textbf{2} &\textbf{\normalsize-2} \\\normalsize-\textbf{1} &\textbf{5}\end{bmatrix}$$

Sol. (i) Given,

$$\text{X+Y} =\begin{bmatrix}7 &0 \\2 &5\end{bmatrix}\space\text{...(i)}\\\text{and}\space \text{X-Y} =\begin{bmatrix}3 &0 \\0 &3\end{bmatrix}\\\text{...(ii)}$$

Adding equations (i) and (ii), we get

$$2X = \begin{bmatrix} 7 &0 \\2 &5\end{bmatrix} + \begin{bmatrix}3 & 0 \\0 &3\end{bmatrix}\\=\begin{bmatrix}10 &0 \\2 &8 \end{bmatrix}\\\text{X}=\frac{1}{2}\begin{bmatrix}10 &0 \\2 &8\end{bmatrix}\\=\begin{bmatrix}5 &0 \\1 &4\end{bmatrix}$$

Subtracting eq. (i) and (ii), we get

$$\text{2Y} =\begin{bmatrix}7 &0 \\2 &5\end{bmatrix}-\begin{bmatrix}3 &0 \\0 &3 \end{bmatrix}\\\Rarr\space \text{Y} = \frac{1}{2}\begin{bmatrix}4 &0 \\2 &2 \end{bmatrix}=\begin{bmatrix}2 &0 \\1 & 1\end{bmatrix}\\\text{(ii)\space}\text{Given},\space\\\text{2X + 3Y} = \begin{bmatrix}2 &3\\4 &0\end{bmatrix}\\\text{...(i)}\\\text{and}\space\text{3X + 2Y} =\begin{bmatrix}2 &\normalsize-2 \\\normalsize-1 & 5\end{bmatrix}\\\text{...(ii)}$$

Multiplying Eq. (i) by 2, Eq. (ii) by 3 and then subtracting, we get

2(2X + 3Y) – 3(3X + 2Y)

$$ = 2\begin{bmatrix}2 &3 \\4 &0\end{bmatrix}-3\begin{bmatrix} 2 &\normalsize-2 \\\normalsize-1 &5\end{bmatrix}\\\Rarr\space 4X + 6Y-9X-6Y\\=\begin{bmatrix}4 &6 \\8 &0\end{bmatrix}-\begin{bmatrix}6 &-6 \\-3 &15 \end{bmatrix}\\\Rarr\space -5X = \begin{bmatrix}4-6 & 6+6 \\8+3 &0-15\end{bmatrix}\\=\begin{bmatrix}-2 &12 \\11 &-15\end{bmatrix}\\\Rarr\space \text{X} =-\frac{1}{5}\begin{bmatrix}\normalsize-2 &12 \\ 11 &\normalsize-15 \end{bmatrix}\\=\begin{bmatrix}\frac{2}{5} &-\frac{12}{5} \\-\frac{11}{5} &3\end{bmatrix}\\\therefore\space\text{Y} =\frac{1}{3}\begin{bmatrix}\frac{6}{5} &\frac{39}{5}\\\frac{42}{5} &-6 \end{bmatrix}$$

$$=\begin{bmatrix}\frac{2}{5} &\frac{13}{5} \\\frac{14}{5} &-2 \end{bmatrix}$$

$$\textbf{8. Find X, if Y =}\begin{bmatrix}\textbf{3} &\textbf{2} \\\textbf{1} &\textbf{4}\end{bmatrix}\\\textbf{and \space 2X+Y} = \begin{bmatrix} \textbf{1} &\textbf{0} \\\textbf{\normalsize-3} &\textbf{2}\end{bmatrix}\textbf{.}\\\textbf{Sol.\space}\text{Given, 2X + Y =}\begin{bmatrix}1 &0 \\\normalsize-3 &2\end{bmatrix}\\\text{and Y} = \begin{bmatrix}3 &2 \\1 & 4 \end{bmatrix}\\\Rarr\space \text{2X} = \begin{bmatrix} 1 &0 \\\normalsize-3 &2\end{bmatrix}- \text{Y}\\=\begin{bmatrix}1 &0 \\\normalsize-3 &2 \end{bmatrix} - \begin{bmatrix}3 &2 \\1 &4\end{bmatrix}\\=\begin{bmatrix} 1-3 & 0-2 \\-3-1 &2-4\end{bmatrix} = \begin{bmatrix}\normalsize-2 \normalsize-2\\ -4 \normalsize-2\end{bmatrix}$$

$$\Rarr\space\text{X}=\frac{1}{2}\begin{bmatrix}\normalsize-2 &\normalsize-2 \\\normalsize-4 & \normalsize-2 \end{bmatrix}\\=\begin{bmatrix}\normalsize-1 & \normalsize-1 \\ \normalsize-2 & \normalsize-1 \end{bmatrix}$$

$$\textbf{9. Find x and y, if} \\\textbf{2}\begin{bmatrix}\textbf{1} &\textbf{3} \\ \textbf{0} &\textbf{x}\end{bmatrix} +\begin{bmatrix}\textbf{y} & \textbf{0} \\\textbf{1} &\textbf{2} \end{bmatrix}\\\begin{bmatrix}\textbf{5} & \textbf{6} \\ \textbf{1} & \textbf{8} \end{bmatrix}\textbf{.}$$

Sol. Given,

$$2\begin{bmatrix}1 &3 \\0 &x \end{bmatrix}+ \begin{bmatrix} y & 0\\1 &2\end{bmatrix}=\begin{bmatrix}5 &6 \\1 &8\end{bmatrix}\\\begin{bmatrix}2 & 6 \\0 &2x \end{bmatrix} +\begin{bmatrix} y &0 \\1 &2 \end{bmatrix}=\begin{bmatrix} 5 &6 \\ 1 &8 \end{bmatrix}\\\Rarr\space \begin{bmatrix} 2+y & 6+0 \\ 0+1 & 2x+2\end{bmatrix}\\=\begin{bmatrix} 5 & 6 \\ 1 & 8 \end{bmatrix}$$

By definition of equality of matrix as the given
matrices are equal, their corresponding elements
are equal. Comparing the corresponding
elements, we get

2 + y = 5 ...(i)

and 2x + 2 = 8 ...(ii)

⇒ y = 5 –2 = 3

and 2x = 8 – 2

$$\Rarr\space \text{y= 3 and x} =\frac{6}{2}=3$$

10. Solve the equation for x, y, z and t, if

$$\textbf{2}\begin{bmatrix} \textbf{x} &\textbf{z} \\ \textbf{y} & \textbf{t}\end{bmatrix}\textbf{+ 3}\begin{bmatrix}\textbf{1} &\textbf{\normalsize-1} \\\textbf{0} & \textbf{2}\end{bmatrix}\\\textbf{= 3}\begin{bmatrix} \textbf{3} & \textbf{5} \\\textbf{4} & \textbf{6}\end{bmatrix}\\\textbf{Sol. \space}\text{Given,\space}$$

$$2\begin{bmatrix}x & z \\ y & t\end{bmatrix} + 3\begin{bmatrix}1 & \normalsize-1 \\ 0 & 2 \end{bmatrix}\\=3\begin{bmatrix} 3 & 5 \\ 4 & 6 \end{bmatrix}\\\Rarr\space\begin{bmatrix} 2x & 2z \\ 2y & 2t\end{bmatrix} +\begin{bmatrix} 3 & \normalsize-3 \\ 0 & 6 \end{bmatrix}\\=\begin{bmatrix} 9 & 15 \\12 & 18 \end{bmatrix}\\\Rarr\space \begin{bmatrix}2x+3 &2z-3 \\2y+0 & 2t+6\end{bmatrix}\\=\begin{bmatrix}9 & 15 \\12 & 8\end{bmatrix}$$

By definition of equality of matrix as the given matrices are equal, then corresponding elements are equal. Comparing the corresponding elements, we get

2x + 3 = 9, 2y + 0 = 12, 2z – 3 = 15 and 2t + 6 = 18

$$\Rarr\space x = \frac{9-3}{2},\space y =\frac{12}{2},\\\text{z}= \frac{15+3}{2}\text{and}\space t =\frac{18-6}{2}\\\Rarr\space \text{x = 3, y = 6, z=9}\\\text{and t = 6.} $$

$$\textbf{11.\space}\textbf{If x}\begin{bmatrix}\textbf{2} \\\textbf{3} \end{bmatrix} \textbf{+ y}\begin{bmatrix} \normalsize-\textbf{1}\\\textbf{1}\end{bmatrix} = \begin{bmatrix}\textbf{10} \\\textbf{5} \end{bmatrix}\textbf{,}\\\textbf{find the values of x and y}\\\textbf{Sol.}\space\text{If x}\begin{bmatrix}2 \\3\end{bmatrix} + y\begin{bmatrix}\normalsize-1 \\1\end{bmatrix} =\begin{bmatrix}10 \\5\end{bmatrix}\\\Rarr\space\begin{bmatrix}2x+y(\normalsize-1)\\3x+y\end{bmatrix} =\begin{bmatrix}10 \\5\end{bmatrix}$$

By definition of equality of matrix as the given
matrices are equal, their corresponding elements
are equal. Comparing the corresponding elements, we get

2x – y = 10 ...(i)

and 3x + y = 5 ...(ii)

Adding equations, (i) and (ii), we get

5x = 15 ⇒ x = 3

Substituting x = 3 in Eq. (i), we get

2 × 3 – y = 10

and y = 6 – 10 = – 4

$$\textbf{12. Given}\space 3\begin{bmatrix}\textbf{x} & \textbf{y}\\\textbf{z} & \textbf{w}\end{bmatrix}\\=\begin{bmatrix}\textbf{x} & \textbf{6} \\\normalsize-\textbf{1} &\textbf{2w}\end{bmatrix} +\begin{bmatrix}\textbf{4} & \textbf{x+y} \\\textbf{z+w} & \textbf{3}\end{bmatrix}\textbf{,}$$

find the values of x, y, z and w.

$$\textbf{Sol.\space}\text{Given,\space} 3\begin{bmatrix}x & y \\z & w \end{bmatrix}=\\\begin{bmatrix}x & 6 \\\normalsize-1 & 2w\end{bmatrix} +\begin{bmatrix}4 & x+y \\z+w & 3\end{bmatrix}\\\Rarr\space\begin{bmatrix}3x & 3y \\3z & 3w\end{bmatrix} = \begin{bmatrix} x+4 & 6+x+y \\\normalsize-1+z+w & 2w+3\end{bmatrix}$$

By definition of equality of matrix as the given matrices are equal, their corresponding elements are equal. Comparing the corresponding elements, we get

3x = x + 4 ⇒ 2x = 4 ⇒ x = 2

and 3y = 6 + x + y ⇒ 2y = 6 + x

$$\Rarr\space y =\frac{6+x}{2}$$

Putting the value of x, we get

$$y =\frac{6+2}{2} =\frac{8}{2}=4$$

Now, 3z = – 1 + z + w,

2z = – 1 + w

$$z =\frac{\text{-1 + w}}{2}\space\text{...(i)}$$

Now, 3w = 2w + 3 ⇒ w = 3

Putting the value of w in Eq. (i), we get

$$z = \frac{-1+3}{2} =\frac{2}{2}=1$$

Hence, the values of x, y, z and w are 2, 4, 1 and 3.

$$\textbf{13.\space IF F(x)} \textbf{=} \begin{bmatrix}\textbf{cos x} & \textbf{- sin x} &\textbf{0} \\\textbf{sin x} &\textbf{cos x} &\textbf{0}\\\textbf{0} &\textbf{0} &\textbf{1}\end{bmatrix}\textbf{,}\\\textbf{then show that}\\\textbf{F(x)F(y) = F(x + y).}$$

Sol. LHS = F(x).F(y)

$$ = \begin{bmatrix}\text{cos x} &\text{- sin x} &0\\\text{sin x} &\text{cos x} &0\\0 &0 &1\end{bmatrix}\\\begin{bmatrix}\text{cos}\space y &\text{- sin}\space y &0\\\text{sin}\space y &\text{cos}\space y &0\\ 0 &0 &1\end{bmatrix}\\=\\\begin{bmatrix}\text{cos x cos y - sin x siny} & \text{- sin y cos x - sin x\space cos y }&0 \\\text{sin x cos y + cos x sin y} &-\text{sin x siny + cos x cos y} &0\\0 &0 &1\end{bmatrix}\\\begin{bmatrix}\because \text{cos (A+B) = cos A cos B - sin A sin B}\\\text{sin}(\text{A+B}) =\text{sin A cos B + sin B cos A}\end{bmatrix}\\=\begin{bmatrix}\text{cos}(x+y) &-\text{sin(x+y)}&0\\\text{sin}(x+y) &\text{cos}(x+y) &0\\ 0 &0 &1\end{bmatrix}$$

F(x) F(y) = F(x + y) = RHS.

Hence proved.

14. Show that

$$\textbf{(i)\space}\begin{bmatrix}\textbf{5} & \textbf{\normalsize-1} \\\textbf{6} & \textbf{7}\end{bmatrix}\begin{bmatrix}\textbf{2} &\textbf{1} \\\textbf{3} & \textbf{4}\end{bmatrix}\neq\\\begin{bmatrix}\textbf{2} &\textbf{1} \\\textbf{3} & \textbf{4}\end{bmatrix}\begin{bmatrix}\textbf{5} & \normalsize\textbf{\normalsize-1} \\\textbf{6} & \textbf{7}\end{bmatrix}\\\textbf{(ii)\space}\begin{bmatrix}\textbf{1} &\textbf{2} &\textbf{3}\\\textbf{0} &\textbf{1}&\textbf{0}\\ \textbf{1}&\textbf{1} &\textbf{0}\end{bmatrix}\begin{bmatrix}\normalsize-\textbf{1} &\textbf{1} &\textbf{0}\\\textbf{0} &\normalsize-\textbf{1} &\textbf{1}\\\textbf{2} &\textbf{3} &\textbf{4}\end{bmatrix}\neq\\\begin{bmatrix}\normalsize-\textbf{1} &\textbf{1} &\textbf{0}\\\textbf{0} &\normalsize-\textbf{1} &\textbf{1}\\\textbf{2} &\textbf{3} &\textbf{4}\end{bmatrix}\begin{bmatrix}\textbf{1} & \textbf{2} & \textbf{3} \\\textbf{0} &\textbf{1} &\textbf{0}\\ \textbf{1} &\textbf{1} &\textbf{0}\end{bmatrix}\\\textbf{Sol.\space(i)\space}\begin{bmatrix}5 & \normalsize-1\\6 &7\end{bmatrix}\begin{bmatrix}2 & \normalsize1\\3 &4\end{bmatrix}\\=\begin{bmatrix}10-3 & 5-4 \\12+21 & 6+28\end{bmatrix}$$

$$=\begin{bmatrix}7 &1 \\33 &34\end{bmatrix}\\\begin{bmatrix}2 &1 \\3 &4 \end{bmatrix}\begin{bmatrix}5 &\normalsize-1 \\6 &7\end{bmatrix}\\=\begin{bmatrix}10+6 &-2+7 \\15+24 &-3+28\end{bmatrix}\\=\begin{bmatrix}16 &5 \\39 &25\end{bmatrix}\\\text{Hence,}\begin{bmatrix}5 &\normalsize-1\\6 &7\end{bmatrix}\begin{bmatrix}2 &1 \\3 &4\end{bmatrix}\neq\\\begin{bmatrix}10-3 &5-4 \\12+21 & 6+28\end{bmatrix}\\=\begin{bmatrix}7 &1 \\33 &34\end{bmatrix}$$

$$\begin{bmatrix}2 &1 \\3 &4\end{bmatrix}\begin{bmatrix}5 &\normalsize-1 \\6 &7\end{bmatrix} =\\\begin{bmatrix} 10+6 &-2+7 \\15+24 &-3+28\end{bmatrix}\\=\begin{bmatrix}16 &5 \\39 &25\end{bmatrix}\\\text{Hence,\space}\begin{bmatrix}5 & \normalsize-1 \\6 &7\end{bmatrix}\begin{bmatrix}2 &1 \\3 &4\end{bmatrix}\neq\\\begin{bmatrix}2 &1 \\3 &4\end{bmatrix}\begin{bmatrix}5 &\normalsize-1 \\6&7\end{bmatrix}\\\text{(ii)\space}\text{Here,}\\\begin{bmatrix}1 &2 &3\\ 0 &1 &0\\1 &1 &0\end{bmatrix}\begin{bmatrix}\normalsize-1 &1 &0 \\0 &\normalsize-1 &1\\ 2 &3 &4\end{bmatrix}$$

$$=\begin{bmatrix}-1 +0+6 & 1-2+9 &0+2+12\\0+0+0 &0+(\normalsize-1)+0 &0+1+0\\-1+0+0 &1-1+0 &0+1+0\end{bmatrix}\\=\begin{bmatrix}5 &8 &14\\0 &\normalsize-1 &1\\\normalsize-1 &0 &1\end{bmatrix}\space\text{and}\\\begin{bmatrix}\normalsize-1 &1 &0\\0 &\normalsize-1 &1\\ 2 &3 &4\end{bmatrix}\begin{bmatrix}1 &2 &3\\0 &1 &0\\ 1 &1 &0\end{bmatrix}\\=\begin{bmatrix}-1+0+0 &-2+1+0 &-3+0+0 \\0+0+1 &0-1+1 &0+0+0\\2+0+4 &4+3+4 &6+0+0 \end{bmatrix}\\=\begin{bmatrix}\normalsize-1 &\normalsize-1 &\normalsize-3 \\1 &0 &0\\ 6 &11 &6\end{bmatrix}$$

Hence, the required result is verified.

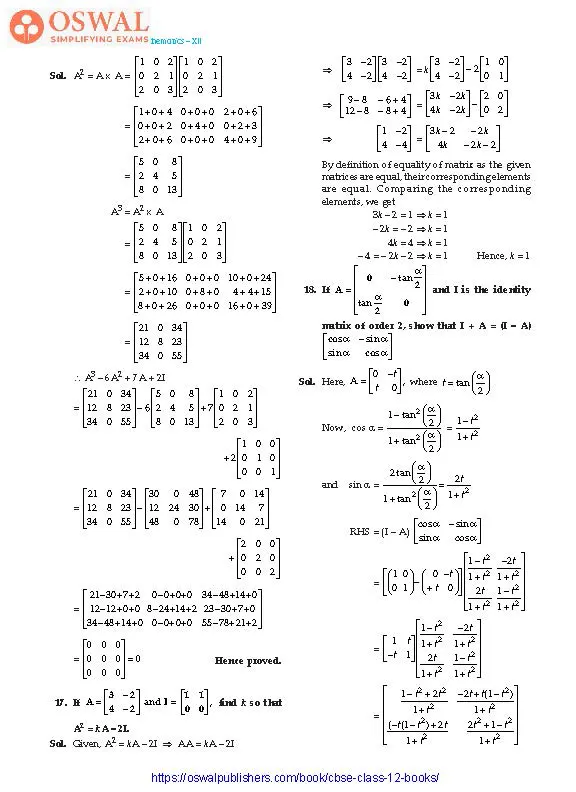
15. Find A² – 5A + 6I if

$$\textbf{A} = \begin{bmatrix}\textbf{2} &\textbf{0} &\textbf{1}\\\textbf{2} &\textbf{1} &\textbf{3}\\ \textbf{1} &\textbf{\normalsize-1} &\textbf{0}\end{bmatrix}$$

Sol. Here, A² = A × A

$$= \begin{bmatrix}2 &0 &1\\2 &1 &3\\ 1 &\normalsize-1 &0\end{bmatrix}\begin{bmatrix}2 &0 &1\\2 &1 &3\\ 1 &\normalsize-1 &0\end{bmatrix}\\=\begin{bmatrix}4+0+1 &0+0-1 &2+0+0\\4+2+3 &0+1-3 &2+3+0\\ 2-2+0 &0-1+0 &1-3+0\end{bmatrix}\\=\begin{bmatrix}5 &\normalsize-1 &2\\9 &\normalsize-2 &5\\ 0 &\normalsize-1 &\normalsize-2\end{bmatrix}$$

∴ A² – 5A + 6I

$$= \begin{bmatrix}5 &\normalsize-1 &2\\9 &\normalsize-2 &5\\ 0 &\normalsize-1 &\normalsize-2\end{bmatrix}-5\begin{bmatrix}2 &0 &1\\2 &1 &3\\ 1 &\normalsize-1 &0\end{bmatrix}\\+6\begin{bmatrix}1 &0&0 \\0 &1&0\\0&0 &1\end{bmatrix}\\=\begin{bmatrix}5 &\normalsize-1 &2 \\9 &\normalsize-2 &5\\0 &\normalsize-1 &\normalsize-2\end{bmatrix}-\begin{bmatrix}10 &0 &5\\10 &5 &15\\5 &\normalsize-5 &0\end{bmatrix}+\\\begin{bmatrix}6 &0 &0\\0 &6 &0\\0 &0 &6\end{bmatrix}\\=\begin{bmatrix}5-10+6 &-1+0+0 &2-5+0\\9-10+0 &-2+5+6 &5-15+0\\ 0-5+10 &-1+5+0 &-2-0+6\end{bmatrix}$$

$$=\begin{bmatrix}1 &\normalsize-1 &\normalsize-3 \\\normalsize-1 &\normalsize-1 &\normalsize-10\\ -5 &4 &4\end{bmatrix}$$

$$\textbf{16. If A =}\space\begin{bmatrix} \textbf{1} &\textbf{0} &\textbf{2}\\ \textbf{0} &\textbf{2} &\textbf{1}\\ \textbf{2} &\textbf{0} &\textbf{3}\end{bmatrix}\textbf{,}\space\\\textbf{prove that}\\\textbf{A}^{\textbf{3}} \textbf{–} \space\textbf{6A}^{\textbf{2}} \textbf{+} \textbf{7A + 2I = 0.}\\\textbf{Sol.}\space\text{A}^{2} = A×A=\\\begin{bmatrix}1 &0 &2\\0 &2 &1\\2 &0 &3\end{bmatrix}\begin{bmatrix}1 &0 &2\\0 &2 &1\\2 &0 &3\end{bmatrix}\\=\begin{bmatrix} 1+0+4 &0+0+0 &2+0+6\\0+0+2 &0+4+0 &0+2+3\\ 2+0+6 &0+0+0 &4+0+9\end{bmatrix}\\=\begin{bmatrix}5 &0 &8\\2 &4 &5\\ 8 &0 &13\end{bmatrix}$$

A³ = A² × A

$$= \begin{bmatrix}5 &0 &8\\2 &4 &5\\8 &0 &13\end{bmatrix}= \begin{bmatrix}1 &0 &2\\0 &2 &1\\2 &0 &3\end{bmatrix}\\=\begin{bmatrix}5+0+16 &0+0+0 &10+0+24\\2+0+10 &0+8+0 &4+4+15\\8+0+26 &0+0+0 &16+0+39\end{bmatrix}\\=\begin{bmatrix}21 &0 &34 \\12 &8 &23\\ 34 &0 &55\end{bmatrix}$$

∴ A³ – 6A² + 7A + 2I

$$=\begin{bmatrix}21 &0 &34\\12 &8 &23\\ 34 &0 &55\end{bmatrix}-6\begin{bmatrix}5 &0 &8\\2 &4 &5\\ 8 &0 &13\end{bmatrix}+\\7\begin{bmatrix}1 &0 &2\\0 &2 &1\\2 &0 &3\end{bmatrix}+2\begin{bmatrix}1 &0 &0\\0 &1 &0\\ 0 &0 &1\end{bmatrix}\\=\begin{bmatrix}21 &0 &34\\12 &8 &23\\ 34 &0 &55\end{bmatrix}-\begin{bmatrix}30 &0 &48\\12 &24 &30\\48 &0 &78\end{bmatrix}+\\\begin{bmatrix}7 &0 &14\\0 &14 &7\\ 14 &0 &21\end{bmatrix}+\begin{bmatrix}2 &0 &0\\0 &2 &0\\0 &0 &2\end{bmatrix}\\=\\\begin{bmatrix}21-30+7+2 &0+0+0+0 &34-48+14+0\\12-12+0+0 &8-24+14+2 &23-30+7+0\\ 34-48+14+0 &0-0+0+0 &55-78+21+2\end{bmatrix}$$

$$= \begin{bmatrix}0 &0 &0\\0 &0 &0\\0 &0 &0\end{bmatrix}=0\\\textbf{Hence proved.}$$

$$\textbf{17. If A =}\begin{bmatrix}\textbf{3} & \normalsize-\textbf{2} \\ \textbf{4} &\normalsize-\textbf{2}\end{bmatrix}\space\textbf{and}\\\textbf{I =}\begin{bmatrix}\textbf{1} &\textbf{1}\\\textbf{0} & \textbf{0}\end{bmatrix}\textbf{,}\space\textbf{find k so that}\\\textbf{A}^{\textbf{2}} \textbf{=}\textbf{kA - 2I}.$$

Sol. Given, A² = kA – 2I

$$\Rarr\space \text{AA} = \text{kA - 2I}$$

$$\Rarr\space \begin{bmatrix}3 &\normalsize-2 \\4 &\normalsize-2\end{bmatrix}\begin{bmatrix}3 &\normalsize-2 \\4 &\normalsize-2\end{bmatrix}\\=k\begin{bmatrix}3 &\normalsize-2 \\4 &\normalsize-2\end{bmatrix}-2\begin{bmatrix}1 &0 \\0 &1\end{bmatrix}\\\Rarr\space\begin{bmatrix}9-8 &-6+4 \\12-8 &-8+4\end{bmatrix}=\\\begin{bmatrix}3k &-2k \\4k &-2k\end{bmatrix}\begin{bmatrix}2 &0\\0 &2\end{bmatrix}\\\Rarr\space\begin{bmatrix}1 &\normalsize-2 \\4 &\normalsize-4\end{bmatrix} =\\\begin{bmatrix}3k-2 & -2k\\4k &-2k-2\end{bmatrix}$$

By definition of equality of matrix as the given matrices are equal, their corresponding elements are equal. Comparing the corresponding elements, we get

3k – 2 = 1 ⇒ k = 1

– 2k = – 2 ⇒ k = 1

4k = 4 ⇒ k = 1

– 4 = – 2k – 2 ⇒ k = 1

Hence, k = 1

$$\textbf{18.\space}\textbf{If A =}\begin{bmatrix}\textbf{0} & -\textbf{tan}\frac{\alpha}{\textbf{2}}\\\textbf{tan}\frac{\alpha}{\textbf{2}} &\textbf{0}\end{bmatrix}$$

and I is the identity matrix of order 2, show that I + A = (I – A)

$$\begin{bmatrix}\textbf{cos}\alpha &\textbf{-sin}\alpha\\\textbf{sin}\alpha &\textbf{cos}\alpha\end{bmatrix}\\\textbf{Sol.\space}\text{Here, A} = \begin{bmatrix}0 &-t \\t &0\end{bmatrix},\\\text{where t = tan}\bigg(\frac{\alpha}{2}\bigg)\\\text{Now, cos}\space\alpha \\=\frac{\text{1 - tan}^{2}\bigg(\frac{\alpha}{2}\bigg)}{\text{1 + tan}^{2}\bigg(\frac{\alpha}{2}\bigg)}\\=\frac{\text{1 - t}^{2}}{1 + t^{2}}$$

and

$$\text{sin}\space\alpha =\frac{\text{2 tan}\bigg(\frac{\alpha}{2}\bigg)}{\text{1 +tan}^{2}\bigg(\frac{\alpha}{2}\bigg)}\\=\frac{2t}{1 + t^{2}}\\\text{RHS = (I-A)}\begin{bmatrix}\text{cos}\space\alpha & -\text{sin}\space\alpha \\\text{sin}\space\alpha &\text{cos}\space\alpha\end{bmatrix}$$

$$ =\space \begin{bmatrix}\begin{pmatrix} 1 &0\\0 &1\end{pmatrix} - \begin{pmatrix}0 &-t \\+t &0\end{pmatrix}\end{bmatrix}\\\begin{bmatrix}\frac{1-t^{2}}{1+t^{2}} &\frac{-2t}{1+t^{2}}\\\frac{2t}{1+t^{2}} & \frac{\text{1 -t}^{2}}{1 + t^{2}}\end{bmatrix}\\= \begin{bmatrix}1 &t\\-t &1\end{bmatrix}\begin{bmatrix}\frac{\text{1 -t}^{2}}{\text{1+t}^{2}} &\frac{\normalsize-2t}{1 + t^{2}}\\\frac{2t}{\text{1 +t}^{2}}& \frac{\text{1-t}^{2}}{1 +t^{2}}\end{bmatrix}\\= \begin{bmatrix}\frac{1-t^{2}+2t^{2}}{\text{1+t}^{2}} &\frac{-2t + t(1 - t^{2})}{\text{1 + t}^{2}} \\\frac{(\normalsize-t(1 - t^{2}) +2t)}{\text{1 + t}^{2}} & \frac{2t^{2}+1-t^{2}}{\text{1 + t}^{2}}\end{bmatrix}\\= \begin{bmatrix} \frac{\text{1 + t}^{2}}{\text{1 +t}^{2}} & \frac{-2t+t-t^{3}}{\text{1 + t}^{2}} \\ \frac{-t+t^{3}+2t}{\text{1 + t}^{2}} & \frac{2t^{2} +1-t^{2}}{\text{1 +t}^{2}}\end{bmatrix}$$

$$= \begin{bmatrix}\frac{\text{1 + t}^{2}}{\text{1 +t}^{2}} &\frac{-t(1 + t^{2})}{\text{1 +t}^{2}} \\ \frac{t(1 +t^{2})}{\text{1 +t}^{2}} & \frac{\text{1+t}^{2}}{\text{1 +t}^{2}}\end{bmatrix}\\= \begin{bmatrix}1 &\normalsize-t \\t &1\end{bmatrix}\\\text{LHS = }\begin{bmatrix} 1 &0 \\0 &1\end{bmatrix} + \begin{bmatrix} 0 &\normalsize-t \\ t & 0\end{bmatrix}\\= \begin{bmatrix}0+1 & -t+0 \\t+0 & 0+1 \end{bmatrix}\\= \begin{bmatrix} 1 & \normalsize-t \\ t &1\end{bmatrix} =\text{RHS}$$

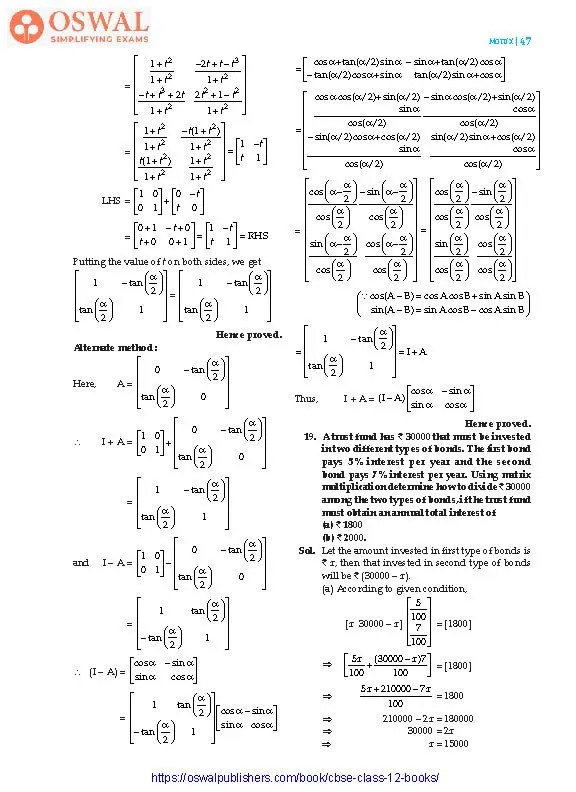
Putting the value of t on both sides, we get

$$\begin{bmatrix} 1 & -\text{tan}\bigg(\frac{\alpha}{2}\bigg)\\\text{tan}\bigg(\frac{\alpha}{2}\bigg) &1\end{bmatrix}\\= \begin{bmatrix} 1 &-\text{tan}\bigg(\frac{\alpha}{2}\bigg) \\\text{tan}\bigg(\frac{\alpha}{2}\bigg) & 1\end{bmatrix}\\\textbf{Hence proved.}$$

Alternate method :

$$\text{Here,\space}\text{A = } \begin{bmatrix}0 & -\text{tan}\bigg(\frac{\alpha}{2}\bigg)\\\text{tan}\bigg(\frac{\alpha}{2}\bigg) & 0\end{bmatrix}\\\therefore\space\text{I + A} =\begin{bmatrix} 1 & 0 \\0 &1\end{bmatrix} +\\\begin{bmatrix}0 & -\text{tan}\bigg(\frac{\alpha}{2}\bigg) \\\text{tan}\bigg(\frac{\alpha}{2}\bigg) &0\end{bmatrix}\\=\begin{bmatrix}1 & -\text{tan}\bigg(\frac{\alpha}{2}\bigg)\\\text{tan}\bigg(\frac{\alpha}{2}\bigg) &1\end{bmatrix}$$

$$\text{and}\space\text{I - A} = \begin{bmatrix}1 & 0\\0 &1\end{bmatrix}-\\\begin{bmatrix}0 & -\text{tan}\bigg(\frac{\alpha}{2}\bigg)\\\text{tan}\bigg(\frac{\alpha}{2}\bigg) & 0\end{bmatrix}\\= \begin{bmatrix}1 &\text{tan}\bigg(\frac{\alpha}{2}\bigg)\\-\text{tan}\bigg(\frac{\alpha}{2}\bigg) & 1\end{bmatrix}\\\therefore\space (\text{I-A}) =\begin{bmatrix}\text{cos}\space\alpha &-\text{sin}\space\alpha\\\text{sin}\space\alpha & \text{cos}\alpha\end{bmatrix}\\=\begin{bmatrix} 1 &\text{tan}\bigg(\frac{\alpha}{2}\bigg) \\-\text{tan}\bigg(\frac{\alpha}{2}\bigg) & 1\end{bmatrix}\\$$

$$\\\begin{bmatrix}\text{cos}\space\alpha &-\text{sin}\space\alpha\\\text{sin}\space\alpha &\text{cos}\space\alpha\end{bmatrix}$$

$$=\\\begin{bmatrix}\text{cos}\alpha + \text{tan}(\alpha/2)\text{sin}\alpha &-\text{sin}\space\alpha + \text{tan}(\alpha/2)\text{cos}\space\alpha\\-\text{tan}(\alpha/2)\text{cos}\alpha + sin\alpha & \text{tan}(\alpha/2)\text{sin}\alpha + \text{cos}\alpha\end{bmatrix}\\=$$

$$\begin{bmatrix}\frac{\text{cos}\space\alpha(\alpha/2) + \text{sin}(\alpha/2)\text{sin}\alpha}{\text{cos}(\alpha/2)} &\frac{-\text{sin}\alpha\text{cos}(\alpha/2) + \text{sin}(\alpha/2)\text{cos}\alpha}{\text{cos}(\alpha/2)}\\\frac{-\text{sin}(\alpha/2)\text{cos}\alpha + \text{cos}(\alpha/2)\text{sin}\alpha}{\text{cos}(\alpha/2)} &\frac{\text{sin}(\alpha/2)\text{sin}\alpha + \text{cos}(\alpha/2)\text{cos}\alpha}{\text{cos}(\alpha/2)}\end{bmatrix}$$

$$= \begin{bmatrix}\frac{\text{cos}\bigg(\alpha -\frac{\alpha}{2}\bigg)}{\text{cos}\bigg(\frac{\alpha}{2}\bigg)} &\frac{\normalsize-\text{sin}\bigg(\alpha -\frac{\alpha}{2}\bigg)}{\text{cos}\bigg(\frac{\alpha}{2}\bigg)}\\\frac{\text{sin}\bigg(\alpha -\frac{\alpha}{2}\bigg)}{\text{cos}\bigg(\frac{\alpha}{2}\bigg)} &\frac{\text{cos}\bigg(\alpha -\frac{\alpha}{2}\bigg)}{\text{cos}\bigg(\frac{\alpha}{2}\bigg)}\end{bmatrix}$$

$$=\begin{bmatrix}\frac{\text{cos}\bigg(\frac{\alpha}{2}\bigg)}{\text{cos}\bigg(\frac{\alpha}{2}\bigg)} & \frac{-\text{sin}\bigg(\frac{\alpha}{2}\bigg)}{\text{cos}\bigg(\frac{\alpha}{2}\bigg)}\\\frac{\text{sin}\bigg(\frac{\alpha}{2}\bigg)}{\text{cos}\bigg(\frac{\alpha}{2}\bigg)} &\frac{\text{cos}\bigg(\frac{\alpha}{2}\bigg)}{\text{cos}\bigg(\frac{\alpha}{2}\bigg)}\end{bmatrix}$$

$$\begin{pmatrix}\because \text{cos}(\text{A - B}) = \text{cos A cos B + sin A sin B}\\\text{sin}(\text{A-B}) =\text{sin A cos B - cos A sin B}\end{pmatrix}\\=\begin{bmatrix}1 & -\text{tan}\bigg(\frac{\alpha}{2}\bigg)\\\text{tan}\bigg(\frac{\alpha}{2}\bigg) &1\end{bmatrix}$$

= I + A

Thus, I+A =(I - A)

$$\begin{bmatrix}\text{cos}\space\alpha &-\text{sin}\space\alpha\\\text{sin}\space\alpha &\text{cos}\space\alpha\end{bmatrix}$$

Hence proved.

19. A trust fund has ₹ 30000 that must be invested in two different types of bonds. The first bond pays 5% interest per year and the second bond pays 7% interest per year. Using matrix multiplication determine how to divide ₹ 30000 among the two types of bonds, if the trust fund must obtain an annual total interest of

(a) ₹ 1800

(b) ₹ 2000.

Sol. Let the amount invested in first type of bonds is ₹ x, then that invested in second type of bonds will be ₹ (30000 – x).

(a) According to given condition,

$$\begin{bmatrix}x &30000-x\end{bmatrix}\begin{bmatrix}\frac{5}{100} \\\frac{7}{100}\end{bmatrix}\\=[1800]\\\Rarr\space\begin{bmatrix}\frac{5x}{100} + \frac{(30000-x)7}{100}\end{bmatrix}\\=[1800]\\\Rarr\space \frac{5x+210000 -7x}{100}\\=1800\\\Rarr\space\text{210000 – 2x = 180000}\\\Rarr\space\text{30000 = 2x}\\\Rarr\space\text{x = 15000}$$

Hence, the amounts invested in the two types of bonds are respectively ₹ 15000 and ₹ (30000 – 15000) = ₹ 15000.

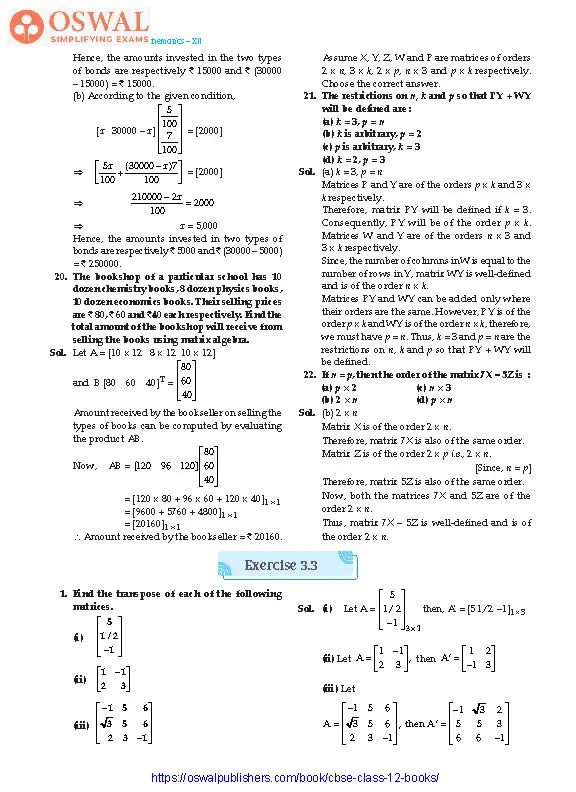
(b) According to the given condition,

$$\begin{bmatrix}x & 30000-x\end{bmatrix}\begin{bmatrix}\frac{5}{100}\\\frac{7}{100}\end{bmatrix}\\=[2000]\\\Rarr\space \begin{bmatrix}\frac{5x}{100} + \frac{(30000 - x)7}{100}\end{bmatrix}\\=[2000]\\\Rarr\space \frac{210000-2x}{100} = 2000\\\Rarr\space\text{x = 5,000}$$

Hence, the amounts invested in two types of bonds are respectively ₹ 5000 and ₹ (30000 – 5000)

= ₹ 250000.

20. The bookshop of a particular school has 10 dozen chemistry books, 8 dozen physics books, 10 dozen economics books. Their selling prices are ₹ 80, ₹ 60 and ₹40 each respectively. Find the total amount of the bookshop will receive from selling the books using matrix algebra.

Sol. Let A = [10 × 12 8 × 12 10 × 12]

$$\text{and}\space\begin{bmatrix} 80 & 60 & 40\end{bmatrix}^{\text{T}} = \begin{bmatrix}80\\60\\40\end{bmatrix}$$

Amount received by the bookseller on selling the types of books can be computed by evaluating the product AB.

$$\text{Now,\space AB = }\begin{bmatrix} 120 &96 &120\end{bmatrix}\begin{bmatrix}80\\60\\40\end{bmatrix}$$

= [120 × 80 + 96 × 60 + 120 × 40]_{1 × 1}

= [9600 + 5760 + 4800]_{1 × 1}

= [20160]_{1 × 1}

∴ Amount received by the bookseller = ₹ 20160.

Assume X, Y, Z, W and P are matrices of orders 2 × n, 3 × k, 2 × p, n × 3 and p × k respectively.

Choose the correct answer.

21. The restrictions on n, k and p so that PY + WY will be defined are :

(a) k = 3, p = n

(b) k is arbitrary, p = 2

(c) p is arbitrary, k = 3

(d) k = 2, p = 3

Sol. (a) k = 3, p = n

Matrices P and Y are of the orders p × k and 3 × k respectively.

Therefore, matrix PY will be defined if k = 3. Consequently, PY will be of the order p × k. Matrices W and Y are of the orders n × 3 and 3 × k respectively.

Since, the number of columns inW is equal to the number of rows in Y, matrix WY is well-defined and is of the order n × k.

Matrices PY and WY can be added only where their orders are the same. However, PY is of the order p × k and WY is of the order n × k, therefore, we must have p = n. Thus, k = 3 and p = n are the restrictions on n, k and p so that PY + WY will be defined.

22. If n = p, then the order of the matrix 7X – 5Z is :

(a) p × 2

(b) 2 × n

(c) n × 3

(d) p × n

Sol. (b) 2 × n

Matrix X is of the order 2 × n.

Therefore, matrix 7X is also of the same order. Matrix Z is of the order 2 × p i.e., 2 × n.

[Since, n = p]

Therefore, matrix 5Z is also of the same order.

Now, both the matrices 7X and 5Z are of the order 2 × n.

Thus, matrix 7X – 5Z is well-defined and is of the order 2 × n.