NCERT Solutions for Class 12 Maths Chapter 3 Matrix - Exercise 3.4
Access Exercises of Class 12 Maths Chapter 3 – Matrix
Exercise 3.1 Solutions: 10 Questions (7 Short Answers, 3 MCQs)
Exercise 3.2 Solutions: 22 Questions (14 Long, 6 Short, 2 MCQs)
Exercise 3.3 Solutions: 12 Questions (10 Short Answers, 2 MCQs)
Exercise 3.4 Solutions: 18 Questions (4 Long, 13 Short, 1 MCQ)
Miscellaneous Exercise Solutions: 15 Questions (7 Long, 5 Short, 3 MCQs)
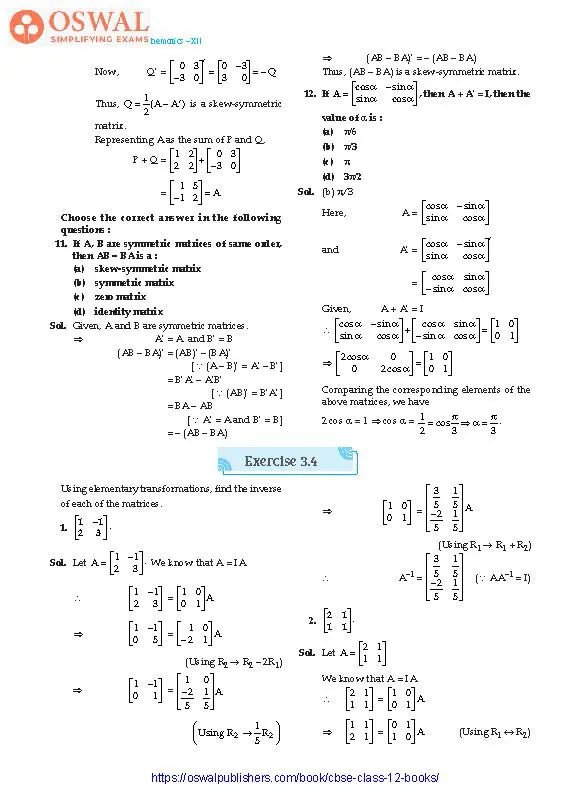
Exercise 3.4
Using elementary transformations, find the inverse of each of the matrices.
$$\textbf{1.}\space\begin{bmatrix}\textbf{1} &\normalsize-\textbf{1}\\\textbf{2} &\textbf{3}\end{bmatrix}\textbf{.}\\\textbf{Sol. \space}\text{Let A} = \begin{bmatrix}1 &\normalsize-1 \\2 &3\end{bmatrix}.$$
We know that A = IA
$$\therefore\space\begin{bmatrix}1 &\normalsize-1\\2 &3\end{bmatrix} = \begin{bmatrix}1 &0 \\0 &1\end{bmatrix}\text{A}\\\Rarr\space \begin{bmatrix}1 &\normalsize-1\\0 &5\end{bmatrix} = \begin{bmatrix}1 & 0\\ \normalsize-2 &1\end{bmatrix}\text{A}$$
(Using R2 → R2 – 2R1)
$$\Rarr\space\begin{bmatrix}1 &\normalsize-1\\0 &5\end{bmatrix} = \begin{bmatrix}1 &0\\\normalsize-2 &1\end{bmatrix}\text{A}\\(\text{Using\space R}_{2}\xrightarrow{}\text{R}_{2} - 2\text{R}_{1})\\\Rarr\space \begin{bmatrix}1 &\normalsize-1\\0 &1\end{bmatrix} = \begin{bmatrix}1 &0\\\frac{\normalsize-2}{5} &\frac{1}{5}\end{bmatrix}\text{A}\\\bigg(\text{Using}\space\text{R}_{2}\xrightarrow{}\frac{1}{5}\text{R}_{2}\bigg)\\\Rarr\space \begin{bmatrix} 1 &0 \\0 &1\end{bmatrix} = \begin{bmatrix}\frac{3}{5} &\frac{1}{5}\\\frac{\normalsize-2}{5} &\frac{1}{5}\end{bmatrix}\text{A}\\(\text{using\space R}_{1}\xrightarrow{}\text{R}_{1} + \text{R}_{2})\\\therefore\space\text{A}^{\normalsize-1} = \begin{bmatrix}\frac{3}{5} &\frac{1}{5}\\\frac{\normalsize-2}{5} &\frac{1}{5}\end{bmatrix}$$
(∵ AA–1 = I)
$$\textbf{2.\space}\begin{bmatrix}\textbf{2} &\textbf{1}\\\textbf{1} &\textbf{1}\end{bmatrix}\textbf{.}\\\textbf{Sol.\space}\text{Let A = } \begin{bmatrix}2 &1\\1 &1\end{bmatrix}$$
We know that A = IA
$$\therefore\space\begin{bmatrix}2 &1\\1 &1\end{bmatrix} = \begin{bmatrix}1 &0\\0 &1\end{bmatrix}\text{A}\\\Rarr\space\begin{bmatrix}1 &1\\2 &1\end{bmatrix} = \begin{bmatrix}0 &1\\1 &0\end{bmatrix}\text{A}\\\text{(\text{Using}\space \text{R}}_{1} \leftrightarrow \text{R}_{2})$$
$$\Rarr\space \begin{bmatrix}1 &1\\0 &\normalsize-1\end{bmatrix} = \begin{bmatrix}0 &1\\1 &\normalsize-2\end{bmatrix}\text{A}\\\text{(Using\space R}_{2}\xrightarrow{}\text{R}_{2} - \text{2R}_{1})\\\Rarr\space \begin{bmatrix}1 &1\\0 &1\end{bmatrix} = \begin{bmatrix}0 &1\\\normalsize-1 &2\end{bmatrix}\text{A}\\(\text{Using R}_{2}\xrightarrow{} (\normalsize-1)\text{R}_{2})\\\Rarr\space\begin{bmatrix}1 &0\\0 &1\end{bmatrix} =\begin{bmatrix}1 &\normalsize-1\\\normalsize-1 &2\end{bmatrix}\text{A}\\\text{(Using R}_{1}\xrightarrow{}\text{R}_{1} -\text{R}_{2})\\\therefore\space \text{A}^{\normalsize-1} = \begin{bmatrix}1 &\normalsize-1\\\normalsize-1 & 2\end{bmatrix}$$
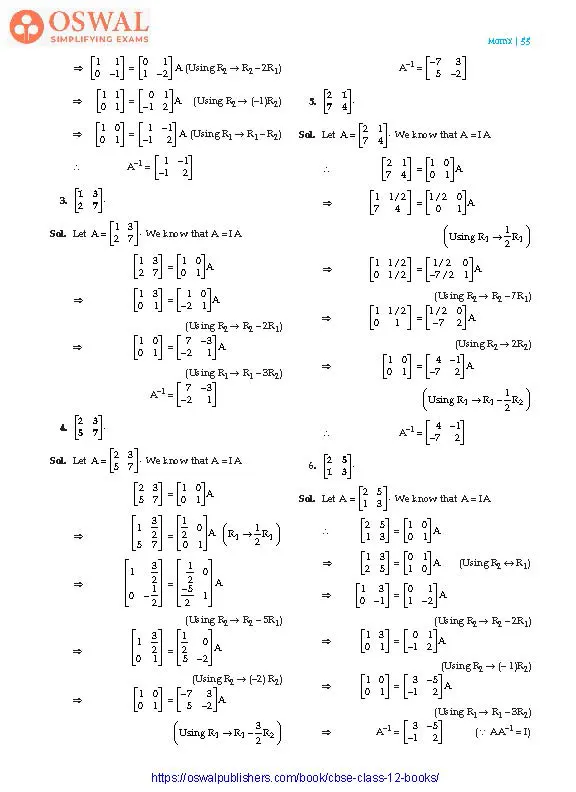
$$\textbf{3.}\space \begin{bmatrix}\textbf{1} &\textbf{3} \\\textbf{2} &\textbf{7}\end{bmatrix}.\\\textbf{Sol.\space}\text{Let A = }\begin{bmatrix}1 &3\\2 &7\end{bmatrix}.$$
We know that A = IA
$$\begin{bmatrix}1 &3\\2 &7\end{bmatrix} = \begin{bmatrix}1 &0\\0 &1\end{bmatrix}\text{A}\\\Rarr\space\begin{bmatrix}1 &3\\0 &1\end{bmatrix} = \begin{bmatrix}1 &0\\\normalsize-2 &1\end{bmatrix}\text{A}\\\text{(Using R}_{2}\xrightarrow{}\text{R}_{2} - 2\text{R}_{1})\\\Rarr\space \begin{bmatrix}1 &0\\0 &1\end{bmatrix} = \begin{bmatrix}7 &\normalsize-3\\\normalsize-2 &1\end{bmatrix}\text{A}\\\text{(Using R}_{1}\xrightarrow {} \text{R}_{1} - 3\text{R}_{2})\\\text{A}^{\normalsize-1} = \begin{bmatrix}7 &\normalsize-3\\\normalsize-2 &1\end{bmatrix}$$
$$\textbf{4.\space}\begin{bmatrix}\textbf{2} &\textbf{3}\\\textbf{5} & \textbf{7}\end{bmatrix}\textbf{.}\\\textbf{Sol.\space}\text{Let A = }\begin{bmatrix}2 &3\\5 &7\end{bmatrix}.$$
We know that A = IA
$$\begin{bmatrix}2 &3\\5 &7\end{bmatrix} = \begin{bmatrix}1 &0\\0 &1\end{bmatrix}\text{A}\\\Rarr\space \begin{bmatrix}1 &\frac{3}{2}\\5 &7\end{bmatrix} = \begin{bmatrix}\frac{1}{2} &0 \\0 &1\end{bmatrix}\text{A}\\\bigg(\text{R}_{1}\xrightarrow{}\frac{1}{2}\text{R}_{1}\bigg)\\\Rarr\space \begin{bmatrix}1 &\frac{3}{2}\\0 &-\frac{1}{2}\end{bmatrix} = \begin{bmatrix}\frac{1}{2} &0\\\frac{\normalsize-5}{2} &1\end{bmatrix}\text{A}\\\text{(Using R}_{2}\xrightarrow{}\text{R}_{2} - 5\text{R}_{1})\\\Rarr\space \begin{bmatrix}1 &\frac{3}{2}\\0 &1\end{bmatrix} = \begin{bmatrix}\frac{1}{2}&0\\5 &\normalsize-2\end{bmatrix}\text{A}\\\text{(Using R}_{2}\xrightarrow{}(\normalsize-2)\text{R}_{2})\\\Rarr\space \begin{bmatrix}1 &0 \\0 &1\end{bmatrix} = \begin{bmatrix}\normalsize-7 &3 \\5 &\normalsize-2\end{bmatrix}\text{A}$$
$$\bigg(\text{Using R}_{1}\xrightarrow{}\text{R}_{1} -\frac{3}{2}\text{R}_{2}\bigg)\\\text{A}^{\normalsize-1} = \begin{bmatrix}\normalsize-7 &3 \\5 &\normalsize-2\end{bmatrix}$$
$$\textbf{5.\space}\begin{bmatrix}\textbf{2} &\textbf{1} \\\textbf{7} &\textbf{4}\end{bmatrix}\textbf{.}\\\textbf{Sol.\space}\text{Let A = } \begin{bmatrix}2 &1\\7 &4\end{bmatrix}.$$
We know that A = IA
$$\therefore\space \begin{bmatrix}2 &1 \\7 &4\end{bmatrix} = \begin{bmatrix}1 &0 \\0 &1\end{bmatrix}\text{A}\\\Rarr\space \begin{bmatrix}1 &\frac{1}{2}\\7 &4\end{bmatrix} = \begin{bmatrix}\frac{1}{2} &0\\0&1\end{bmatrix}\text{A}\\\bigg(\text{Using}\space\text{R}_{1}\xrightarrow{}\frac{1}{2}\text{R}_{1}\bigg)\\\Rarr\space \begin{bmatrix}1 &\frac{1}{2}\\0 &\frac{1}{2}\end{bmatrix} = \begin{bmatrix}\frac{1}{2} &0 \\-\frac{7}{2} &1\end{bmatrix}\\\text{(Using}\space\text{R}_{2}\xrightarrow{}\text{R}_{2} - 7\text{R}_{1})\\\Rarr\space \begin{bmatrix}1 &\frac{1}{2}\\0 &1\end{bmatrix} = \begin{bmatrix}\frac{1}{2} &0\\\normalsize-7 &2\end{bmatrix}\text{A}\\\text{(Using}\space \text{R}_{2}\xrightarrow{}2\text{R}_{2})\\\Rarr\space \begin{bmatrix}1 &0\\0 &1\end{bmatrix} = \begin{bmatrix}4 &\normalsize-1 \\\normalsize-7 &2\end{bmatrix}\text{A}$$
$$\bigg(\text{Using R}_{1}\xrightarrow{}\text{R}_{1}-\frac{1}{2}\text{R}_{2}\bigg)\\\therefore\space \text{A}^{\normalsize-1} = \begin{bmatrix}4 &\normalsize-1\\\normalsize-7 &2\end{bmatrix}$$
$$\textbf{6.\space}\begin{bmatrix}\textbf{2} &\textbf{5} \\\textbf{1} &\textbf{3}\end{bmatrix}\textbf{.}\\\textbf{Sol.\space}\text{Let A = }\begin{bmatrix}2 &5 \\1 & 3\end{bmatrix}.$$
We know that A = IA
$$\therefore\space \begin{bmatrix}2 &5\\1 &3\end{bmatrix} = \begin{bmatrix}1 &0\\0 &1\end{bmatrix}\text{A}\\\Rarr\space\begin{bmatrix}1 &3\\2 &5\end{bmatrix} = \begin{bmatrix}0 &1 \\1 &0\end{bmatrix}\text{A}\\\text{(Using R}_{2}\leftrightarrow\text{R}_{1})\\\Rarr\space \begin{bmatrix}1 &3 \\2 &5\end{bmatrix} = \begin{bmatrix}0 &1 \\1 &\normalsize-2\end{bmatrix}\text{A}\\(\text{Using R}_{2}\xrightarrow{} \text{R}_{2}- 2\text{R}_1)\\\Rarr\space \begin{bmatrix}1 &3 \\0 &1\end{bmatrix} = \begin{bmatrix}0 &1 \\\normalsize-1 &2\end{bmatrix}\text{A}\\\text{(Using}\space\text{R}_{2}\xrightarrow{}(\normalsize-1)\text{R}_{2})\\\Rarr\space \begin{bmatrix}1 &0 \\0 &1\end{bmatrix} = \begin{bmatrix}3 &\normalsize-5\\\normalsize-1 & 2\end{bmatrix}\text{A}\\\text{(Using R}_{1}\xrightarrow{}\text{R}_{1} - 3\text{R}_{2})$$
$$\Rarr\space\text{A}^{\normalsize-1} = \begin{bmatrix}3 &\normalsize-5 \\\normalsize-1 &2\end{bmatrix}$$
(∵ AA–1 = I)
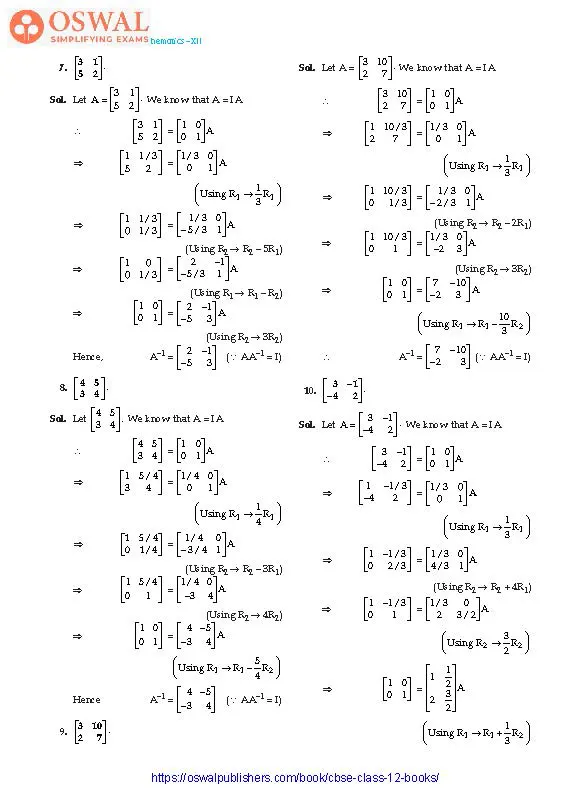
$$\textbf{8.}\begin{bmatrix}\textbf{4} &\textbf{5} \\\textbf{3} &\textbf{4}\end{bmatrix}\textbf{.}\\\textbf{Sol.\space}\text{Let}\space \begin{bmatrix}4 &5 \\3 &4\end{bmatrix}.$$
We know that A = IA
$$\therefore\space\begin{bmatrix}4 &5 \\3 &4\end{bmatrix} = \begin{bmatrix}1 &0 \\0 &1\end{bmatrix}\text{A}\\\Rarr\space \begin{bmatrix}1 &\frac{5}{4}\\3 &4\end{bmatrix} = \begin{bmatrix}\frac{1}{4} &0\\0 & 1\end{bmatrix}\text{A}\\\bigg(\text{Using}\space\text{R}_{1}\xrightarrow{}\frac{1}{4}\text{R}_{1}\bigg)\\\Rarr\space\begin{bmatrix}1 &\frac{5}{4}\\0 &\frac{1}{4}\end{bmatrix} = \begin{bmatrix}\frac{1}{4} & 0 \\-\frac{3}{4} &1\end{bmatrix}\text{A}\\(\text{Using R}_{2}\xrightarrow{}4\text{R}_{2})\\\Rarr\space \begin{bmatrix}1 &0\\0 &1\end{bmatrix} = \begin{bmatrix}4 &\normalsize-5\\\normalsize-3 &4\end{bmatrix}\text{A}\\\bigg(\text{Using\space R}_{1}\xrightarrow{} \text{R}_{1} -\frac{5}{4}\text{R}_{2}\bigg)\\\text{Hence}\space \text{A}^{\normalsize-1} = \begin{bmatrix}4 &\normalsize-5\\\normalsize-3 &4\end{bmatrix}$$
(∵ AA–1 = I)
$$\textbf{9.\space}\begin{bmatrix}\textbf{3} &\textbf{10}\\\textbf{2} &\textbf{7}\end{bmatrix}\textbf{.}\\\textbf{Sol.\space}\text{Let A = } \begin{bmatrix}3 &10\\2 &7\end{bmatrix}.$$
We know that A = IA
$$\therefore\space\begin{bmatrix}3 &10\\2 &7\end{bmatrix} = \begin{bmatrix}1 &0\\0 &1\end{bmatrix}\text{A}\\\Rarr\space \begin{bmatrix}1 &\frac{10}{3}\\2 &7\end{bmatrix} = \begin{bmatrix}\frac{1}{3} &0\\0 & 1\end{bmatrix}\text{A}\\\bigg(\text{Using R}_{1}\xrightarrow{}\frac{1}{3}\text{R}_{1}\bigg)\\\Rarr\space \begin{bmatrix}1 &\frac{10}{3}\\0 &\frac{1}{3}\end{bmatrix} = \begin{bmatrix}\frac{1}{3} &0\\\frac{-2}{3} &1\end{bmatrix}\text{A}\\\text{(Using R}_{2}\xrightarrow{}\text{R}_{2} - 2\text{R}_{1})\\\Rarr\space\begin{bmatrix}1 &\frac{10}{3}\\0 &1\end{bmatrix} = \begin{bmatrix}\frac{1}{3} &0\\\normalsize-2 &3\end{bmatrix}\text{A}\\\text{(Using} \space \text{R}_{2}\xrightarrow{} 3\text{R}_{2})\\\Rarr\space\begin{bmatrix}1 &0\\0 &1\end{bmatrix} = \begin{bmatrix}7 &-10\\-2 &3\end{bmatrix}\text{A}$$
$$\bigg(\text{Using}\space \text{R}_{1}\xrightarrow{}\text{R}_{1}-\frac{10}{3}\text{R}_{2}\bigg)\\\therefore\space\text{A}^{\normalsize-1} = \begin{bmatrix}7 &-10\\\normalsize-2 &3\end{bmatrix}$$
(∵ AA-1=I)
$$\textbf{10.\space }\begin{bmatrix}\textbf{3} &\textbf{\normalsize-1}\\\textbf{\normalsize-4} &\textbf{2}\end{bmatrix}\textbf{.}\\\textbf{Sol.\space}\text{Let A = }\begin{bmatrix}3 &\normalsize-1\\\normalsize-4 &2\end{bmatrix}$$
We know that A = IA
$$\therefore\space \begin{bmatrix}3 &\normalsize-1 \\\normalsize-4 &2\end{bmatrix} = \begin{bmatrix}1 &0 \\0 &1\end{bmatrix}\text{A}\\\Rarr\space\begin{bmatrix}1 &\frac{\normalsize-1}{3}\\-4 &2\end{bmatrix} =\begin{bmatrix}\frac{1}{3} &0 \\0 &1\end{bmatrix}\text{A}\\\bigg(\text{Using R}_{1}\xrightarrow{}\frac{1}{3}\text{R}_{1}\bigg)\\\Rarr\space \begin{bmatrix}1 &\frac{\normalsize-1}{3}\\0 &\frac{2}{3}\end{bmatrix} = \begin{bmatrix}\frac{1}{3} &0\\\frac{4}{3} &1\end{bmatrix}\text{A}\\\text{(Using}\space\text{R}_{2}\xrightarrow{}\text{R}_{2} + 4R_{1})\\\Rarr\space \begin{bmatrix}1 &\frac{\normalsize-1}{3}\\0 &1\end{bmatrix} = \begin{bmatrix}\frac{1}{3} &0\\2 &\frac{3}{2}\end{bmatrix}\text{A}\\\bigg(\text{Using R}_{2}\xrightarrow{}\frac{3}{2}\text{R}_{2}\bigg)$$
$$\Rarr\space\begin{bmatrix}1 &0\\0 &1\end{bmatrix} = \begin{bmatrix}1 &\frac{1}{2}\\2 &\frac{3}{2}\end{bmatrix}\text{A}\\\bigg(\text{Using R}_{1}\xrightarrow{}\text{R}_{1} + \frac{1}{3}\text{R}_{2}\bigg)\\\therefore\text{A}^{\normalsize-1}\begin{bmatrix}1 &\frac{1}{2}\\2 &\frac{3}{2}\end{bmatrix}$$
(∵ AA–1 = I)
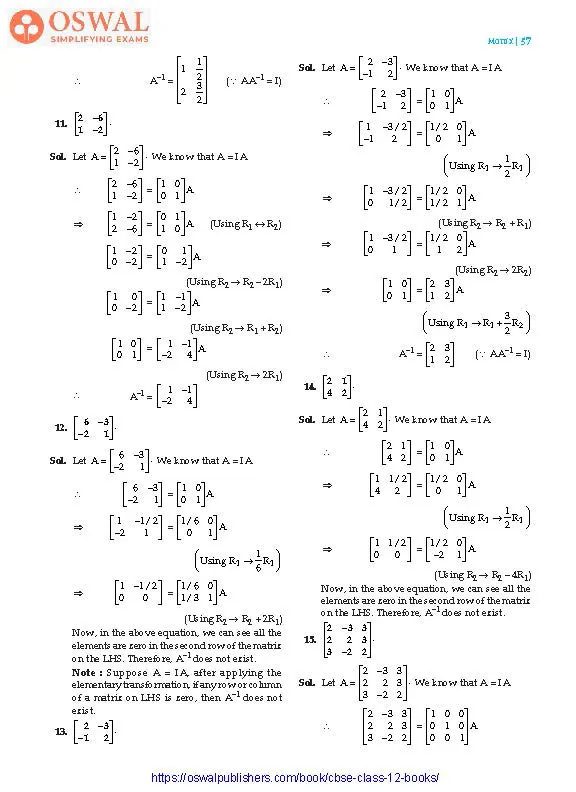
$$\textbf{11.\space}\begin{bmatrix}\textbf{2} &\textbf{\normalsize-6}\\\textbf{1} &\textbf{\normalsize-2}\end{bmatrix}\textbf{.}\\\textbf{Sol.\space}\text{Let A = } \begin{bmatrix}2 &\normalsize-6\\1 & -2\end{bmatrix}.$$
We know that A = IA
$$\therefore\space\begin{bmatrix}2 & \normalsize-6\\1 &\normalsize-2\end{bmatrix} =\begin{bmatrix} 1 &0\\0 &1\end{bmatrix}\text{A}\\\Rarr\space \begin{bmatrix}1 &\normalsize-2\\2 &\normalsize-6\end{bmatrix} = \begin{bmatrix}0 & 1\\1 &0\end{bmatrix}\text{A}\\(\text{Using R}_{1}\leftrightarrow\text{R}_{2})\\\begin{bmatrix}1 &\normalsize-2\\0 & \normalsize-2\end{bmatrix} = \begin{bmatrix}0 &1\\ 1 &\normalsize-2\end{bmatrix}\text{A}\\\text{(Using R}_{2}\xrightarrow{}\text{R}_{2} - 2\text{R}_{1})\\\begin{bmatrix}1 &0\\0 &\normalsize-2\end{bmatrix} = \begin{bmatrix}1 &\normalsize-1\\1 &\normalsize-2\end{bmatrix}\text{A}\\\text{(Using}\space \text{R}_{2}\xrightarrow{}\text{R}_{1} + \text{R}_{2})\\\begin{bmatrix}1 &0\\0 &\normalsize1\end{bmatrix} = \begin{bmatrix}1 & \normalsize-1\\\normalsize-2 & 4\end{bmatrix}\text{A}\\\text{(Using}\space \text{R}_{2}\xrightarrow{}2\text{R}_{1})$$
$$\therefore\space \text{A}^{\normalsize-1} = \begin{bmatrix}1 &\normalsize-1\\\normalsize-2 &4\end{bmatrix}$$
$$\textbf{12.\space}\begin{bmatrix}\textbf{6} & \textbf{\normalsize-3} \\\textbf{\normalsize-2} &\textbf{1}\end{bmatrix}\textbf{.}\\\textbf{Sol.\space}\text{Let A = }\begin{bmatrix}6 & \normalsize-3\\\normalsize-2 &1\end{bmatrix}$$.
We know that A = IA
$$\therefore\space \begin{bmatrix}6 &\normalsize-3\\\normalsize-2 &1\end{bmatrix} = \begin{bmatrix}1 &0\\0 &1\end{bmatrix}\text{A}\\\Rarr\space \begin{bmatrix} 1 &\frac{-1}{2}\\\normalsize-2 &1\end{bmatrix} = \begin{bmatrix} \frac{1}{6} &0\\0&1\end{bmatrix}\text{A}\\\bigg(\text{Using R}_{1}\xrightarrow{}\frac{1}{6}\text{R}_{1}\bigg)\\\Rarr\space\begin{bmatrix}1 &\frac{\normalsize-1}{2}\\0 & 0\end{bmatrix} = \begin{bmatrix}\frac{1}{6} &0\\\frac{1}{3} &1\end{bmatrix}\text{A}\\\text{Using R}_{2}\xrightarrow{}\text{R}_{2} + 2\text{R}_{1}$$
Now, in the above equation, we can see all the elements are zero in the second row of the matrix on the LHS. Therefore, A–1 does not exist.
Note : Suppose A = IA, after applying the elementary transformation, if any row or column of a matrix on LHS is zero, then A–1 does not exist.
$$\textbf{13.\space}\begin{bmatrix}\textbf{2} &\textbf{\normalsize-3}\\\textbf{\normalsize-1} & \textbf{2}\end{bmatrix}\\\textbf{Sol.\space}\text{Let \space A = }\begin{bmatrix}2 &\normalsize-3\\\normalsize-1 & 2\end{bmatrix}.$$
We know that A = IA
$$\therefore\space\begin{bmatrix}2 & \normalsize-3\\\normalsize-1 &2\end{bmatrix} = \begin{bmatrix}1 & 0\\0 &1\end{bmatrix}\text{A}\\\Rarr\space\begin{bmatrix}1 &-\frac{3}{2}\\\normalsize-1 &2\end{bmatrix} = \begin{bmatrix}\frac{1}{2} &0\\0 &1\end{bmatrix}\text{A}\\\bigg(\text{Using R}_{1}\xrightarrow{}\frac{1}{2}\text{R}_{1}\bigg)\\\Rarr\space \begin{bmatrix}1 & \frac{\normalsize-3}{2}\\\normalsize-1 & 2\end{bmatrix} = \begin{bmatrix}\frac{1}{2} &0\\\frac{1}{2} &1\end{bmatrix}\text{A}\\\text{(Using}\space\text{R}_{2}\xrightarrow{}\text{R}_{2}+ \text{R}_{1})\\\Rarr\space \begin{bmatrix}1 &\frac{-3}{2}\\0 & 1\end{bmatrix} = \begin{bmatrix}\frac{1}{2} &0\\1 &2\end{bmatrix}\text{A}\\(\text{Using R}_{2}\xrightarrow{}2 \text{R}_{2})\\\Rarr\space \begin{bmatrix}1 &0 \\0 &1\end{bmatrix} = \begin{bmatrix} 2 &3 \\1 & 2\end{bmatrix}\text{A}$$
$$\bigg(\text{Using R}_{1}\xrightarrow{}\text{R}_{1} + \frac{3}{2}\text{R}_{2}\bigg)\\\therefore\space\text{A}^{\normalsize-1} = \begin{bmatrix}2 &3\\1 &2\end{bmatrix}$$
(∵ AA–1 = I)
$$\textbf{14.\space}\begin{bmatrix}\textbf{2} &\textbf{1} \\\textbf{4} &\textbf{2}\end{bmatrix}\textbf{.}\\\textbf{Sol.\space}\text{Let A = }\begin{bmatrix}2 & 1 \\4 &2\end{bmatrix}.$$
We know that A = IA
$$\therefore\space \begin{bmatrix}2 & 1 \\ 4 & 2\end{bmatrix} = \begin{bmatrix}1 &0 \\0 &1\end{bmatrix}\text{A}\\\Rarr\space\begin{bmatrix}1 &\frac{1}{2}\\4 &2\end{bmatrix} = \begin{bmatrix}\frac{1}{2} &0\\0 &1\end{bmatrix} \text{A}\\\bigg(\text{Using R}_{1}\xrightarrow{}\frac{1}{2}\text{R}_{1}\bigg)\\\Rarr\space \begin{bmatrix}1 &\frac{1}{2}\\0 &0\end{bmatrix} = \begin{bmatrix}\frac{1}{2} &0\\\normalsize-2 &1\end{bmatrix}\text{A}\\\text{(Using R}_{2}\xrightarrow{} \text{R}_{2} - 4\text{R}_{1})$$
Now, in the above equation, we can see all the elements are zero in the second row of the matrix on the LHS. Therefore, A–1 does not exist.
$$\textbf{15.\space}\begin{bmatrix}\textbf{2} &\textbf{\normalsize-3} &\textbf{3}\\\textbf{2} &\textbf{2} &\textbf{3}\\\textbf{3}&\textbf{\normalsize-2} &\textbf{2}\end{bmatrix}\textbf{.}\\\textbf{Sol.\space}\text{Let A = }\begin{bmatrix}2 &\normalsize-3 &3\\2 &2 &3\\ 3 &\normalsize-2 &2\end{bmatrix}.$$
We know that A = IA
$$\therefore\space\begin{bmatrix}2 &\normalsize-3 &3\\2 &2 &3\\3 &\normalsize-2 &2\end{bmatrix} = \begin{bmatrix} 1 &0 &0\\0 &1 &0\\0 &0 &1\end{bmatrix}\text{A}\\\Rarr\space \begin{bmatrix}1 &1 &4\\2 &2&3\\3 &\normalsize-2 &2\end{bmatrix}\begin{bmatrix}1 &1 &\normalsize-1\\0 &1 &0\\0 &0 &1\end{bmatrix}\text{A}\\\text{(Using R}_{1}\xrightarrow{}\text{R}_{1} + \text{R}_{2} - \text{R}_{3})\\\Rarr\space\begin{bmatrix}1 &1 &4\\0 &0 &\normalsize-5\\0 &\normalsize-5 &\normalsize-10\end{bmatrix} = \begin{bmatrix}1 &1 &\normalsize-1\\\normalsize-2 &\normalsize-1 &2\\\normalsize-3&\normalsize-3 &4\end{bmatrix}\text{A}$$
$$\text{(Using R}_{2}\xrightarrow{}\text{R}_{2} = 2R_{1}\\\text{and}\space\text{R}_{3}\xrightarrow{}\text{R}_{3} - 3\text{R}_{1})\\\Rarr\\\space \begin{bmatrix}1 &1 &4\\0 &\normalsize-5 &\normalsize-10\\ 0 &0 &\normalsize-5\end{bmatrix} = \begin{bmatrix}1 &1 &\normalsize-1\\\normalsize-3 &\normalsize-3 &4\\\normalsize-2 &\normalsize-1 &2\end{bmatrix}\text{A}\\\text{(Using R}_{2}\leftrightarrow \text{R}_{3})\\\Rarr\space \begin{bmatrix}1 &1 &4\\0 &1 &2\\ 0 &0 &2\end{bmatrix} = \begin{bmatrix}1 &1 &\normalsize-1\\\frac{3}{5} &\frac{3}{5} &\frac{\normalsize-4}{5}\\\frac{2}{5} &\frac{1}{5}&\frac{\normalsize-2}{5}\end{bmatrix}\text{A}\\\begin{pmatrix}\text{Using}\space\text{R}_{2}\xrightarrow{}\normalsize-\frac{1}{5}\text{R}_{2}\\\text{and R}_{3}\xrightarrow{}-\frac{1}{5}\text{R}_{3}\end{pmatrix}$$
$$\Rarr\space\\\begin{bmatrix}1 &1 &0\\0 &1 &0\\ 0 &0 &1\end{bmatrix} = \begin{bmatrix}-\frac{3}{5} &\frac{1}{5} &\frac{3}{5}\\-\frac{1}{5} &\frac{1}{5} &0\\\frac{2}{5} &\frac{1}{5} &\frac{\normalsize-2}{5}\end{bmatrix}\text{A}\\\text{(Using}\space\text{R}_{2}\xrightarrow{}\text{R}_{2} - 2\text{R}_{3}\space\text{and}\\\text{R}_{1}\xrightarrow{}\text{R}_{1} - 4\text{R}_{3})\\\Rarr\space \begin{bmatrix} 1 &0 &0\\0 &1 &0\\0 &0 &1\end{bmatrix} = \\\begin{bmatrix}-\frac{2}{5} &0 &\frac{3}{5}\\-\frac{1}{5} &\frac{1}{5} &0\\\frac{2}{5} &\frac{1}{5} &-\frac{2}{5}\end{bmatrix}\text{A}\\\text{(Using R}_{1}\xrightarrow{}\text{R}_{1} - \text{R}_{2})$$
$$\Rarr\space \text{I}_{3} = -\frac{1}{5}\begin{bmatrix}2 &0 &\normalsize-3\\1 &\normalsize-1 &0\\\normalsize-2 &\normalsize-1 &2\end{bmatrix}\text{A}\\\therefore\space \text{A}^{\normalsize-1} =-\frac{1}{5}\begin{bmatrix}2 &0 &\normalsize-3\\1 &\normalsize-1 &0\\\normalsize-2 &\normalsize-1 &2\end{bmatrix}$$
(∵ AA–1 = I3)
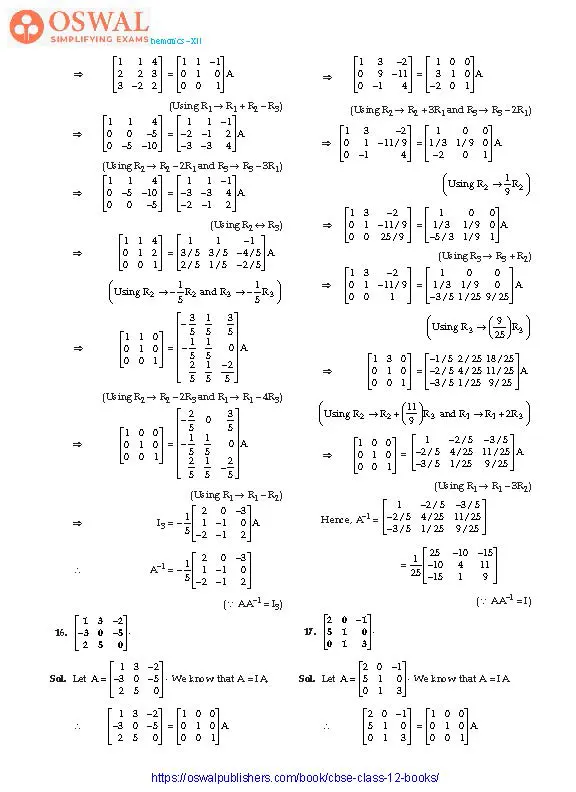
$$\textbf{16.\space}\begin{bmatrix}\textbf{1} &\textbf{3} &\textbf{\normalsize-2}\\\textbf{\normalsize-3} &\textbf{0} &\textbf{\normalsize-5}\\\textbf{2} &\textbf{5} &\textbf{0}\end{bmatrix}\textbf{.}\\\textbf{Sol.\space}\text{Let A = }\begin{bmatrix}1 &3 &\normalsize-2\\\normalsize-3 &0 &\normalsize-5\\2 &5 &0\end{bmatrix}.$$
We know that A = IA,
$$\therefore\space\begin{bmatrix}1 &3 &\normalsize-2\\\normalsize-3 &0 &\normalsize-5\\ 2 &5 &0\end{bmatrix} = \begin{bmatrix}1 &0 &0\\0 &1 &0\\0 &0 &1\end{bmatrix}\text{A}\\\Rarr\space\begin{bmatrix}1 &3 &\normalsize-2\\0 &9 &\normalsize-11\\0 &\normalsize-1 &4\end{bmatrix} = \begin{bmatrix}1 &0 &0\\3 &1 &0\\\normalsize-2 &0 &1\end{bmatrix}\text{A}\\\text{(Using}\space\text{R}_{2}\xrightarrow{}\text{R}_{2}+ 3\text{R}_{1}\\\text{and}\space \text{R}_{3}\xrightarrow{}\text{R}_{3} - 2\text{R}_{1})\\\Rarr\\\begin{bmatrix}1 &3 &\normalsize-2\\0 &1 &\frac{\normalsize-11}{9}\\ 0 &0 &\frac{25}{9}\end{bmatrix} = \begin{bmatrix}1 &0 &0\\\frac{1}{3} &\frac{1}{9} &0\\\normalsize-2 &0 &1\end{bmatrix}\text{A}\\\bigg(\text{Using R}_{2}\xrightarrow{}\frac{1}{9}\text{R}_{2}\bigg)$$
$$\Rarr\space \begin{bmatrix}1 &3 &\normalsize-2\\0 &1 &\frac{-11}{9}\\0 &0 &\frac{25}{9}\end{bmatrix} =\begin{bmatrix}1 &0 &0\\\frac{1}{3} &\frac{1}{9} &0\\-\frac{5}{3} &\frac{1}{9} &1\end{bmatrix}\text{A}\\\text{(Using R}_{3}\xrightarrow{}\text{R}_{3} + \text{R}_{2})\\\Rarr \begin{bmatrix}1 &3 &\normalsize-2\\0 &1 &-\frac{11}{9}\\ 0 &0 &1\end{bmatrix} = \begin{bmatrix}1 &0&0\\\frac{1}{3} &\frac{1}{9} &0\\\frac{-3}{5} &\frac{1}{25} &\frac{9}{25}\end{bmatrix}\text{A}\\\bigg(\text{Using}\space \text{R}_{3}\xrightarrow{}\bigg(\frac{9}{25}\bigg)\text{R}_{3}\bigg)\\\Rarr\space\begin{bmatrix}1 &3 &0\\0 &1 &0\\0&0 &1\end{bmatrix} = \begin{bmatrix}\frac{\normalsize-1}{5} &\frac{2}{25} &\frac{18}{25}\\\frac{\normalsize-2}{5} &\frac{4}{25}&\frac{11}{25}\\\frac{\normalsize-3}{5}&\frac{1}{25}&\frac{9}{25}\end{bmatrix}\text{A}$$
$$\begin{pmatrix}\text{Using R}_{2}\xrightarrow{}\text{R}_{2} + \bigg(\frac{11}{9}\bigg)\text{R}_{3}\\\text{and}\space \text{R}_{1}\xrightarrow{}\text{R}_{1} + 2\text{R}_{3}\end{pmatrix}\\\Rarr\space\begin{bmatrix}1 &0 &0\\0 &1 &0\\0 &0 &1\end{bmatrix} = \begin{bmatrix}1 &\frac{\normalsize-2}{5} &\frac{\normalsize-3}{5}\\\frac{\normalsize-2}{5} &\frac{4}{25}&\frac{11}{25}\\\frac{-3}{5} &\frac{1}{25} &\frac{9}{25}\end{bmatrix}\text{A}\\\text{(Using}\space\text{R}_{1}\xrightarrow{}\text{R}_{1} - 3\text{R}_{2})\\\text{Hence, A}^{\normalsize-1} = \begin{bmatrix}1 &\frac{\normalsize-2}{5} &\frac{\normalsize-3}{5}\\\frac{\normalsize-2}{5} &\frac{4}{25} &\frac{11}{25}\\\frac{\normalsize-3}{25} &\frac{1}{25} &\frac{9}{25}\end{bmatrix}\\=\frac{1}{25}\begin{bmatrix}25 &-10 &-15\\-10 &4 &11\\\normalsize-15 &1 &9\end{bmatrix}$$
(∵ AA–1 = I)
$$\textbf{17.\space}\begin{bmatrix}\textbf{2} &\textbf{0} &\textbf{\normalsize-1}\\\textbf{5} &\textbf{1} &\textbf{0}\\\textbf{0} &\textbf{1} &\textbf{3}\end{bmatrix}\\\textbf{Sol.\space}\text{Let A = }\begin{bmatrix}2 &0 &\normalsize-1\\5 &1 &0\\0 &1 &3\end{bmatrix}.$$
We know that A = IA
$$\therefore\space \begin{bmatrix}2 &0 &\normalsize-1\\5 &1 &0\\0 &1 &3\end{bmatrix} = \begin{bmatrix}1 &0 &0\\0 &1 &0\\0 &0 &1\end{bmatrix}\text{A}\\\Rarr\space\begin{bmatrix}1 &0 &\frac{\normalsize-1}{2}\\5 &1 &0\\0 &1 &3\end{bmatrix} = \begin{bmatrix}\frac{1}{2} &0 &0\\0 &1 &0\\0 &0 &1\end{bmatrix}\text{A}\\\bigg(\text{Using R}_{1}\xrightarrow{}\bigg(\frac{1}{2}\bigg)\text{R}_{1}\bigg)\\\Rarr\space\begin{bmatrix}1 &0 &\frac{\normalsize-1}{2}\\0 &1 &\frac{5}{2}\\0 &1 &3\end{bmatrix} = \begin{bmatrix}\frac{1}{2} &0 &0\\\frac{\normalsize-5}{2} &1 &0\\0 &1 &1\end{bmatrix}\text{A}\\\text{(Using R}_{2}\xrightarrow{}\text{R}_{2} - 5\text{R}_{1})$$
$$\Rarr\space\begin{bmatrix}1 &0 &\frac{\normalsize-1}{2}\\0 &1 &\frac{5}{2}\\0 &1 &\frac{1}{2}\end{bmatrix} = \begin{bmatrix}\frac{1}{2} &0 &0\\-\frac{5}{2} &1 &0\\\frac{5}{2} &\normalsize-1 &0\end{bmatrix}\text{A}\\\text{(Using}\space\text{R}_{3}\xrightarrow{}\text{R}_{3} - \text{R}_{2})\\\Rarr\space \begin{bmatrix}1 &0 &\frac{\normalsize-1}{2}\\0 &1 &\frac{5}{2}\\0 &0 &1\end{bmatrix}=\begin{bmatrix}\frac{1}{2} &0 &0\\\frac{-5}{2} &1 &0\\ 5&\normalsize-2 &2\end{bmatrix}\text{A}\\\text{(Using}\space\text{R}_{3}\xrightarrow{} 2\text{R}_{3})\\\Rarr\space \begin{bmatrix}1 &0 &0\\0 &1 &0\\0 &0 &1\end{bmatrix} = \begin{bmatrix}3 &\normalsize-1 &1\\\normalsize-15 &6 &\normalsize-5\\ 5 &-2 &2\end{bmatrix}\text{A}\\\begin{pmatrix}\text{Using R}_{2}\xrightarrow{}\text{R}_{2}\frac{5}{2}\text{R}_{3}\\\text{and}\space\text{R}_{1} + \frac{1}{2}\text{R}_{3}\end{pmatrix}$$
$$\therefore\space\text{A}^{\normalsize-1} = \begin{bmatrix}3 &\normalsize-1 &1\\-15 &6 &\normalsize-5\\5 &\normalsize-2 &2\end{bmatrix}$$
(∵ AA–1 = I)
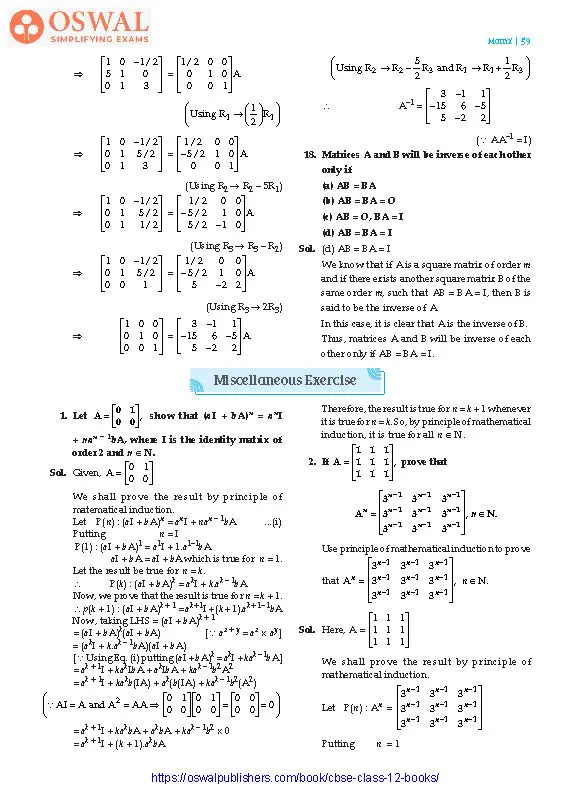
18. Matrices A and B will be inverse of each other only if
(a) AB = BA
(b) AB = BA = O
(c) AB = O, BA = I
(d) AB = BA = I
Sol. (d) AB = BA = I
We know that if A is a square matrix of order m and if there exists another square matrix B of the same order m, such that AB = BA = I, then B is said to be the inverse of A.
In this case, it is clear that A is the inverse of B. Thus, matrices A and B will be inverse of each other only if AB = BA = I.
