NCERT Solutions for Class 12 Maths Chapter 3 Matrix - Miscellaneous Exercise
Access Exercises of Class 12 Maths Chapter 3 – Matrix
Exercise 3.1 Solutions: 10 Questions (7 Short Answers, 3 MCQs)
Exercise 3.2 Solutions: 22 Questions (14 Long, 6 Short, 2 MCQs)
Exercise 3.3 Solutions: 12 Questions (10 Short Answers, 2 MCQs)
Exercise 3.4 Solutions: 18 Questions (4 Long, 13 Short, 1 MCQ)
Miscellaneous Exercise Solutions: 15 Questions (7 Long, 5 Short, 3 MCQs)
Miscellaneous Exercise
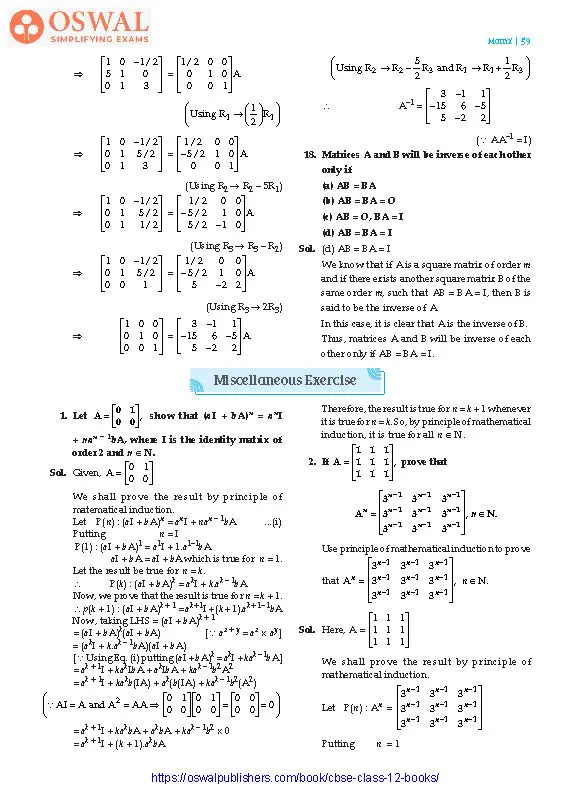
$$\textbf{1. Let A =}\begin{bmatrix}\textbf{0} &\textbf{1}\\\textbf{0}&\textbf{0}\end{bmatrix}\textbf{,}\space\textbf{show that}$$
(aI + bA)n = anI + nan – 1bA, where I is the identity matrix of order 2 and n ∈ N.
$$\textbf{Sol.}\space\text{Given, A = }\begin{bmatrix}0 &1 \\0 &0\end{bmatrix}$$
We shall prove the result by principle of matematical induction.
Let P(n) : (aI + bA)n = anI + nan – 1bA ...(i)
Putting n = I
P(1) : (aI + bA)1 = a1I + 1.a1–1bA
aI + bA = aI + bA which is true for n = 1.
Let the result be true for n = k.
∴ P(k) : (aI + bA)k = akI + k.ak – 1bA
Now, we prove that the result is true for n = k + 1.
∴ p(k + 1) : (aI + bA)k + 1 = ak + 1I + (k + 1).ak + 1 – 1bA
Now, taking LHS = (aI + bA)k + 1
= (aI + bA)k(aI + bA)
[∵ ax + y = ax × ay]
= (akI + k.ak – 1bA)(aI + bA)
[∵ Using Eq. (i) putting (aI + bA)k = akI + kak – 1bA]
= ak + 1I + kakIbA + akIbA + kak – 1b2A2
= ak + 1I + kakb(IA) + ak(b(IA) + kak – 1b2(A2)
$$\begin{pmatrix}\because\space\text{AI = A}\space\text{and}\space \text{A}^{2} = \text{AA}\\\Rarr\space\begin{bmatrix}0 &1 \\0 &0\end{bmatrix} \begin{bmatrix}0 &1 \\0 &0\end{bmatrix} = \begin{bmatrix}0 &0 \\0 &0\end{bmatrix} = 0\end{pmatrix}$$
= ak + 1I + kakbA + akbA + kak – 1b2 × 0
= ak + 1I + (k + 1).akbA
Therefore, the result is true for n = k + 1 whenever it is true for n = k. So, by principle of mathematical induction, it is true for all n ∈ N.
$$\textbf{2. If A =}\space\begin{bmatrix}\textbf{1} &\textbf{1} &\textbf{1}\\\textbf{1} &\textbf{1} &\textbf{1}\\\textbf{1} &\textbf{1} &\textbf{1}\end{bmatrix}\textbf{,}\space\\\textbf{prove that}\\\textbf{A}^{\textbf{n}} = \begin{bmatrix}\textbf{3}^{\textbf{n-1}} &\textbf{3}^{\textbf{n-1}} &\textbf{3}^{\textbf{n-1}}\\\textbf{3}^{\textbf{n-1}} &\textbf{3}^{\textbf{n-1}} &\textbf{3}^{\textbf{n-1}}\\\textbf{3}^{\textbf{n-1}} &\textbf{3}^{\textbf{n-1}} &\textbf{3}^{\textbf{n-1}}\end{bmatrix}\textbf{,}\space \textbf{n}\epsilon\textbf{N}.$$
Use principle of mathematical induction to prove that
$$\textbf{A}^{\textbf{n}} \textbf{=} \begin{bmatrix}\textbf{3}^{\textbf{n-1}} &\textbf{3}^{\textbf{n-1}} &\textbf{3}^{\textbf{n-1}}\\\textbf{3}^{\textbf{n-1}} &\textbf{3}^{\textbf{n-1}} &\textbf{3}^{\textbf{n-1}}\\\textbf{3}^{\textbf{n-1}} &\textbf{3}^{\textbf{n-1}} &\textbf{3}^{\textbf{n-1}}\end{bmatrix}\textbf{,}\space \textbf{n}\epsilon\textbf{N}\textbf{.}$$
$$\textbf{Sol.\space}\text{Here, A = }\begin{bmatrix}1 &1 &1\\1 &1 &1\\1 &1 &1\end{bmatrix}$$
We shall prove the result by principle of mathematical induction.
$$\text{Let P(n) : A}^{n} = \begin{bmatrix}3^{n-1} &3^{n-1} &3^{n-1}\\3^{n-1} &3^{n-1} &3^{n-1}\\3^{n-1} &3^{n-1} &3^{n-1}\end{bmatrix}$$
Putting n = 1
$$\text{P(1) : A}^{1} = \begin{bmatrix}3^{1-1} &3^{1-1} &3^{1-1}\\3^{1-1} &3^{1-1} &3^{1-1}\\3^{1-1} &3^{1-1} &3^{1-1}\end{bmatrix}\\=\begin{bmatrix}3^{0} &3^{0} &3^{0}\\3^{0} &3^{0} &3^{0}\\3^{0} &3^{0} &3^{0}\end{bmatrix} = \begin{bmatrix}1 &1 &1\\1 &1 &1\\1 &1 &1\end{bmatrix}\\\text{...(i)}$$
which is true for n = 1. Let the result be true for n = k.
$$\therefore\space\text{P(K) : A}^{k} = \begin{bmatrix}3^{k-1} &3^{k-1} &3^{k-1}\\3^{k-1} &3^{k-1} &3^{k-1}\\3^{k-1} &3^{k-1} &3^{k-1}\end{bmatrix}\\\text{...(ii)}$$
Putting n = k + 1
$$\therefore\space\text{P}(k+1) : A^{k+1} =\\ \begin{bmatrix}3^{k} &3^{k} &3^{k}\\3^{k} &3^{k} &3^{k}\\3^{k} &3^{k} &3^{k}\end{bmatrix}\\\text{Now,\space LHS = A}^{\text{k+1}} = \text{A}^{\text{k}}.\text{A}^{\text{I}}\\\text{A}^{k}.\text{A}^{I} = \\\begin{bmatrix}3^{k-1} &3^{k-1} &3^{k-1}\\3^{k-1} &3^{k-1} &3^{k-1}\\3^{k-1} &3^{k-1} &3^{k-1}\end{bmatrix}\begin{bmatrix}1 &1 &1\\1 &1 &1\\ 1 &1 &1\end{bmatrix}$$
[Using Eqs. (i) and (ii)]
$$=\\\begin{bmatrix}3^{k-1} + 3^{k-1} + 3^{k-1} &3^{k-1}+3^{k-1}+3^{k-1} &3^{k-1}+3^{k-1}+3^{k-1}\\3^{k-1} + 3^{k-1} + 3^{k-1} &3^{k-1} + 3^{k-1} + 3^{k-1} &3^{k-1} + 3^{k-1}+ 3^{k-1}\\3^{k-1}+3^{k-1}+ 3^{k-1} &3^{k-1} + 3^{k-1} + 3^{k-1} &3^{k-1} + 3^{k-1} + 3^{k-1}\end{bmatrix}$$
Using multiplication of matrix,
$$= \begin{bmatrix}3×3^{k-1} &3×3^{k-1} &3×3^{k-1}\\3×3^{k-1} &3×3^{k-1} &3×3^{k-1}\\3×3^{k-1} &3×3^{k-1} &3×3^{k-1}\end{bmatrix}\\=\begin{bmatrix}3^{k} &3^{k} &3^{k}\\3^{k} &3^{k} &3^{k}\\3^{k} &3^{k} &3^{k}\end{bmatrix} = \text{RHS}$$
Therefore, the result is true for n = k + 1 whenever it is true for n = k. So, by principle of mathematical induction it is true for all n ∈ N.
$$\textbf{3. If\space A} = \begin{bmatrix}\textbf{3} &\textbf{\normalsize-4}\\\textbf{1} &\textbf{\normalsize-1}\end{bmatrix}\textbf{,}\space\textbf{then prove that}\\\textbf{A}^{\textbf{n}} =\begin{bmatrix}\textbf{1+2n} &\textbf{-4n} \\\textbf{n} &\textbf{1-2n}\end{bmatrix}\textbf{,}\space\textbf{where n is any}\\\textbf{positive integer.}$$
Sol. We are required to prove that for all n ∈ N.
$$\text{P(n) = A}^{n} = \begin{bmatrix}1 +2n &-4n \\n &1-2n\end{bmatrix}$$
Let n = 1
$$\text{P(1) : A}^{1} = \begin{bmatrix}1 +2(1) &-4(1)\\1 &1-2(1)\end{bmatrix}\\=\begin{bmatrix}3 &\normalsize-4\\1 &\normalsize-1\end{bmatrix}\\\text{...(i)}$$
∴ which is true for n = 1.
Let the result be true for n = k.
$$\text{P(K) = A}^{k} = \begin{bmatrix}1+2k &-4k\\k &1-2k\end{bmatrix}\\\text{...(ii)}$$
Let n = k + 1
Then, P(k + 1) : Ak + 1 =
$$\begin{bmatrix}1 +2(k+1) &-4(k+1)\\k+1 &1-2(k+1)\end{bmatrix}\\=\begin{bmatrix}2k+3 &-4k+4\\k+1 &-2k-1\end{bmatrix}$$
Now, LHS = Ak + 1 = AkA1
$$= \begin{bmatrix}1 +2k &-4k \\k &1-2k\end{bmatrix}\begin{bmatrix}3 &-4 \\1 &\normalsize-1\end{bmatrix}$$
[Using Eqs. (i) and (ii)]
$$=\\\begin{bmatrix}(1 +2k).3 + (\normalsize-4k).1 &(1 + 2k).(\normalsize-4) + (\normalsize-4k).(\normalsize-1)\\k.3 +(1 - 2k).1 &k.(\normalsize-4) + (1-2k).(\normalsize-1)\end{bmatrix}\\=\begin{bmatrix} 3+2k & -4-4k\\k+1 &-1-2k\end{bmatrix}\\=\begin{bmatrix}1 + 2(k+1) &-4(k+1)\\k+1 &1-2(k+1)\end{bmatrix} $$
Therefore, the result is true for n = k + 1 whenever it is true for n = k. So, by principle of mathematical induction, it is true for all n ∈ N.
4. If A and B are symmetric matrices, prove that AB – BA is a skew-symmetric matrix.
Sol. Here, A and B are symmetric matrices, then
A′ = A and B′ = B.
Now, (AB – BA)′ = (AB)′ – (BA)′
[∵ (A – B)′ = A′ – B′ and (AB)′ = B′A′)
= B′A′ – A′B′
= BA – AB
(∵ B′ = B and A′ = A)
= – (AB – BA)
∴ (AB – BA)′ = – (AB – BA)
Thus, (AB – BA) is a skew-symmetric matrix.
5. Show that the matrix B′AB is symmetric or skew-symmetric according as A is symmetric or skew-symmetric.
Sol. We suppose that A is a symmetric matrix, then
A′ = A
Consider (B′AB) = {B′(AB)}′ = (AB)′(B′)′
[∵ (AB)′ = B′A′]
= B′A′(B) [∵ (B′) = B]
= B′(A′B) = B′(AB)
[∵ A′ = A]
∴ (B′AB)′ = B′AB
which shows that B′AB is a symmetric matrix.
Now, we suppose that A is a skew-symmetric
matrix.
Then, A′ = – A
Consider (B′AB)′ = [B′(AB)]′
= (AB)′(B′)′
[∵ (AB)′ = B′A′ and (B′)′ = B]
= (B′A′)B = B′(– A)B
= – B′AB [∵ A′ = – A]
∴ (B′AB)′ = – B′AB which shows that B′AB is a skew-symmetric matrix.
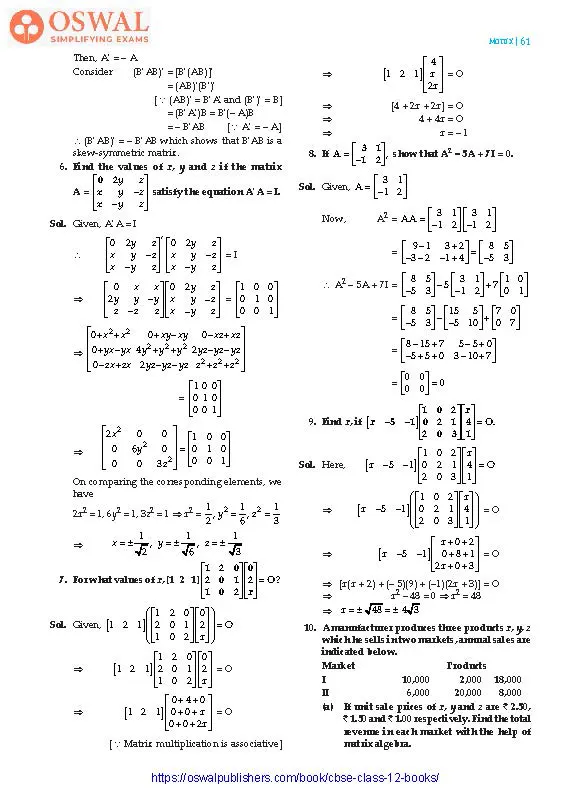
6. Find the values of x, y and z if the matrix
$$\text{A = }\begin{bmatrix}\textbf{0} &\textbf{2y} &\textbf{z}\\\textbf{x} &\textbf{y} &\textbf{\normalsize-z}\\\textbf{x} &\textbf{-y} &\textbf{z}\end{bmatrix}\space\\\textbf{satisfy the equation A}'\textbf{A = I.}$$
Sol. Given, A′A = I
$$\therefore\space \begin{bmatrix}0 &2y &z\\x &y &\normalsize-z\\x &\normalsize-y &\normalsize-z\end{bmatrix}\begin{bmatrix}0 &2y &z\\x &y &\normalsize-z\\x &\normalsize-y &\normalsize-z\end{bmatrix} \\=\space\text{I}\\\Rarr\space\begin{bmatrix}0 &x &x\\2y &y &\normalsize-y\\z &\normalsize-z &z\end{bmatrix}\begin{bmatrix}0 &2y &z\\x &y &-z\\x &-y &z\end{bmatrix}\\=\begin{bmatrix}1 &0 &0\\0 &1 &0\\0&0 &1\end{bmatrix}\\\Rarr\\\space\begin{bmatrix}0 +x^{2}+x^{2} &0+xy-xy &0-xz+xz\\0+yx-yx &4y^{2}+y^{2}+y^{2} &2yz-yz-yz\\0-zx+zx &2yz-yz-yz &z^{2}+z^{2}+z^{2}\end{bmatrix}$$
$$= \begin{bmatrix}1 &0 &0\\0 &1 &0\\0 &0 &1\end{bmatrix}\\\Rarr\\\space \begin{bmatrix}2x^{2} &0 &0\\0 &6y^{2} &0\\0 &0 &3z^{2}\end{bmatrix} = \begin{bmatrix}1 &0 &0\\0 &1 &0\\0 &0 &1\end{bmatrix} $$
On comparing the corresponding elements, we have
2x2 = 1, 6y2 = 1, 3z2 = 1
$$\Rarr\space x^{2} =\frac{1}{2}, y^{2} =\frac{1}{6}, z^{2} =\frac{1}{3}\\\Rarr\space x=\pm\frac{1}{\sqrt{2}}, y =\pm\frac{1}{\sqrt{6}} = z=\pm\frac{1}{\sqrt{3}}$$
7. For what values of x,
$$\begin{bmatrix}\textbf{1} &\textbf{2} &\textbf{1}\end{bmatrix}\begin{bmatrix}\textbf{1} &\textbf{2} &\textbf{0}\\\textbf{2} &\textbf{0} &\textbf{1}\\\textbf{1} &\textbf{0} &\textbf{2}\end{bmatrix}\begin{bmatrix}\textbf{0}\\\textbf{2}\\\textbf{x}\end{bmatrix}\\\textbf{= O}\space\textbf{?}\\\textbf{Sol.\space}\text{Given,}\\\begin{bmatrix}1 &2 &1\end{bmatrix}\begin{pmatrix} \begin{bmatrix}1 &2 &0\\ 2 &0 &1\\1 &0 &2\end{bmatrix}\begin{bmatrix}\textbf{0}\\\textbf{2}\\\textbf{x}\end{bmatrix}\end{pmatrix} \text{= O}\\\Rarr\space \\\begin{bmatrix}1 &2 &1\end{bmatrix}\begin{bmatrix}1 &2 &0\\2 &0 &1\\1&0 &2\end{bmatrix}\begin{bmatrix}0\\2\\ x\end{bmatrix} =\text{O}\\\Rarr\space \begin{bmatrix}1 &2 &1\end{bmatrix}\begin{bmatrix}0+4+0\\0+0+x\\0+0+2x\end{bmatrix} =\text{O}$$
[∵ Matrix multiplication is associative]
$$\Rarr\space \begin{bmatrix}1&2&1\end{bmatrix}\begin{bmatrix}4\\x\\2x\end{bmatrix} =\text{O}$$
$$\Rarr\space\lbrack4 + 2x+2x\rbrack =\text{O}\\\Rarr\space 4+4x = \text{O}\\\Rarr\space x =\normalsize-1$$
$$\textbf{8.\space If A = }\begin{bmatrix}\textbf{3} &\textbf{1} \\\textbf{\normalsize-1} &\textbf{2}\end{bmatrix}\textbf{,}\\\textbf{show that A}^2 \textbf{– 5A + 7I = 0.}\\\textbf{Sol.\space}\text{Given, A = }\begin{bmatrix}3 &1 \\\normalsize-1 &2\end{bmatrix}\\\text{Now, A}^{2} \text{= AA} = \\\begin{bmatrix}3 &1\\\normalsize-1 &2\end{bmatrix}\begin{bmatrix}3 &1\\\normalsize-1 &2\end{bmatrix}\\=\begin{bmatrix}9-1 &3+2 \\-3-2 &-1+4\end{bmatrix}= \begin{bmatrix}8 &5\\\normalsize-5 &3\end{bmatrix}\\\therefore\space \text{A}^{2} - \text{5A +7I} =\\\begin{bmatrix}8 &5\\\normalsize-5 &3\end{bmatrix}-5\begin{bmatrix}3 &1\\\normalsize-1 &2\end{bmatrix} +7\begin{bmatrix}1 &0\\0 &1\end{bmatrix}$$
$$=\begin{bmatrix}8 &5 \\\normalsize-5 &3\end{bmatrix} - \begin{bmatrix}15 &5 \\\normalsize-5 &10\end{bmatrix}+\\\begin{bmatrix}7 &0\\0 &7\end{bmatrix}\\= \begin{bmatrix}8-15+7 &5-5+0\\-5+5+0 & 3-10+7\end{bmatrix}\\=\begin{bmatrix}0 &0\\0&0\end{bmatrix} = 0$$
$$\textbf{9. Find x, if}\space\begin{bmatrix}\textbf{x} &\textbf{\normalsize-5} &\textbf{\normalsize-1}\end{bmatrix}\\\begin{bmatrix}\textbf{1} &\textbf{0} &\textbf{2}\\\textbf{0} &\textbf{2} &\textbf{1}\\\textbf{2} &\textbf{0} &\textbf{3}\end{bmatrix}\begin{bmatrix} \textbf{x}\\\textbf{4}\\\textbf{1}\end{bmatrix} = \textbf{O.}\\\textbf{Sol.\space}\text{Here,}\\\begin{bmatrix}x &\normalsize-5 &\normalsize-1\end{bmatrix}\begin{bmatrix}1 &0 &2\\0 &2 &1\\2 &0 &3\end{bmatrix}\begin{bmatrix}x\\ 4\\1\end{bmatrix}\\=\text{O}\\\Rarr\space\begin{bmatrix}x &\normalsize-5 &\normalsize-1\end{bmatrix}\\\begin{pmatrix}\begin{bmatrix}1 &0 &2\\0 &2 &1\\2 &0 &3\end{bmatrix}\begin{bmatrix}x\\4\\1\end{bmatrix}\end{pmatrix} =\text{O}$$
$$\Rarr\space\\\begin{bmatrix}x &\normalsize-5 &\normalsize-1\end{bmatrix}\begin{bmatrix}x+0+2\\0+8+1\\2x+0+3\end{bmatrix}\\= \text{O}\\\Rarr\space\lbrack x(x+2) + (\normalsize-5)(9) +\\ (\normalsize-1)(2x+3)\rbrack = \text{O}\\\Rarr\space x^{2}-48=0\\\Rarr\space x^{2} = 48\\\Rarr\space x = \pm\sqrt{48} \\=\pm4\sqrt{3}$$
10. A manufacturer produces three products x, y, z which he sells in two markets, annual sales are indicated below.
| Market | |||
| I | 10,000 | 2,000 | 18,000 |
| II | 6,000 | 20,000 | 8,000 |
(a) If unit sale prices of x, y and z are ₹ 2.50, ₹ 1.50 and ₹ 1.00 respectively. Find the total revenue in each market with the help of matrix algebra.
(b) If the unit costs of the above three commodities are ₹ 2.00, ₹ 1.00 and 50 paise respectively. Find the gross profit.
Sol. Matrix representing the sales is
$$\text{S} = \begin{bmatrix}10000 &2000 &18000\\6000 &20000 &8000\end{bmatrix}$$
(a) Matrix representing the sale price per unit
is
$$\text{B = }\begin{bmatrix}2.50 \\1.50\\1.00\end{bmatrix}$$
Total revenue in each market is given by the product.
$$\text{AB} =\\\begin{bmatrix}10000 &2000 &18000\\6000 &20000 &8000\end{bmatrix}\begin{bmatrix}\frac{5}{2}\\\frac{3}{2}\\1\end{bmatrix}\\=\begin{bmatrix}10000×\frac{5}{2} + 2000×\\\frac{3}{2} + 18000×1\\6000×\frac{5}{2} +\\ 20000×\frac{3}{2} + 8000×1\end{bmatrix}\\=\begin{bmatrix}25,000 + 3,000 + 18,000\\15,000 + 30,000 + 8,000\end{bmatrix}\\=\begin{bmatrix}46,000\\53,000\end{bmatrix}$$
Hence, total revenue of market I = ₹ 46,000
Total revenue of market II = ₹53,000
(b) Let, the unit cost price of products x, y and z per market be denoted by matrix C.
$$\text{Let C = }\begin{bmatrix}2.00\\1.00\\0.50\end{bmatrix} \begin{array}{cc}x \\ y\\z\end{array}\\\text{Total cost =}\\\begin{bmatrix}10,000 &2,000 &18,000\\6,000 &20,000 &8,000\end{bmatrix}\begin{bmatrix}2.00\\1.00\\0.50\end{bmatrix}\\=\begin{bmatrix}20,000 + 2,000 + 9,000\\12,000 + 20,000 + 4,000\end{bmatrix}\\=\begin{bmatrix}31000\\36000\end{bmatrix}$$
Profit in market I = ₹ (46000 – 31000)
= ₹15000
and profit in market II = ₹ (53000 – 36000)
= ₹ 17000
∴ The gross profit = ₹ (15000 + 17000)
= ₹ 32000
11. Find the matrix X so that
$$\textbf{X}\begin{bmatrix}\textbf{1} &\textbf{2} &\textbf{3}\\\textbf{4} &\textbf{5} &\textbf{6}\end{bmatrix} = \begin{bmatrix}\textbf{\normalsize-7} &\textbf{-8} &\textbf{-9}\\\textbf{2} &\textbf{4} &\textbf{6}\end{bmatrix}\textbf{.}\\\textbf{Sol.\space}\text{Here, X}\\\begin{bmatrix}1 &2 &3\\4 &5 &6\end{bmatrix} =\begin{bmatrix}\normalsize-7 &\normalsize-8 &\normalsize-9\\2 &4 &6\end{bmatrix}$$
The matrix given on the RHS of the equation is a 2 × 3 matrix and the one given on the LHS of the equation is as a 2 × 3 matrix. Therefore, X has to be a 2 × 2 matrix.
$$\text{Now, let X = }\begin{bmatrix}a &c \\b & d\end{bmatrix}\\\text{Therefore, we have}\\\begin{bmatrix}a &c \\b & d\end{bmatrix}\begin{bmatrix}1 &2 &3\\4 &5 &6\end{bmatrix}\\=\begin{bmatrix}\normalsize-7 &\normalsize-8 &\normalsize-9\\2 &4 &6\end{bmatrix}\\\Rarr\space\begin{bmatrix}a+4c &2a+5c &3a+6c\\b+4d &2b+5d &3b+6d\end{bmatrix}\\=\begin{bmatrix}-7 &-8 &-9\\2 &4 &6\end{bmatrix}$$
Equating the corresponding elements of the two matrices, we have
a + 4c = – 7, 2a + 5c = – 8, 3a + 6c = – 9
b + 4d = 2, 2b + 5d = 4, 3b + 6d = 6
Now, a + 4c = – 7 ⇒ a = – 7 – 4c
2a + 5c = – 8 ⇒ – 14 – 8c + 5c = – 8
⇒ – 3c = 6 ⇒ c = – 2
∴ a = – 7 – 4(– 2) = – 7 + 8 = 1
Now, b + 4d = 2 ⇒ b = 2 – 4d and 2b + 5d = 4
⇒ 4 – 8d + 5d = 4
⇒ –3d = 0 ⇒ d = 0
∴ b = 2 – 4(0) = 2
Thus, a = 1, b = 2, c = – 2, d = 0
Hence, the required matrix
$$\text{X is}\begin{bmatrix}1 &\normalsize-2\\2 &0\end{bmatrix}.$$
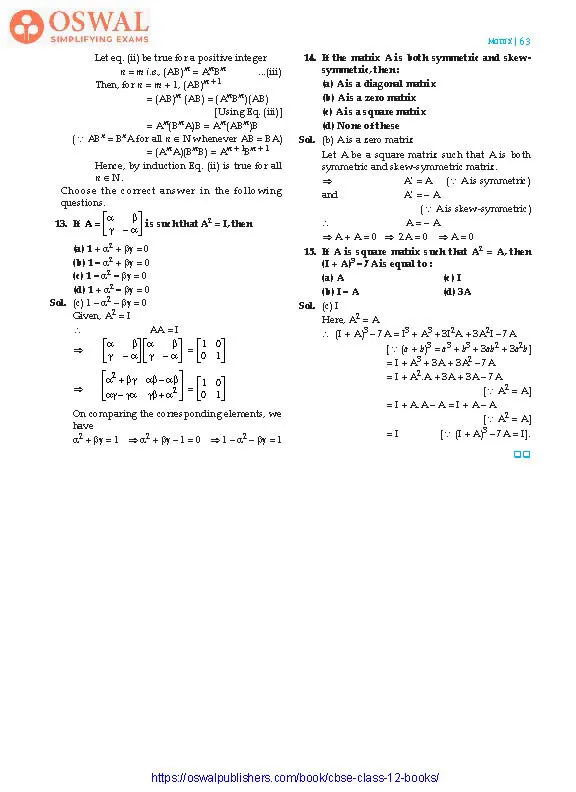
12. If A and B are square matrices of the same order such that AB = BA, then prove by induction that ABn = BnA. Further, prove that (AB)n = AnBn for all n ∈ N.
Sol. (a) Given, that AB = BA ...(i)
We want to prove that ABn = BnA ...(ii)
For n = 1, eq. (ii) is obviously true.
Let eq. (ii) be true for a positive integer n = m.
i.e., ABm = BmA ...(iii)
Then, for n = m + 1, ABm + 1 = A(BmB) = (ABm)B
(Associative law of matrix multiplication)
= (BmA)B [Using Eq. (iii)]
= Bm(AB) = Bm(BA)
[Using Eq. (i)]
= (BmB)A = Bm + 1A
Hence, by induction Eq. (ii) is true for all n ∈ N.
(b) Here, given that
AB = BA ...(i)
We want to prove that
(AB)n = AnBn ...(ii)
For n = 1, eq. (ii) is obviously true.
[∵ from eq. (i)]
Let eq. (ii) be true for a positive integer
n = m i.e., (AB)m = AmBm ...(iii)
Then, for n = m + 1, (AB)m + 1
= (AB)m (AB) = (AmBm)(AB)
[Using Eq. (iii)]
= Am(BmA)B = Am(ABm)B
(∵ ABn = BnA for all n ∈ N whenever AB = BA)
= (AmA)(BmB) = Am + 1Bm + 1
Hence, by induction Eq. (ii) is true for all n ∈ N.
Choose the correct answer in the following questions.
$$\textbf{13. If A =}\begin{bmatrix}\alpha &\beta\\\gamma &\normalsize-\alpha\end{bmatrix}\space$$
is such that A2 = I, then
(a) 1 + α2 + βγ = 0
(b) 1 – α2 + βγ = 0
(c) 1 – α2 – βγ = 0
(d) 1 + α2 – βγ = 0
Sol. (c) 1 – α2 – bγ = 0
Given, A2 = I
∴ AA = I
$$\Rarr\space\begin{bmatrix}\alpha &\beta\\\gamma &-\alpha\end{bmatrix}\begin{bmatrix}\alpha &\beta\\\gamma &-\alpha\end{bmatrix}\\= \begin{bmatrix}1 &0\\0 &1\end{bmatrix}\\\Rarr\space \begin{bmatrix}\alpha^{2} +\beta\gamma &\alpha\beta -\alpha\beta\\\alpha\gamma-\gamma\alpha &\gamma\beta +\alpha^{2}\end{bmatrix}\\=\begin{bmatrix}1 &0\\0 &1\end{bmatrix}$$
On comparing the corresponding elements, we have
$$\alpha^{2} +\beta\gamma = 1\\\Rarr\space \alpha^{2} + \beta\gamma-1 =0\\\Rarr\space 1 -\alpha^{2} -\beta\gamma = 1$$
14. If the matrix A is both symmetric and skewsymmetric, then :
(a) A is a diagonal matrix
(b) A is a zero matrix
(c) A is a square matrix
(d) None of these
Sol. (b) A is a zero matrix
Let A be a square matrix such that A is both
symmetric and skew-symmetric matrix.
⇒ A′ = A (∵ A is symmetric)
and A′ = – A
(∵ A is skew-symmetric)
∴ A = – A
$$\Rarr\space \text{A + A = 0}\\\Rarr\space\text{2A = 0}\\\Rarr\space\text{A = 0}$$
15. If A is square matrix such that A2 = A, then (I + A)3 – 7A is equal to :
(a) A
(b) I – A
(c) I
(d) 3A
Sol. (c) I
Here, A2 = A
∴ (I + A)3 – 7A = I3 + A3 + 3I2A + 3A2I – 7A
[∵ (a + b)3 = a3 + b3 + 3ab2 + 3a2b]
= I + A3 + 3A + 3A2 – 7A
= I + A2.A + 3A + 3A – 7A
[∵ A2 = A]
= I + A.A – A = I + A – A
[∵ A2 = A]
= I [∵ (I + A)3 – 7A = I].
