NCERT Solutions for Class 12 Maths Chapter 6 - Application of Derivatives - Exercise 6.2
Access Exercises of Class 12 Maths Chapter 6 Application of Derivatives
Exercise 6.1 Solutions: 18 Questions (10 Long, 6 Short, 2 MCQs)
Exercise 6.2 Solutions: 19 Questions (10 Long, 7 Short, 2 MCQs)
Exercise 6.3 Solutions: 27 Questions (14 Long, 11 Short, 2 MCQs)
Exercise 6.4 Solutions: 9 Questions (7 Short, 2 MCQs)
Exercise 6.5 Solutions: 29 Questions (15 Long, 11 Short, 3 MCQs)
Miscellaneous Exercise Solutions: 24 Questions (14 Long, 4 Short, 6 MCQs)
Exercise 6.2
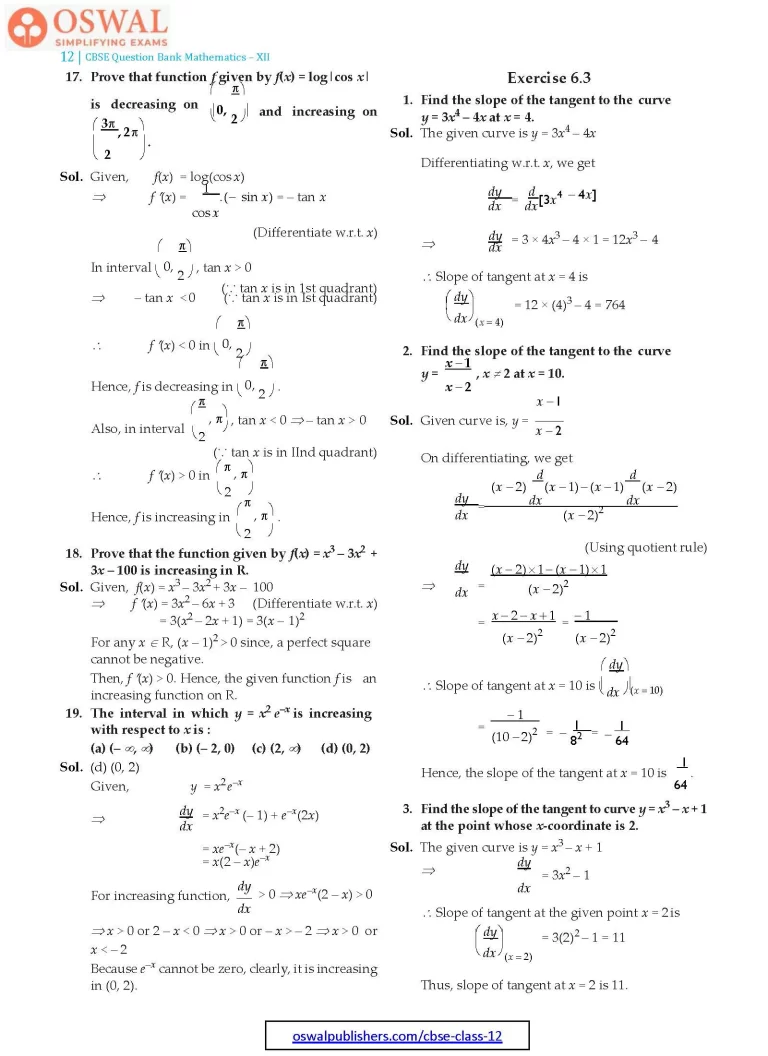
1. Show that the function given by f(x) = 3x + 17 is increasing on R.
Sol. Let x1 and x2 be any two numbers in R, where x1 < x2.
Then, we have
f(x) = 3x + 17
⇒ f(x1) = 3x1 + 17
and
f(x2) = 3x2 + 17
[∵ f(x) = 3x + 17 (given)]
Now , we see that f(x1) < f(x2).
Hence, f is strictly increasing on R.
Alternate method
Given, f(x) = 3x + 17. On differentiating w.r.t. x, we get
f ′(x) = 3 > 0, in every interval of R.
Thus, the function is increasing on R.
2. Show that the function given by f(x) = e2x is increasing on R.
Sol. Let x1 and x2 be any two numbers in R, where
x1 < x2.
Given, f(x) = e2x
Then, we have x1 < x2
⇒ 2x1 < 2x2
⇒ e2x1 < e2x2
⇒ f(x1) < f(x2)
Hence, f is strictly increasing on R.
Alternate method
Given, f(x) = e2x
On differentiating both sides w.r.t. x, we get
$$\frac{d}{dx}f(x)=\frac{d}{dx}(e^{2x})$$
⇒ f ′(x) = 2e2x
(i) When x > 0, then
$$2e^{2x}=2\bigg[1+2x+\frac{(2x)^2}{2!}+...\bigg]>0$$
∴ f ′(x) > 0
(ii) When x = 0, then
2e2x = 2.1 = 2 > 0
∴ f ′(x) > 0
(iii) When x < 0, then
2e2x = 2 × e2(– z)
$$=\frac{2}{e^{2z}}\space\text{(Here x = – z, where z > 0)}\\=\frac{2}{\bigg[1+2z+\frac{(2z)^2}{2!}+.....\bigg]}>0$$
∴ f ′(x) > 0
Thus, for all values of x, f ′(x) > 0. So, f(x) is strictly increasing function on R.
3. Show that the function given by f(x) = sin x is
$$\textbf{(a)\space increasing in}\space\bigg(\textbf{0},\frac{\pi}{\textbf{2}}\bigg)\\\textbf{(b)\space decreasing in}\space\bigg(\frac{\pi}{\textbf{2}},\pi\bigg).$$
(c) Neither increasing nor decreasing in (0, π).
Sol. The given function is f(x) = sin x
On differentiating w .r.t. x, we get f ′(x) = cos x
$$\textbf{(a)}\space\text{Since, for each x}\epsilon\space\bigg(0,\frac{\pi}{2}\bigg),\\\text{cos x > 0;}\\\text{we have f}^ ′(x) > 0\\(\because\space \text{cos x in Ist quadrant is positive}).\\\text{Hence, f is increasing in}\space\bigg(0,\frac{\pi}{2}\bigg).\\\textbf{(b)\space}\text{Since, for each x}\epsilon\bigg(\frac{\pi}{2},\pi\bigg),\\\text{cos x < 0;}\\\text{we have}\space f'(x)>0$$
(∵ cos x in IInd quadrant is negative)
$$\text{Hence, f is decreasing in}\space\bigg(\frac{\pi}{2},\pi\bigg).$$
(c) When x ∈ (0, π). We see that f ′(x) > 0 in
$$\bigg(0,\frac{\pi}{2}\bigg)\space\text{and f'(x)< 0 in}\space\bigg(\frac{\pi}{2},\pi\bigg).$$
So, f ′(x) is positive and negative in (0, π).
Thus, f(x) is neither increasing nor decreasing in (0, π).
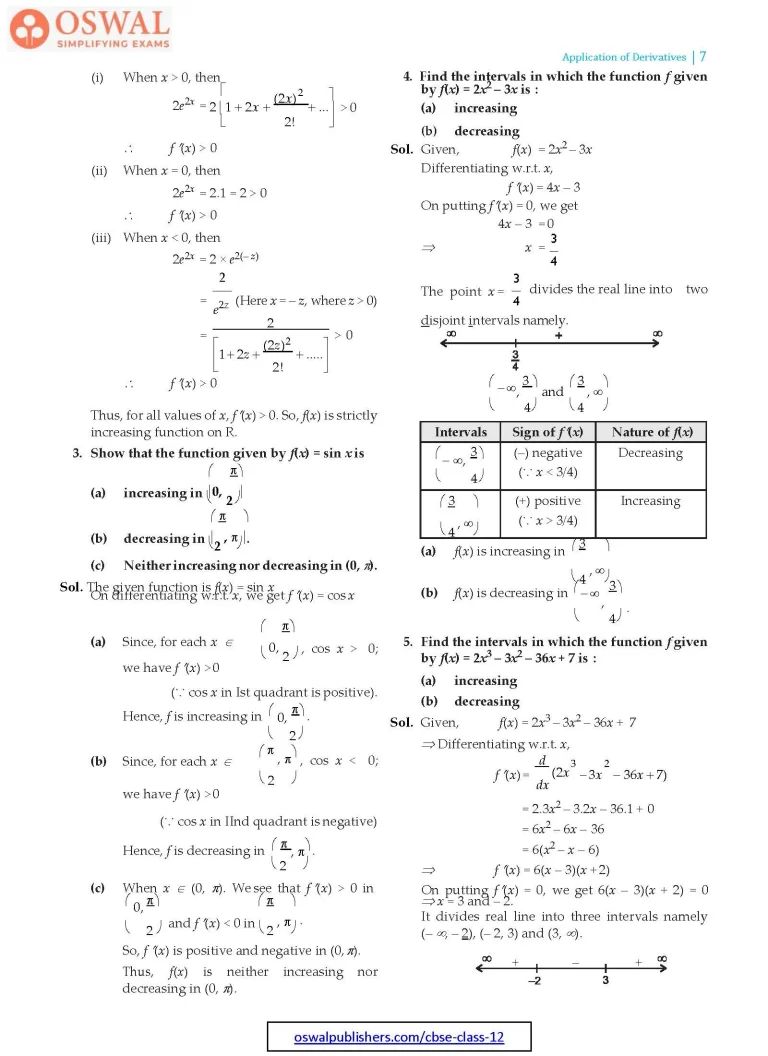
4. Find the intervals in which the function f given by f(x) = 2x2 – 3x is :
(a) increasing
(b) decreasing
Sol. Given, f(x) = 2x2 – 3x
Differentiating w.r.t. x,
f ′(x) = 4x – 3
On putting f ′(x) = 0, we get
4x – 3 = 0
$$\Rarr\space x=\frac{3}{4}$$
$$\text{The point x =}\frac{3}{4}\space\text{divides the real line into}\\\text{two disjoint intervals namely.}$$
$$\bigg(-\infty,\frac{3}{4}\bigg)\space\text{and}\space\bigg(\frac{3}{4},\infty\bigg)$$
| Intervals | Sign of f ′(x) | Nature of f(x) |
| $$\bigg(-\infty,\frac{3}{4}\bigg)$$ | (–) negative (∵ x < 3/4) |
Decreasing |
| $$\bigg(\frac{3}{4},\infty\bigg)$$ | (+) positive (∵ x > 3/4) |
Increasing |
$$\textbf{(a)\space}\text{f(x) is increasing in}\space\bigg(\frac{3}{4},\infty\bigg)\\\textbf{(b)}\space\text{f(x) is decreasing in}\space\bigg(-\infty,\frac{3}{4}\bigg).$$
5. Find the intervals in which the function f given by f(x) = 2x3 – 3x2 – 36x + 7 is :
(a) increasing
(b) decreasing
Sol. Given, f(x) = 2x3 – 3x2 – 36x + 7
⇒ Differentiating w.r.t. x,
$$\text{f'(x)}=\frac{d}{dx}\space(2x^3-3x^2-36x+7)$$
= 2.3x2 – 3.2x – 36.1 + 0
= 6x2 – 6x – 36
= 6(x2 – x – 6)
⇒ f ′(x) = 6(x – 3)(x + 2)
On putting f ′(x) = 0, we get 6(x – 3)(x + 2) = 0
⇒ x = 3 and – 2.
It divides real line into three intervals namely
(– ∞, – 2), (– 2, 3) and (3, ∞).
| Intervals | Sign of f ′(x) | Nature of f(x) |
| (– ∞, – 2) | (–) (–) = + ve (∵ x < – 2 i.e., x is – 3, – 4, – 5 ... for these values, (x – 3) and (x – 2) both will be negative) |
Increasing |
| (– 2, 3) | (–) (+) = – ve (∵ 3 > x < – 2 i.e., x is 1, 2, 0, – 1 ... for these values, (x – 3) will be negative and (x + 2) will be positive) |
Decreasing |
| (3, ∞) | (+) (+) = + ve (∵ x > 3 i.e., x is 4, 5, 6, ... for these values, (x – 3) and (x + 2) both are positive) |
Increasing |
(a) Function is increasing in (– ∞, – 2) ∪ (3, ∞).
(b) Function is decreasing in (– 2, 3).
6. Find the interval in which the following functions are strictly increasing or decreasing :
(a) x2 + 2x – 5
(b) 10 – 6x – 2x2
(c) – 2x3 – 9x2 – 12x + 1
(d) 6 – 9x – x2
(e) (x + 1)3 (x – 3)3
Sol. (a) Let f(x) = x2 + 2x – 5
⇒ f ′(x) = 2x + 2
(By differentiating w.r.t. x)
Putting f ′(x) = 0, we get
2x + 2 = 0
⇒ 2x = – 2
⇒ x = – 1
x = – 1 divides real line into two intervals namely (– ∞, – 1) and (– 1, ∞).
| Intervals | Sign of f ′(x) | Nature of f(x) |
| (– ∞, – 1) | –ve | Strictly Decreasing |
| (– 1, ∞) | +ve | Strictly Increasing |
Therefore, f(x) is strictly increasing when
x > – 1 and strictly decreasing when x < – 1.
(b) Let f(x) = 10 – 6x – 2x2
⇒ f ′(x) = 0 – 6 – 2.2x
= – 6 – 4x
On putting f ′(x) = 0, we get
– 6 – 4x = 0
$$\Rarr\space x=\frac{-3}{2}$$
which divides real line into two intervals namely
$$\bigg(-\infty,\frac{-3}{2}\bigg)\space\text{and}\space\bigg(\frac{-3}{2},\infty\bigg).$$
| Intervals | Sign of f ′(x) | Nature of f(x) |
| (– ∞, – 3/2) | + ve | Strictly Increasing |
| (– 3/2, ∞) | – ve | Strictly Decreasing |
$$\text{Hence, f is strictly increasing for}\space\\x\lt-\frac{3}{2}\\\text{and strictly decreasing for x}\gt -\frac{3}{2}.$$
(c) Given, f(x) = – 2x3 – 9x2 – 12x + 1
⇒ f ′(x) = – 2.3x2 – 9.2x – 12
= – 6x2 – 18x – 12
On putting f ′(x) = 0, we get
– 6x2 – 18x – 12 = 0
⇒ – 6(x + 2)(x + 1) = 0
⇒ x = – 2, – 1
which divides real line into three intervals (– ∞, – 2), (– 2, – 1) and (– 1, ∞).
| Intervals | Sign of f ′(x) | Nature of f(x) |
| (– ∞, – 2) | (–)(–)(–) = – ve | Strictly Decreasing |
| (– 2, – 1) | (–)(–)(+) = + ve | Strictly Increasing |
| (– 1, ∞) | (–)(+)(+) = – ve | Strictly Decreasing |
Therefore, f(x) is strictly increasing in – 2 < x < – 1 and strictly decreasing for x < – 2 and x > – 1.
(d) Given, f(x) = 6 – 9x – x2
⇒ f ′(x) = – 9 – 2x
On putting f ′(x) = 0, we get
– 9 – 2x = 0
$$\Rarr\space x=-\frac{9}{2}.$$
which divides the real line in two disjoint intervals
$$\bigg(-\infty,\frac{-9}{2}\bigg)=\bigg(\frac{-9}{2},\infty\bigg) $$
| Intervals | Sign of f ′(x) | Nature of f(x) |
| (– ∞, – 9/2) | + ve | Strictly Increasing |
| (– 9/2, ∞) | – ve | Strictly Decreasing |
Therefore, f(x) is strictly increasing when
$$x\lt-\frac{9}{2}\space\text{and strictly decreasing when}\\x\gt-\frac{9}{2}.$$
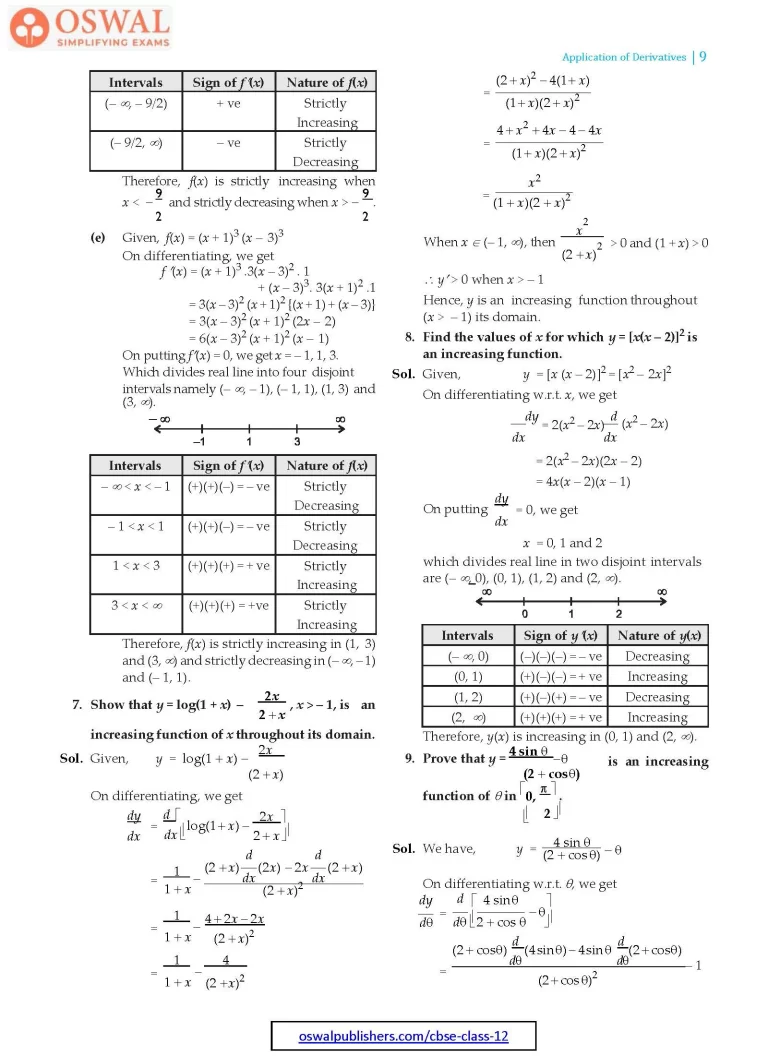
(e) Given, f(x) = (x + 1)3 (x – 3)3
On differentiating, we get
f ′(x) = (x + 1)3 .3(x – 3)2 . 1
+ (x – 3)3. 3(x + 1)2 .1
= 3(x – 3)2 (x + 1)2 {(x + 1) + (x – 3)}
= 3(x – 3)2 (x + 1)2 (2x – 2)
= 6(x – 3)2 (x + 1)2 (x – 1)
On putting f ′(x) = 0, we get x = – 1, 1, 3.
Which divides real line into four disjoint intervals namely (– ∞, – 1), (– 1, 1), (1, 3) and (3, ∞).
| Intervals | Sign of f ′(x) | Nature of f(x) |
| – ∞ < x < – 1 | (+)(+)(–) = – ve | Strictly Decreasing |
| – 1 < x < 1 | (+)(+)(–) = – ve | Strictly Decreasing |
| 1 < x < 3 | (+)(+)(+) = + ve | Strictly Increasing |
| 3 < x < ∞ | (+)(+)(+) = +ve | Strictly Increasing |
Therefore, f(x) is strictly increasing in (1, 3) and (3, ∞) and strictly decreasing in (– ∞, – 1) and (– 1, 1).
$$\textbf{7. Show that y = log(1 + x)-}\frac{\textbf{2x}}{\textbf{2+x}},\\\textbf{x}\gt\textbf{-1},\space\textbf{is an increasing function}\\\textbf{ of x throughout its domain.}$$
$$\textbf{Sol.}\space\text{Given}\space y=\text{log(1+x)}-\frac{2x}{2+x}$$
On differentiating, we get
$$\frac{dy}{dx}=\frac{d}{dx}\bigg[\text{log(1+x)}-\frac{2x}{2+x}\bigg]\\=\frac{1}{1+x}-\frac{(2+x)\frac{d}{dx}(2x)-2x\frac{d}{dx}(2+x)}{(2+x)^2}\\=\frac{1}{1+x}-\frac{4+2x-2x}{(2+x)^2}\\=\frac{1}{1+x}-\frac{4}{(2+x)^2}\\=\frac{(2+x)^2-4(1+x)}{(1+x)(2+x)^2}\\=\frac{4+x^2+4x-4-4x}{(1+x)(2+x)^2}\\=\frac{x^2}{(1+x)(2+x)^2}$$
When x ∈ (– 1, ∞), then
$$\frac{x^2}{(2+x)^2}\gt0\space\text{and (1 + x)} \gt 0\\\therefore\space y'\gt0\space \text{when x > – 1}$$
Hence, y is an increasing function throughout (x > – 1) its domain.
8. Find the values of x for which y = [x(x – 2)]2 is an increasing function.
Sol. Given, y = [x (x – 2)]2 = [x2 – 2x]2
On differentiating w.r.t. x, we get
$$\frac{dy}{dx}=2(x^2-2x)\frac{d}{dx}(x^2-2x)$$
= 2(x2 – 2x)(2x – 2)
= 4x(x – 2)(x – 1)
$$\text{On putting}\space\frac{dy}{dx}=0,\space\text{we get}$$
x = 0, 1 and 2
which divides real line in two disjoint intervals are (– ∞, 0), (0, 1), (1, 2) and (2, ∞).
| Intervals | Sign of y ′(x) | Nature of y(x) |
| (– ∞, 0) | (–)(–)(–) = – ve | Decreasing |
| (0, 1) | (+)(–)(–) = + ve | Increasing |
| (1, 2) | (+)(–)(+) = – ve | Decreasing |
| (2, ∞) | (+)(+)(+) = + ve | Increasing |
Therefore, y(x) is increasing in (0, 1) and (2, ∞).
$$\textbf{9. Prove that y =}\space\frac{\textbf{4} \textbf{sin}\theta}{\textbf{(2+}\textbf{cos}\space\theta)}-\theta\\\space\textbf{is an increasing}\space\textbf{function of}\space\theta\space \textbf{in}\space\\\bigg[\textbf{0},\frac{\pi}{\textbf{2}}\bigg].\\\textbf{Sol.}\space\text{We have,}\space y=\frac{4 \text{sin}\space\theta}{(2 + \text{cos}\space\theta)}-\theta$$
On differentiating w.r.t. θ, we get
$$\frac{dy}{d\theta}=\frac{d}{d\theta}\bigg[\frac{4 \text{sin}\theta}{(2+\text{cos}\space\theta)}-\theta\bigg]\\=\\\frac{(2+\text{cos}\theta)\frac{d}{d\theta}(4 \text{sin}\theta)- \text{4 sin}\space\theta\frac{d}{d\theta}(2+\text{cos}\space\theta)}{(\text{2+ cos}\theta)^2}$$
$$=\frac{4\text{cos}\space\theta(2+\text{cos}\theta)-4 \text{sin}\theta(-\text{sin}\space\theta)}{(2+\text{cos}\space\theta)^2}-1\\=\frac{8\space\text{cos}\theta + 4 \text{cos}^2\theta+ 4\text{sin}^2\theta}{(2+\text{cos}\space\theta)^2}-1\\=\frac{\text{8 cos}\space\theta + 4(\text{cos}^2\theta + 4 \space\text{sin}^2\theta)}{(2+\text{cos}\theta)^2}-1\\=\frac{8\space\text{cos}\space\theta + 4}{(2 + \text{cos}\space\theta)^2}-1\\=\frac{8\space\text{cos}\space\theta - (2 + \text{cos}\space\theta)^2}{(2 + \text{cos}\theta)^2}\\=\frac{8\space \text{cos}\theta + 4 - 4-\text{cos}^2\theta - 4 \text{cos}\space\theta}{(2 + \text{cos}\space\theta)^2}\\=\frac{4 \text{cos}\theta - \text{cos}^2\theta}{(2 + \text{cos}\space\theta)^2}$$
$$=\space\frac{\text{cos}\space\theta(4-\text{cos}\space\theta)}{(2 + \text{cos}\theta)^2}\\\text{In interval}\space\bigg[0,\frac{\pi}{2}\bigg],\space\text{we have cos} \theta \geq 0.$$
Also, 4 > cos θ
⇒ (4 – cos θ) > 0.
∴ cos θ(4 – cos θ) ≥ 0 and also (2 + cos θ)2 > 0
$$\Rarr\space\frac{\text{cos}\space\theta(4-\text{cos}\theta)}{(2 + \text{cos}\space\theta)^2}\geq0\\\Rarr\space\frac{dy}{d\theta}\geq0$$
Hence, given function is increasing in interval
$$\bigg[0,\frac{\pi}{2}\bigg].$$
Note : Since, it is a continuous function,
(i) for increasing function, f ′ ≥ 0.
(ii) for strictly increasing function, f ′ > 0.
10. Prove that the logarithmic function is increasing in (0, ∞).
Sol. Let f(x) = log x
$$\Rarr\space f'(x)=\frac{1}{x}$$
When x ∈ (0, ∞), f ′(x) ≥ 0. Therefore, f(x) is increasing in (0, ∞).
11. Prove that the function f given by f(x) = x2 – x + 1 is neither strictly increasing nor decreasing on (– 1, 1).
Sol. Given, f(x) = x2 – x + 1
⇒ f ′(x) = 2x – 1
On putting f ′(x) = 0, we get x = 1/2
$$x = \frac{1}{2}\space\text{divides the given interval}\\\text{ into two intervals as}\bigg(-1,\frac{1}{2}\bigg)\space\text{and}\space \bigg(\frac{1}{2},1\bigg).$$
| Intervals | Sign of f ′(x) | Nature of f(x) |
| (– 1, 1/2) | – ve | Strictly decreasing |
| (1/2, 1) | + ve | Strictly increasing |
∴ f ′(x) does not have same sign throughout the interval (– 1, 1).
Thus, f(x) is neither increasing nor decreasing strictly in the interval (– 1, 1).
12. Which of the following function are strictly decreasing on
$$\bigg(\textbf{0},\frac{\pi}{\textbf{2}}\bigg).$$
(a) cos x (b) cos 2x (c) cos 3x (d) tan x
Sol. (a) Let f(x) = cos x, then f ′(x) = – sin x.
$$\text{In interval}\space\bigg(0,\frac{\pi}{2}\bigg),\text{f'(x) }\lt 0$$
Therefore, f(x) is strictly decreasing on $$\bigg(0,\frac{\pi}{2}\bigg).$$
(b) Let f(x) = cos 2x ⇒ f ′(x) = – 2 sin 2x.
$$\text{In interval}\space\bigg(0,\frac{\pi}{2}\bigg),\space\text{f'(x)}\lt 0$$
Because sin 2x will either lie in the first or second quadrant which will give a positive value.
Therefore, f(x) is strictly decreasing on
$$\bigg(0,\frac{\pi}{2}\bigg).$$
(c) Let f(x) = cos 3x ⇒ f ′(x) = – 3 sin 3x.
$$\text{In interval}\space\bigg(0,\frac{\pi}{3}\bigg)\space,\text{f(x)}\lt 0$$
Because sin 3x will either lie in the first or second quadrant which will give a positive value.
Therefore, f(x) is strictly decreasing on
$$\bigg(0,\frac{\pi}{3}\bigg).\\\text{When x}\epsilon\space\bigg(\frac{\pi}{3},\frac{\pi}{2}\bigg),\space\text{then f }'(x) \gt 0$$
Because sin 3x will lie in the third quadrant.
Therefore, f(x) is not strictly decreasing on
$$\bigg(0,\frac{\pi}{2}\bigg).$$
(d) Let f(x) = tan x ⇒ f ′(x) = sec2 x. In interval
$$x\epsilon\space\bigg(0,\frac{\pi}{2}\bigg),\space\text{f ′(x) > 0.}\\\text{Therefore, f(x) is not strictly decreasing on}\\\bigg(0,\frac{\pi}{2}\bigg).$$
13. On which of the following intervals is the function f given by f(x) = x100 + sin x – 1 decreasing?
(a) (0, 1)
$$\textbf{(b)}\space\bigg(\frac{\pi}{\textbf{2}},\pi\bigg)\\\textbf{(c)\space}\bigg(\textbf{0},\frac{\pi}{\textbf{2}}\bigg)\\\textbf{(d) None of these}$$
Sol. (d) None of these
Given, f(x) = x100 + sin x – 1
⇒ f ′(x) = 100x99 + cos x
(a) In interval (0, 1), cos x > 0 and 100x99 > 0
∴ f ′(x) = 0
$$\begin{bmatrix}\because\space \text{In}\space\bigg(0,\frac{\pi}{2}\bigg),\space\text{cos x}\gt\text{0 \space i.e.,(0,1.57)}\\\text{cos x}\gt 0\space \therefore (0,1),\space \text{cos x}\gt 0\end{bmatrix}$$
Thus, function f is increasing in interval (0, 1).
$$\text{(b) In interval}\space\bigg(\frac{\pi}{2},\pi\bigg),\space\text{cos x}\lt 0\\\text{and}\space 100x^{99}\gt 0.$$
Also, 100x99 > cos x
$$\therefore\space \text{f'(x)}\gt 0\space\text{in}\space\bigg(\frac{\pi}{2},\pi\bigg)$$
Thus, function f is increasing in interval
$$\bigg(\frac{\pi}{2},\pi\bigg).$$
$$\text{(c)}\space\text{In interval}\space\bigg(0,\frac{\pi}{2}\bigg),\space\text{cos x}\gt 0\\\text{and 100 x}^{99}\gt 0.$$
∴ 100x99 + cos x > 0
$$\Rarr\space\text{f'(x)}\gt 0 \space\text{in}\space\bigg(0,\frac{\pi}{2}\bigg)\\\text{Thus, f is increasing in interval}\space\bigg(0,\frac{\pi}{2}\bigg).$$
Hence, function f is not decreasing in the given intervals.
The correct answer is (b).
14. For what value of a the function f given by f(x) = x2 + ax + 1 is increasing on [1, 2].
Sol. Given, f(x) = x2 + ax + 1
⇒ f ′(x) = 2x + a
Now , function f will be increasing in (1, 2), if
f ′(x) > 0 in (1, 2).
⇒ 2x > – a
⇒ x > – a/2
Therefore, we have to find the least value of a such that x > – a/2, when x ∈ (1, 2).
Then the least value of a for f to be increasing on (1, 2) is given by,
– a/2 = 1
⇒ a = – 2
Hence, the least value of a = – 2.
15. Let I be any interval disjoint from [– 1, 1]. Prove that the function f given by
$$\textbf{f(x)}= \textbf{x +} \frac{\textbf{1}}{\textbf{x}}\space\textbf{is increasing on I.}\\\textbf{Sol.}\space\text{Given,\space f(x)=}x+\frac{1}{x}\\\Rarr\space\text{f'(x)=}1-\frac{1}{x^2}=\frac{x^2-1}{x^2}$$
(Differentiating w.r.t. x)
Since, intervals are in disjoint from [– 1, 1].
Therefore,
if x < – 1, then f ′(x) > 0. If x > 1, then f ′(x) > 0.
∴ f(x) is increasing on interval I disjoint from [– 1, 1].
16. Prove that the function f given by f(x) = log(sin x) is increasing on
$$\bigg(0,\frac{\pi}{2}\bigg)\space\textbf{and strictly decreasing on}\\\bigg(\frac{\pi}{2},\pi\bigg).$$
Sol. Given, f(x) = log(sin x)
$$\Rarr\space\text{f'(x)}=\frac{1}{\text{sin x}}\space\text{(cos x)= cot x}$$
(Differentiate w.r.t. x)
$$\text{In interval}\space\bigg(0,\frac{\pi}{2}\bigg),\text{f}'\text{(x) = cot x > 0.}$$
Because cot x in the Ist quadrant is positive.
$$\therefore\space\text{f is increasing in}\space\bigg(0,\frac{\pi}{2}\bigg).\space\\\text{Also, in interval}\bigg(\frac{\pi}{2},\pi\bigg),\\\space\text{f ′(x) = cot x < 0.}$$
Because cot x in the IInd quadrant is negative.
$$\therefore\space\text{f is decreasing in}\space\bigg(\frac{\pi}{2},\pi\bigg).$$
17. Prove that function f given by f(x) = log|cos x| is decreasing on
$$\bigg(\textbf{0},\frac{\pi}{\textbf{2}}\bigg)\space\textbf{and increasing on}\space\\\bigg(\frac{\textbf{3}\pi}{\textbf{2}},\textbf{2}\pi\bigg).$$
Sol. Given, f(x) = log(cos x)
$$\Rarr\space\text{f'(x)}=\frac{1}{\text{cos x}}.(-\text{sin x})=-\text{tan x}$$
(Differentiate w.r.t. x)
$$\text{In interval}\space\bigg(0,\frac{\pi}{2}\bigg),\space\text{tan x}\gt 0$$
(∵ tan x is in 1st quadrant)
⇒ – tan x < 0 (∵ tan x is in Ist quadrant)
$$\therefore\space \text{f'(x)}\lt 0\space\text{in}\space\bigg(0,\frac{\pi}{2}\bigg)\\\text{Hence, f is decreasing in}\space\bigg(0,\frac{\pi}{2}\bigg).\\\text{Also, in interval}\space\bigg(\frac{\pi}{2},\pi\bigg),\\\text{tan x < 0 ⇒ – tan x > 0}\\(\because \space \text{tan x is in IInd quadrant})\\\therefore\space\text{f'(x)>0}\space\text{in}\space\bigg(\frac{\pi}{2},\pi\bigg)\\\text{Hence, f is increasing in}\space\bigg(\frac{\pi}{2},\pi\bigg).$$
18. Prove that the function given by f(x) = x3 – 3x2 + 3x – 100 is increasing in R.
Sol. Given, f(x) = x3 – 3x2 + 3x – 100
⇒ f ′(x) = 3x2 – 6x + 3 (Differentiate w.r.t. x)
= 3(x2 – 2x + 1) = 3(x – 1)2
For any x ∈ R, (x – 1)2 > 0 since, a perfect square cannot be negative.
Then, f ′(x) > 0. Hence, the given function f is an increasing function on R.
19. The interval in which y = x2 e–x is increasing with respect to x is :
(a) (– ∞, ∞) (b) (– 2, 0) (c) (2, ∞) (d) (0, 2)
Sol. (d) (0, 2)
Given, y = x2 e–x
$$\Rarr\space \frac{dy}{dx}=x^2e^{-x}(-1) + e^{-x}(2x)$$
= xe–x(– x + 2)
= x(2 – x)e–x
$$\text{For increasing function,}\space\frac{dy}{dx}\gt0$$
$$\Rarr\space\text{xe}^{-x}(2-x)\gt0$$
⇒ x > 0 or 2 – x < 0 ⇒ x > 0 or – x > – 2 ⇒ x > 0 or x < – 2
Because e–x cannot be zero, clearly, it is increasing in (0, 2).
