NCERT Solutions for Class 12 Maths Chapter 12 - Linear Programming - Exercise 12.1
Access Exercises of Class 12 Maths Chapter 12 – Linear Programming
Exercise 12.1 Solutions 10 Questions
Exercise 12.2 Solutions 11 Questions
Miscellaneous Exercise On Chapter 12 Solutions 10 Questions
Exercise 12.1
Solve the following Linear Programming Problems graphically :
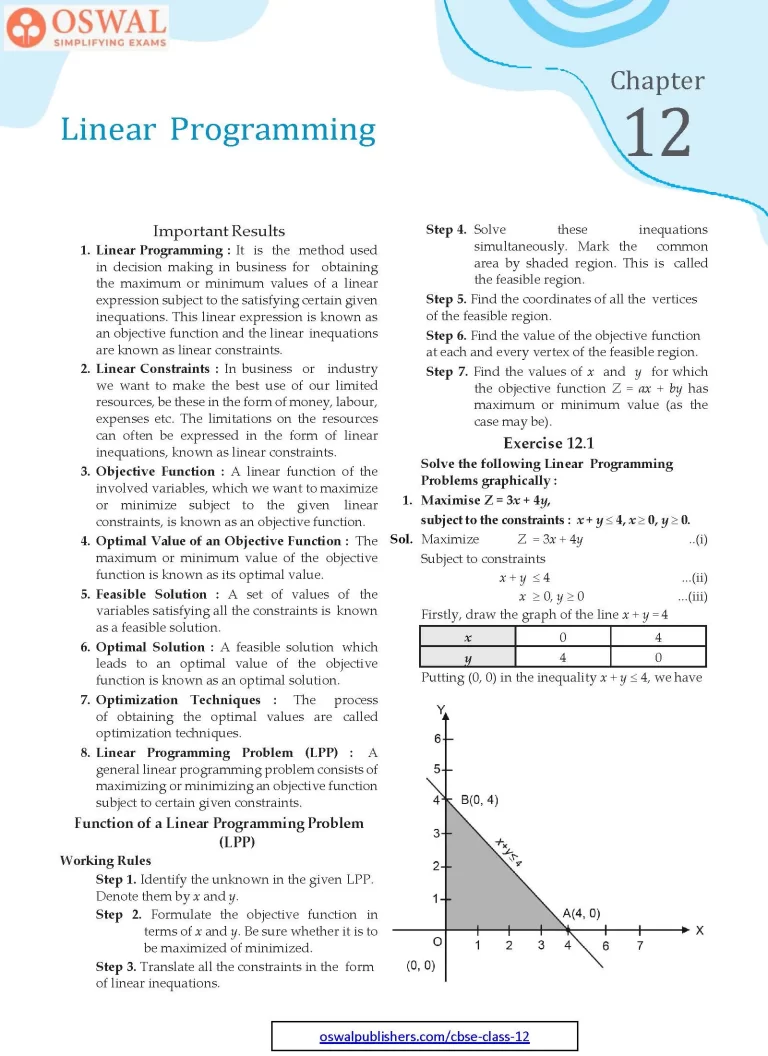
1. Maximise Z = 3x + 4y, subject to the constraints : x + y ≤ 4, x ≥ 0, y ≥ 0.
Sol. Maximize Z = 3x + 4y ..(i)
Subject to constraints
x + y ≤ 4 ...(ii)
x ≥ 0, y ≥ 0 ...(iii)
Firstly, draw the graph of the line x + y = 4
| x | 0 | 4 |
| y | 4 | 0 |
Putting (0, 0) in the inequality x + y ≤ 4, we have
0 + 0 ≤ 4 ⇒ 0 ≤ 4 (which is true)
So, the half plane is towards the origin.
Since, x, y ≥ 0
So, the feasible region lies in the first quadrant.
∴ Feasible region is OABO.
The corner points of the feasible region are O(0, 0), A(4, 0) and B(0, 4). The values of Z at these points are as follows :
| Corner Point | Z = 3x + 4y |
| O(0, 0) | 0 |
| A(4, 0) | 12 |
| B(0, 4) | 16 → Maximum |
Therefore, the maximum value of Z is 16 at the point B(0, 4).
Note : While plotting the graph, please be careful about the inequalities in which directions we have to plot this.
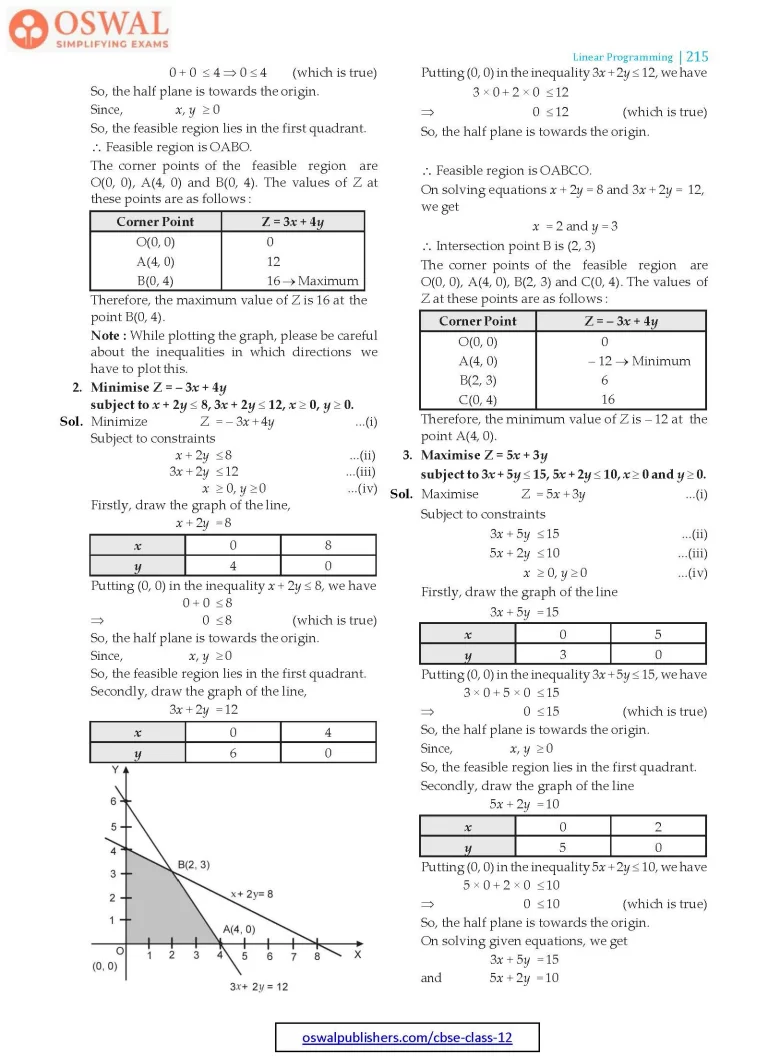
2. Minimise Z = – 3x + 4y subject to x + 2y ≤ 8, 3x + 2y ≤ 12, x ≥ 0, y ≥ 0.
Sol. Minimize Z = – 3x + 4y ...(i)
Subject to constraints
x + 2y ≤ 8 ...(ii)
3x + 2y ≤ 12 ...(iii)
x ≥ 0, y ≥ 0 ...(iv)
Firstly, draw the graph of the line,
x + 2y = 8
| x | 0 | 8 |
| y | 4 | 0 |
Putting (0, 0) in the inequality x + 2y ≤ 8, we have
0 + 0 ≤ 8
⇒ 0 ≤ 8 (which is true)
So, the half plane is towards the origin.
Since, x, y ≥ 0
So, the feasible region lies in the first quadrant.
Secondly, draw the graph of the line,
3x + 2y = 12
| x | 0 | 4 |
| y | 6 | 0 |
Putting (0, 0) in the inequality 3x + 2y ≤ 12, we have
3 × 0 + 2 × 0 ≤ 12
$$\Rarr\space\text{0}\leq 12\space\text{(which is true)}$$
So, the half plane is towards the origin.
∴ Feasible region is OABCO.
On solving equations x + 2y = 8 and 3x + 2y = 12, we get
x = 2 and y = 3
∴ Intersection point B is (2, 3)
The corner points of the feasible region are O(0, 0), A(4, 0), B(2, 3) and C(0, 4). The values of Z at these points are as follows :
| Corner Point | Z = – 3x + 4y |
| O(0, 0) | 0 |
| A(4, 0) | – 12 → Minimum |
| B(2, 3) | 6 |
| C(0, 4) | 16 |
Therefore, the minimum value of Z is – 12 at the point A(4, 0).
3. Maximise Z = 5x + 3y subject to 3x + 5y ≤ 15, 5x + 2y ≤ 10, x ≥ 0 and y ≥ 0.
Sol. Maximise Z = 5x + 3y ...(i)
Subject to constraints
3x + 5y ≤ 15 ...(ii)
5x + 2y ≤ 10 ...(iii)
x ≥ 0, y ≥ 0 ...(iv)
Firstly, draw the graph of the line
3x + 5y = 15
| x | 0 | 5 |
| y | 3 | 0 |
Putting (0, 0) in the inequality 3x + 5y ≤ 15, we have
3 × 0 + 5 × 0 ≤ 15
⇒ 0 ≤ 15 (which is true)
So, the half plane is towards the origin.
Since, x, y ≥ 0
So, the feasible region lies in the first quadrant.
Secondly, draw the graph of the line
5x + 2y = 10
| x | 0 | 2 |
| y | 5 | 0 |
Putting (0, 0) in the inequality 5x + 2y ≤ 10, we have
5 × 0 + 2 × 0 ≤ 10
⇒ 0 ≤ 10 (which is true)
So, the half plane is towards the origin.
On solving given equations, we get
3x + 5y = 15
and 5x + 2y = 10
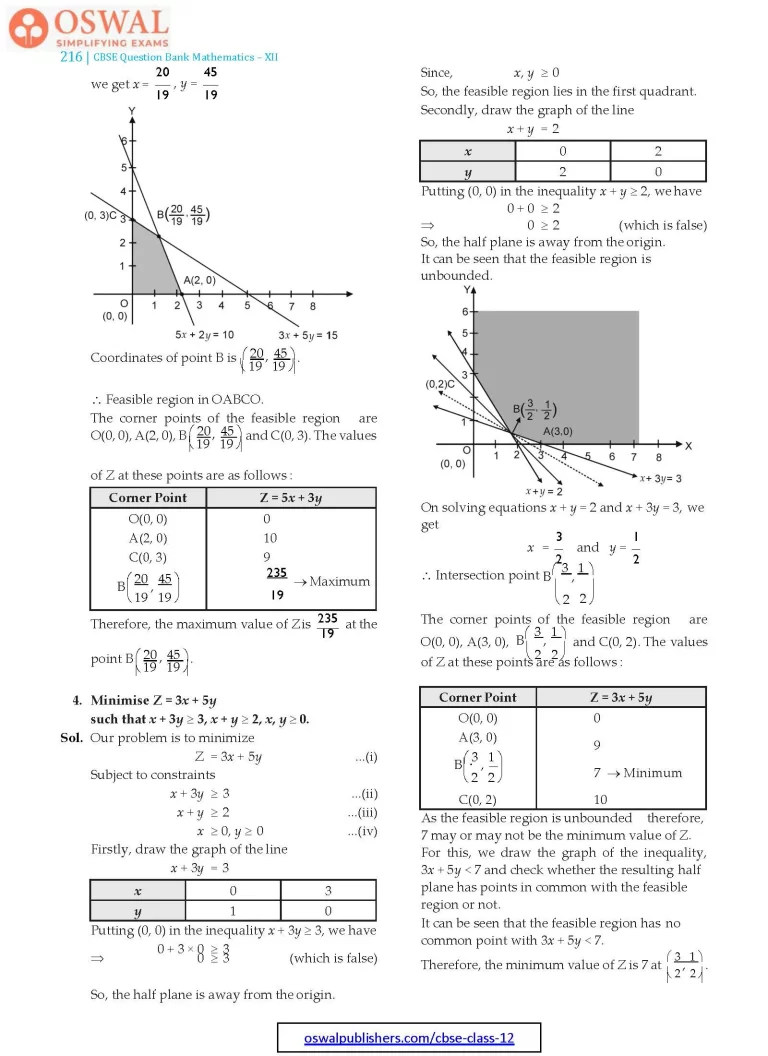
$$\text{we get x = }\frac{20}{19}, y = \frac{45}{19}$$
$$\text{Coordinates of point B is}\\\bigg(\frac{20}{19},\frac{45}{19}\bigg).$$
∴ Feasible region in OABCO.
The corner points of the feasible region are O(0, 0), A(2, 0), $$\text{B}\bigg(\frac{20}{19},\frac{45}{19}\bigg)\space\text{and}\space \text{C(0,3)}.$$
The values of Z at these points are as follows :
| Corner Point | Z = 5x + 3y |
| O(0, 0) | 0 |
| A(2, 0) | 10 |
| C(0, 3) | 9 |
| $$\text{B}\bigg(\frac{20}{19},\frac{45}{19}\bigg)$$ | $$\frac{235}{19}\xrightarrow{}\text{Maximum}$$ |
Therefore, the maximum value of Z is
$$\frac{235}{19}\space\text{at the point}\space\text{B}\bigg(\frac{20}{19},\frac{45}{19}\bigg).$$
4. Minimise Z = 3x + 5y such that x + 3y ≥ 3, x + y ≥ 2, x, y ≥ 0.
Sol. Our problem is to minimize
Z = 3x + 5y ...(i)
Subject to constraints
x + 3y ≥ 3 ...(ii)
x + y ≥ 2 ...(iii)
x ≥ 0, y ≥ 0 ...(iv)
Firstly, draw the graph of the line
x + 3y = 3
| x | 0 | 3 |
| y | 1 | 0 |
Putting (0, 0) in the inequality x + 3y ≥ 3, we have
0 + 3 × 0 ≥ 3
⇒ 0 ≥ 3 (which is false)
So, the half plane is away from the origin.
Since, x, y ≥ 0
So, the feasible region lies in the first quadrant.
Secondly, draw the graph of the line
x + y = 2
| x | 0 | 2 |
| y | 2 | 0 |
Putting (0, 0) in the inequality x + y ≥ 2, we have
0 + 0 ≥ 2
$$\Rarr\space 0\geq2\space\text{(which is false)}$$
So, the half plane is away from the origin.
It can be seen that the feasible region is unbounded.
On solving equations x + y = 2 and x + 3y = 3, we get
$$x = \frac{3}{2}\space\text{and} y =\frac{1}{2}\\\therefore\space\text{Intersection point}\space\text{B}\bigg(\frac{3}{2},\frac{1}{2}\bigg)$$
The corner points of the feasible region are
O(0, 0), A(3, 0),$$\text{B}\bigg(\frac{3}{2},\frac{1}{2}\bigg)\space\text{and}\space\text{C(0,2).}$$
The values of Z at these points are as follows :
| Corner Point | Z = 3x + 5y |
| O(0, 0) | 0 |
| A(3, 0) | 9 |
| $$\text{B}\bigg(\frac{3}{2},\frac{1}{2}\bigg)$$ | $$7\xrightarrow{}\space\text{Minimum}$$ |
| C(0, 2) | 10 |
As the feasible region is unbounded therefore, 7 may or may not be the minimum value of Z.
For this, we draw the graph of the inequality, 3x + 5y < 7 and check whether the resulting half plane has points in common with the feasible region or not.
It can be seen that the feasible region has no common point with 3x + 5y < 7.
Therefore, the minimum value of Z is 7 at
$$\bigg(\frac{3}{2},\frac{1}{2}\bigg).$$
5. Maximise Z = 3x + 2y subject to x + 2y ≤ 10, 3x + y ≤ 15, x, y ≥ 0.
Sol. Maximise, Z = 3x + 2y ...(i)
Subject to constraints
x + 2y ≤ 10 ...(ii)
3x + y ≤ 15 ...(iii)
x ≥ 0, y ≥ 0 ...(iv)
Firstly, draw the graph of the line
x + 2y = 10
| x | 0 | 10 |
| y | 5 | 0 |
Putting (0, 0) in the inequality x + 2y ≤ 10, we have
0 + 2 × 0 ≤ 10
⇒ 0 ≤ 10 (which is true)
So, the half plane is towards the origin.
Since x, y ≥ 0
So, the feasible region lies in the first quadrant.
Secondly, draw the graph of the line
3x + y = 15
| x | 0 | 5 |
| y | 15 | 0 |
Putting (0, 0) in the inequality 3x + y ≤ 15, we have
3 × 0 + 0 ≤ 15
⇒ 0 ≤ 15 (which is true)
So, the half plane is towards the origin.
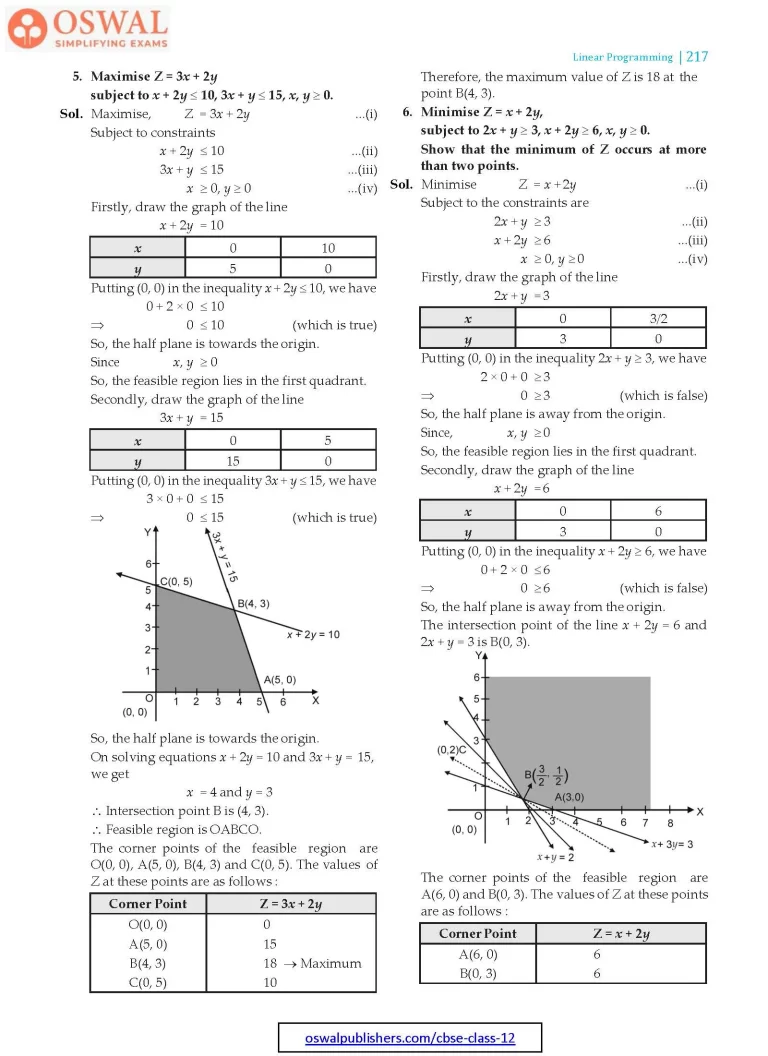
On solving equations x + 2y = 10 and 3x + y = 15, we get
x = 4 and y = 3
∴ Intersection point B is (4, 3).
∴ Feasible region is OABCO.
The corner points of the feasible region are O(0, 0), A(5, 0), B(4, 3) and C(0, 5). The values of Z at these points are as follows :
Therefore, the maximum value of Z is 18 at the point B(4, 3).
6. Minimise Z = x + 2y, subject to 2x + y ≥ 3, x + 2y ≥ 6, x, y ≥ 0.
Show that the minimum of Z occurs at more than two points.
Sol. Minimise Z = x + 2y ...(i)
Subject to the constraints are
2x + y ≥ 3 ...(ii)
x + 2y ≥ 6 ...(iii)
x ≥ 0, y ≥ 0 ...(iv)
Firstly, draw the graph of the line
2x + y = 3
| Corner Point | Z = 3x + 2y |
| O(0, 0) | 0 |
| A(5, 0) | 15 |
| B(4, 3) | $$18\xrightarrow{}\space\text{Maximum}$$ |
| C(0, 5) | 10 |
| x | 0 | $$\frac{3}{2}$$ |
| y | 3 | 0 |
Putting (0, 0) in the inequality 2x + y ≥ 3, we have
2 × 0 + 0 ≥ 3
⇒ 0 ≥ 3 (which is false)
So, the half plane is away from the origin.
Since, x, y ≥ 0
So, the feasible region lies in the first quadrant.
Secondly, draw the graph of the line
x + 2y = 6
| x | 0 | 6 |
| y | 3 | 0 |
Putting (0, 0) in the inequality x + 2y ≥ 6, we have
0 + 2 × 0 ≤ 6
⇒ 0 ≥ 6 (which is false)
So, the half plane is away from the origin.
The intersection point of the line x + 2y = 6 and 2x + y = 3 is B(0, 3).
The corner points of the feasible region are A(6, 0) and B(0, 3). The values of Z at these points are as follows :
| Corner Point | Z = x + 2y |
| A(6, 0) | 6 |
| B(0, 3) | 6 |
It can be seen that the value of Z at points A and B is same. It we take any other point such as (2, 2) on line x + 2y = 6, then Z = 6. Thus, the minimum value of Z occurs for more than 2 points. Therefore, the value of Z is minimum at every point on the line x + 2y = 6.
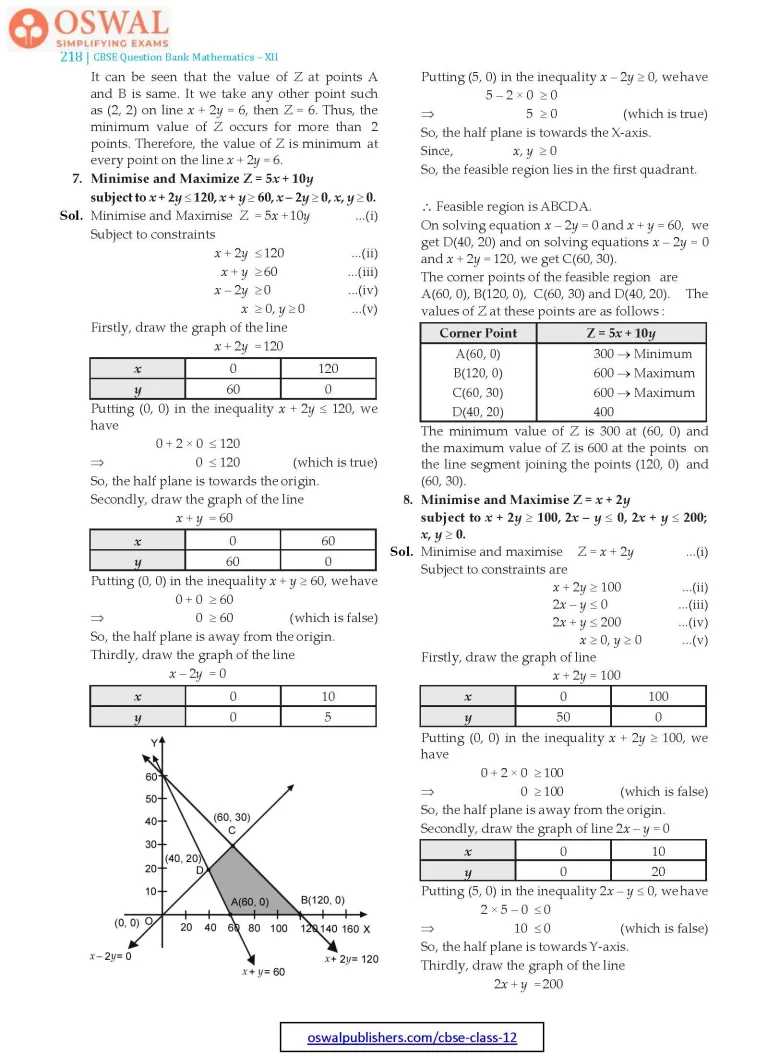
7. Minimise and Maximize Z = 5x + 10y subject to x + 2y ≤ 120, x + y ≥ 60, x – 2y ≥ 0, x, y ≥ 0.
Sol. Minimise and Maximise Z = 5x + 10y ...(i)
Subject to constraints
x + 2y ≤ 120 ...(ii)
x + y ≥ 60 ...(iii)
x – 2y ≥ 0 ...(iv)
x ≥ 0, y ≥ 0 ...(v)
Firstly, draw the graph of the line
x + 2y = 120
| x | 0 | 120 |
| y | 60 | 0 |
Putting (0, 0) in the inequality x + 2y ≤ 120, we have
0 + 2 × 0 ≤ 120
⇒ 0 ≤ 120 (which is true)
So, the half plane is towards the origin.
Secondly, draw the graph of the line
x + y = 60
| x | 0 | 60 |
| y | 60 | 0 |
Putting (0, 0) in the inequality x + y ≥ 60, we have 0 + 0 ≥ 60
⇒ 0 ≥ 60 (which is false)
So, the half plane is away from the origin.
Thirdly, draw the graph of the line
x – 2y = 0
| x | 0 | 10 |
| y | 0 | 5 |
Putting (5, 0) in the inequality x – 2y ≥ 0, we have
5 – 2 × 0 ≥ 0
⇒ 5 ≥ 0 (which is true)
So, the half plane is towards the X-axis.
Since, x, y ≥ 0
So, the feasible region lies in the first quadrant.
∴ Feasible region is ABCDA.
On solving equation x – 2y = 0 and x + y = 60, we get D(40, 20) and on solving equations x – 2y = 0 and x + 2y = 120, we get C(60, 30).
The corner points of the feasible region are A(60, 0), B(120, 0), C(60, 30) and D(40, 20). The values of Z at these points are as follows :
| Corner Point | Z = 5x + 10y |
| A(60, 0) | $$300\xrightarrow{}\space\text{Minimum}$$ |
| B(120, 0) | $$600\xrightarrow{}\space\text{Maximum}$$ |
| C(60, 30) | $$600\xrightarrow{}\space\text{Maximum}$$ |
| D(40, 20) | 400 |
The minimum value of Z is 300 at (60, 0) and the maximum value of Z is 600 at the points on the line segment joining the points (120, 0) and (60, 30).
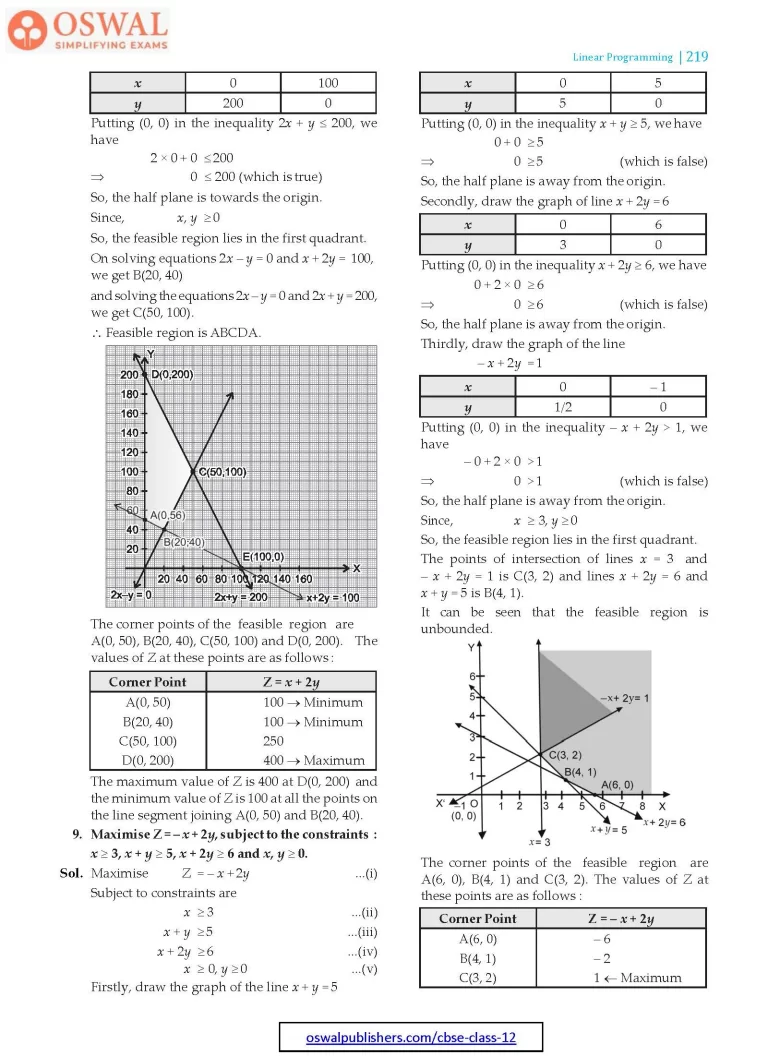
8. Minimise and Maximise Z = x + 2y subject to x + 2y ≥ 100, 2x – y ≤ 0, 2x + y ≤ 200; x, y ≥ 0.
Sol. Minimise and maximise Z = x + 2y ...(i)
Subject to constraints are
x + 2y ≥ 100 ...(ii)
2x – y ≤ 0 ...(iii)
2x + y ≤ 200 ...(iv)
x ≥ 0, y ≥ 0 ...(v)
Firstly, draw the graph of line
x + 2y = 100
| x | 0 | 100 |
| y | 50 | 0 |
Putting (0, 0) in the inequality x + 2y ≥ 100, we have
0 + 2 × 0 ≥ 100
⇒ 0 ≥ 100 (which is false)
So, the half plane is away from the origin.
Secondly, draw the graph of line 2x – y = 0
| x | 0 | 10 |
| y | 0 | 20 |
Putting (5, 0) in the inequality 2x – y ≤ 0, we have
2 × 5 – 0 ≤ 0
⇒ 10 ≤ 0 (which is false)
So, the half plane is towards Y-axis.
Thirdly, draw the graph of the line
2x + y = 200
| x | 0 | 100 |
| y | 200 | 0 |
Putting (0, 0) in the inequality 2x + y ≤ 200, we have
2 × 0 + 0 ≤ 200
⇒ 0 ≤ 200 (which is true)
So, the half plane is towards the origin.
Since, x, y ≥ 0
So, the feasible region lies in the first quadrant.
On solving equations 2x – y = 0 and x + 2y = 100, we get B(20, 40)
and solving the equations 2x – y = 0 and 2x + y = 200, we get C(50, 100).
∴ Feasible region is ABCDA.
The corner points of the feasible region are A(0, 50), B(20, 40), C(50, 100) and D(0, 200). The values of Z at these points are as follows :
| Corner Point | Z = x + 2y |
| A(0, 50) | $$\text{100}\xrightarrow{}\text{Minimum}$$ |
| B(20, 40) | $$\text{100}\xrightarrow{}\text{Minimum}$$ |
| C(50, 100) | 250 |
| D(0, 200) | $$\text{400}\space\xrightarrow{}\space\text{Maximum}$$ |
The maximum value of Z is 400 at D(0, 200) and the minimum value of Z is 100 at all the points on the line segment joining A(0, 50) and B(20, 40).
9. Maximise Z = – x + 2y, subject to the constraints : x ≥ 3, x + y ≥ 5, x + 2y ≥ 6 and x, y ≥ 0.
Sol. Maximise Z = – x + 2y ...(i)
Subject to constraints are
x ≥ 3 ...(ii)
x + y ≥ 5 ...(iii)
x + 2y ≥ 6 ...(iv)
x ≥ 0, y ≥ 0 ...(v)
Firstly, draw the graph of the line x + y = 5
| x | 0 | 5 |
| y | 5 | 0 |
Putting (0, 0) in the inequality x + y ≥ 5, we have
0 + 0 ≥ 5
⇒ 0 ≥ 5 (which is false)
So, the half plane is away from the origin.
Secondly, draw the graph of line x + 2y = 6
| x | 0 | 6 |
| y | 3 | 0 |
Putting (0, 0) in the inequality x + 2y ≥ 6, we have
0 + 2 × 0 ≥ 6
⇒ 0 ≥ 6 (which is false)
So, the half plane is away from the origin.
Thirdly, draw the graph of the line
– x + 2y = 1
| x | 0 | -1 |
| y | $$\frac{1}{2}$$ | 0 |
Putting (0, 0) in the inequality – x + 2y > 1, we have
– 0 + 2 × 0 > 1
⇒ 0 > 1 (which is false)
So, the half plane is away from the origin.
Since, x ≥ 3, y ≥ 0
So, the feasible region lies in the first quadrant.
The points of intersection of lines x = 3 and – x + 2y = 1 is C(3, 2) and lines x + 2y = 6 and x + y = 5 is B(4, 1).
It can be seen that the feasible region is unbounded.
The corner points of the feasible region are A(6, 0), B(4, 1) and C(3, 2). The values of Z at these points are as follows :
| Corner Point | Z = – x + 2y |
| A(6, 0) | – 6 |
| B(4, 1) | – 2 |
| C(3, 2) | $$1\xleftarrow{}\space\text{Maximum}$$ |
As the feasible region is unbounded therefore, Z = 1 may or may not be the maximum value. For this, we graph the inequality, – x + 2y > 1 and check whether the resulting half plane has points in common with the feasible region or not.
The resulting feasible region has points in common with the feasible region. Therefore, Z = 1 is not the maximum value.
Hence, Z has no maximum value.
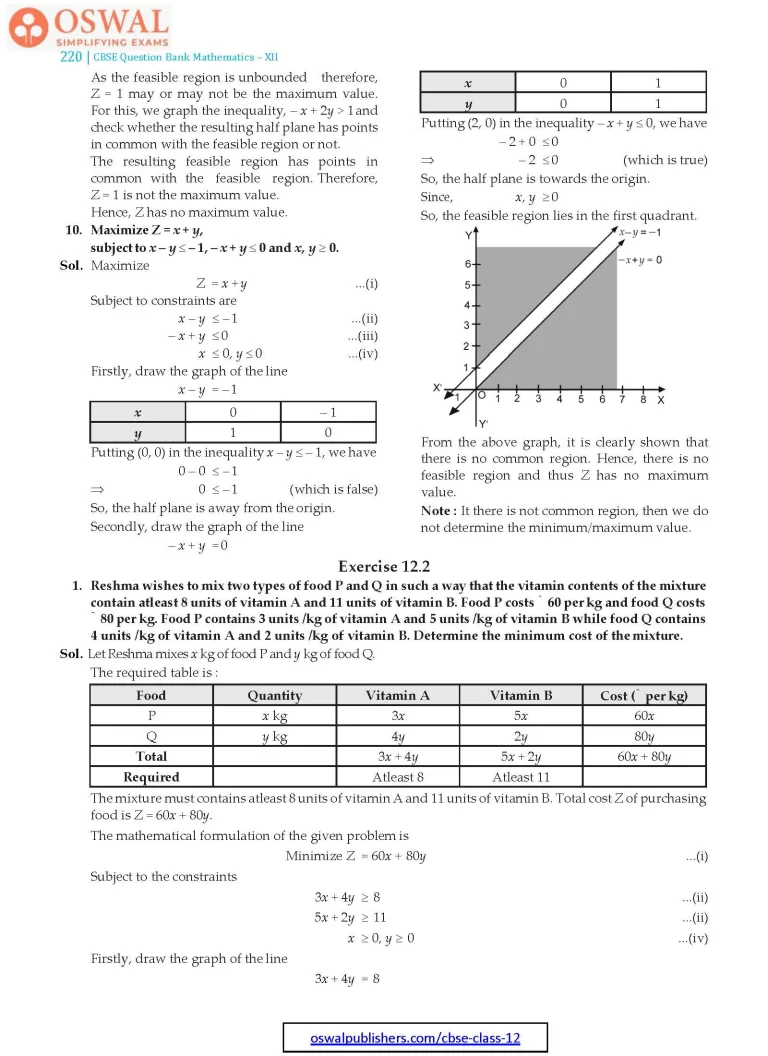
10. Maximize Z = x + y, subject to x – y ≤ – 1, – x + y ≤ 0 and x, y ≥ 0.
Sol. Maximize
Z = x + y ...(i)
Subject to constraints are
x – y ≤ – 1 ...(ii)
– x + y ≤ 0 ...(iii)
x ≤ 0, y ≤ 0 ...(iv)
Firstly, draw the graph of the line
x – y = – 1
Putting (0, 0) in the inequality x – y ≤ – 1, we have
0 – 0 ≤ – 1
⇒ 0 ≤ – 1 (which is false)
So, the half plane is away from the origin.
Secondly, draw the graph of the line
– x + y = 0
| x | 0 | 1 |
| y | 0 | 1 |
| x | 0 | -1 |
| y | 1 | 0 |
Putting (2, 0) in the inequality – x + y ≤ 0, we have
– 2 + 0 ≤ 0
⇒ – 2 ≤ 0 (which is true)
So, the half plane is towards the origin.
Since, x, y ≥ 0
So, the feasible region lies in the first quadrant.
From the above graph, it is clearly shown that there is no common region. Hence, there is no feasible region and thus Z has no maximum value.
Note : It there is not common region, then we do not determine the minimum/maximum value.
Share page on
