NCERT Solutions for Class 12 Maths Chapter 4 Determinants - Exercise 4.2
Access Exercises of Class 12 Maths Chapter 4 –Determinants
Exercise 4.1 Solutions: 8 Questions (2 Long, 5 Short Answers, 1 MCQ)
Exercise 4.2 Solutions: 16 Questions(7 Long, 7 Short, 2 MCQs)
Exercise 4.3 Solutions: 5 Questions ( 4 Short Answers, 1 MCQ)
Exercise 4.4 Solutions: 5 Questions (4 Long, 1 MCQ)
Exercise 4.5 Solutions: 18 Questions (11 Long, 5 Short, 2 MCQs)
Exercise 4.6 Solutions: 16 Questions (13 Long, 3 Short)
Miscellaneous Exercise Solutions: 19 Questions (15 Long, 1 Short, 3 MCQs)
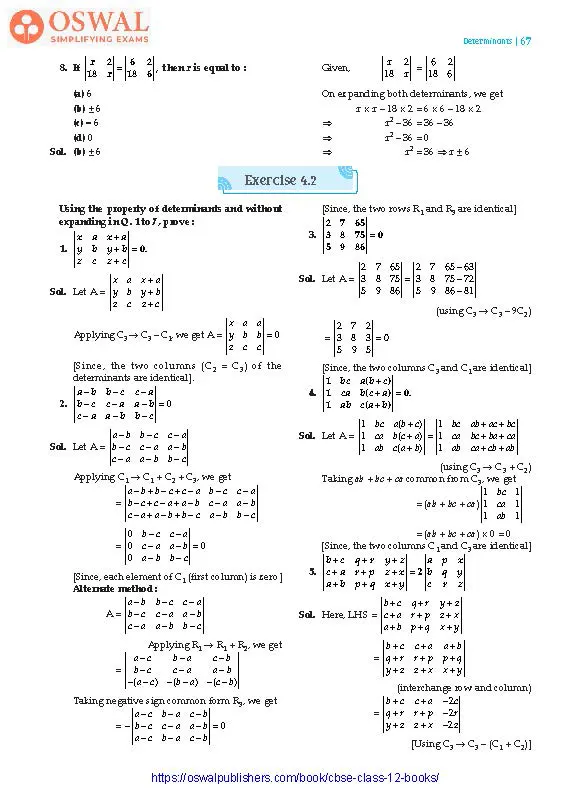
Exercise 4.2
Using the property of determinants and without expanding in Q. 1 to 7, prove :
$$\textbf{1.\space}\begin{vmatrix}\textbf{x} &\textbf{a} &\textbf{x + a}\\\textbf{y} &\textbf{b} &\textbf{y + b}\\\textbf{z} &\textbf{c} &\textbf{z+c}\end{vmatrix} \textbf{= 0.}\\\textbf{Sol.}\space\text{let A} = \begin{vmatrix}x &a &x+a\\y &b &y+b\\z &c &z+c\end{vmatrix}\\\text{Applying C}_{3}\xrightarrow{}\text{C}_{3} - \text{C}_{1},\\\text{we get A} = \begin{vmatrix}x &a &a\\y &b &b\\z &c &c\end{vmatrix} =0$$
[Since, the two columns (C2 = C3) of the determinants are identical].
$$\textbf{2.}\begin{vmatrix}\textbf{a-b} &\textbf{b-c} &\textbf{c-a}\\\textbf{b-c} &\textbf{c-a} &\textbf{a-b}\\\textbf{c-a} &\textbf{a-b} &\textbf{b-c}\end{vmatrix} \textbf{= 0}\\\textbf{Sol.\space}\text{Let A =}\begin{vmatrix}a-b &b-c &c-a\\b-c &c-a &a-b\\c-a &a-b &b-c\end{vmatrix}\\\text{Applying}\space\text{C}_{1}\xrightarrow{}\text{C}_{1} + \text{C}_{2} +\text{C}_{3},\\\text{we get}\\=\begin{vmatrix}a-b+b-c+c-a &b-c &c-a\\b-c+c-a+a-b &c-a &a-b\\c-a+a-b+b+b &a-b &b-c\end{vmatrix}\\=\begin{vmatrix}0 &b-c &c-a\\0 &c-a &a-b\\0 &a-b &b-c\end{vmatrix} = 0$$
[Since, each element of C1 (first column) is zero]
Alternate method :
$$\text{A = }\begin{vmatrix}a-b &b-c &c-a\\b-c &c-a &a-b\\c-a &a-b &b-c\end{vmatrix}\\\text{Applying R}_{1}\xrightarrow{}\text{R}_{1} + \text{R}_{2},\\\text{we get}\\=\begin{vmatrix}a-c &b-a &c-b\\b-c &c-a &a-b\\-(a-c) &-(b-a) &-(c-b)\end{vmatrix}$$
Taking negative sign common form R3, we get
$$= -\begin{vmatrix}a-c &b-a &c-b\\b-c &c-a &a-b\\a-c &b-a &c-b\end{vmatrix} =\text{0}$$
[Since, the two rows R1 and R3 are identical]
$$\textbf{3.\space}\begin{vmatrix}\textbf{2} &\textbf{7} &\textbf{65}\\\textbf{3} &\textbf{8} &\textbf{75}\\\textbf{5} &\textbf{9} &\textbf{86}\end{vmatrix} \textbf{= 0}\\\textbf{Sol.\space}\text{Let A} = \begin{vmatrix}2 &7 &65\\3 &8 &75\\5 &9 &86\end{vmatrix}\\=\begin{vmatrix}2 &7 &65-63\\3 &8 &75-72\\5 &9 &86-81\end{vmatrix}$$
$$\text{(using C}_{3}\xrightarrow{}\text{C}_{3} -9\text{C}_{2})\\=\begin{vmatrix}2 &7 &2\\3 &8 &3\\5 &9 &5\end{vmatrix} =0$$
[Since, the two columns C3 and C1 are identical]
$$\textbf{4.\space}\begin{vmatrix}\textbf{1} &\textbf{bc} &\textbf{a(b+c)}\\\textbf{1} &\textbf{ca} &\textbf{b(c+a)}\\\textbf{1} &\textbf{ab} &\textbf{c(a+b)}\end{vmatrix} \textbf{= 0.}\\\textbf{Sol.\space}\text{Let A} = \begin{vmatrix}1 &bc &a(b+c)\\1 &ca &b(c+a)\\1 &ab &c(a+b)\end{vmatrix}\\=\begin{vmatrix}1 &bc &ab + ac + bc\\1 &ca &bc+ba+ca\\1 &ab &ca+cb+ab\end{vmatrix}\\\text{(using\space C}_{3}\xrightarrow{}\text{C}_{3} + \text{C}_{2})$$
Taking ab + bc + ca common from C3, we get
$$= (ab + bc + ca)\begin{vmatrix}1 &bc &1\\1 &ca &1\\1 &ab &1\end{vmatrix} $$
= (ab + bc + ca) × 0 = 0
[Since, the two columns C1 and C3 are identical]
$$\textbf{5.\space}\begin{vmatrix}\textbf{b+c} &\textbf{q+r} &\textbf{y+z}\\\textbf{c+a} &\textbf{r+p} &\textbf{z+x} \\\textbf{a+b} &\textbf{p+q} &\textbf{x+y}\end{vmatrix}\\= \textbf{2}\begin{vmatrix}\textbf{a} &\textbf{p} &\textbf{x}\\\textbf{b} &\textbf {q} &\textbf{y}\\\textbf{c} &\textbf{r} &\textbf{z}\end{vmatrix}\\\textbf{Sol.\space}\text{Here, LHS = }\begin{vmatrix}b+c &q+r &y+z\\c+a &r+p &z+x\\a+b &p+q &x+y\end{vmatrix}\\=\begin{vmatrix}b+c &c+a &a+b\\q+r &r+p &p+q\\y+z &z+x &x+y\end{vmatrix}$$
(interchange row and column)
$$= \begin{vmatrix}b+c &c+a &-2c\\q+r &r+p &-2r\\y+z &z+x &x+y\end{vmatrix}\\\lbrack\text{Using C}_{3}\xrightarrow{}\text{C}_{3}-(\text{C}_{1} + \text{C}_{2})\rbrack\\=-2\begin{vmatrix}b+c &c+a &c\\q+r &r+p &r\\y+z &z+x &z\end{vmatrix}$$
(taking ‘–2’ common from C3)
$$= - 2\begin{vmatrix}b &a &c\\q &p &r\\y &x &z\end{vmatrix}$$
(Applying C1 → C1 – C3 and C2 → C2 – C3)
$$= 2\begin{vmatrix}a &b &c\\p &q &r\\x &y &z\end{vmatrix}\\\qquad= 2\begin{vmatrix}a &p &x\\b &q &y\\c &r &z\end{vmatrix}= \text{RHS}$$
(interchange column and row)
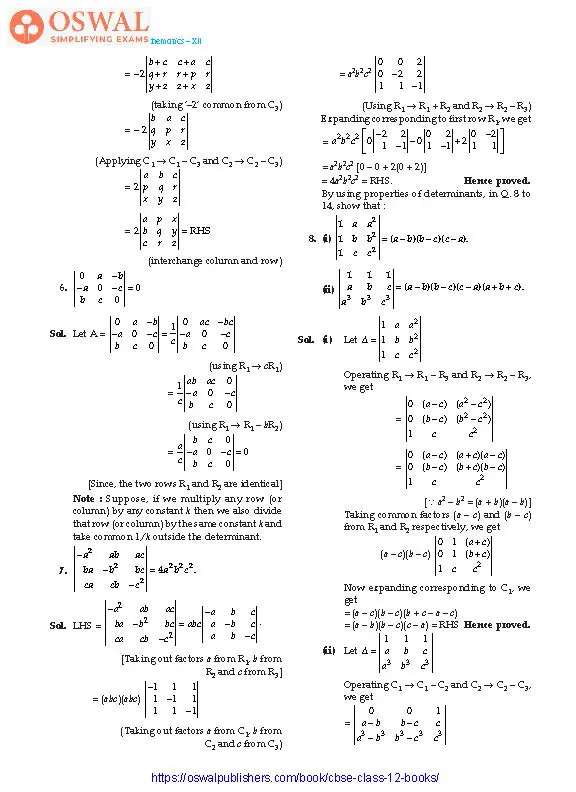
$$\textbf{6.\space}\begin{vmatrix}\textbf{0} &\textbf{a} &\textbf{\normalsize-b}\\\textbf{\normalsize-a} &\textbf{0} &\textbf{\normalsize-c}\\\textbf{b} &\textbf{c} &\textbf{0}\end{vmatrix} \textbf{= 0}\\\textbf{Sol.\space}\text{Let A} = \begin{vmatrix}0 &a &\normalsize-b\\\normalsize-a &0 &\normalsize-c\\b &c &0\end{vmatrix}\\=\frac{1}{c}\begin{vmatrix}0 &ac &\normalsize-bc\\\normalsize-a &0 &\normalsize-c\\b &c &0\end{vmatrix}$$
$$(\text{using}\space\text{R}_{1}\xrightarrow{}c\text{R}_{1})\\=\frac{1}{c}\begin{vmatrix}ab &ac &0\\\normalsize-a &0 &\normalsize-c\\b &c &0\end{vmatrix}\\\text{(using R}_{1}\xrightarrow{}\text{R}_{1}-b\text{R}_{2})\\=\frac{a}{c}\begin{vmatrix}b &c &0\\\normalsize-a &0 &\normalsize-c\\ b &c &0\end{vmatrix} = 0$$
[Since, the two rows R1 and R2 are identical]
Note : Suppose, if we multiply any row (or column) by any constant k then we also divide that row (or column) by the same constant k and take common 1/k outside the determinant.
$$\textbf{7.\space}\begin{vmatrix}-\textbf{a}^{\textbf{2}} &\textbf{ab} &\textbf{ac}\\\textbf{ba} &\textbf{-b}^{2} &\textbf{bc}\\\textbf{ca} &\textbf{cb} &\textbf{-c}^{2}\end{vmatrix}\\\textbf{= 4a}^{\textbf{2}}\textbf{b}^{\textbf{2}}\textbf{c}^{\textbf{2}}.\\\textbf{Sol.\space}\text{LHS = } \begin{vmatrix}-a^{2} &ab &ac\\ba &-b^{2} &bc\\\text{ca} &\text{cb} &\text{-c}^{2}\end{vmatrix}\\=\text{abc}\begin{vmatrix}-a &b &c\\a &-b &c\\a &b &-c\end{vmatrix}.$$
[Taking out factors a from R1, b from R2 and c from R3]
$$=\space \text{(abc)(abc)}\begin{vmatrix}\normalsize-1 &1 &1\\1 &\normalsize-1 &1\\1 &1 &\normalsize-1\end{vmatrix}$$
(Taking out factors a from C1, b from C2 and c from C3)
$$= a^{2}b^{2}c^{2}\begin{vmatrix}0 &0 &2\\0 &\normalsize-2 &2\\1 &1 &\normalsize-1\end{vmatrix}\\(\text{Using R}_{1}\xrightarrow{}R_{1} + R_{2}\\\text{and}\space \text{R}_{2}\xrightarrow{}\text{R}_{2} - \text{R}_{3})$$
Expanding corresponding to first row R1, we get
$$= a^{2}b^{2}c^{2}\begin{bmatrix}0\begin{vmatrix}-2 &2\\ 1 &\normalsize-1\end{vmatrix}-0\begin{vmatrix}0 &2\\1 &\normalsize-1\end{vmatrix}\\+2\begin{vmatrix}0 &\normalsize-2\\1 &1\end{vmatrix}\end{bmatrix}$$
= a2b2c2 [0 – 0 + 2(0 + 2)]
= 4a2b2c2 = RHS. Hence proved.
By using properties of determinants, in Q. 8 to 14, show that :
$$\textbf{8.\space (i)\space}\begin{vmatrix}\textbf{1} &\textbf{a} &\textbf{a}^{\textbf{2}}\\\textbf{1} &\textbf{b} &\textbf{b}^{\textbf{2}}\\\textbf{1} &\textbf{c} &\textbf{c}^{\textbf{2}}\end{vmatrix}\\\textbf{=(a-b)}\textbf{(b-c)}\textbf{(c-a)}\textbf{.}\\\textbf{(ii)\space}\begin{vmatrix}\textbf{1} &\textbf{1} &\textbf{1}\\\textbf{a} &\textbf{b} &\textbf{c}\\\textbf{a}^{\textbf{3}} &\textbf{b}^{\textbf{3}} &\textbf{c}^{\textbf{3}}\end{vmatrix}\\\textbf{=}\space\textbf{(a-b)} \textbf{(b-c)}\textbf{(c-a)}\textbf{(a + b + c)}\textbf{.}\\\textbf{Sol.\space}\text{(i)\space Let}\space\Delta = \begin{vmatrix}1 &a &a^{2}\\1 &b &b^{2}\\1 &c &c^{2}\end{vmatrix}\\\text{Operating R}_{1}\xrightarrow{}\text{R}_{1}-\text{R}_{3}\\\text{and}\space\text{R}_{2}\xrightarrow{}\text{R}_{2} - \text{R}_{3},\\\text{we get}$$
$$= \begin{vmatrix}0 &(a-c) &(a^{2}-c^{2})\\0 &(b-c) &(b^{2}-c^{2})\\1 &c &c^{2}\end{vmatrix}\\=\begin{vmatrix}0 &(a-c) &(a+c)(a-c)\\0 &(b-c) &(b+c)(b-c)\\1 &c &c^{2}\end{vmatrix}\\\lbrack\because\space a^{2} - b^{2} = (a+b)(a-b)\rbrack$$
Taking common factors (a – c) and (b – c) from R1 and R2 respectively, we get
$$(a-c)(b-c)\begin{vmatrix}0 &1 &(a+c)\\0 &1 &(b+c)\\1 &c &c^{2}\end{vmatrix}$$
Now expanding corresponding to C1, we get
= (a – c)(b – c)(b + c – a – c)
= (a – b)(b – c)(c – a) = RHS Hence proved.
$$\textbf{(ii)\space}\text{Let}\space\Delta = \begin{vmatrix}1 &1 &1\\a &b &c\\a^{3} &b^{3} &c^{3}\end{vmatrix}\\\text{Operating}\space\text{C}_{1}\xrightarrow{}\text{C}_{1}\text{C}_{2}\\\text{and}\space\text{C}_{2}\xrightarrow{}\text{C}_{2} - \text{C}_{3},\\\text{we get}\\=\begin{vmatrix}0 &0 &1\\a-b &b-c &c\\ a^{3} -b^{3} &b^{3} -c^{3} &c^{3}\end{vmatrix}\\=\\\begin{vmatrix}0 &0 &1\\(a-b) &(b-c) &c\\(a-b)(a^{2} +ab+b^{2}) &(b-c)(b^{2} + bc+c^{2}) &c^{3}\end{vmatrix}$$
[∵ x3 – y3 = (x – y)(x2 + xy + y2)]
Taking common (a – b) from C1 and (b – c) from C2, we get
$$= (a-b)(b-c)\\\begin{vmatrix}0 &0 &1\\1 &1 &c\\ a^{2}+ab+b^{2} &b^{2}+bc+c^{2} &c^{3}\end{vmatrix}$$
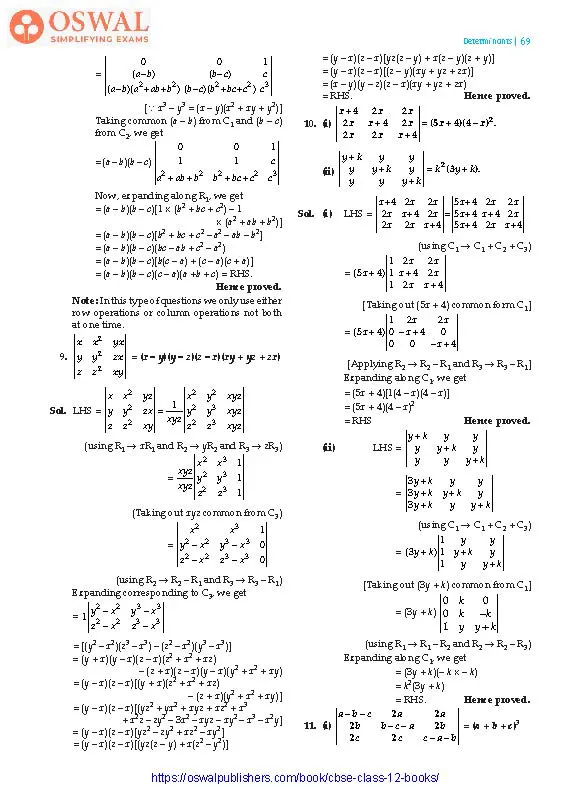
Now, expanding along R1, we get
= (a – b)(b – c)[1 × (b2 + bc + c2) – 1 × (a2 + ab + b2)]
= (a – b)(b – c)[b2 + bc + c2 – a2 – ab – b2]
= (a – b)(b – c)(bc – ab + c2 – a2)
= (a – b)(b – c)[b(c – a) + (c – a)(c + a)]
= (a – b)(b – c)(c – a)(a +b + c) = RHS.
Hence proved.
Note : In this type of questions we only use either row operations or column operations not both at one time.
$$\textbf{9.\space}\begin{vmatrix}\textbf{x} &\textbf{x}^{\textbf{2}} &\textbf{yx}\\\textbf{y} &\textbf{y}^{\textbf{2}} &\textbf{zx}\\\textbf{z} &\textbf{z}^{\textbf{2}} &\textbf{xy}\end{vmatrix}\\\textbf{=}\textbf{(x-y)(y-z)(z-x)(xy+yz+zx)}\\\textbf{Sol.\space}\text{LHS}\begin{vmatrix} x &x^{2} &yz\\y &y^{2} &zx\\z &z^{2} &xy\end{vmatrix}\\=\frac{1}{xyz}\begin{vmatrix}x^{2} &y^{2} &xyz\\y^{2} &y^{3} &xyz\\z^{2} &z^{3} &xyz\end{vmatrix}\\\text{(using R}_{1}\xrightarrow{}x\text{R}_{1}\\\text{and}\space \text{R}_{2}\xrightarrow{}y\text{R}_{2}\\\text{and}\space \text{R}_{3}\xrightarrow{}zR_{3})$$
$$=\frac{xyz}{xyz}\begin{vmatrix}x^{2} &x^{3} &1\\y^{2} &y^{3} &1\\z^{2} &z^{3} &1\end{vmatrix}$$
(Taking out xyz common from C3)
$$= \begin{vmatrix}x^{2} &x^{3} &1\\y^{2}-x^{2} &y^{3}-x^{3} &0\\z^{2}-x^{2} &z^{3}-x^{3} &0\end{vmatrix} $$
(using R2 → R2 – R1 and R3 → R3 – R1)
Expanding corresponding to C3, we get
$$= 1 \begin{vmatrix}y^{2}-x^{2} &y^{3} - x^{3}\\z^{2}-x^{2} &z^{3}-x^{3}\end{vmatrix}$$
= [(y2 – x2)(z3 – x3) – (z2 – x2)(y3 – x3)]
= (y + x)(y – x)(z – x)(z2 + x2 + xz) – (z + x)(z – x)(y – x)(y2 + x2 + xy)
= (y – x)(z – x)[(y + x)(z2 + x2 + xz) – (z + x)(y2 + x2 + xy)]
= (y – x)(z – x)[(yz2 + yx2 + xyz + xz2 + x3 + x2z – zy2 – 3x2 – xyz – xy2 – x3 – x2y]
= (y – x)(z – x)[yz2 – zy2 + xz2 – xy2]
= (y – x)(z – x)[(yz(z – y) + x(z2 – y2)]
= (y – x)(z – x)[yz(z – y) + x(z – y)(z + y)]
= (y – x)(z – x)[(z – y)(xy + yz + zx)]
= (x – y)(y – z)(z – x)(xy + yz + zx)
= RHS. Hence proved.
$$\textbf{10. \space (i)}\begin{vmatrix}\textbf{x+4} &\textbf{2x} &\textbf{2x}\\ \textbf{2x} &\textbf{x+4} &\textbf{2x}\\\textbf{2x} &\textbf{2x} &\textbf{x+4}\end{vmatrix}\\\textbf{=}\textbf{(5x+4) (4-x)}^{2}.\\\textbf{(ii)\space}\begin{vmatrix}\textbf{y+k} &\textbf{y} &\textbf{y}\\\textbf{y} &\textbf{y+k} &\textbf{y}\\\textbf{y}&\textbf{y} &\textbf{y+k}\end{vmatrix}\\\textbf{=}\space\textbf{k}^{\textbf{2}}\textbf{(3y+k)}\textbf{.}$$
Sol. (i) LHS =
$$\begin{vmatrix}x+4 &2x &2x\\2x &x+4 &2x\\2x &2x &x+4\end{vmatrix}\\=\begin{vmatrix}5x+4 &2x &2x\\5x+4 &x+4 &2x\\5x+4 &2x &x+4\end{vmatrix}\\(\text{using}\space \text{C}_{1}\xrightarrow{}\text{C}_{1} + \text{C}_{2} + \text{C}_{3})\\=(5x+4)\begin{vmatrix}1 &2x &2x\\1 &x+4 &2x\\ 1 &2x &x+4\end{vmatrix}\\\lbrack\text{Taking out (5x+4)}\\\text{common form C}_{1}\rbrack\\=(5x+4)\begin{vmatrix} 1 &2x &2x\\ 1 &\normalsize-x+4 &2x\\1 &2x &\normalsize-x+4\end{vmatrix}$$
[Applying R2 → R2 – R1 and R3 → R3 – R1]
Expanding along C1, we get
= (5x + 4)[1(4 – x)(4 – x)]
= (5x + 4)(4 – x)2
= RHS Hence proved.
$$\textbf{(ii)}\space \text{LHS}=\begin{vmatrix}3y+k &y &y\\3y+k &y+k &y\\3y+k &y &y+k\end{vmatrix}\\=\begin{vmatrix}3y+k &y &y\\3y+k &y+k &y\\3y+k &y &y+k\end{vmatrix}\\\text{(using C}_{1}\xrightarrow{}\text{C}_{1} + \text{C}_{2} + \text{C}_{3})\\=(3y+k)\begin{vmatrix}1 &y &y\\1 &y+k &y\\1 &y &y+k\end{vmatrix}\\\lbrack \text{Taking out (3y + k) common from }C_{1}\rbrack\\ = (3y+k)\begin{vmatrix} 0 &k &0\\0 &k &-k\\1 &y &y+k\end{vmatrix}$$
(using R1 → R1 – R2 and R2 → R2 – R3)
Expanding along C1, we get
= (3y + k)(– k × – k)
= k2(3y + k)
= RHS. Hence proved.
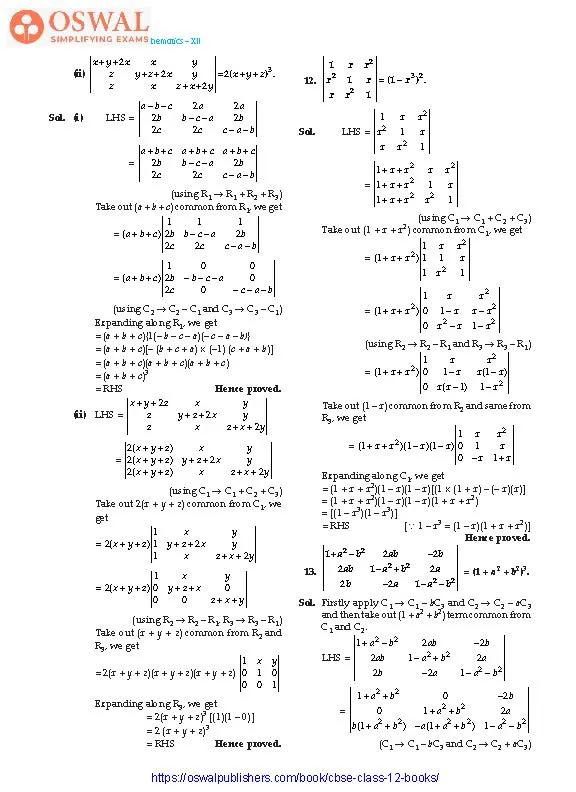
$$\textbf{11\space (i)\space}\begin{vmatrix}\textbf{a-b-c} &\textbf{2a} &\textbf{2a}\\\textbf{2b} &\textbf{b-c-a} &\textbf{2b}\\\textbf{2c} &\textbf{2c} &\textbf{c-a-b}\end{vmatrix}\\\textbf{= (a + b+c)}^\textbf{3}\\\textbf{(ii)\space}\begin{vmatrix}\textbf{x+y+2x} &\textbf{x} &\textbf{y}\\\textbf{z} &\textbf{y+z+2x} &\textbf{y}\\\textbf{z} &\textbf{x} &\textbf{z+x+2y}\end{vmatrix}\\\textbf{=}\space \textbf{2(x+y+z)}^{\textbf{3}}. $$
$$\textbf{Sol.\space (i)\space}\text{LHS = }\\\begin{vmatrix}a-b-c &2a &2a\\2b &b-c-a &2b\\2c &2c &c-a-b\end{vmatrix}\\=\begin{vmatrix}a+b+c &a+b+c &a+b+c\\2b &b-c-a &2b\\2c &2c &c-a-b\end{vmatrix}\\(\text{using}\space \text{R}_{1}\xrightarrow{}\text{R}_{1} + \text{R}_{2} + \text{R}_{3})$$
Take out (a + b + c) common from R1, we get
$$= (a+b+c)\\\begin{vmatrix}1 &1 &1\\2b &b-c-a &2b\\2c &2c &c-a-b\end{vmatrix}\\=\text{(a +b+c)}\\\begin{vmatrix} 1 &0 &0\\2b &-b-c-a &0\\ 2c &0 &-c-a-b\end{vmatrix}$$
(using C2 → C2 – C1 and C3 → C3 – C1)
Expanding along R1, we get
= (a + b + c){1(– b – c – a)(– c – a – b)}
= (a + b + c)[– (b + c + a) × (–1) (c + a + b)]
= (a + b + c)(a + b + c)(a + b + c)
= (a + b + c)3
= RHS Hence proved.
$$\textbf{(ii)\space}\text{LHS} =\\\begin{vmatrix}x+y+2z &x &y\\z &y+z+2x &y\\ z &x &z+x+2y\end{vmatrix}\\=\begin{vmatrix}2(x + y +z) &x &y\\2(x+y+z) &y+z+2x &y\\2(x+y+z) &x &z+x+2y\end{vmatrix}\\(\text{using}\space\text{C}_{1}\xrightarrow{}\text{C}_{1} + \text{C}_{2} + \text{C}_{3})$$
Take out 2(x + y + z) common from C1, we get
$$= 2(x+y+z)\\\begin{vmatrix}1 &x &y\\1 &y+z+2x &y\\ 1 &x &z+x+2y\end{vmatrix}$$
$$= 2(x+y+z)\\\begin{vmatrix}1 &x &y\\0 &y+z+x &0\\0 &0 &z+x+y\end{vmatrix}$$
(using R2 → R2 – R1, R3 → R3 – R1)
Take out (x + y + z) common from R2 and R3, we get
$$= 2(x+y+z)(x+y+z)(x+y+z)\\\begin{vmatrix}1 &x &y\\0 &1 &0\\0 &0 &1\end{vmatrix}$$
Expanding along R3, we get
= 2(x + y + z)3 [(1)(1 – 0)]
= 2 (x + y + z)3
= RHS Hence proved.
$$\textbf{12.\space}\begin{vmatrix}\textbf{1} &\textbf{x} &\textbf{x}^{\textbf{2}}\\\textbf{x}^{\textbf{2}} &\textbf{1} &\textbf{x}\\\textbf{x} &\textbf{x}^{\textbf{2}} &\textbf{1}\end{vmatrix}\\\textbf{(1-x}^{\textbf{3}})^{\textbf{2}}.$$
Sol. LHS =
$$\begin{vmatrix}1 &x &x^{2}\\x^{2} &1 &x\\x &x^{2} &1\end{vmatrix}\\=\begin{vmatrix}1 + x +x^{2} &x &x^{2}\\1 +x +x^{2} &1 &x\\1 +x+x^{2} &x^{2} &1\end{vmatrix}\\(\text{using}\space\text{C}_{1}\xrightarrow{}\text{C}_{1} + \text{C}_{2} + \text{C}_{3})$$
Take out (1 + x + x2) common from C1, we get
$$= (1 + x+x^{2})\begin{vmatrix}1 &x &x^{2}\\1 &1 &x\\1 &x^{2} &1\end{vmatrix}\\=(1 + x +x^{2})\begin{vmatrix}1 &x &x^{2}\\0 &1-x &x-x^{2}\\0 &x^{2}-x &1-x^{2}\end{vmatrix}\\\text{(using}\space \text{R}_{2}\xrightarrow{}\text{R}_{2} - \text{R}_{1}\\\text{and}\space \text{R}_{3}\xrightarrow{}\text{R}_{3} - \text{R}_{1})\\=(1 + x+x^{2})\\\begin{vmatrix}1 &x &x^{2}\\0 &1-x &x(1-x)\\0 &x(x-1) &1-x^{2}\end{vmatrix}$$
Take out (1 – x) common from R2 and same from R3, we get
$$= (1 + x +x^{2})(1-x)(1-x)\\\begin{vmatrix}1 &x &x^{2}\\0 &1 &x\\0 &\normalsize-x &1+x\end{vmatrix}$$
Expanding along C1, we get
= (1 + x + x2)(1 – x)(1 – x)[(1 × (1 + x) – (– x)(x)]
= (1 + x + x2)(1 – x)(1 – x)(1 + x + x2)
= [(1 – x3)(1 – x3)]
= RHS [∵ 1 – x3 = (1 – x)(1 + x + x2)]
Hence proved.
$$\textbf{13.\space}\begin{vmatrix}\textbf{1 +a}^{\textbf{2}}- \textbf{b}^{\textbf{2}} &\textbf{2ab} &\textbf{-2b}\\\textbf{2ab} &\textbf{1 - a}^{\textbf{2}} \textbf{+} \textbf{b}^{\textbf{2}} &\textbf{2a}\\\textbf{2b} &\textbf{\normalsize-2a} &\textbf{1-a}^{\textbf{2}}\textbf{- b}^{\textbf{2}}\end{vmatrix}\\\textbf{=}\space\textbf{(1 + a}^{2} \textbf{+ b}^{\textbf{2}})^{\textbf{3}}\textbf{.}$$
Sol. Firstly apply C1 → C1 – bC3 and C2 → C2 – aC3 and then take out (1 + a2 + b2) term common from C1 and C2.
$$\text{LHS = }\begin{vmatrix}1 + a^{2}-b^{2} &2ab &-2b\\2ab &1-a^{2}+b^{2} &2a\\2b &-2a &1-a^{2}-b^{2}\end{vmatrix}\\=\\\begin{vmatrix}1 +a^{2}+b^{2} &0 &-2b\\0 &1 + a^{2}+b^{2} &2a\\b(1 +a^{2} + b^{2}) &-a(1+a^{2}+b^{2}) &1-a^{2}-b^{2}\end{vmatrix}\\(\text{C}_{1}\xrightarrow{} \text{C}_{1} - b\text{C}_{3}\\\text{and}\space \text{C}_{2}\xrightarrow{}\text{C}_{2} + a\text{C}_{3})\\= (1 +a^{2}+ b^{2})^{2}\\\begin{vmatrix}1 &0 &-2b\\0 &1 &2a\\b &-a &1-a^{2}-b^{2}\end{vmatrix}$$
(Taking out (1 + a2 + b2) common from C1 and same from C2)
$$=\space (1 + a^{2} + b^{2})^{2}\\\begin{vmatrix}1 &0 &-2b\\0 &1 &2a\\0 &0 &1 + a^{2} +b^{2}\end{vmatrix}\\(\text{R}_{3}\xrightarrow{}\text{R}_{3}-b\text{R}_{1}+a\text{R}_{2})$$
Expanding along R1, we get
= (1 + a2 + b2)2[1(1 + a2 + b2)]
= (1 + a2 + b2)3 = RHS
Hence proved.
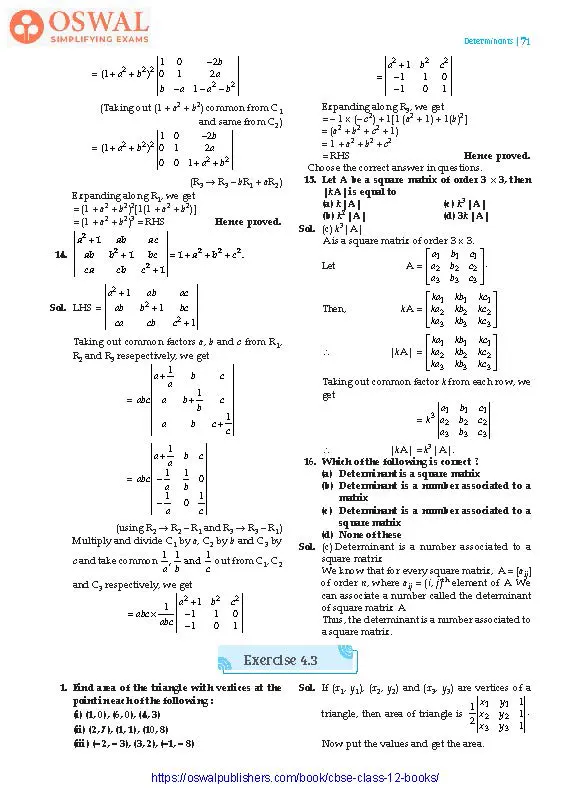
$$\textbf{14.\space}\begin{vmatrix}\textbf{a}^{\textbf{2}}\textbf{+ 1} &\textbf{ab} &\textbf{ac}\\\textbf{ab} &\textbf{b}^{\textbf{2}}\textbf{+ 1} &\textbf{bc}\\\textbf{ca} &\textbf{cb} &\textbf{c}^{\textbf{2}}\textbf{+ 1}\end{vmatrix}\\\textbf{=}\textbf{1 + a}^{\textbf{2}} \textbf{+ b}^{\textbf{2}}\textbf{+ c}^{\textbf{2}}\textbf{.}\\\textbf{Sol.\space}\text{LHS = }\begin{vmatrix}a^{2}+1 &ab &ac\\ab &b^{2}+1 &bc\\ca &cb &c^{2}+1\end{vmatrix} $$
Taking out common factors a, b and c from R1, R2 and R3 resepectively, we get
$$=\space\text{abc}\begin{vmatrix}a+\frac{1}{a} &b &c\\ a &b + \frac{1}{b} &c\\ a &b &c + \frac{1}{c}\end{vmatrix}\\ =\text{abc}\begin{vmatrix}a +\frac{1}{a} &b &c\\-\frac{1}{a} &\frac{1}{b} &0\\-\frac{1}{a} &0 &\frac{1}{c}\end{vmatrix}\\(\text{using}\space\text{R}_{2}\xrightarrow{}\text{R}_{2} -\text{R}_{1}\\\text{and}\space\text{R}_{3}\xrightarrow{}\text{R}_{3} - \text{R}_{1})$$
Multiply and divide C1 by a, C2 by b and C3 by c and take common
$$\frac{1}{a},\frac{1}{b}\text{and}\frac{1}{c}$$
out from C1, C2 and C3 respectively, we get
$$= abc×\frac{1}{abc}\begin{vmatrix}a^{2}+1 &b^{2} &c^{2}\\\normalsize-1 &1 &0\\\normalsize-1 &0 &1\end{vmatrix}\\=\begin{vmatrix}a^{2}+1 &b^{2} &c^{2}\\\normalsize-1 &1 &0\\\normalsize-1 &0 &1\end{vmatrix}$$
Expanding along R3, we get
= – 1 × (– c2) + 1[1 (a2 + 1) + 1(b)2]
= (a2 + b2 + c2 + 1)
= 1 + a2 + b2 + c2
= RHS
Hence proved.
15. Let A be a square matrix of order 3 × 3, then |kA| is equal to
(a) k|A|
(b) k2|A|
(c) k3|A|
(d) 3k|A|
Sol. (c) k3|A|
A is a square matrix of order 3 × 3.
$$\text{Let\space}\text{A = }\begin{vmatrix}a_{1} &b_{1} &c_{1}\\a_{2} &b_{2} &c_{2}\\a_{3} &b_{3} &c_{3}\end{vmatrix}.\\\text{Then,}\space \text{kA} =\begin{vmatrix}ka_{1} &kb_{1} &kc_{1}\\ka_{2} &kb_{2} &kb_{3}\\ka_{3} &kb_{3} &kc_{3}\end{vmatrix}\\\therefore\space|kA| =\begin{bmatrix}ka_{1} &kb_{1} &kc_{1}\\ka_{2} &kb_{2} &kc_{2}\\ka_{3}&kb_{3} &kc_{3}\end{bmatrix}$$
Taking out common factor k from each row, we get
$$=k^{3}\begin{vmatrix}a_{1} &b_{1} &c_{1}\\a_{2} &b_{2} &c_{2}\\a_{3} &b_{3} &c_{3}\end{vmatrix}\\\therefore|kA| = k^{3}|\text{A}|.$$
16. Which of the following is correct ?
(a) Determinant is a square matrix
(b) Determinant is a number associated to a matrix
(c) Determinant is a number associated to a square matrix
(d) None of these
Sol. (c) Determinant is a number associated to a square matrix
We know that for every square matrix, A = [aij] of order n, where aij = (i, j)th element of A. We can associate a number called the determinant of square matrix A.
Thus, the determinant is a number associated to a square matrix.
