NCERT Solutions for Class 12 Maths Chapter 4 Determinants - Exercise 4.3
Access Exercises of Class 12 Maths Chapter 4 –Determinants
Exercise 4.1 Solutions: 8 Questions (2 Long, 5 Short Answers, 1 MCQ)
Exercise 4.2 Solutions: 16 Questions(7 Long, 7 Short, 2 MCQs)
Exercise 4.3 Solutions: 5 Questions ( 4 Short Answers, 1 MCQ)
Exercise 4.4 Solutions: 5 Questions (4 Long, 1 MCQ)
Exercise 4.5 Solutions: 18 Questions (11 Long, 5 Short, 2 MCQs)
Exercise 4.6 Solutions: 16 Questions (13 Long, 3 Short)
Miscellaneous Exercise Solutions: 19 Questions (15 Long, 1 Short, 3 MCQs)
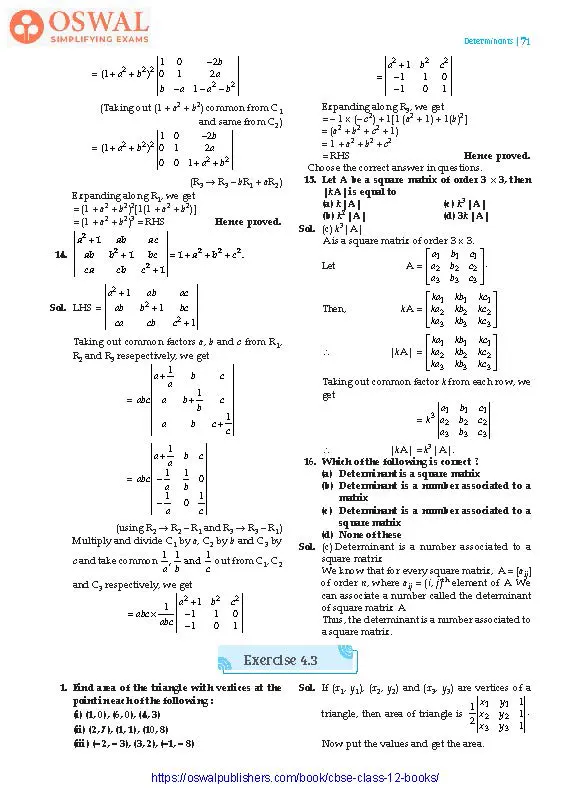
Exercise 4.3
1. Find area of the triangle with vertices at the point in each of the following :
(i) (1, 0), (6, 0), (4, 3)
(ii) (2, 7), (1, 1), (10, 8)
(iii) (– 2, – 3), (3, 2), (–1, – 8)
Sol. If (x1, y1), (x2, y2) and (x3, y3) are vertices of a triangle, then area of triangle is
$$\frac{1}{2}\begin{vmatrix}x_{1} &y_{1} &1\\x_{2} &y_{2} &1\\x_{3} &y_{3} &1\end{vmatrix}.$$
Now put the values and get the area.
$$\textbf{(i) Required area =}\\\space\frac{1}{2}\begin{vmatrix}1 &0 &1\\6 &0 &1\\4 &3 &1\end{vmatrix}\\=\frac{1}{2}\lbrack1(0-3)-0(6-4)\\+ 1(18-0)\rbrack\\=\frac{1}{2}|-3+18|=\frac{15}{2}\space\text{sq. unit}$$
$$\textbf{(ii)\space} \text{Required area} \\=\frac{1}{2}\begin{vmatrix}2 &7 &1\\1 &1 &1\\10 &8 &1\end{vmatrix}\\=\frac{1}{2}\begin{vmatrix}2(1-8)-7(1-10) +1(8-10)\end{vmatrix}\\=\frac{1}{2}|2(\normalsize-7)-7(\normalsize-9)+1(\normalsize-2)|\\=\frac{1}{2}|-14+63-2|\\=\frac{47}{2}\space\text{sq unit}$$
$$\textbf{(iii)\space}\text{Required area =}\\\frac{1}{2}\begin{vmatrix}-2 &-3 &1\\ 3 &2 &1\\\normalsize-1 &-8 &1\end{vmatrix}\\=\frac{1}{2}|-2(2+8)+3(3+1)\\+1(-24+2)|\\=\frac{1}{2}|-20+12-22|\\=\frac{1}{2}|-30| = 15\space\text{sq unit}$$
Note : Area of triangle is always positive.
2. Show that points A(a, b + c), B(b, c + a), C(c, a + b) are collinear.
Sol. Three points are said to be collinear if area of triangle generated by these three points is equal to zero.
Area of ΔABC
$$=\frac{1}{2}\begin{vmatrix}a &b+c &1\\b &c+a &1\\c &a+b &1\end{vmatrix}\\=\frac{1}{2}\begin{vmatrix}a\lbrace(c+a)×1 - (a+b)×1\rbrace -\\(b+c)\lbrace b×1-1×c\rbrace\\+1\lbrace b×(a+b) - (c+a)×c\rbrace\end{vmatrix}\\=\frac{1}{2}\lbrace a(c+a-a-b)-(b+c)(b-c)\\+1(ab+b^{2}-c^{2}-ac)\rbrace\\=\frac{1}{2}\begin{vmatrix}\text{ac - ab- b}^{2}+c^{2}+ab+\\b^{2}-c^{2}-ac\end{vmatrix}\\=\frac{1}{2}×0=0$$
Since, area of ΔABC = 0
Hence, points A(a, b + c), B(b, c + a) and C(c, a + b) are collinear.
3. Find the value of k, if area of triangle is 4 sq units and vertices are :
(i) (k, 0), (4, 0) (0, 2)
(ii) (– 2, 0), (0, 4), (0, k)
$$\textbf{Sol.}\space \text{Area of triangle =}\\\frac{1}{2}\begin{vmatrix}x_{1} &y_{1} &1\\x_{2} &y_{2} &1\\x_{3} &y_{3} &1\end{vmatrix}\\\textbf{(i)\space}\text{Given,}\space\frac{1}{2}\begin{vmatrix}k &0 &1\\4 &0 &1\\0 &2 &1\end{vmatrix}=4\\\Rarr\begin{vmatrix}k(0-2) + (8-0)\end{vmatrix}=8\\\Rarr\space k(0-2)+1(8-0) = \pm8 $$
On taking positive sign
– 2k + 8 = 8
⇒ – 2k = 0 ⇒ k = 0
On taking negative sign
– 2k + 8 = – 8
⇒ – 2k = 16 ⇒ k = 8
∴ k = 0, 8
$$\text{(ii)\space}\text{Given,}\frac{1}{2}\begin{vmatrix}\normalsize-2 &0 &1\\0 &4 &1\\0 &k &1\end{vmatrix}\\=4\\\Rarr\space \begin{vmatrix}-2(4-k)+1(0-0)\end{vmatrix}\\=8\\\Rarr\space \lbrack-2(4-k)\rbrack\\=\pm \space8\\\Rarr\space\lbrack-8+2k\rbrack =\pm\space 8$$
On taking positive sign,
2k – 8 = 8
⇒ 2k = 16 ⇒ k = 8
On taking negative sign,
2k – 8 = – 8
2k = 0 ⇒ k = 0
∴ k = 0, 8
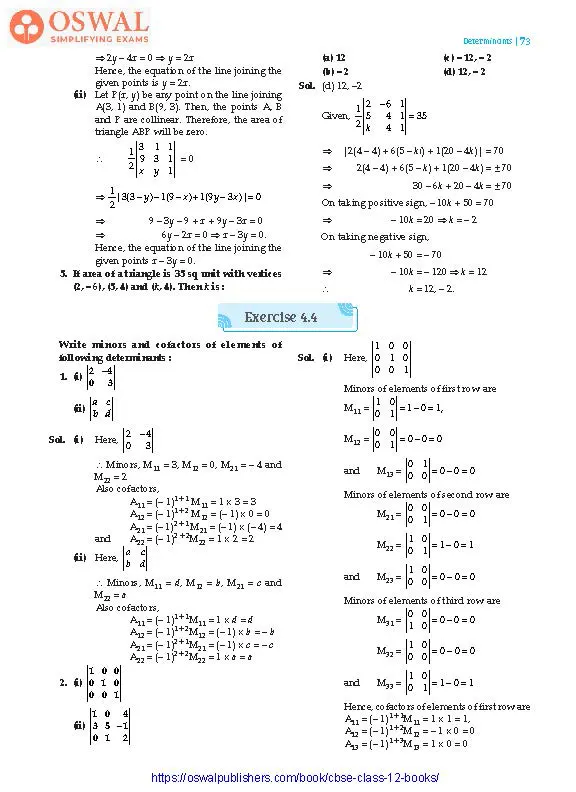
4. (i) Find the equation of the line joining (1, 2) and (3, 6) using determinants.
(ii) Find the equation of the line joining (3, 1) and (9, 3) using determinants.
Sol. If using the relation
$$\frac{1}{2}\begin{vmatrix}x_{1} &y_{1} &1\\x_{2} &y_{2} &1\\x_{3} &y_{3} &1\end{vmatrix} = 0$$
for finding the equation of line. Make (x1, y1) as (1, 2) and (x2, y2) and (3, 6) and (x3, y3) as (x, y) solve to get equation of line.
(i) Let P(x, y) be any point on the line joining A(1, 2) and B(3, 6). If the points A, B and P are collinear, then the area of triangle ABP will be zero.
$$\therefore\space\frac{1}{2}\begin{vmatrix}1 &2 &1\\3 &6 &1\\x &y &1\end{vmatrix}= 0\\\Rarr\frac{1}{2}\lbrack1(6-y)-2(3-x)+\\1(3y-6x)\rbrack=0\\\Rarr\space 6-y-6+2x+3y-6x=0\\\Rarr\space 2y-4x=0\\\Rarr\space y=2x$$
Hence, the equation of the line joining the given points is y = 2x.
(ii) Let P(x, y) be any point on the line joining A(3, 1) and B(9, 3). Then, the points A, B and P are collinear. Therefore, the area of triangle ABP will be zero.
$$\therefore\space \frac{1}{2}\begin{vmatrix}3 &1 &1\\9 &3 &1\\x &y &1\end{vmatrix}=0\\\Rarr\space\frac{1}{2}\begin{vmatrix}3(3-y)-1(9-x)+\\1(9y-3x)\end{vmatrix}= 0\\\Rarr\space 9-3y-9+x+9y-3x=0\\\Rarr\space 6y-2x=0\\\Rarr\space x-3y = 0.$$
Hence, the equation of the line joining the given points x – 3y = 0.
5. If area of a triangle is 35 sq unit with vertices (2, – 6), (5, 4) and (k, 4). Then k is :
(a) 12
(b) – 2
(c) – 12, – 2
(d) 12, – 2
Sol. (d) 12, –2
$$\text{Given,\space}\frac{1}{2}\begin{vmatrix}2 &-6 &1\\5 &4 &1\\ k &4 &1\end{vmatrix}=35\\\Rarr\space \begin{vmatrix}2(4-4) +\\ 6(5-ki) + 1(20-4k)\end{vmatrix}=70\\\Rarr\space 2(4-4) +6(5-k)+ 1(20-4k)\\=\pm70\\\Rarr\space 30-6k+20-4k=\pm70$$
On taking positive sign, – 10k + 50 = 70
$$\Rarr\space -10k =20\\\Rarr\space k =-2$$
On taking negative sign,
– 10k + 50 = – 70
$$\Rarr\space -10k =-120\\\Rarr\space k=12\\\therefore\space k=12,-2.$$
Share page on
