NCERT Solutions for Class 12 Maths Chapter 4 Determinants - Exercise 4.5
Access Exercises of Class 12 Maths Chapter 4 –Determinants
Exercise 4.1 Solutions: 8 Questions (2 Long, 5 Short Answers, 1 MCQ)
Exercise 4.2 Solutions: 16 Questions(7 Long, 7 Short, 2 MCQs)
Exercise 4.3 Solutions: 5 Questions ( 4 Short Answers, 1 MCQ)
Exercise 4.4 Solutions: 5 Questions (4 Long, 1 MCQ)
Exercise 4.5 Solutions: 18 Questions (11 Long, 5 Short, 2 MCQs)
Exercise 4.6 Solutions: 16 Questions (13 Long, 3 Short)
Miscellaneous Exercise Solutions: 19 Questions (15 Long, 1 Short, 3 MCQs)
Exercise 4.5
$$\textbf{1.\space}\begin{vmatrix}\textbf{1} &\textbf{2}\\\textbf{3} &\textbf{4}\end{vmatrix}\\\textbf{Sol.\space}\text{Let A} =\begin{vmatrix}1 &2\\3 &4\end{vmatrix}$$
∴ A11 = 4, A12 = – 3, A21 = – 2 and A22 = 1
$$\therefore\space\text{A}_{11}= 4, \text{A}_{12} =\normalsize-3,\\\text{A}_{21} =\normalsize-2\space\text{and}\space\text{A}_{22} = 1\\\therefore\space\text{adj}\space \text{A} = \begin{vmatrix}\text{A}_{11} &\text{A}_{12}\\\text{A}_{21} &\text{A}_{22}\end{vmatrix}^{\text{T}}\\=\begin{bmatrix}4 &\normalsize-3\\\normalsize-2 &1\end{bmatrix}^{\text{T}} = \begin{bmatrix}4 &\normalsize-2\\\normalsize-3 &1\end{bmatrix}$$
$$\textbf{2.\space}\begin{bmatrix}\textbf{1} &\textbf{\normalsize-1} &\textbf{2}\\\textbf{2} &\textbf{3} &\textbf{5}\\\textbf{\normalsize-2} &\textbf{0} &\textbf{1}\end{bmatrix}\textbf{.}\\\textbf{Sol.\space}\text{Let A =}\begin{bmatrix}1 &\normalsize-1 &2\\2 &3 &5\\\text{\normalsize-2} &\text{0} &\text{1}\end{bmatrix}$$
Cofactors of elements of first row are
$$\text{A}_{11}=\begin{vmatrix}3 &5\\0 &1\end{vmatrix}\\=3-0=3\\\text{A}_{12} = -\begin{vmatrix}2 &5\\-2 &1\end{vmatrix}\\=-(2+10)=-12\\\text{A}_{13} =\begin{vmatrix}2 &3\\\normalsize-2 &0\end{vmatrix}\\0-(\normalsize-6) = 6 $$
Cofactors of elements of second row are
$$\text{A}_{21} =-\begin{vmatrix}-1 &2\\0 &1\end{vmatrix}\\=-(-1-0)=1,\\\text{A}_{22} =\begin{vmatrix}1 &2\\\normalsize-2 &1\end{vmatrix}\\=(1+4)=5,\\\text{A}_{23} =-1\begin{vmatrix}1 &\normalsize-1\\\normalsize-2 &0\end{vmatrix}\\-(0-2)=2$$
$$\text{A}_{31} =\begin{vmatrix}-1 &2\\3 &5\end{vmatrix}\\=(-5-6)=-11,\\\text{A}_{32} =-\begin{vmatrix}1 &2\\2 &5\end{vmatrix}\\=-(5-4)=-1,\\\text{A}_{33}=\begin{vmatrix}1 &\normalsize-1\\2 &3\end{vmatrix}\\= 3+2 = 5\\\therefore\space\text{adj}(\text{A}) =\begin{vmatrix}3 &\normalsize-12 &6\\1 &5 &2\\\normalsize-11 &\normalsize-1 &5\end{vmatrix}^{\text{T}}$$
$$=\begin{bmatrix}3 &1 &\normalsize-11\\\normalsize-12 &5 &\normalsize-1\\6 &2 &5\end{bmatrix}$$
Verify A(adj A) = (adj A)A = |A|In in Q. 3 and 4.
$$\textbf{3.\space}\begin{bmatrix}\textbf{2} &\textbf{3}\\\textbf{\normalsize-4} &\textbf{\normalsize-6}\end{bmatrix}\textbf{.}\\\textbf{Sol.\space}\text{Let A =}\begin{bmatrix}2 &3\\\normalsize-4 &\normalsize-6\end{bmatrix},\\\text{|A| = }\begin{bmatrix}2 &3\\-4 &-6\end{bmatrix}\\= -12-(-12)\\\text{-12+12 = 0}\\\therefore\space|\text{A}|\text{I} =0\begin{bmatrix}1 &0\\0 &1\end{bmatrix}\\=\begin{bmatrix}0 &0\\0 &0\end{bmatrix}=0\\\text{Cofactors of A are A}_{11} =\normalsize-6,\\\text{A}_{12}=4,\text{A}_{21}=\normalsize-3,\text{A}_{22}=2$$
$$\therefore\space\text{adj}(A) = \begin{bmatrix}\normalsize-6 &4\\\normalsize-3 &2\end{bmatrix}^{\text{T}} \\=\begin{bmatrix}\normalsize-6 &\normalsize-3\\4 &2\end{bmatrix}\\\text{Now,\space}\text{(adj A)}\text{A}=\\\begin{bmatrix}\normalsize-6 &\normalsize-3\\4 &2\end{bmatrix}\begin{bmatrix}2 &3\\\normalsize-4 &\normalsize-6\end{bmatrix}\\=\begin{bmatrix}-12+12 &-18+18\\8-8 &12-12\end{bmatrix}\\=\begin{bmatrix}0 &0\\0 &0\end{bmatrix}=0$$
Hence, A(adj A) = (adj A)A = |A|I2.
$$\textbf{4.\space}\begin{vmatrix}\textbf{1} &\textbf{\normalsize-1} &\textbf{2}\\\textbf{3} &\textbf{0} &\textbf{\normalsize-2}\\\textbf{1} &\textbf{0} &\textbf{3}\end{vmatrix}\\\textbf{sol.\space}\text{Let }\space\text{A =}\begin{vmatrix}1 &\normalsize-1 &2\\3 &0 &\normalsize-2\\1 &0 &3\end{vmatrix}\\\text{Now,\space}|\text{A}| = \begin{vmatrix}1 &\normalsize-1 &2\\3 &0 &\normalsize-2\\1 &0 &3\end{vmatrix}$$
= 1(0 – 0) – (– 1)(9 + 2) + 2(0 – 0)
= 0 + 11 + 0 = 11
$$\therefore\space|\text{A}|\text{I} = 11\begin{vmatrix}1 &0 &0\\0 &1 &0\\0 &0 &1\end{vmatrix} =\\\begin{bmatrix}11 &0 &0\\0 &11 &0\\0 &0 &11\end{bmatrix}$$
Cofactors of A are
A11 = 0, A12 = – (9 + 2) = – 11
A13 = 0, A21 = – (– 3 – 0) = 3,
A22 = 3 – 2 = 1, A23 = – (0 + 1) = – 1,
A31 = 2 – 0 = 2, A32 = – (– 2 – 6) = 8,
A33 = 0 + 3 = 3
$$\therefore\space\text{adj (A)} = \begin{bmatrix}0 &\normalsize-11 &0\\3 &1 &\normalsize-1\\2 &8 &3\end{bmatrix}^{\text{T}}\\=\begin{bmatrix}0 &3 &2\\\normalsize-11 &1 &8\\0 &\normalsize-1 &3\end{bmatrix}\\\text{Now, A(adj A)} =\begin{bmatrix}1 &\normalsize-1 &2\\3 &0 &\normalsize-2\\1 &0 &3\end{bmatrix}\\\begin{bmatrix}0 &3 &2\\\normalsize-11 &1 &8\\0 &\normalsize-1 &3\end{bmatrix}$$
$$=\begin{bmatrix}0+11+0 &3-1-2 &2-8+6\\0+0+0 &9+0+2 &6+0-6\\0+0+0 &3+0-3 &2+0+9\end{bmatrix}\\=\begin{bmatrix}11 &0 &0\\0 &11 &0\\0 &0 &11\end{bmatrix}\\\text{Also, (adj A)A =}\space\begin{bmatrix}0 &3 &2\\-11 &1 &8\\0 &\normalsize-1 &8\end{bmatrix}\\\begin{bmatrix}1 &\normalsize-1 &2\\3 &0 &\normalsize-2\\1 &0 &3\end{bmatrix}\\=\begin{bmatrix}0+9+2 &0+0+0 &0-6+0\\-11+3+8 &11+0+0 &-22-2+24\\0-3+3 &0+0+0 &0+2+9\end{bmatrix}$$
$$= \begin{bmatrix}11 &0 &0\\0 &11 &0\\0 &0 &11\end{bmatrix} $$
Hence, A(adj A) = (adj A)A = |A|I3
Find the inverse of each of the matrices (if it exists).
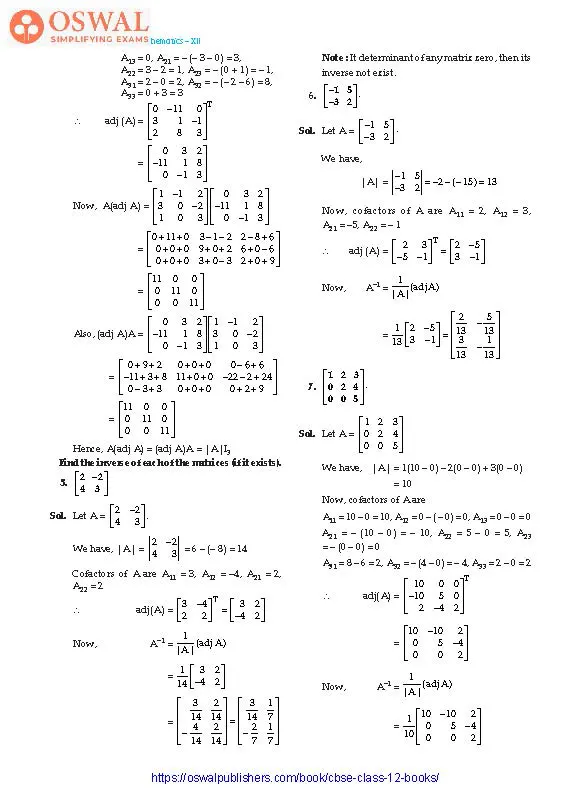
$$\textbf{5.\space}\begin{bmatrix}\textbf{2} &\textbf{\normalsize-2}\\\textbf{4} &\textbf{3}\end{bmatrix}\\\textbf{Sol.\space}\text{Let A} =\begin{bmatrix}2 &\normalsize-2\\4 &3\end{bmatrix}.\\\text{We have,}\\|\text{A}| = \begin{bmatrix}2 &\normalsize-2\\ 4 &3\end{bmatrix}\\=6-(\normalsize-8) = 14$$
Cofactors of A are A11 = 3, A12 = –4, A21 = 2,
A22 = 2
$$\therefore\space\text{adj(A)} =\begin{bmatrix}3 &\normalsize-4\\2 &2\end{bmatrix}^{\text{T}}\\=\begin{bmatrix}3 &2\\\normalsize-4 &2\end{bmatrix}\\\text{Now,\space}\text{A}^{\normalsize-1}=\frac{1}{\text{|A|}}\space\text{(adj\space A)}\\=\frac{1}{14}\begin{bmatrix}3 &2\\\normalsize-4 &2\end{bmatrix}\\=\begin{bmatrix}\frac{3}{14} &\frac{2}{14}\\-\frac{4}{14} &\frac{2}{14}\end{bmatrix}\\=\begin{bmatrix}\frac{3}{14} &\frac{1}{7}\\-\frac{2}{7} &\frac{1}{7}\end{bmatrix}$$
Note : It determinant of any matrix zero, then its inverse not exist.
$$\textbf{6.\space}\begin{bmatrix}\textbf{\normalsize-1} &\textbf{5}\\\textbf{-3} &\textbf{2}\end{bmatrix}\textbf{.}\\\textbf{Sol.\space}\text{Let A} = \begin{bmatrix}-1 &5\\-3 &2\end{bmatrix}.\\\text{we have}\\\text{|A| = }\begin{vmatrix}\normalsize-1 &5\\\normalsize-3 &2\end{vmatrix}\\=-2-(-15)=13$$
Now, cofactors of A are A11 = 2, A12 = 3, A21 = –5, A22 = – 1
$$\therefore\space \text{adj(A)} =\begin{bmatrix}2 &3\\\normalsize-5 &\normalsize-1\end{bmatrix}^{\text{T}}\\=\begin{bmatrix}2 &\normalsize-5\\3 &\normalsize-1\end{bmatrix}\\\text{Now, A}^{\normalsize-1} =\frac{1}{|A|}\text{(adj A)}\\=\frac{1}{13}\begin{bmatrix}2 &\normalsize-5\\3 &\normalsize-1\end{bmatrix}\\=\begin{bmatrix}\frac{2}{13} &-\frac{5}{13}\\\frac{3}{13} &-\frac{1}{13}\end{bmatrix}$$
$$\textbf{7.\space}\begin{bmatrix}\textbf{1} &\textbf{2} &\textbf{3}\\\textbf{0} &\textbf{2} &\textbf{4}\\\textbf{0} &\textbf{0} &\textbf{5}\end{bmatrix}\textbf{.}\\\textbf{Sol.\space}\text{Let A} = \begin{bmatrix}1 &2 &3\\0 &2 &4\\0 &0 &5\end{bmatrix}$$
We have, |A| = 1(10 – 0) – 2(0 – 0) + 3(0 – 0)
= 10
Now, cofactors of A are
A11 = 10 – 0 = 10, A12 = 0 – ( – 0) = 0, A13 = 0 – 0 = 0
A21 = – (10 – 0) = – 10, A22 = 5 – 0 = 5, A23 = – (0 – 0) = 0
A31 = 8 – 6 = 2, A32 = – (4 – 0) = – 4, A33 = 2 – 0 = 2
$$\therefore\space \text{adj(A)}\space= \space\begin{bmatrix}10 &0 &0\\\normalsize-10 &5 &0\\2 &\normalsize-4 &2\end{bmatrix}^{\text{T}}\\=\begin{bmatrix}10 &-10 &2\\0 &5 &-4\\0 &0 &2\end{bmatrix}\\\text{Now,\space}\text{A}^{\normalsize-1}\frac{1}{|\text{A}|}\space\text{(adj A)}\\=\frac{1}{10}\begin{bmatrix}10 &\normalsize-10 &2\\0 &5 &\normalsize-4\\0 &0 &2\end{bmatrix}\\=\begin{bmatrix}1 &\normalsize-1 &\frac{1}{5}\\0 &\frac{1}{2} &-\frac{2}{5}\\0 &0 &\frac{1}{5}\end{bmatrix}$$
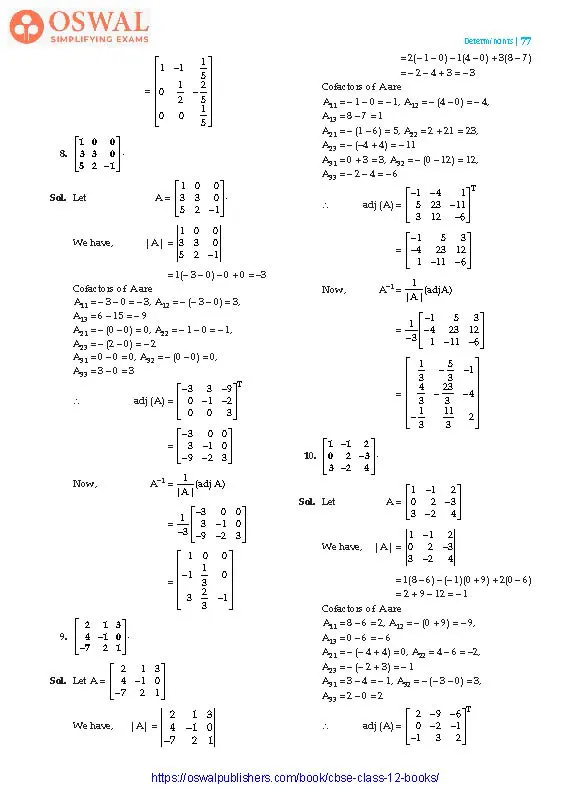
$$\textbf{8.\space}\begin{bmatrix}\textbf{1} &\textbf{0} &\textbf{0}\\\textbf{3} &\textbf{3} &\textbf{0}\\\textbf{5} &\textbf{2} &\textbf{\normalsize-1}\end{bmatrix}\textbf{.}\\\textbf{Sol.\space}\text{Let}\space\text{A} = \begin{bmatrix}1 &0 &0\\3 &3 &0\\5 &2 &\normalsize-1\end{bmatrix}\\\text{We have,}\space|\text{A}| =\begin{vmatrix}1 &0 &0\\3 &3 &0\\5 &2 &\normalsize-1\end{vmatrix}$$
= 1(– 3 – 0) – 0 + 0 = –3
Cofactors of A are
A11 = – 3 – 0 = – 3, A12 = – (– 3 – 0) = 3,
A13 = 6 – 15 = – 9
A21 = – (0 – 0) = 0, A22 = – 1 – 0 = – 1,
A23 = – (2 – 0) = – 2
A31 = 0 – 0 = 0, A32 = – (0 – 0) = 0,
A33 = 3 – 0 = 3
$$\therefore\space\text{adj(A) = }\begin{bmatrix}-3 &3 &\normalsize-9\\0 &\normalsize-1 &\normalsize-2\\0 &0 &3\end{bmatrix}^{\text{T}}\\=\begin{bmatrix}-3 &0 &0\\3 &\normalsize-1 &0\\\normalsize-9 &\normalsize-2 &3\end{bmatrix}\\\text{Now,\space A}^{\normalsize-1}=\frac{1}{|\text{A}|}(\text{adj A})\\=\frac{1}{-3}\begin{bmatrix}-3 &0 &0\\3 &\normalsize-1 &0\\\normalsize-9 &\normalsize-2 &3\end{bmatrix}\\=\begin{bmatrix}1 &0 &0\\\normalsize-1 &\frac{1}{3} &0\\3 &\frac{2}{3} &\normalsize-1\end{bmatrix}$$
$$\textbf{9.\space}\begin{bmatrix}\textbf{2} &\textbf{1} &\textbf{3}\\\textbf{4} &\textbf{\normalsize-1} &\textbf{0}\\\textbf{\normalsize-7} &\textbf{2} &\textbf{1}\end{bmatrix}\\\textbf{Sol.\space}\text{Let A = }\begin{bmatrix}2 &1 &3\\4 &\normalsize-1 &0\\\normalsize-7 &2 &1\end{bmatrix}\\\text{We have,}\space\\\text{|A| =}\begin{vmatrix}2 &1 &3\\4 &\normalsize-1 &0\\\normalsize-7 &2 &1\end{vmatrix}$$
= 2(– 1 – 0) – 1(4 – 0) + 3(8 – 7)
= – 2 – 4 + 3 = – 3
Cofactors of A are
A11 = – 1 – 0 = – 1, A12 = – (4 – 0) = – 4,
A13 = 8 – 7 = 1
A21 = – (1 – 6) = 5, A22 = 2 + 21 = 23,
A23 = – (–4 + 4) = – 11
A31 = 0 + 3 = 3, A32 = – (0 – 12) = 12,
A33 = – 2 – 4 = – 6
$$\therefore\space\text{adj(A)} =\begin{bmatrix}\normalsize-1 &\normalsize-4 &1\\5 &\normalsize23 &\normalsize-11\\3 &12 &\normalsize-6\end{bmatrix}^{\text{T}}\\=\begin{bmatrix}\normalsize-1 &5 &3\\\normalsize-4 &23 &12\\1 &\normalsize-11 &\normalsize-6\end{bmatrix}\\\text{Now,\space}\text{A}^{\normalsize-1} =\frac{1}{|\text{A}|}\space\text{(adj A)}\\=\frac{1}{\normalsize-3}\begin{bmatrix}-1 &5 &3\\-4 &23 &12\\ 1 &\normalsize-11 &\normalsize-6\end{bmatrix}\\=\begin{bmatrix}\frac{1}{3} &-\frac{5}{3} &\normalsize-1\\\frac{4}{3} &-\frac{23}{3} &\normalsize-4\\-\frac{1}{3} &\frac{11}{3} &2\end{bmatrix}$$
$$\textbf{10.\space}\begin{bmatrix}\textbf{1} &\textbf{\normalsize-1} &\textbf{2}\\\textbf{0} &\textbf{2} &\textbf{\normalsize-3}\\\textbf{3} &\textbf{\normalsize-2} &\textbf{4}\end{bmatrix}\textbf{.}\\\text{Let\space A =}\begin{bmatrix}1 &\normalsize-1 &2\\0 &2 &\normalsize-3\\3 &\normalsize-2 &4\end{bmatrix}\\\text{we have,}\space|\text{A}| =\begin{vmatrix}1 &\normalsize-1 &2\\0 &2 &\normalsize-3\\3 &\normalsize-2 &4\end{vmatrix}$$
= 1(8 – 6) – (– 1)(0 + 9) + 2(0 – 6)
= 2 + 9 – 12 = – 1
Cofactors of A are
A11 = 8 – 6 = 2, A12 = – (0 + 9) = – 9,
A13 = 0 – 6 = – 6
A21 = – (– 4 + 4) = 0, A22 = 4 – 6 = –2,
A23 = – (– 2 + 3) = – 1
A31 = 3 – 4 = – 1, A32 = – (– 3 – 0) = 3,
A33 = 2 – 0 = 2
$$\therefore\space\text{adj(A) = }\begin{bmatrix}2 &\normalsize-9 &\normalsize-6\\0 &\normalsize-2 &\normalsize-1\\\normalsize-1 &3 &2\end{bmatrix}^{\text{T}}\\=\begin{bmatrix}2 &0 &\normalsize-1\\\normalsize-9 &\normalsize-2 &3\\\normalsize-6 &\normalsize-1 &2\end{bmatrix}\\\text{Now,\space}\text{A}^{\normalsize-1}\frac{1}{|\text{A}|}(\text{adj A})\\=\frac{1}{\normalsize-1}\begin{bmatrix}2 &0 &\normalsize-1\\-9 &\normalsize-2 &3\\\normalsize-6 &\normalsize-1 &2\end{bmatrix}\\=\begin{bmatrix}\normalsize-2 &0 &1\\9 &2 &\normalsize-3\\6 &1 &\normalsize-2\end{bmatrix}$$
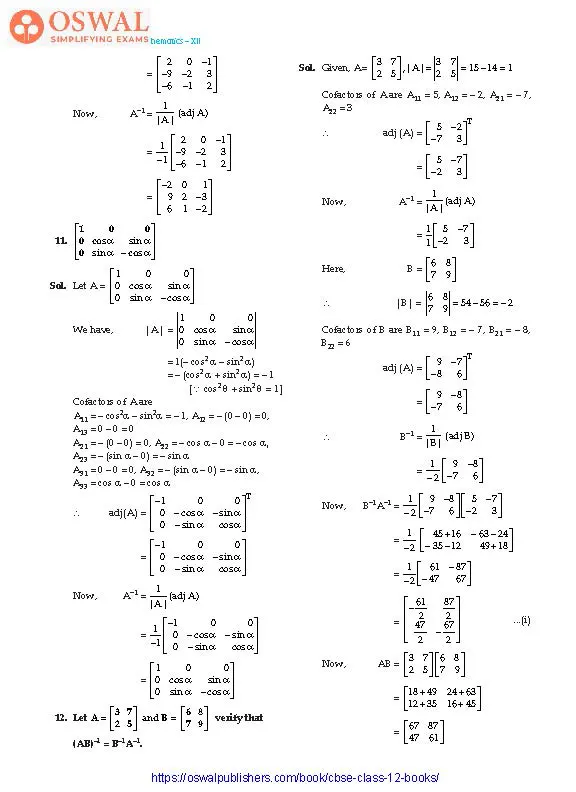
$$\textbf{11.\space}\begin{bmatrix}\textbf{1} &\textbf{0} &\textbf{0}\\\textbf{0} &\textbf{cos}\space\alpha &\textbf{sin}\space\alpha\\\textbf{0} &\textbf{sin}\space\alpha &\textbf{-cos}\space\alpha\end{bmatrix}\\\textbf{Sol.\space}\text{Let A = }\begin{bmatrix}1 &0 &0\\0 &\text{cos}\space\alpha &\text{cos}\space\alpha\\0 &\text{sin}\space\alpha &\text{-cos}\space\alpha\end{bmatrix}\\\text{We have,\space}\\|\text{A}| = \begin{vmatrix}1 &0 &0\\0 &\text{cos}\space\alpha &\text{sin}\space\alpha\\0 &\text{sin}\space\alpha &-\text{cos}\space\alpha\end{vmatrix}$$
= 1(– cos2 α – sin2 α)
= – (cos2 α + sin2 α) = – 1
[∵ cos2 θ + sin2θ = 1]
Cofactors of A are
A11 = – cos2α – sin2α = – 1, A12 = – (0 – 0) = 0,
A13 = 0 – 0 = 0
A21 = – (0 – 0) = 0, A22 = – cos α – 0 = – cos α,
A23 = – (sin α – 0) = – sin α
A31 = 0 – 0 = 0, A32 = – (sin α – 0) = – sin α,
A33 = cos α – 0 = cos α
$$\therefore\space\text{adj(A)} =\begin{bmatrix}\text{\normalsize-1} &\text{0} &\text{0}\\\text{0} &\normalsize-\text{cos}\alpha &-\text{sin}\alpha\\0 &\text{-sin}\space\alpha &\text{cos}\space\alpha\end{bmatrix}^{\text{T}}\\=\begin{bmatrix}\normalsize-1 &0 &0\\0 &-\text{cos}\space\alpha &-\text{sin}\space\alpha\\0 &-\text{sin}\space\alpha &\text{cos}\alpha\end{bmatrix}\\\text{Now,\space}\text{A}^{\normalsize-1}=\frac{1}{|\text{A}|}\space\text{(adj A)}\\=\frac{1}{\normalsize-1}\begin{bmatrix}\normalsize-1 &0 &0\\0 &-\text{cos}\alpha &-\text{sin}\space\alpha\\0 &-\text{sin}\space\alpha &\text{cos}\space\alpha\end{bmatrix}\\=\begin{bmatrix}1 &0 &0\\0 &\text{cos}\space\alpha &\text{sin}\space\alpha\\0 &\text{sin}\space\alpha &-\text{cos}\space\alpha\end{bmatrix}$$
$$\textbf{12.\space Let A =}\begin{bmatrix}\textbf{3} &\textbf{7}\\\textbf{2} &\textbf{5}\end{bmatrix}\space\textbf{and B =}\begin{bmatrix}\textbf{6} &\textbf{8}\\\textbf{7} &\textbf{9}\end{bmatrix}$$
verify that (AB)–1 = B–1A–1.
$$\textbf{Sol.\space}\text{Given, A} = \begin{bmatrix}3 &7\\2 &5\end{bmatrix},\\\text{|A|} = \begin{vmatrix}3 &7\\2 &5\end{vmatrix}\\= 15-14 = 1$$
Cofactors of A are A11 = 5, A12 = – 2, A21 = – 7, A22 = 3
$$\therefore\space\text{adj(A)} = \begin{bmatrix}5 &\normalsize-2\\\normalsize-7 &3\end{bmatrix}^{\text{T}}\\=\begin{bmatrix}5 &\normalsize-7\\\normalsize-2 &3\end{bmatrix}\\\text{Now,\space}\text{A}^{\normalsize-1}=\frac{1}{|\text{A}|}\space\text{(adj A)}\\=\frac{1}{1}\begin{bmatrix}5 &\normalsize-7\\\normalsize-2 &3\end{bmatrix}\\\text{Here, \space}\text{B = }\begin{bmatrix}6 &8\\7 &9\end{bmatrix}\\\therefore\space|\text{B}| = \begin{bmatrix}6 &8\\7 &9\end{bmatrix}$$
= 54 − 56 = − 2
Cofactors of B are B11 = 9, B12 = – 7, B21 = – 8, B22 = 6
$$\text{adj(A)} = \begin{bmatrix}9 &\normalsize-7\\\normalsize-8 &6\end{bmatrix}^{\text{T}}\\=\begin{bmatrix}9 &\normalsize-8\\\normalsize-7 &6\end{bmatrix}\\\therefore\text{B}^{\normalsize-1} =\frac{1}{|\text{B}|}\space\text{(adj B)}\\=\frac{1}{\normalsize-2}\begin{bmatrix}9 &\normalsize-8\\\normalsize-7 &6\end{bmatrix}\\\text{Now,\space B}^{\normalsize-1}\text{A}^{\normalsize-1}\\=\frac{1}{\normalsize-2}\begin{bmatrix}9 &\normalsize-8\\\normalsize-7 &6\end{bmatrix}\begin{bmatrix}5 &\normalsize-7\\\normalsize-2 &3\end{bmatrix}\\=\frac{1}{\normalsize-2}\begin{bmatrix}45+16 &-63-24\\-35-12 &49+18\end{bmatrix}$$
$$=\frac{1}{\normalsize-2}\begin{bmatrix}61 &-87\\-47 &67\end{bmatrix}\\=\begin{bmatrix}-\frac{61}{2} &\frac{87}{2}\\\frac{47}{2} &-\frac{67}{2}\end{bmatrix}\space\text{...(i)}\\\text{Now,\space}\text{AB} = \begin{bmatrix}3 &7\\2 &5\end{bmatrix}\begin{bmatrix}6 &8\\7 &9\end{bmatrix}\\=\begin{bmatrix}18+49 &24+63\\12+35 &16+45\end{bmatrix}\\=\begin{bmatrix}67 &87\\47 &61\end{bmatrix}\\\therefore\space|\text{AB}| =\begin{vmatrix}67 &87\\47 &61\end{vmatrix}$$
= 67 × 61 – 47 × 87
= 4087 – 4089 = – 2
Cofactors of AB are A11 = 61, A12 = – 47, A21 = – 87, A22 = 67
$$\text{adj(AB)} =\begin{bmatrix}61 &-47\\-87 &67\end{bmatrix}^{\text{T}}\\=\begin{bmatrix}61 &-87\\-47 &67\end{bmatrix}\\\therefore\space\text{(AB)}^{\normalsize-1}=\frac{1}{|\text{AB}|}\space\text{(adj AB)}\\=\frac{1}{\normalsize-2}\begin{bmatrix}61 &-87\\-47 &67\end{bmatrix}\\=\frac{1}{2}\begin{bmatrix}-\frac{61}{2} &\frac{87}{2}\\\frac{47}{2} &-\frac{67}{2}\end{bmatrix}\space\text{...(ii)}$$
From Eqs. (i) and (ii), we get (AB)–1 = B–1A–1
Hence, the given result is proved.
$$\textbf{13. If A =}\space\begin{bmatrix}\textbf{3} &\textbf{1}\\\textbf{\normalsize-1} &\textbf{2}\end{bmatrix}\textbf{,}$$
show that A2 – 5A + 7I = O.
Hence, find A–1.
$$\textbf{Sol.\space}\text{Given, A} =\begin{bmatrix}3 &1\\\normalsize-1 &2\end{bmatrix}\\\therefore\space\text{A}^{2} =\text{AA}\\=\begin{bmatrix}3 &1\\\normalsize-1 &2\end{bmatrix}\begin{bmatrix}3 &1\\\normalsize-1 &2\end{bmatrix}\\=\begin{bmatrix}9-1 &3+2\\-3-2 &-1+4\end{bmatrix}\\=\begin{bmatrix}8 &5\\-5 &3\end{bmatrix}$$
Now, A2 – 5A + 7I
$$=\begin{bmatrix}8 &5\\-5 &3\end{bmatrix}-5\begin{bmatrix}3 &1\\-1 &2\end{bmatrix}\\+ 7\begin{bmatrix}1 &0\\0 &1\end{bmatrix}\\=\begin{bmatrix}8 &5\\-5 &3\end{bmatrix}-\begin{bmatrix}15 &5\\\normalsize-5 &10 \end{bmatrix}\\+\begin{bmatrix}7 &0\\0 &7\end{bmatrix}\\=\begin{bmatrix}8-15+7 &5-5+0\\-5+5+0 &3-10+7\end{bmatrix}\\=\begin{vmatrix}0 &0\\0 &0\end{vmatrix}=\text{O}$$
∴ A2 – 5A + 7I = O
$$\because\space|\text{A}| =\begin{vmatrix}3 &1\\\normalsize-1 &2\end{vmatrix}$$
= 6 + 1 = 7 ≠ 0
∴ A–1 exists.
Now, A.A – 5A = – 7I
Multiplying by A–1 on both sides, we get
A.A(A–1) – 5AA–1 = – 7IA–1
$$\Rarr\space\text{AI - 5I} =-7\text{A}^{\normalsize-1}$$
(using AA–1 = I and IA–1 = A–1)
$$\Rarr\space\text{A}^{\normalsize-1}=\frac{1}{7}(\text{A - 5I})\\\Rarr\space\text{A}^{\normalsize-1}=\frac{1}{7}(\text{5I - A})\\=\frac{1}{7}\bigg(\begin{bmatrix}5 &0\\0 &5\end{bmatrix} -\begin{bmatrix}3 &1\\\normalsize-1 &2\end{bmatrix}\bigg)\\=\frac{1}{7}\begin{bmatrix}2 &\normalsize-1\\1 &3\end{bmatrix}\\\therefore\space\text{A}^{\normalsize-1}=\frac{1}{7}\begin{bmatrix}2 &\normalsize-1\\1 &3\end{bmatrix}$$
Note : When we multiply by A–1 in the given equation, it is necessary that |A| ≠ 0. If |A|= 0, then A–1 does not exist.
$$\textbf{14. For the matrix A =}\begin{bmatrix}\textbf{3} &\textbf{2}\\\textbf{1} &\textbf{1}\end{bmatrix}\textbf{,}$$
find the numbers a and b such that A2 + aA + bI = O.
$$\textbf{Sol.\space}\text{Given, A} =\begin{bmatrix}3 &2\\1 &1\end{bmatrix}\\\text{A}^{2} =\text{AA} =\begin{bmatrix}3 &2\\1 &1\end{bmatrix}\begin{bmatrix}3 &2\\1 &1\end{bmatrix}\\=\begin{bmatrix}9+2 &6+2\\3+1 &2+1\end{bmatrix} =\begin{bmatrix}11 &8\\4 &3\end{bmatrix}$$
Given, A2 + aA = bI = O
On putting the values of A2, A and I, we get
$$\begin{bmatrix}11 &8\\4 &3\end{bmatrix}+a\begin{bmatrix}3 &2\\1 &1\end{bmatrix}+\\b\begin{bmatrix}1 &0\\0 &1\end{bmatrix}=\text{O}\\\Rarr\space\begin{bmatrix}11+3a+b &8+2a+b\\4+a+0 &3+a+b\end{bmatrix} =\text{O}\\\Rarr\space\begin{bmatrix}11+3a+b &8+2a\\4+a &3+a+b\end{bmatrix}\\=\begin{bmatrix}0 &0\\0 &0\end{bmatrix}$$
If two matrices are equal, then their corresponding elements are equal.
$$\Rarr\space\text{11+3a + b} =0\space\text{...(i)}$$
8 + 2a = 0 ...(ii)
4 + a = 0 ...(iii)
and 3 + a + b = 0 ...(iv)
Solving equations (iii) and (iv), we get
$$\text{4 + a = 0}\space\Rarr\space\text{a = -4}\\\text{and\space 3+a+b =0}\\\Rarr\space 3-4+b=0\\\Rarr\space b=1$$
Thus, a = – 4 and b = 1
$$\textbf{15.\space For the matrix A=}\begin{bmatrix}\textbf{1} &\textbf{1} &\textbf{1}\\\textbf{1} &\textbf{2} &\textbf{\normalsize-3}\\\textbf{2} &\textbf{\normalsize-1} &\textbf{3}\end{bmatrix}\textbf{.}$$
Show that A3 – 6A2 + 5A + 11I = O. Hence, find A–1.
$$\textbf{Sol.\space} \text{Given,\space}\text{A} = \begin{bmatrix}1 &1 &1\\1 &2 &\normalsize-3\\2 &\normalsize-1 &3\end{bmatrix}\\\therefore\space\text{A}^{2} =\text{AA}=\\\begin{bmatrix}1 &1 &1\\1 &2 &\normalsize-3\\2 &\normalsize-1 &3\end{bmatrix}\begin{bmatrix}1 &1 &1\\1 &2 &\normalsize-3\\2 &\normalsize-1 &3\end{bmatrix}\\=\begin{bmatrix}1+1+2 &1+2-1 &1-3+3\\1+2-6 &1+4+3 &1-6-9\\2-1+6 &2-2-3 &2+3+9\end{bmatrix}\\=\begin{bmatrix}4 &2 &1\\-3 &8 &\normalsize-14\\7 &\normalsize-3 &14\end{bmatrix}$$
and A3 = A2A =
$$\begin{bmatrix}4 &2 &1\\\normalsize-3 &8 &-14\\7 &\normalsize-3 &14\end{bmatrix}\begin{bmatrix}1 &1 &1\\1 &2 &\normalsize-3\\2 &\normalsize-1 &3\end{bmatrix}\\=\begin{bmatrix}4+2+2 &4+4-1 &4-6+3\\-3+8-28 &-3+16+14 &-3-24-42\\7-3+28 &7-6-14 &7+9+42\end{bmatrix}\\=\begin{bmatrix}8 &7 &1\\-23 &27 &-69\\ 32 &-13 &58\end{bmatrix}$$
∴ A3 – 6A2 + 5A + 11I
$$=\begin{bmatrix}8 &7 &1\\\normalsize-23 &27 &-69\\32 &-13 &58\end{bmatrix}-\begin{bmatrix}24 &12 &6\\\normalsize-18 &48 &-84\\42 &-18 &84\end{bmatrix}+\\\begin{bmatrix}5 &5 &5\\5 &10 &\normalsize-5\\10 &\normalsize-5 &15\end{bmatrix}+\begin{bmatrix}11 &0 &0\\0 &11 &0\\0 &0 &11\end{bmatrix}\\$$
$$=\\\begin{bmatrix}8-24+5+11 &7-12+5+0 \\&1-6+5+0\\-23+18+5+0 &27-48+10+11 \\&-69+84-15+0\\32-42+10+0 &-13+18-5+0 \\&58-84+15+11\end{bmatrix}$$
$$=\begin{bmatrix}0 &0 &0\\0 &0 &0\\0 &0 &0\end{bmatrix} = 0$$
Now, A3 – 6A2 + 5A + 11I = O
⇒ (AAA)A–1 – 6(AA)A–1 + 5AA–1 + 11IA–1 = O
(Pre-multiplying by A–1 as |A| ≠ 0)
$$\begin{bmatrix}\because\space |\text{A}| =\begin{vmatrix}1 &1 &1\\1 &2 &\normalsize-3\\2 &\normalsize-1 &3\end{vmatrix}\\= 1(6 – 3) – 1(3 + 6) + 1(– 1 – 4)\\=3-9-5=-11\neq 0\end{bmatrix}\\\Rarr\space\text{AA}(\text{AA}^{\normalsize-1})-6\text{A}(\text{AA}^{\normalsize-1}) + 5(\text{AA}^{\normalsize-1})+\\11(1\text{A}^{\normalsize-1}) =\text{O}\\\Rarr\space\text{AAI - 6AI + 5I + 11A}^{\normalsize-1} =\text{O}$$
(using AA–1 = I and IA–1 = A–1)
$$\Rarr\space\text{A}^{2}-6\text{A}+5\text{I} =-11\space\text{A}^{\normalsize-1}$$
(using AAI = A2 and AI = A)
$$\Rarr\space\text{A}^{\normalsize-1}=-\frac{1}{11}(\text{A}^{2}-6\text{A}+5\text{I})\\\Rarr\space\text{A}^{\normalsize-1}=\frac{1}{11}(-\text{A}^{2}+6\text{A}-5\text{I})\\=\frac{1}{11}\begin{Bmatrix}-\begin{bmatrix}4 &2 &1\\\normalsize-3 &8 &\normalsize-14\\7 &\normalsize-3 &\normalsize14\end{bmatrix} +\\ 6\begin{bmatrix}1 &1 &1\\1 &2 &\normalsize-3\\ 2 &\normalsize-1 &3\end{bmatrix}-5\begin{bmatrix}1 &0 &0\\0 &1 &0\\0 &0 &1\end{bmatrix}\end{Bmatrix}\\=\frac{1}{11}\begin{Bmatrix}\begin{bmatrix}\normalsize-4 &\normalsize-2 &\normalsize-1\\3 &\normalsize-8 &\normalsize14\\\normalsize-7 &3 &\normalsize-14\end{bmatrix} + \begin{bmatrix}6 &6 &6\\6 &12 &-18\\12 &\normalsize-6 &18\end{bmatrix}\\-\begin{bmatrix}5 &0 &0\\0 &5 &0\\0 &0 &5\end{bmatrix}\end{Bmatrix}$$
$$=\frac{1}{11}\begin{bmatrix}-4+6-5 &\normalsize-2+6+0\\& -1+6-0\\3+6-0 &-8+12-5 \\&14-18-0\\\normalsize-7+12-0 &3-6+0\\ &-14+18-5\end{bmatrix}\\=\frac{1}{11}\begin{bmatrix}\normalsize-3 &4 &5\\9 &\normalsize-1 &\normalsize-4\\5 &\normalsize-3 &\normalsize-1\end{bmatrix}$$
$$\textbf{16.\space}\textbf{If A} =\begin{bmatrix}\textbf{2} &\textbf{\normalsize-1} &\textbf{1}\\\textbf{\normalsize-1} &\textbf{2} &\textbf{\normalsize-1}\\\textbf{1} &\textbf{\normalsize-1} &\textbf{2}\end{bmatrix}$$
verify that A3 – 6A2 + 9A – 4I = O.
Hence, find A–1.
$$\textbf{Sol.\space}\text{Given A =}\begin{bmatrix}2 &\normalsize-1 &1\\\normalsize-1 &2 &\normalsize-1\\1 &\normalsize-1 &2\end{bmatrix}\\\therefore\space\text{A}^{2} =\text{AA}\\\begin{bmatrix}2 &\normalsize-1 &1\\\normalsize-1 &2 &\normalsize-1\\1 &\normalsize-1 &2\end{bmatrix}\begin{bmatrix}2 &\normalsize-1 &1\\\normalsize-1 &2 &\normalsize-1\\1 &\normalsize-1 &2\end{bmatrix}\\=\\\begin{bmatrix}4+1+1 &-2-2-1 &2+1+2\\-2-2-1 &1+4+1 &-1-2-2\\2+1+2 &\normalsize-1-2-2 &1+1+4\end{bmatrix}$$
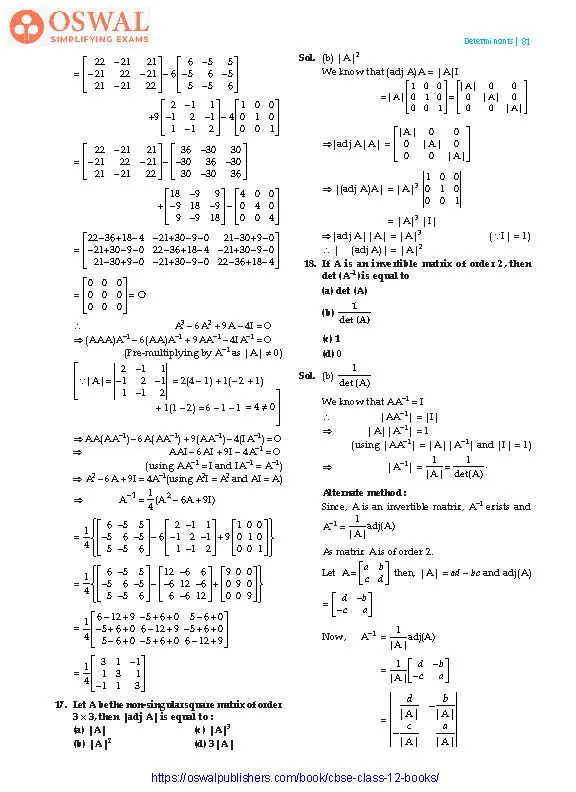
$$= \begin{bmatrix}6 &\normalsize-5 &5\\\normalsize-5 &6 &\normalsize-5\\5 &\normalsize-5 &6\end{bmatrix}\\\text{and\space A}^{3} = \text{A}^{2}A =\\\begin{bmatrix}6 &\normalsize-5 &5\\\normalsize-5 &6 &\normalsize-5\\5 &\normalsize-5 &6\end{bmatrix}\begin{bmatrix}2 &\normalsize-1 &1\\\normalsize-1 &2 &\normalsize-1\\1 &\normalsize-1 &2\end{bmatrix}\\=\\\begin{bmatrix}12+5+5 &-6-10-5\\&6+5+10\\-10-6-5 &5+12+5 \\&-5-6-10\\10+5+6 &-5-10-6\\&5+5+12\end{bmatrix}$$
$$=\space\begin{bmatrix}22 &\normalsize-21 &21\\\normalsize-21 &22 &\normalsize-21\\21 &\normalsize-21 &22\end{bmatrix}$$
∴ A3 – 6A2 + 9A – 4I
$$=\\\begin{bmatrix}22 &-21 &21\\\normalsize-21 &22 &\normalsize-21\\22 &\normalsize-21 &22\end{bmatrix}-6\begin{bmatrix}6 &\normalsize-5 &5\\\normalsize-5 &6&\normalsize-5\\5 &\normalsize-5 &6\end{bmatrix}\\+9\begin{bmatrix}2 &\normalsize-1 &1\\\normalsize-1 &2 &\normalsize-1\\1&\normalsize-1 &2\end{bmatrix}-4\begin{bmatrix}1 &0 &0\\0 &1 &0\\0 &0 &1\end{bmatrix}\\=\\\begin{bmatrix}22 &\normalsize-21 &21\\\normalsize-21 &22 &\normalsize-21\\21 &\normalsize-21 &22\end{bmatrix}-\begin{bmatrix}36 &\normalsize-30 &30\\\normalsize-30 &36 &\normalsize-30\\30 &\normalsize-30 &36\end{bmatrix}\\ +\begin{bmatrix}18 &\normalsize-9 &9\\\normalsize-9 &18 &\normalsize-9\\9 &\normalsize-9 &18\end{bmatrix} - \begin{bmatrix}4 &0 &0\\0 &4 &0\\0 &0 &4\end{bmatrix}$$
$$=\\\begin{bmatrix}22-36+18-4 &-21+30-9-0 \\&21-30+9-0\\-21+30-9-0 &22-36+18-4\\&-21+30-9-0\\21-30+9-0 &-21+30-9-0 \\&22-36+18-4\end{bmatrix}\\=\begin{bmatrix}0 &0 &0\\0 &0 &0\\0 &0 &0\end{bmatrix} =\text{O}$$
∴ A3 – 6A2 + 9A – 4I = O
⇒ (AAA)A–1 – 6(AA)A–1 + 9AA–1 – 4IA–1 = O
(Pre-multiplying by A–1 as |A| ≠ 0)
$$\begin{bmatrix}∵ |\text{A}| =\begin{vmatrix}2 &\normalsize-1 &1\\\normalsize-1 &2 &\normalsize-1\\1 &\normalsize-1 &2\end{vmatrix}\\= 2(4-1) +1(-2+1)\\+1(1-2) =6-1-1\\= 4\neq0\end{bmatrix}$$
$$\Rarr\space\text{AA}(\text{AA}^{\normalsize-1})-6\text{A}(\text{AA}^{\normalsize-1})+\\9(\text{AA}^{\normalsize-1})-4(\text{IA}^{\normalsize-1}) = \text{O}\\\Rarr\space\text{AAI – 6AI + 9I – 4A}^{\normalsize–1} = \text{O}$$
(using AA–1 = I and IA–1 = A–1)
$$\Rarr\space\text{A}^{2}-6A+9I = 4A^{\normalsize-1}$$
(using A2I = A2 and AI = A)
$$\Rarr\space\text{A}^{\normalsize-1} =\frac{1}{4}(\text{A}^{2}-6\text{A}+9\text{I})\\=\frac{1}{4}\begin{Bmatrix}\begin{bmatrix}6 &\normalsize-5 &5 \\\normalsize-5 &6 &\normalsize-5\\5 &\normalsize-5 &6\end{bmatrix}-\\6\begin{bmatrix}2 &\normalsize-1 &1\\\normalsize-1 &2 &\normalsize-1\\1 &\normalsize-1 &2\end{bmatrix}+9\begin{bmatrix}1 &0 &0\\0 &1 &0\\0 &0 &1\end{bmatrix}\end{Bmatrix}\\=\frac{1}{4}\begin{Bmatrix}\begin{bmatrix}6 &\normalsize-5 &5\\\normalsize-5 &6 &\normalsize-5\\5 &\normalsize-5 &6\end{bmatrix}-\begin{bmatrix}12 &\normalsize-6&6\\\normalsize-6 &12 &\normalsize-6\\6 &-6 &12\end{bmatrix}\\+\begin{bmatrix}9 &0 &0\\0 &9 &0\\0 &0 &9\end{bmatrix}\end{Bmatrix}$$
$$=\frac{1}{4}\begin{bmatrix}6-12+9 &-5+6+0 \\&5-6+0\\-5+6+0 &6-12+9\\&-5+6+0\\5-6+0 &-5+6+0 \\&6-12+9\end{bmatrix}\\=\frac{1}{4}\begin{bmatrix}3 &1 &\normalsize-1\\1 &3 &1\\\normalsize-1 &1 &3\end{bmatrix}$$
17. Let A be the non-singular square matrix of order 3 × 3, then |adj A| is equal to :
(a) |A|
(b) |A|2
(c) |A|3
(d) 3|A|
Sol. (b) |A|2
We know that (adj A)A = |A|I
$$=|\text{A}|\begin{bmatrix}1 &0 &0\\0 &1 &0\\0 &0 &1\end{bmatrix} =\begin{bmatrix}|\text{A}| &0 &0\\0 &\text{|A|} &0\\0 &0 &|\text{A}|\end{bmatrix}\\\Rarr\space|\text{adj A}||\text{A}| =\\\begin{bmatrix}|\text{A}| &0 &0\\0 &|\text{A}| &0\\0 &0 &|\text{A}|\end{bmatrix}\\\Rarr\space|\text{(adj A) A}| =\\|\text{A}|^{3}\begin{vmatrix}1 &0 &0\\0 &1 &0\\0 &0 &1\end{vmatrix}$$
= |A|3 |I|
$$\Rarr\space\text{adj A}|\text{A}| =|\text{A}|^{3}$$
(∵ I| = 1)
∴ |(adj A)| = |A|2
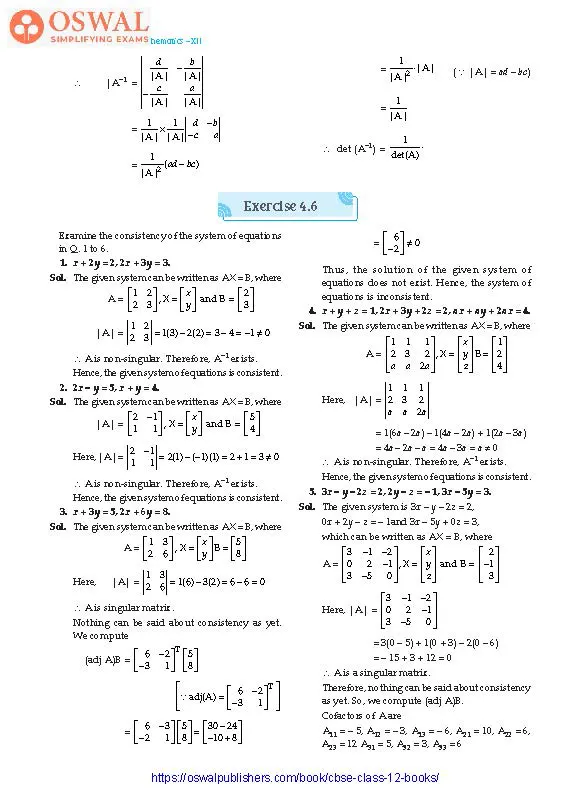
18. If A is an invertible matrix of order 2, then det (A–1) is equal to
(a) det (A)
$$\textbf{(b)}\space\frac{1}{\textbf{det}\textbf{(A)}}$$
(c) 1
(d) 0
$$\textbf{Sol.\space}\space(b)\space\frac{1}{\text{det}(\text{A})}$$
We know that AA–1 = I
∴ |AA–1| = |I|
⇒ |A||A–1| = 1
(using |AA–1| = |A||A–1| and |I| = 1)
$$\Rarr\space|\text{A}^{\normalsize-1}|=\frac{1}{|\text{A}|}=\frac{1}{\text{det}(A)}.$$
Alternate method :
Since, A is an invertible matrix, A–1 exists and
$$\text{A}^{\normalsize-1} =\frac{1}{|\text{A}|}\space\text{adj(A)}$$
As matrix A is of order 2.
$$\text{Let A =}\begin{bmatrix}a &b\\c &d\end{bmatrix}\space\text{then,}\\\text{|A|} = ad - bc\space\text{and}\space\text{adj}(A)\\=\begin{bmatrix}d &\normalsize-b\\\normalsize-c &a\end{bmatrix}\\\text{Now,\space A}^{\normalsize-1} =\frac{1}{|\text{A}|}\text{adj(A)}\\=\frac{1}{|\text{A}|}\begin{bmatrix}d &\normalsize-b\\\normalsize-c &a\end{bmatrix}\\=\begin{vmatrix}\frac{d}{|\text{A}|} &\frac{b}{|\text{A}|}\\-\frac{c}{|\text{A}|} &\frac{a}{|\text{A}|}\end{vmatrix}\\\therefore\space|\text A|^{\normalsize-1} =\begin{vmatrix}\frac{d}{|\text{A}|} &-\frac{b}{|\text{A}|}\\-\frac{c}{|\text{A}|} &\frac{a}{|\text{A}|}\end{vmatrix}$$
$$=\frac{1}{|\text{A}|}×\frac{1}{|\text{A}|}\begin{vmatrix}d &-b\\-c &a\end{vmatrix}\\=\frac{1}{|\text{A}|^{2}}(ad - bc)\\=\frac{1}{|\text{A}|^{2}}.|\text{A}|$$
(∵ |A| = ad – bc)
$$=\frac{1}{|\text{A}|}\\\therefore\space\text{del(A} ^{\normalsize-1}) =\frac{1}{\text{det (A)}}.$$
