NCERT Solutions for Class 12 Maths Chapter 4 Determinants - Miscellaneous Exercise
Access Exercises of Class 12 Maths Chapter 4 –Determinants
Exercise 4.1 Solutions: 8 Questions (2 Long, 5 Short Answers, 1 MCQ)
Exercise 4.2 Solutions: 16 Questions(7 Long, 7 Short, 2 MCQs)
Exercise 4.3 Solutions: 5 Questions ( 4 Short Answers, 1 MCQ)
Exercise 4.4 Solutions: 5 Questions (4 Long, 1 MCQ)
Exercise 4.5 Solutions: 18 Questions (11 Long, 5 Short, 2 MCQs)
Exercise 4.6 Solutions: 16 Questions (13 Long, 3 Short)
Miscellaneous Exercise Solutions: 19 Questions (15 Long, 1 Short, 3 MCQs)
Miscellaneous Exercise
1. Prove that the determinant
$$\begin{bmatrix}\textbf{x} &\textbf{sin}\space\theta &\textbf{cos}\space\theta\\\textbf{\normalsize-sin}\space\theta &\textbf{\normalsize-x} &\textbf{1}\\\textbf{cos}\space\theta &\textbf{1} &\textbf{x}\end{bmatrix}$$
is independent of θ.
$$\textbf{Sol.\space}\text{Let A = }\begin{vmatrix}\textbf{x} &\textbf{sin}\space\theta &\textbf{cos}\space\theta\\-\textbf{sin}\space\theta &\textbf{\normalsize-x} &1\\\textbf{cos}\space\theta &\textbf{1} &\textbf{x}\end{vmatrix}$$
Expanding to corresponding first row, we get
$$\text{A} = x\begin{vmatrix}-x &1\\1 &x\end{vmatrix}-\text{sin}\space\theta\begin{vmatrix}-\text{sin}\space\theta &1\\\text{cos}\space\theta &x\end{vmatrix}\\+ \text{cos}\space\theta\begin{vmatrix}-\text{sin}\space\theta &\normalsize-x\\\text{cos}\space\theta &1\end{vmatrix}$$
= x (– x2 – 1) – sin θ(– x sin θ – cos θ) + cos θ(– sin θ + x cos θ)
= – x3 – x + x sin2θ + sin θ cos θ – sin θ cos θ + x cos2 θ
= – x3 – x + x(sin2 θ + cos2 θ)
= – x3 – x + x (∵ sin2 θ + cos2 θ = 1)
= – x3.
Hence, A is independent of θ.
2. Without expanding the determinant, prove that
$$\begin{vmatrix}\textbf{a} &\textbf{a}^{2} &\textbf{bc}\\\textbf{b} &\textbf{b}^{2} &\textbf{ca}\\\textbf{c} &\textbf{c}^{2} &\textbf{ab}\end{vmatrix}\textbf{=}\begin{vmatrix}\textbf{1} &\textbf{a}^{\textbf{2}} &\textbf{a}^{\textbf{3}}\\\textbf{1} &\textbf{b}^{2} &\textbf{b}^{3}\\1 &\textbf{c}^{2} &\textbf{c}^{3}\end{vmatrix}\\\textbf{Sol.\space}\text{LHS = }\begin{vmatrix}a &a^{2} &bc\\b &b^{2} &ca\\c &c^{2} &ab\end{vmatrix}$$
Operating R1 → aR1, R2 → bR2 and R3 → cR3 we obtain
$$=\frac{1}{abc}\begin{vmatrix}a^{2} &a^{3} &abc\\b^{2} &b^{3} &abc\\c^{2} &c^{3} &abc\end{vmatrix}\\=\frac{1}{abc}×abc\begin{vmatrix}a^{2} &a^{3} &1\\b^{2} &b^{3} &1\\c^{2} &c^{3} &1\end{vmatrix}$$
[Taking out factor abc from C3]
$$=(\normalsize-1)^{2}\begin{vmatrix}1 &a^{2} &a^{3}\\1 &b^{2} &b^{3}\\1 &c^{2} &c^{3}\end{vmatrix}$$
(using C1 ↔ C3 and C2 ↔ C3)
$$=\begin{vmatrix}1 &a^{2} &a^{3}\\1 &b^{2} &b^{3}\\ 1& c^{2} & c^{3}\end{vmatrix}=\text{RHS}$$
Note : When we multiply any row or column of a determinant by any constant k then it is necessary that determinant divides by k.
$$\textbf{3.\space}\textbf{Evaluate}\\\space\begin{vmatrix}\textbf{cos}\alpha\textbf{cos}\beta &\textbf{cos}\alpha\textbf{sin}\beta&-\textbf{sin\space}\alpha\\\textbf{-sin}\space\beta &\textbf{cos}\space\beta &\textbf{0}\\\textbf{sin}\space\alpha\textbf{cos}\space\beta &\textbf{sin}\space\alpha\textbf{sin}\space\beta &\textbf{cos}\space\alpha\end{vmatrix}$$
Sol. Given, determinant
$$=\begin{vmatrix}\text{cos}\alpha\text{cos}\beta &\text{cos}\alpha\text{sin}\beta &-\text{sin}\space\alpha\\-\text{sin}\space\beta &\text{cos}\space\beta &0\\\text{sin}\alpha\text{cos}\beta &\text{sin}\space\alpha\text{sin}\beta &\text{cos}\space\alpha\end{vmatrix}$$
Expanding corresponding to R1, we get
= cos α cos β (cos α cos β – 0) – cos α sin β(– cos α sin β – 0) – sin α(– sin2 β sin α – cos2 β sin α)
= cos2 α cos2 β + cos2 α sin2 β + sin2 α (sin2 β + cos2 β)
= cos2 α (cos2 β + sin2 β) + sin2 α (sin2 β + cos2 β)
= cos2 α (1) sin2 α (1) = cos2 α + sin2 α = 1.
[∵ sin2 θ + cos2 θ = 1]
4. If a, b and c are real numbers and
$$\Delta =\begin{vmatrix}\textbf{b+c} &\textbf{c+a} &\textbf{a+b}\\\textbf{c+a} &\textbf{a+b} &\textbf{b+c}\\\textbf{a+b} &\textbf{b+c} &\textbf{c+a}\end{vmatrix} \textbf{= 0.}$$
Show that either
a + b + c = 0 or a = b = c.
$$\textbf{Sol.\space}\text{Given,\space}\Delta =\begin{vmatrix}b+c &c+a &a+b\\\text{c+a} &a+b &b+c\\a+b &b+c &c+a\end{vmatrix}\\=\begin{vmatrix}2(a+b+c) &c+a &a+b\\2(a+b+c) &a+b &b+c\\2(a+b+c) &b+c &c+a\end{vmatrix}$$
(using C1 → C1 + C2 + C3)
$$= 2(a+b+c)\begin{vmatrix}1 &c+a &a+b\\1 &a+b &b+c\\1 &b+c &c+a\end{vmatrix}$$
(Taking out factor 2(a + b) + c) from C1)
$$= 2(a+b+c)\begin{vmatrix}1 &c+a &a+b\\0 &b-c &c-a\\0 &b-a &c-b\end{vmatrix}$$
(using R2 → R2 – R1, R2 → R3 – R1)
By expanding along C1, we get
= 2(a + b + c)[(b – c)(c – b) – (b – a)(c – a)]
= 2(a + b + c) [bc – c2 – b2 + bc – bc + ac + ab – a2]
= 2(a + b + c)[ab + bc + ca – a2 – b2 – c2]
It is given that Δ = 0,
∴ (a + b + c) × 2[ab + bc + ca – a2 – b2 – c2] = 0
⇒ Either (a + b + c) = 0
or 2[ab + bc + ca – a2 – b2 – c2] = 0
If 2[ab + bc + ca – a2 – b2 – c2] = 0
⇒ –(2a2 + 2b2 + 2c2 – 2ab – 2bc – 2ca) = 0
[Taking negative sign common]
⇒ 2a2 + 2b2 + 2c2 – 2ab – 2bc – 2ca = 0
⇒ (a2 + b2 – 2ab) + (b2 + c2 – 2bc) + (c2 + a2 – 2ca) = 0
⇒ (a – b)2 + (b – c)2 + (c – a2)= 0
[∵ (x – y)2 = x2 + y2 – 2xy]
⇒ (a – b)2 = (b – c)2 = (c – a)2 = 0
[∵ (a – b)2, (b – c)2, (c – a)2 are positive]
⇒ (a – b)2 = (b – c)2 = (c – a)2 = 0
⇒ a = b = c
Hence, if Δ = 0, then either a + b + c = 0 or a = b = c.
5. Solve the equation, if
$$\begin{vmatrix}\textbf{x+a} &\textbf{x} &\textbf{x}\\\textbf{x} &\textbf{x+a} &\textbf{x}\\\textbf{x} &\textbf{x} &\textbf{x+a}\end{vmatrix}\\\textbf{= 0, a}\neq\textbf{0,}$$
$$\textbf{Sol.\space}\text{Given,\space}\begin{vmatrix}x+a &x &x\\x &x+a &x\\x &x &x+a\end{vmatrix}\\= 0\\\Rarr\space\begin{vmatrix}3x+a &3x+a &3x+a\\ x &x+a &x\\x &x &x+a\end{vmatrix}=0$$
(using R1 → R1 + R2 + R3)
$$\Rarr\space(3x+a)\begin{vmatrix}1 &1 &1\\x &x+a &x\\x &x &x+a\end{vmatrix}= 0$$
(Taking out (3x + a) from R1)
$$\Rarr\space(3x+a)\begin{vmatrix}1 &0 &0\\x &a &0\\x &0 &a\end{vmatrix}=0,$$
(using C2 → C2 – C1 and C3 → C3 – C1)
Expanding corresponding to R1 (first row), we get
(3x + a)[1(a × a – 0)] = 0
⇒ a2(3x + a) = 0
But a ≠ 0 (given). Therefore, 3x + a = 0
$$\Rarr\space x =-\frac{a}{3}.$$
6. Prove that
$$\begin{vmatrix}\textbf{a}^{\textbf{2}} &\textbf{bc} &\textbf{ac+c}^{\textbf{2}}\\\textbf{a}^{2}\textbf{+ ab} &\textbf{b}^{\textbf{2}} &\textbf{ac}\\\textbf{ab} &\textbf{b}^{\textbf{2}}\textbf{+bc} &\textbf{c}^{\textbf{2}}\end{vmatrix}$$= 4a2b2c2.
$$\textbf{Sol.\space}\text{LHS =}\space\begin{vmatrix}a^{2} &bc &ac+c^{2}\\a^{2}+ab &b^{2} &ac\\ab &b^{2}+bc &c^{2}\end{vmatrix}\\= abc\begin{vmatrix}a &c &a+c\\a+b &b &a\\b &b+c &c\end{vmatrix}$$
(Take out a from C1, b fom C2 and c from C3)
$$=abc\begin{vmatrix}0 &c &a+c\\2b &b &a\\2b &b+c &c\end{vmatrix}$$
(using C1 → C1 + C2 – C3)
$$=\space abc\begin{vmatrix}0 &c &a+c\\0 &\normalsize-c &a-c\\2b &b+c &c\end{vmatrix}$$
$$\lbrack\text{using}\space\text{R}_{2}\xrightarrow{}\text{R}_{2}-\text{R}_{3}\rbrack$$
Expanding along C1, we get
= (abc) [(2b){c(a – c) + c(a + c)}]
= 2(ab2c)(2ac) = 4a2b2c2 = RHS
Hence proved.
$$\textbf{7.\space If A}^{\normalsize-1} =\begin{bmatrix}\textbf{3} &\textbf{\normalsize-1} &\textbf{\normalsize1}\\\textbf{\normalsize-15} &\textbf{6} &\textbf{\normalsize-5}\\\textbf{5} &\textbf{\normalsize-2} &\textbf{2}\end{bmatrix}\\\textbf{and B = }\begin{bmatrix}\textbf{1} &\textbf{2} &\textbf{-2}\\\textbf{-1} &\textbf{3} &\textbf{0}\\\textbf{0} &\textbf{-2} &\textbf{1}\end{bmatrix}\textbf{,}\\\textbf{find\space (AB)}^{\normalsize-1}\textbf{.}$$
Sol. We know that (AB)–1 = B–1A–1 and A–1 is known, therefore, we proceed to find B–1.
$$\text{Here,\space |B| =}\begin{vmatrix}1 &2 &\normalsize-2\\\normalsize-1 &3 &0\\0 &\normalsize-2 &1\end{vmatrix}$$
= 1(3 – 0) – 2(– 1 – 0) – 2(2 – 0)
= 3 + 2 – 4 = 1 ≠ 0
∴ B–1 exists. Cofactors of B are
B11 = (3 – 0) = 3, B12 = – (– 1 – 0) = 1, B13 = (2 – 0) = 2,
B21 = – (2 – 4) = 2,B22 = 1 – 0 = 1, B23 = – (– 2 – 0) = 2,
B31 = (0 + 6) = 6, B32 = – (0 – 2)= 2, B33 = 3 + 2 = 5,
$$\therefore\space\text{adj B} =\begin{bmatrix}3 &1 &2\\2 &1 &2\\6 &2 &5\end{bmatrix}^{\text{T}}=\begin{bmatrix}3 &2 &6\\1 &1 &2\\2 &2 &5\end{bmatrix}\\\therefore\space\text{B}^{\normalsize-1}=\frac{1}{|\text{B}|}\space\text{adj(B)}\\=\frac{1}{1}\begin{bmatrix}3 &2 &6\\1 &1 &2\\2 &2 &5\end{bmatrix}=\begin{bmatrix}3 &2 &6\\1 &1 &2\\2 &2 &5\end{bmatrix}$$
Now, (AB)–1 = B–1A–1
$$=\begin{bmatrix}3 &2 &6\\1 &1 &2\\2 &2 &5\end{bmatrix}\begin{bmatrix}3 &\normalsize-1 &1\\\normalsize-15 &6 &\normalsize-5\\5 &\normalsize-2 &2\end{bmatrix}\\=\\\begin{bmatrix}9-30+30 &-3+12-12 &3-10+12\\3-15+10 &-1+6-4 &1-5+4\\6-30+25 &-2+12-10 &2-10+10\end{bmatrix}\\=\begin{bmatrix}9 &\normalsize-3 &5\\\normalsize-2 &1 &0\\1 &0 &2\end{bmatrix}.$$
$$\textbf{8.\space Let A = }\begin{bmatrix}\textbf{1} &\textbf{2} &\textbf{1}\\\textbf{2} &\textbf{3} &\textbf{1}\\\textbf{1} &\textbf{1} &\textbf{5}\end{bmatrix}\textbf{,}\space\textbf{verify that}$$
(a) [adj A]–1 = adj (A–1)
(b) (A–1)–1 = A.
$$\textbf{Sol.\space}\text{Given, A = }\begin{vmatrix}1 &2 &1\\2 &3 &1\\1 &1 &5\end{vmatrix}$$
|A| = 1(15 – 1) – 2(10 – 1) + 1(2 – 3)
= 14 – 18 – 1
= – 5 ≠ 0
Hence, its inverse exists.
Cofactors of A
A11 = (– 1)1+1 (15 – 1) = 14
A12 = (– 1)1+2 (10 – 1) = –9
A13 = (– 1)1+3 (2 – 3) = –1
A21 = (– 1)2+1 (10 – 1) = –9
A22 = (– 1)2+2 (5 – 1) = 4
A23 = (– 1)2+3 (1 – 2) = 1
A31 = (– 1)3+1 (2 – 3) = –1
A32 = (– 1)3+2 (1 – 2) = –1
A33 = (– 1)3+3 (3 – 4) = –1
$$\text{adj(A)} = \begin{bmatrix}14 &\normalsize-9 &\normalsize-1\\\normalsize-9 &4 &1\\\normalsize-1 &1 &\normalsize-1\end{bmatrix}\\\therefore\space\text{A}^{\normalsize-1}=\frac{1}{|\text{A}|}\space\text{(adj A)}\\=\frac{1}{\normalsize-5}\begin{bmatrix}14 &\normalsize-9 &\normalsize-1\\\normalsize-9 &4 &1\\\normalsize-1 &1 &\normalsize-1\end{bmatrix}\\\text{(a) Here, adj (A) =}\\\begin{bmatrix}14 &\normalsize-9 &\normalsize-1\\\normalsize-9 &4 &1\\\normalsize-1 &1 &\normalsize-1\end{bmatrix} =\text{B(let)}$$
∴ |B| = 14 (– 4 – 1) + 9(9 + 1) – 1(– 9 + 4)
= –70 + 90 + 5 = 25
$$\therefore\space\text{B}^{\normalsize-1}=\frac{1}{|\text{B}|}\space\text{adj B}$$
C11 = –5, C12 = – 10, C13 = –5, C21 = –10, C22 = –15
C23 = –5, C31 = – 5, C32 = –5, C33 = –25
$$\text{adj B = }\begin{bmatrix}\normalsize-5 &\normalsize-10 &\normalsize-5\\\normalsize-10 &\normalsize-15 &\normalsize-5\\ \normalsize-5 &\normalsize-5 &\normalsize-25\end{bmatrix}\\\text{B}^{\normalsize-1}=\frac{1}{|\text{B}|}\space\text{adj B}\\=\frac{1}{25}\begin{bmatrix}\normalsize-5 &\normalsize-10 &\normalsize-5\\\normalsize-10 &\normalsize-15 &\normalsize-5\\\normalsize-5 &\normalsize-5 &\normalsize-25\end{bmatrix}\\=\begin{bmatrix}\frac{\normalsize-1}{5} &\frac{\normalsize-2}{5} &\frac{\normalsize-1}{5}\\\frac{\normalsize-2}{5} &\frac{\normalsize-3}{5} &\frac{\normalsize-1}{5}\\\frac{\normalsize-1}{5} &\frac{\normalsize-1}{5} &\normalsize-1\end{bmatrix}$$
$$\text{adj B = }\begin{bmatrix}\normalsize-5 &\normalsize-10 &\normalsize-5\\\normalsize-10 &\normalsize-15 &\normalsize-5\\ \normalsize-5 &\normalsize-5 &\normalsize-25\end{bmatrix}\\\text{B}^{\normalsize-1}=\frac{1}{|\text{B}|}\space\text{adj B}\\=\frac{1}{25}\begin{bmatrix}\normalsize-5 &\normalsize-10 &\normalsize-5\\\normalsize-10 &\normalsize-15 &\normalsize-5\\\normalsize-5 &\normalsize-5 &\normalsize-25\end{bmatrix}\\=\begin{bmatrix}\frac{\normalsize-1}{5} &\frac{\normalsize-2}{5} &\frac{\normalsize-1}{5}\\\frac{\normalsize-2}{5} &\frac{\normalsize-3}{5} &\frac{\normalsize-1}{5}\\\frac{\normalsize-1}{5} &\frac{\normalsize-1}{5} &\normalsize-1\end{bmatrix}$$
$$\\\text{LHS : }[\text{adj A}]^{\normalsize-1}=\\\begin{bmatrix}\frac{\normalsize-1}{15} &\frac{\normalsize-2}{5} &\frac{\normalsize-1}{5}\\\frac{\normalsize-2}{5} &\frac{\normalsize-3}{5} &\frac{\normalsize-1}{5}\\\frac{\normalsize-1}{5} &\frac{\normalsize-1}{5} &\normalsize-1\end{bmatrix}$$
RHS : adj (A–1) = adj C
$$\text{A}^{\normalsize-1} =\text{C} =\begin{bmatrix}\frac{\normalsize-14}{5} &\frac{9}{5} &\frac{1}{5}\\\frac{9}{5} &\frac{\normalsize-4}{5} &\frac{\normalsize-1}{5}\\\frac{1}{5} &\frac{\normalsize-1}{5} &\frac{1}{5}\end{bmatrix}$$
Cofactors of C
B11 = –1/5, B12 = –2/5, B13 = 1/5,
B21 = –2/5, B22 = –3/5, B23 = –1/5,
B31 = –1/5, B32 = –1/5, B33 = –1
RHS = adj C = adj (A–1) =
$$\begin{bmatrix}\frac{\normalsize-1}{5} &\frac{\normalsize -2}{5} &\frac{\normalsize-1}{5}\\\frac{\normalsize-2}{5} &\frac{\normalsize-3}{5} &\frac{\normalsize-1}{5}\\\frac{\normalsize-1}{5} &\frac{\normalsize-1}{5} &\normalsize-1\end{bmatrix}$$
Hence, LHS = RHS Hence proved.
$$\text{(b)\space (A}^{\normalsize-1})^{\normalsize-1}=\frac{1}{|\text{A}|}\space\text{(adj A}^{\normalsize-1})\\=\frac{1}{\normalsize-5}\begin{bmatrix}\frac{\normalsize-1}{5} &\frac{\normalsize-2}{5} &\frac{\normalsize-1}{5}\\\frac{\normalsize-2}{5} &\frac{\normalsize-3}{5} &\frac{\normalsize-1}{5}\\\frac{\normalsize-1}{5} &\frac{\normalsize-1}{5} &\normalsize-1\end{bmatrix}\\=\begin{bmatrix}1 &2 &1\\ 2 &3 &1\\1 &1 &3\end{bmatrix}=\text{A}\\\textbf{Hence proved.}$$
$$\textbf{9. Evaluate}\space\begin{vmatrix}\textbf{x} &\textbf{y} &\textbf{x+y}\\\textbf{y} &\textbf{x+y} &\textbf{x}\\\textbf{x+y} &\textbf{x} &\textbf{y}\end{vmatrix}\textbf{.}\\\textbf{Sol.\space}\text{Let A =}\begin{vmatrix}x &y &x+y\\y &x+y &x\\x+y &x &y\end{vmatrix}\\=\begin{vmatrix}2(x+y) &y &x+y\\2(x+y) &x+y &x\\2(x+y) &x &y\end{vmatrix}$$
(using C1 → C1 + C2 + C3)
$$=2(x+y)\begin{vmatrix}1 &y &x+y\\0 &x &\normalsize-y\\0 &x-y &-x\end{vmatrix}$$
(using R2 → R2 – R1; R3 → R3 – R1)
Expanding along R1, we get
= 2(x + y) × 1{– x2 + y(x – y)}
= 2(x + y)(– x2 + xy – y2)
= – 2(x + y)(x2 – xy + y2)
= – 2(x2 + y2)
10. Evalaute
$$\begin{vmatrix}\textbf{1} &\textbf{x} &\textbf{y}\\\textbf{1} &\textbf{x+y} &\textbf{y}\\\textbf{1} &\textbf{x} &\textbf{x+y}\end{vmatrix}\textbf{.}\\\textbf{Sol.\space}\text{Let A = }\begin{vmatrix}1 &x &y\\1 &x+y &y\\1 &x &x+y\end{vmatrix}\\=\begin{vmatrix}1 &x &y\\0 &y &0\\0 &0 &x\end{vmatrix}$$
(using R2 → R2 – R1 and R3 → R3 – R1)
Expanding along C1, we get A = 1(yx – 0) = xy
Using properties of determinants in Q. 11 to 15, prove that :
$$\textbf{11.\space}\begin{vmatrix}\alpha &\alpha^{2} &\beta+\gamma\\\beta &\beta^{2} &\gamma + \alpha\\\gamma &\gamma^{2} &\alpha+\beta\end{vmatrix}\\=(\beta - \gamma)(\gamma -\alpha)(\alpha-\beta)(\alpha+\beta+\gamma).$$
$$\textbf{Sol.\space}\text{Let A = }\begin{vmatrix}\alpha &\alpha^{2} &\beta+\gamma\\\beta &\beta^{2} &\gamma +\alpha\\\gamma &\gamma^{2} &\alpha +\beta\end{vmatrix}\\=\begin{vmatrix}\alpha &\alpha^{2} &\alpha+\beta+\gamma\\\beta&\beta^{2} &\alpha +\gamma+\beta\\\gamma &\gamma^{2} &\alpha+\beta+\gamma\end{vmatrix}$$
(using C3 → C3 + C1)
$$=(\alpha +\beta+\gamma)\begin{vmatrix}\alpha &\alpha^{2} &1\\\beta &\beta^{2} &1\\\gamma &\gamma^{2} &1\end{vmatrix}$$
(Taking out (α + β + γ) common from C1)
$$=\space(\alpha +\beta +\gamma)\begin{vmatrix}\alpha &\alpha^{2} &1\\\beta-\alpha &\beta^{2} -\alpha^{2} &0\\\gamma-\alpha &\gamma^{2}-\alpha^{2} &0\end{vmatrix}$$
(using R2 → R2 – R1 and R3 → R3 – R1)
Expanding along C3, we get
= (α + β + γ) [(β – α)(γ2 – α2) – (γ – α)(β2 – α2)]
= (α + β + γ) [(β – α)(γ – α)(γ + α) – (γ – α)(β – α)(β + α)]
= (α + β + γ)(β – α)(γ – α)[γ + α – β – α]
= (α + β + γ)(β – α)(γ – α)(γ – β)
= (α + β + γ)(α – β)(β – γ)(γ – α) = RHS.
Hence proved.
$$\textbf{12.}\space\begin{vmatrix}\textbf{x} &\textbf{x}^{2} &\textbf{1+px}^{\textbf{3}}\\\textbf{y} &\textbf{y}^{\textbf{2}} &\textbf{1+ py}^{3}\\\textbf{z} &\textbf{z}^{2} &\textbf{1 + pz}^{\textbf{3}}\end{vmatrix}$$
= (1 + pxyz)(x – y)(y – z)(z – x),
where p is any scalar.
Sol. Firstly, we write the given determinant as sum of two determinants, since all elements of C3 are expressed as sum of two terms and then apply operations in both determinant separately.
$$\text{LHS =}\begin{vmatrix}x &x^{2} &1+px^{3}\\y &y^{2} &1+py^{3}\\z &z^{2} &1+pz^{3}\end{vmatrix}\\=\begin{vmatrix}x &x^{2} &1\\y &y^{2} &1\\z &z^{2} &1\end{vmatrix} +\begin{vmatrix}x &x^{2} &px^{3}\\y &y^{2} &py^{3}\\z &z^{2} &pz^{3}\end{vmatrix}$$
In the first derterminant, interchange C1 and C3; C2 and C3 and in the second determinant take out p from C3, we get
$$(\normalsize-1)^{2}\begin{vmatrix}1 &x &x^{2}\\1 &y &y^{2}\\1 &z &z^{2}\end{vmatrix}+p\begin{vmatrix}x &x^{2} &x^{3}\\y &y^{2} &y^{3}\\z &z^{2} &z^{3}\end{vmatrix}\\=\begin{vmatrix}1 &x &x^{2}\\1 &y &y^{2}\\1 &z &z^{2}\end{vmatrix} + \text{pxyz}\begin{vmatrix}1 &x &x^{2}\\1 &y &y^{2}\\1 &z &z^{2}\end{vmatrix}$$
[Taking out x from R1, y from R2; and z from R3 in the second determinant]
$$= (1 + pxyz)\begin{vmatrix}1 &x &x^{2}\\ 1 &y &y^{2}\\1 &z &z^{2}\end{vmatrix}\\=\text{(1 + pxyz)}\begin{vmatrix}1 &x &x^{2}\\0 &y-x &y^{2}-x^{2}\\0 &z-x &z^{2}-x^{2}\end{vmatrix}$$
(using R2 → R2 – R1 and R3 → R3 – R1)
Expanding along C1, we get
= (1 + pxyz)[(y – x)(z2 – x2) – (z – x)(y2 – x2)
= (1 + pxyz)[(y – x)(z – x)(z + x) – (z – x)(y – x)(y + x)]
= (1 + pxyz)(y – x)(z – x)[(z + x) – (y + x)]
= (1 + pxyz)(y – x)(z – x)[(z + x – y – x)]
= (1 + pxyz)[(y – x)(z – x)(z – y)
= (1 + pxyz)(x – y)(y – z)(z – x)
Hence proved.
$$\textbf{13.\space}\begin{vmatrix}\textbf{3a} &\textbf{-a+b} &\textbf{-a+c}\\\textbf{-b+a} &\textbf{3b} &\textbf{-b+c}\\\textbf{-c+a} &\textbf{-c+b} &\textbf{3c}\end{vmatrix}$$
= 3(a + b + c)(ab + bc + ca).
$$\textbf{Sol.\space}\text{Let A} =\\\begin{vmatrix}3a &-a+b &-a+c\\-b+a &3b &-b+c\\-c+a &-c+b &3c\end{vmatrix}\\=\begin{vmatrix}a+b+c &-a+b &-a+c\\a+b+c &3b &-b+c\\a+b+c &-c+b &3c\end{vmatrix}$$
(using C1 → C1 + C2 + C3)
$$=(a+b+c)\begin{vmatrix}1 &-a+b &-a+c\\1 &3b &-b+c\\1 &-c+b &3c\end{vmatrix}$$
(Taking out (a + b + c) common from C1)
$$= (a +b+c)\begin{vmatrix}1 &-a+b &-a+c\\0 &2b+a &a+b\\0 &a-c &2c+a\end{vmatrix}$$
(using R2 → R2 – R1 and R3 → R3 – R1)
Expanding along C1, we get
= (a + b + c)[(2b + a)(2c + a) – (a – b)(a – c)]
= (a + b + c)[4bc + 2ab + 2ac + a2 – a2 + ac + ba – bc]
= (a + b + c)(3ab + 3bc + 3ac)
= 3(a + b + c)(ab + bc + ca)
= RHS Hence proved.
$$\textbf{14.\space}\begin{vmatrix}\textbf{1} &\textbf{1+p} &\textbf{1+p+q}\\\textbf{2} &\textbf{3+2p} &\textbf{4+3p+2q}\\\textbf{3} &\textbf{6+3}\textbf{p} &\textbf{10+6p+3q}\end{vmatrix}\\\textbf{= 1.}\\\textbf{Sol.}\space \text{LHS} = \begin{vmatrix}1 &1+p &1+p+q\\2 &3+2p &4+3p+2q\\3&6+3p &10+6p+3q\end{vmatrix}\\=\begin{vmatrix}1 &1+p &1+p+q\\0 &1 &2+p\\0 &3 &7+3p\end{vmatrix}$$
(using R2 → R2 – 2R1 and R3 → R3 – 3R1)
$$=\begin{vmatrix}1 &1+p &1+p+q\\0 &1 &2+p\\0 &0 &1\end{vmatrix}$$
(using R3 → R3 – 3R2)
Expanding along C1, we get
= 1(1 × 1 – 0) = 1 = RHS.
$$\textbf{15.\space}\begin{vmatrix}\textbf{sin}\space\alpha &\textbf{cos}\space\alpha &\textbf{cos}(\alpha+\delta)\\\textbf{sin}\space\beta &\textbf{cos}\space\beta &\textbf{cos}(\beta +\delta)\\\textbf{sin}\space\gamma &\textbf{cos}\space\gamma &\textbf{cos}\space(\gamma +\delta)\end{vmatrix}\\\textbf{= 0.}\\\textbf{Sol.\space}\text{Let A = }\\\begin{vmatrix}\text{sin}\space\alpha &\text{cos}\space\alpha &\text{cos}(\alpha +\delta)\\\text{sin}\space\beta &\text{cos}\space\beta &\text{cos}(\gamma +\delta)\\\text{sin}\space\gamma &\text{cos}\space\gamma &\text{cos}(\gamma +\delta)\end{vmatrix}\\=\begin{vmatrix}\text{sin}\space\alpha &\text{cos}\space\alpha &\text{cos}\space\alpha\text{cos}\space\delta -\text{sin}\alpha\text{sin}\space\delta\\\text{sin}\space\beta &\text{cos}\space\beta &\text{cos}\beta\text{cos}\delta - \text{sin}\beta\text{sin}\space\delta\\\text{sin}\space\gamma &\text{cos}\space\gamma &\text{cos}\gamma\text{cos}\delta -\text{sin}\gamma\text{sin}\delta\end{vmatrix}\\\lbrack\because\space\text{cos}(\text{A+B}) =\text{cos A cos B - sin A sinB}\rbrack$$
$$=\begin{vmatrix}\text{sin}\space\alpha &\text{cos}\space\alpha &\text{cos}\alpha\text{cos}\delta\\\text{sin}\space\beta &\text{cos}\space\beta &\text{cos}\beta\text{cos}\delta\\\text{sin}\gamma &\text{cos}\space\gamma &\text{cos}\gamma\text{cos}\delta\end{vmatrix} + \\\begin{vmatrix}\text{sin}\space\alpha &\text{cos}\space\alpha &-\text{sin}\space\alpha\text{sin}\delta\\\text{sin}\space\beta &\text{cos}\space\beta &-\text{sin}\beta\text{sin}\delta\\\text{sin}\space\gamma &\text{cos}\space\gamma &-\text{sin}\gamma\text{sin}\space\delta\end{vmatrix}$$
Taking common cos δ from C3 in first determinant and taking common – sin δ from C3 in second determinant.
$$=\space\text{cos}\space\delta\begin{vmatrix}\text{sin}\space\alpha &\text{cos}\space\beta &\text{cos}\space\alpha\\\text{sin}\space\beta &\text{cos}\space\beta &\text{cos}\space\beta\\\text{sin}\space\gamma &\text{cos}\space\gamma &\text{cos}\space\gamma\end{vmatrix}-\\\text{sin}\space\delta\begin{vmatrix}\text{sin}\space\alpha &\text{cos}\space\alpha & sin\space\alpha\\\text{sin}\space\beta &\text{cos}\space\beta &\text{sin}\space\beta\\\text{sin}\space\gamma &\text{cos}\space\gamma &\text{sin}\space\gamma\end{vmatrix}$$
=cos δ.0−sin δ.0 = 0
[Since, C2 and C3 in first determinant C1 and C3 in second determinant are identical so values of determinants are zero.]
Hence proved.
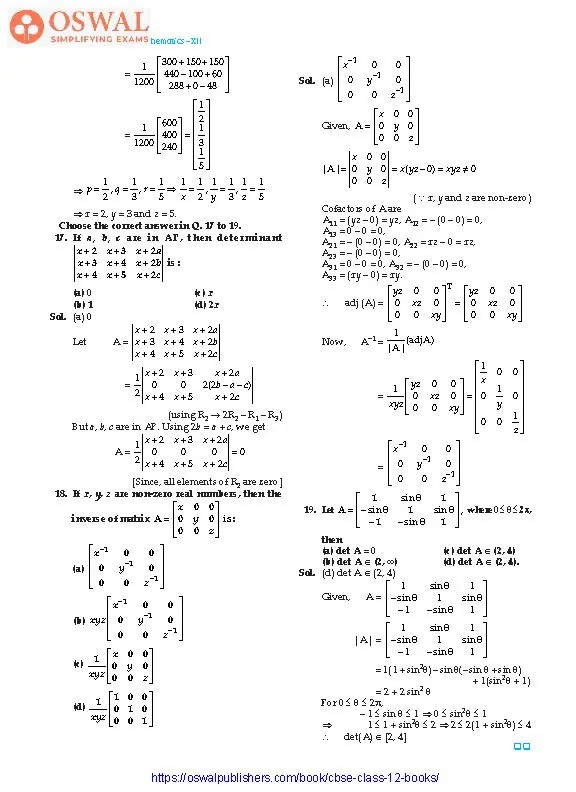
16. Solve the system of equations
$$\frac{\textbf{2}}{\textbf{x}}+\frac{\textbf{3}}{\textbf{y}}+\frac{\textbf{10}}{\textbf{z}} \textbf{= 4;}\\\frac{\textbf{4}}{\textbf{x}}-\frac{\textbf{6}}{\textbf{y}}+\frac{\textbf{5}}{\textbf{z}}\textbf{= 1}\\\textbf{and}\space\frac{\textbf{6}}{\textbf{x}} \textbf{+}\frac{\textbf{9}}{\textbf{y}}\textbf{-}\frac{\textbf{20}}{\textbf{x}} \textbf{= 2.}$$
$$\textbf{consider}\frac{\textbf{1}}{\textbf{x}} = \textbf{p},\frac{\textbf{1}}{\textbf{y}} = \textbf{q},\frac{\textbf{1}}{\textbf{z}} = \textbf{r}$$
to make a standard form of equations and use the matrix method to solve the three equations in p, q and r.
$$\textbf{Sol.\space}\text{Let}\space\frac{1}{x}=p,\frac{1}{y}= q\space\text{and}\frac{1}{z} = r,$$
then the given equations become
2p + 3q + 10r = 4, 4p – 6q + 5r = 1, 6p + 9q – 20r = 2
This system can be written as AX = B, where
$$\text{A =} \begin{bmatrix}2 &3 &10\\4 &\normalsize-6 &5\\6 &9 &\normalsize-20\end{bmatrix}\textbf{,}\\\text{X} =\begin{bmatrix}p\\q\\r\end{bmatrix},\text{B} =\begin{bmatrix}4 \\1\\2\end{bmatrix}\\\text{Here, |A| =}\begin{vmatrix}2 &3 &10\\4 &\normalsize-6 &5\\6 &9 &\normalsize-20\end{vmatrix}$$
= 2(120 – 45) – 3(– 80 – 30) + 10(36 + 36)
= 150 + 330 + 720 = 1200
Thus, A is non-singular. Therefore, its inverse exists.
Therefore, the above system is consistent and has a unique solution given by X = A–1B
Cofactors of A are
A11 = 120 – 45 = 75, A12 = – (– 80 – 30) = 110, A13 = (36 + 36) = 72
A21 = – (– 60 – 90) = 150, A22 = (– 40 – 60) = – 100, A23 = – (18 – 18) = 0,
A31 = 15 + 60 = 75, A32 = – (10 – 40) = 30, A33 = – 12 – 12 = – 24
$$\therefore\space\text{adj (A)} = \begin{vmatrix}75 &110 &72\\150 &-100 &0\\75 &30 &\normalsize-24\end{vmatrix}^{\text{T}}\\=\begin{bmatrix}75 &150 &75\\110 &-100 &30\\72 &0 &-24\end{bmatrix}\\\text{Now,\space}\text{A}^{\normalsize-1}\frac{1}{|\text{A}|}\space\text{(adj A)}\\=\frac{1}{1200}\begin{bmatrix}75 &150 &75\\110 &-100 &30\\72 &0 &\normalsize-24\end{bmatrix}\\\because\space\text{X} =\text{A}^{\normalsize-1}\text{B}$$
$$\Rarr\space\begin{bmatrix}p\\q\\r\end{bmatrix} =\frac{1}{1200}\\\begin{bmatrix}75 &150 &75\\110 &-100 &30\\72 &0 &-24\end{bmatrix}\begin{bmatrix}4 \\1 \\2\end{bmatrix}\\=\frac{1}{1200}\space\begin{bmatrix}300 + 150+150\\440-100+60\\288+0-48\end{bmatrix}\\=\frac{1}{1200}\begin{bmatrix}600\\400\\240\end{bmatrix}=\begin{bmatrix}\frac{1}{2}\\\frac{1}{3}\\\frac{1}{5}\end{bmatrix}\\\Rarr\space\text{p} =\frac{1}{2}, q =\frac{1}{3}, r =\frac{1}{5}$$
$$\Rarr\space\frac{1}{x} = \frac{1}{2}, \frac{1}{y}=\frac{1}{3}, \frac{1}{z}= \frac{1}{5}$$
⇒ x = 2, y = 3 and z = 5.
Choose the correct answer in Q. 17 to 19.
17. If a, b, c are in AP, then determinant
$$\begin{vmatrix}\textbf{x+2} &\textbf{x+3} &\textbf{x+2a}\\\textbf{x+3} &\textbf{x+4} &\textbf{x+2b}\\\textbf{x+4} &\textbf{x+5} &\textbf{x+2c}\end{vmatrix}\space\textbf{is :}$$
(a) 0
(b) 1
(c) x
(d) 2x
Sol. (a) 0
$$\text{Let A} = \begin{vmatrix}\text{x+2} &\text{x+3} &\text{x+2a}\\\text{x+3} &\text{x+4} &\text{x+2b}\\\text{x+4} &\text{x+5} &\text{x+2c}\end{vmatrix}\\=\frac{1}{2}\begin{vmatrix}x+2 &x+3 &x+2a\\0 &0 &2(2b-a-c)\\\text{x+4} &\text{x+5} &\text{x+2c}\end{vmatrix}$$
(using R2 → 2R2 – R1 – R3)
But a, b, c are in AP. Using 2b = a + c, we get
$$\text{A}=\frac{1}{2}\begin{vmatrix}x+2 &x+3 &x+2a\\0 &0 &0\\x+4 &x+5 &x+2c\end{vmatrix} = 0$$
[Since, all elements of R2 are zero]
18. If x, y, z are non-zero real numbers, then the inverse of matrix A =
$$\begin{bmatrix}\textbf{x} &\textbf{0} &\textbf{0}\\\textbf{0} &\textbf{y} &\textbf{0}\\\textbf{0} &\textbf{0} &\textbf{z}\end{bmatrix}\space\textbf{is :}\\\textbf{(a)\space}\begin{bmatrix}\textbf{x}^{\textbf{-1}} &\textbf{0} &\textbf{0}\\\textbf{0} &\textbf{y}^{\normalsize-1} &\textbf{0}\\\textbf{0} &\textbf{0} &\textbf{z}^{\normalsize-1}\end{bmatrix}\\\textbf{(b)\space}\textbf{xyz}\begin{bmatrix}\textbf{x}^{\normalsize-1} &\textbf{0} &\textbf{0}\\\textbf{0} &\textbf{y}^{\normalsize-1} &\textbf{0}\\\textbf{0} &\textbf{0} &\textbf{z}^{\normalsize-1}\end{bmatrix}\\\textbf{(c)\space}\frac{\textbf{1}}{\textbf{xyz}}\begin{bmatrix}\textbf{x} &\textbf{0} &\textbf{0}\\\textbf{0} &\textbf{y} &\textbf{0}\\\textbf{0} &\textbf{0} &\textbf{z}\end{bmatrix}$$
$$\textbf{(d)\space}\frac{\textbf{1}}{\textbf{xyz}}\begin{bmatrix}\textbf{1} &\textbf{0} &\textbf{0}\\\textbf{0} &\textbf{1} &\textbf{0}\\\textbf{0} &\textbf{0} &\textbf{1}\end{bmatrix}\\\textbf{Sol.\space}\text{(a)\space}\begin{bmatrix}x^{\normalsize-1}&0 &0\\0 &y^{\normalsize-1} &0\\0 &0 &z^{\normalsize-1}\end{bmatrix}\\\text{Given A =}\begin{bmatrix}x &0 &0\\0 &y &0\\0 &0 &z\end{bmatrix}\\\text{|A|} =\begin{vmatrix}x &0 &0\\0 &y &0\\0 &0 &z\end{vmatrix}$$
= x(yz - 0) = xyz ≠ 0
(∵ x, y and z are non-zero)
Cofactors of A are
A11 = (yz – 0) = yz, A12 = – (0 – 0) = 0, A13 = 0 – 0 = 0,
A21 = – (0 – 0) = 0, A22 = xz – 0 = xz, A23 = – (0 – 0) = 0,
A31 = 0 – 0 = 0, A32 = – (0 – 0) = 0, A33 = (xy – 0) = xy.
(∵ x, y and z are non-zero)
Cofactors of A are
A11 = (yz – 0) = yz, A12 = – (0 – 0) = 0, A13 = 0 – 0 = 0,
A21 = – (0 – 0) = 0, A22 = xz – 0 = xz, A23 = – (0 – 0) = 0,
A31 = 0 – 0 = 0, A32 = – (0 – 0) = 0, A33 = (xy – 0) = xy.
$$\therefore\space\text{adj (A)}= \begin{bmatrix}yz &0 &0\\0 &xz &0\\0 &0 &xy\end{bmatrix}^{\text{T}}\\=\begin{bmatrix}yz &0 &0\\0 &xz &0\\0 &0 &xy\end{bmatrix}\\\text{Now,\space A}^{\normalsize-1} =\frac{1}{|\text{A}|}\space\text{(adj A)}\\=\frac{1}{xyz}\begin{bmatrix}yz &0 &0\\0 &xz &0\\0 &0 &xy\end{bmatrix}$$
$$=\begin{bmatrix}\frac{1}{x} &0 &0\\0 &\frac{1}{y} &0\\0 &0 &\frac{1}{z}\end{bmatrix}\\=\begin{bmatrix}x^{\normalsize-1} &0 &0\\0 &y^{\normalsize-1} &0\\0 &0 &z^{\normalsize-1}\end{bmatrix}$$
$$\textbf{19.\space Let A =}\begin{bmatrix}\textbf{1} &\textbf{sin}\space\theta &\textbf{1}\\\textbf{-}\textbf{sin}\space\theta &\textbf{1} &\textbf{sin}\space\theta\\\textbf{-1} &-\textbf{sin}\space\theta &\textbf{1}\end{bmatrix}\textbf{,}$$
where 0 ≤ θ ≤ 2π, then
(a) det A = 0
(b) det A ∈ (2, ∞)
(c) det A ∈ (2, 4)
(d) det A ∈ (2, 4).
Sol. (d) det A ∈ (2, 4)
$$\text{Given, A = }\begin{bmatrix}1 &\text{sin}\space\theta &1\\-\text{sin}\space\theta &1 &\text{sin}\space\theta\\\normalsize-1 &-\text{sin}\space\theta &1\end{bmatrix}\\|\text{A}| =\begin{bmatrix}1 &\text{sin}\space\theta &1\\-\text{sin}\space\theta &1 &\text{sin}\space\theta\\\normalsize-1 &-\text{sin}\space\theta &1\end{bmatrix}$$
= 1( 1 + sin2θ) – sin θ(– sin θ + sin θ) + 1(sin2θ + 1)
= 2 + 2 sin2 θ
For 0 ≤ θ ≤ 2π,
– 1 ≤ sin θ ≤ 1 ⇒ 0 ≤ sin2θ ≤ 1
⇒ 1 ≤ 1 + sin2θ ≤ 2 ⇒ 2 ≤ 2(1 + sin2θ) ≤ 4
∴ det(A) ∈ [2, 4]
