NCERT Solutions for Class 12 Maths Chapter 7 - Integrals - Exercise 7.2
Exercise 7.1 Solutions 22 Questions
Exercise 7.2 Solutions 39 Questions
Exercise 7.3 Solutions 24 Questions
Exercise 7.4 Solutions 25 Questions
Exercise 7.5 Solutions 23 Questions
Exercise 7.6 Solutions 24 Questions
Exercise 7.7 Solutions 11 Questions
Exercise 7.8 Solutions 6 Questions
Exercise 7.9 Solutions 22 Questions
Exercise 7.10 Solutions 10 Questions
Exercise 7.11 Solutions 21 Questions
Miscellaneous Exercise on Chapter 7 Solutions 44 Questions
Exercise 7.2
Direction (Q.1 to 37) Integrate the following functions.
$$\textbf{1.}\space\frac{\textbf{2x}}{\textbf{1+x}^\textbf{2}}\\\textbf{Sol.}\space\text{Let}\space\text{I}=\int\frac{2x}{1+x^2}dx$$
Let 1 + x2 = t
On differentiating w.r.t. x, we get
2x dx = dt
$$\Rarr\space dx=\frac{dt}{2x}\\\therefore\space\text{I}=\int\frac{2x}{t}\frac{dt}{2x}=\int\frac{1}{t}dt$$
= log |t| + C
= log |1 + x2| + C
$$\textbf{2.}\space\frac{\textbf{(log x)}^\textbf{2}}{\textbf{x}}\\\textbf{Sol.}\space\text{Let}\space\text{I}=\int\frac{\text{(log\space x)}^\text{2}}{\text{x}}\space\text{dx}$$
Let log x = t
On differentiating w.r.t. x, we get
$$\frac{1}{x}=\frac{dt}{dx}\\\Rarr\space dx=x dt\\\therefore\space\text{I}=\int\frac{1}{x(t)}xdt=\int t^2dt=\frac{t^3}{3}+\text{C}\\=\frac{(log\space x)^3}{3}+\text{C}$$
$$\textbf{3.}\space\frac{\textbf{1}}{\textbf{x + x log x}}\\\textbf{Sol.}\space\text{Let}\space\text{I}=\int\frac{1}{x(1+\text{log x})}dx$$
Let 1 + log x = t
On differentiating w.r.t. x, we get
$$\frac{1}{x}=\frac{dt}{dx}\\\Rarr\space dx=x dt\\\therefore\space \text{I}=\int\frac{1}{x(t)}x dt=\int\frac{1}{t}dt$$
= log |t| + C
= log |1 + log x| + C.
4. sin x sin (cos x)
Sol. Let I = ∫ sin x sin (cos x) dx
Let cos x = t
On differentiating w.r.t. x, we get
$$-\text{sin x}=\frac{dt}{dx}\\\Rarr\space dx=\frac{dt}{-\text{sin x}}\\\therefore\space \text{I}=\int\text{sin x sin(t)}\frac{dt}{-\text{sin x}}$$
= – ∫ sin t dt = – [– cos t] + C
= cos (cos x) + C
5. sin (ax + b) cos (ax + b)
Sol. Let I = ∫ sin (ax + b) cos (ax + b) dx
Let sin (ax + b) = t
On differentiating w.r.t. x, we get
$$a\space\text{cos}(ax+b)=\frac{dt}{dx}\\\Rarr\space dx=\frac{dt}{a \text{cos}(ax+b)}\\\therefore\space\text{I}=\int\space\text{t cos(ax+b)}\space\frac{dt}{\text{a cos}(ax+b)}\\=\frac{1}{a}\int t dt=\frac{1}{a}.\frac{t^2}{2}+\text{C}\\=\frac{[\text{sin}(ax+b)]^2}{2a}+\text{C}$$
$$\textbf{6}.\sqrt{\textbf{ax+b}}\\\textbf{Sol.}\space\int\sqrt{ax+b}\space dx=\int(ax+b)^{1/2}dx\\\bigg[\because\space\int(ax+b)^n dx=\frac{(ax+b)^{n+1}}{a(n+1)}\bigg]\\=\frac{(ax+b)^{(1/2)+1}}{a\bigg(\frac{1}{2}+1\bigg)}+\text{C}\\=\frac{(ax+b)^{3/2}}{a\bigg(\frac{3}{2}\bigg)}+\text{C}\\=\frac{2}{3a}(ax+b)^{3/2}+\text{C}$$
$$\textbf{7.}\space \textbf{x}\sqrt{\textbf{x+2}}\\\textbf{Sol.}\space\int x\sqrt{x+2}dx\\=\int(x+2-2)\sqrt{x+2}\space dx\\=\int(x+2)\sqrt{x+2}dx-2\int\sqrt{x+2}\space dx\\=\int(x+2)^{3/2}dx-2\int(x+2)^{1/2}dx\\=\frac{(x+2)^{(3/2)+1}}{(3/2)+1}-2\frac{(x+2)^{(1/2)+1}}{(1/2)+1}+\text{C}\\\bigg[\because\space\int x^ndx=\frac{x^{n+1}}{n+1}\bigg]$$
$$=\frac{2}{5}(x+2)^{5/2}-\frac{2×2}{3}(x+2)^{3/2}+\text{C}\\=\frac{2}{5}(x+2)^{5/2}-\frac{4}{3}(x+2)^{3/2}+\text{C}$$
Alternate Method
Let x + 2 = t or x = (t – 2)
$$\Rarr\space 1=\frac{dt}{dx}\Rarr dx=dt\\\text{Then,}\space\int x\sqrt{x+2}dx=\int(t-2)\sqrt{t}\space dt\\=\int(t^{3/2}-2 t^{1/2})dt\\=\frac{t^{(3/2)+1}}{(3/2)+1}-2\frac{t^{(1/2)+1}}{(1/2)+1}+\text{C}\\=\frac{2}{5}t^{5/2}-\frac{4}{3}t^{3/2}+\text{C}\\=\frac{2}{5}(x+2)^{5/2}-\frac{4}{3}(x+2)^{3/2}+\text{C}$$
$$\textbf{8.\space} \textbf{x}\sqrt{\textbf{1+2x}^\textbf{2}}\\\textbf{Sol.}\space\text{Let}\space \text{I}=\int x\sqrt{1+2x^2}dx$$
Let 1 + 2x2 = t
On differentiating w.r.t. x, we get
$$4x=\frac{dt}{dx}\\\Rarr\space dx=\frac{dt}{4x}\\\therefore\space \text{I}=\int x\sqrt{t}\frac{dt}{dx}=\frac{1}{4}\int\sqrt{t}\space dt\\\frac{1}{4}\int t^{1/2}dt\\=\frac{1}{4}\frac{t^{(1/2)+1}}{(1/2)+1}+\text{C}\\=\frac{1}{6}(1+2x^2)^{3/2}+\text{C}$$
Note : In the given type of integrals, it is better to substitute the root functions so that it may be converted into a standard integral.
$$\textbf{9.}\space\textbf{(4x+2)}\space\sqrt{\textbf{x}^\textbf{2}\textbf{+x+1}}\\\textbf{Sol.}\space\text{I}=\int(4x+2)\sqrt{x^2+x+1}\space dx.$$
Let x2 + x + 1 = t
On differentiating w.r.t. x, we get
$$2x+1=\frac{dt}{dx}\\\Rarr\space dx=\frac{dt}{(2x+1)}\\\therefore\space\text{I}=\int(4x+2)\sqrt{t}\frac{dt}{(2x+1)}\\=\int2(2x+1)\sqrt{t}\frac{dt}{(2x+1)}\\=2\int\sqrt{t} dt\\=2\frac{t^{(1/2)+1}}{(1/2)+1}+\text{C}\\=\frac{4}{3}(x^2+x+1)^{3/2}+\text{C}$$
$$\textbf{10.}\space\frac{1}{x-\sqrt{x}}\\\textbf{Sol.}\space\text{Let}\space\text{I}=\int\frac{1}{x-\sqrt{x}}dx\\=\int\frac{1}{\sqrt{x}(\sqrt{x}-1)}dx\\\text{Let}\space\sqrt{x}-1=t\\\Rarr\space \frac{1}{2\sqrt{x}}=\frac{dt}{dx}\\\Rarr\space dx=2\sqrt{x}\space dt\\\therefore\space\text{I}=\int\frac{1}{\sqrt{x}t}2\sqrt{x}dt\\=\int\frac{2}{t}dt$$
$$=2.\text{log}|t|+\text{C}\\=2\space\text{log}|\sqrt{x}-1|+\text{C}$$
$$\textbf{11.}\space\frac{\textbf{x}}{\sqrt{\textbf{x+4}}},\space \textbf{x}\gt \textbf{0}\\\textbf{Sol.}\space\int\frac{x}{\sqrt{x+4}}dx\\=\int\frac{x+4-4}{\sqrt{x+4}}dx\\=\int\frac{x+4}{\sqrt{x+4}}dx-4\int\frac{1}{\sqrt{x+4}}dx\\=\int(x+4)^{1/2}dx-4\int(x+4)^{-1/2}dx\\=\frac{(x+4)^{(1/2)+1}}{(1/2)+1}-4\frac{(x+4)^{\frac{-1}{2}+1}}{(-1/2)+1}+\text{C}\\=\frac{2}{3}\space(x+4)^{3/2}-8(x+4)^{1/2}+\text{C}$$
12. (x3 – 1)1/3 x5.
Sol. Let I = ∫(x3 – 1)1/3 x5dx = ∫(x3 – 1)1/3 x3 . x2dx
Let x3 – 1 = t
$$\Rarr\space x^3= t+1$$
Differentiating w.r.t. x, we get
$$3x^2=\frac{dt}{dx}\\\Rarr\space dx=\frac{dt}{3x^2}\\\therefore\space \text{I}=\int t^{1/3}(t+1)x^2\frac{dt}{3x^2}\\=\frac{1}{3}\int(t^{4/3}+t^{1/3})dt\\=\frac{1}{3}\bigg[\frac{t^{7/3}}{\frac{7}{3}}+\frac{t^{4/3}}{\frac{4}{3}}\bigg]+\text{C}\\=\frac{1}{3}\bigg[\frac{3}{7}t^{7/3}+\frac{3}{4}t^{4/3}\bigg]+\text{C}\\=\frac{1}{7}t^{7/3}+\frac{1}{4}t^{4/3}+\text{C}$$
$$=\frac{1}{7}(x^3-1)^{7/3}+\frac{1}{4}(x^3-1)^{4/3}+\text{C}$$
$$\textbf{13.}\space \frac{\textbf{x}^\textbf{2}}{\textbf{(2+3x}^\textbf{3})^\textbf{3}}\textbf{dx}\\\textbf{Sol.}\space\text{Let}\space\text{I}=\int\frac{x^2}{(2+3x^3)^3}dx\\\text{Let}\space 2+3x^3=t\\\Rarr\space 9x^2=\frac{dt}{dx}\\\Rarr\space dx=\frac{dt}{9x^2}\\\therefore\space \text{I}=\int\frac{x^2}{t^3}\frac{dt}{9x^2}=\frac{1}{9}\int\frac{1}{t^3}dt\\=\frac{1}{9}\int t^{\normalsize -3}dt=\frac{1}{9}\bigg(\frac{t^{\normalsize-3+1}}{\normalsize -3+1}\bigg)+\text{C}$$
$$=\frac{1}{9}×\frac{1}{-2}t^{\normalsize-2}+\text{C}=\frac{-1}{18t^{2}}+\text{C}\\=\frac{-1}{18(2+ 3x^3)^2}+\text{C}$$
$$\textbf{14.}\space\frac{\textbf{1}}{\textbf{x(log x)}^\textbf{m}}\textbf{dx}\\\textbf{Sol.}\space\text{Let}\space \text{I}=\int\frac{1}{x(log\space x)^m}dx\\\text{Let}\space \text{log x}=t\\\Rarr\space \frac{1}{x}=\frac{dt}{dx}\\\Rarr\space dx=x dt\\\therefore\space\text{I}=\int\frac{1}{x(t)^m}x dt=\int t^{-m}\space dt\\=\frac{t^{-m+1}}{-m+1}+\text{C}\\=\frac{(log \space x)^{1-m}}{1-m}+\text{C}$$
$$\textbf{15.}\space\frac{\textbf{x}}{\textbf{9-4x}^\textbf{2}}\\\textbf{Sol.}\space\text{Let}\space\text{I}=\int\frac{x}{9-4x^2}dx\\\text{Let}\space 9-4x^2=t\\\Rarr\space -8x=\frac{dt}{dx}\\\Rarr\space dx=\frac{dt}{-8x}\\\therefore\space \text{I}=\int\frac{x}{t}×\frac{dt}{-8x}\\=\frac{1}{-8}\int\frac{1}{t}dt=\\\frac{1}{-8}\text{log}|t|+\text{C}$$
$$=-\frac{1}{8}\text{log}|9-4x^2|+\text{C}\\=\frac{1}{8}\text{log}\begin{vmatrix}\frac{1}{9-4x^2}\end{vmatrix}+\text{C}\\\bigg[\because\space -alog b=alog b^{\normalsize-1}= alog\frac{1}{b}\bigg]$$
16. e(2x + 3)
Sol. $$\text{Let}\space\text{I}=\int e^{(2x+3)}dx\\\text{Let}\space 2x+3=t\\\Rarr2=\frac{dt}{dx}\\\Rarr\space dx=\frac{dt}{2}\\\therefore\space \text{I}=\int e^t\frac{dt}{2}=\frac{1}{2}\int e^tdt=\frac{1}{2}e^t+\text{C}\\=\frac{1}{2}e^{(2x+3)}+\text{C}$$
$$\textbf{17.}\space \frac{\textbf{x}}{\textbf{e}^{\textbf{x}^{\textbf{2}}}}\\\textbf{Sol.}\space\text{Let}\space \text{I}=\int\frac{x}{e^{x^{2}}}dx\\\text{Let}\space x^2= t\Rarr\space 2x=\frac{dt}{dx}\\\Rarr\space dx=\frac{dt}{2x}\\\therefore\space \text{I}=\int\frac{x}{e^t}\frac{dt}{2x}=\frac{1}{2}\int e^{-t}dt\\=\frac{1}{2}e^{-t}+\text{C}\\=-\frac{1}{2}e^{\normalsize -(x^2)}+\text{C}$$
$$\textbf{18.}\space\frac{\textbf{e}^{\textbf{tan}^{\normalsize-1}\textbf{x}}}{\textbf{1+x}^\textbf{2}}\textbf{dx}\\\textbf{Sol.}\space\text{Let}\space\text{I}=\int\frac{e^{\text{tan}^{\normalsize-1}x}}{1+x^2}dx\\\text{Let}\space \text{tan}^{\normalsize-1}x=t\\\Rarr\space \frac{1}{1+x^2}=\frac{dt}{dx}$$
$$\Rarr\space dx=(1+x^2)dt\\\therefore\space \text{I}=\int\frac{e^t}{1+x^2}(1+x^2)dt$$
= ∫ etdt = et + C = etan–1 x + C
$$\textbf{19.}\space\frac{\textbf{e}^{\textbf{2x}}\textbf{-1}}{\textbf{e}^\textbf{2x}\textbf{+ 1}}\\\textbf{Sol.}\space\text{Let}\space \text{I}=\int\frac{e^{2x}-1}{e^{2x}+1}dx\\=\int\frac{(e^x.e^x-1)}{e^x.e^x+1}dx\\=\int\frac{e^x\bigg(e^x-\frac{1}{e^x}\bigg)}{e^x\bigg(e^x+\frac{1}{e^x}\bigg)}dx\\=\int\frac{(e^x-e^{-x})}{(e^x+e^{-x})}dx\\\text{Let}\space e^x+e^{\normalsize -x}=t$$
$$\Rarr\space e^x-e^{-x}=\frac{dt}{dx}\\\Rarr\space dx=\frac{dt}{e^x-e^{-x}}\\\therefore\space\text{I}=\int\frac{(e^x-e^{-x})}{t}×\frac{dt}{e^x-e^{-x}}\\=\int\frac{1}{t}dt=\text{log}|t|+\text{C}$$
= log|ex + e–x| + C
$$\textbf{20.}\space \frac{\textbf{e}^{\textbf{2x}}\textbf{-e}^{\textbf{-2x}}}{\textbf{e}^{\textbf{2x}}+\textbf{e}^{\textbf{-2x}}}\\\textbf{Sol.}\space \text{Let}\space \text{I}=\int\frac{e^{2x}-e^{-2x}}{e^{2x}+e^{-2x}}dx\\\text{Let}\space e^{2x }+e^{-2x}=t\\\Rarr\space 2e^{2x}-2e^{-2x}=\frac{dt}{dx}\\\Rarr\space dx=\frac{dt}{2(e^{2x}+e^{-2x})}\\\therefore\space\text{I}=\frac{e^{2x}-e^{-2x}}{t}×\frac{dt}{2[e^{2x}-e^{-2x}]}\\=\frac{1}{2}\int\frac{1}{t} dt=\frac{1}{2}\text{log}|t|+\text{C}$$
$$=\frac{1}{2}\text{log}|e^{2x}+e^{\normalsize -2x}|+\text{C}$$
21. tan2(2x – 3)
Sol. Let I = tan2(2x – 3) dx
$$=\int\text{sec}^2(2x-3)dx-\int1dx\\(\because\space \text{tan}^2x=\text{sec}^2x-1)\\\text{Let 2x-3 = t}\\\Rarr\space 2 dx = dt\\\Rarr dx=\frac{1}{2}dt\\\therefore\space \text{I}=\frac{1}{2}\int(\text{sec}^2t)dt-\int 1 dx\\=\frac{1}{2}\text{tan t- x+C}\\=\frac{1}{2}\text{tan}(2x-3)-x+C\\=\frac{tan(2x-3)}{2}-x+\text{C}$$
22. sec2(7 – 4x)
Sol. Let I = sec2(7 – 4x) dx
$$\text{Let}\space 7-4x=t \\\Rarr\space -4 =\frac{dt}{dx}\\\Rarr dx=\frac{dt}{-4}\\\therefore\space \text{I}=\int\text{sec}^2t.\frac{dt}{4}\\=-\frac{1}{4}\text{tan t}+\text{C}\\=-\frac{1}{4}\text{tan}(7-4x)+\text{C}$$
$$\textbf{23.}\space \frac{\textbf{sin}^{\normalsize-1}x}{\sqrt{\textbf{1-x}^\textbf{2}}}\\\textbf{Sol.}\space\text{Let}\space \text{I}=\int\frac{\text{sin}^{\normalsize-1}x}{\sqrt{1-x^2}}dx\\\text{Let \space sin}^{\normalsize-1}x=t\\\Rarr\space \frac{1}{\sqrt{1-x^2}}=\frac{dt}{dx}\\\Rarr dx=\sqrt{1-x^2}dt\\\therefore\space \text{I}=\int\frac{t}{\sqrt{1-x^2}}\sqrt{1-x^2}dt\\=\int tdt=\frac{t^2}{2}+\text{C}=\\\frac{(\text{sin}^{\normalsize-1}x)^2}{2}+\text{C}$$
$$\textbf{24.}\space \frac{\textbf{2 cos x - 3 sin x}}{\textbf{6 cos x + 4 sin x}}\\\textbf{Sol.}\space \text{Let}\space\text{I}=\int\frac{\text{2 cos x - 3 sin x}}{\text{6 cos x + 4 sin x}}dx\\=\int\frac{2\text{cos x - 3 sinx }}{2( 3cos x + 2 sin x)}dx\\\text{Let 3 cos x + 2 sin x = t}\\\Rarr\space -3 \space\text{sin x}+ 2\space \text{cos x}=\frac{dt}{dx}\\\Rarr\space dx=\frac{dt}{\text{2 cos x - 3 sin x}}\\\therefore\space\text{I}=\int\frac{\text{2 cos x - 3 sin x}}{2(t)}\frac{dt}{\text{2 cos x - 3 sin x}}\\=\frac{1}{2}\int\frac{1}{t}dt=\frac{1}{2}\text{log}|t|+\text{C}$$
$$=\frac{1}{2}\space\text{log}|3 \text{cos}x + 2 sin x |+\text{C}$$
$$\textbf{25.\space}\frac{\textbf{1}}{\textbf{cos}^\textbf{2}\textbf{x}(\textbf{1 - tan x})^2}\\\textbf{Sol.}\space \text{Let}\space \text{I}=\int\frac{1}{\text{cos}^2x(1-\text{tan x})^2}dx\\=\int\frac{\text{sec}^2dx}{(1-\text{tanx})^2}dx$$
Let 1 – tan x = t
$$\Rarr\space -\text{sec}^2x=\frac{dt}{dx}\\\Rarr\space dx=\frac{dt}{-\text{sec}^2x}\\\therefore\space\text{I}=\int\frac{\text{sec}^2x}{t^2}\frac{dt}{(-\text{sec}^2x)}\\=-\int t^{\normalsize-2} dt=-\bigg(\frac{-t^{-2+1}}{-2+1}\bigg)+\text{C}\\=\frac{1}{t}+\text{C}\\=\frac{1}{1- tan \space x}+\text{C}$$
$$\textbf{26.}\space \frac{\textbf{cos}\sqrt{x}}{\sqrt{x}}\\\textbf{Sol.}\space\text{Let}\space \text{I}=\int\frac{\text{cos}\sqrt{x}}{\sqrt{x}}dx\\\text{Let}\space\sqrt{x}= t\\\Rarr\space \frac{1}{2\sqrt{x}}=\frac{dt}{dx}\\\Rarr\space dx=2\sqrt{x}dt\\\therefore\space \text{I}=\int\frac{\text{cos t}}{\sqrt{x}} 2\sqrt{x}dt$$
= 2 ∫ cos t dt = 2 sin t + C
$$= 2\space\text{sin}\sqrt{x}+\text{C}$$
$$\textbf{27.}\sqrt{\textbf{sin 2x cos 2x}}\\\textbf{Sol.}\space\text{Let}\space \text{I}=\int\sqrt{\text{sin 2x}}cos 2x dx\\\text{Let}\space \text{sin 2x}=t\\\Rarr\space 2\text{cos}\space 2x =\frac{dt}{dx}\\\Rarr\space dx=\frac{dt}{\text{2 cos 2x}}\\\therefore\space \text{I}=\int\sqrt{t}\text{cos 2x}\frac{dt}{\text{2 cos 2x}}\\=\frac{1}{2}\int\sqrt{t}dt\\=\frac{1}{2}\frac{t^{1/2 +1}}{\bigg(\frac{1}{2}+1\bigg)}+\text{C}$$
$$\frac{1}{3}t^{3/2}+\text{C}\\=\frac{1}{3}(\text{sin 2x})^{3/2}+\text{C}$$
$$\textbf{28.}\space\frac{\textbf{cos x}}{\sqrt{\textbf{ 1+ sin x}}}\\\textbf{Sol.}\space\text{Let}\space \text{I}=\int\frac{\text{cos x}}{\sqrt{1+ sin x}}dx\\=\int(1 + sin x)^{-1/2}\text{cos x}dx\\\text{Let\space} \text{1 + sin x = t }\\\Rarr\space \text{cos x}=\frac{dt}{dx}\\\Rarr\space dx=\frac{dt}{\text{cos x}}\\\therefore\space \text{I}=\int (t)^{-1/2}\text{cos x}\frac{dt}{\text{cos x}}$$
$$=\frac{t^{(-1/2)+1}}{\bigg(-\frac{1}{2}+1\bigg)}+\text{C}\\ =2t^{1/2}+\text{C}\\=2\sqrt{ 1 + sin x}+\text{C}$$
29. cot x log sin x
$$\textbf{Sol.}\space\text{Let}\space \text{I}=\int\text{cot x log sin x dx}\\\text{Let log sin x = t}\\\Rarr\space \frac{1}{\text{sin x}}×\text{cos x}=\frac{dt}{dx}\\\Rarr\space\text{cot x}=\frac{dt}{dx}\\\Rarr\space dx=\frac{dt}{\text{cot x}}\\\therefore\space \text{I}=\int \text{cot x.t}\frac{dt}{cot x}\\=\int t \space dt=\frac{t^2}{2}+\text{C}\\=\frac{(log \space sin x)^2}{2}+\text{C}$$
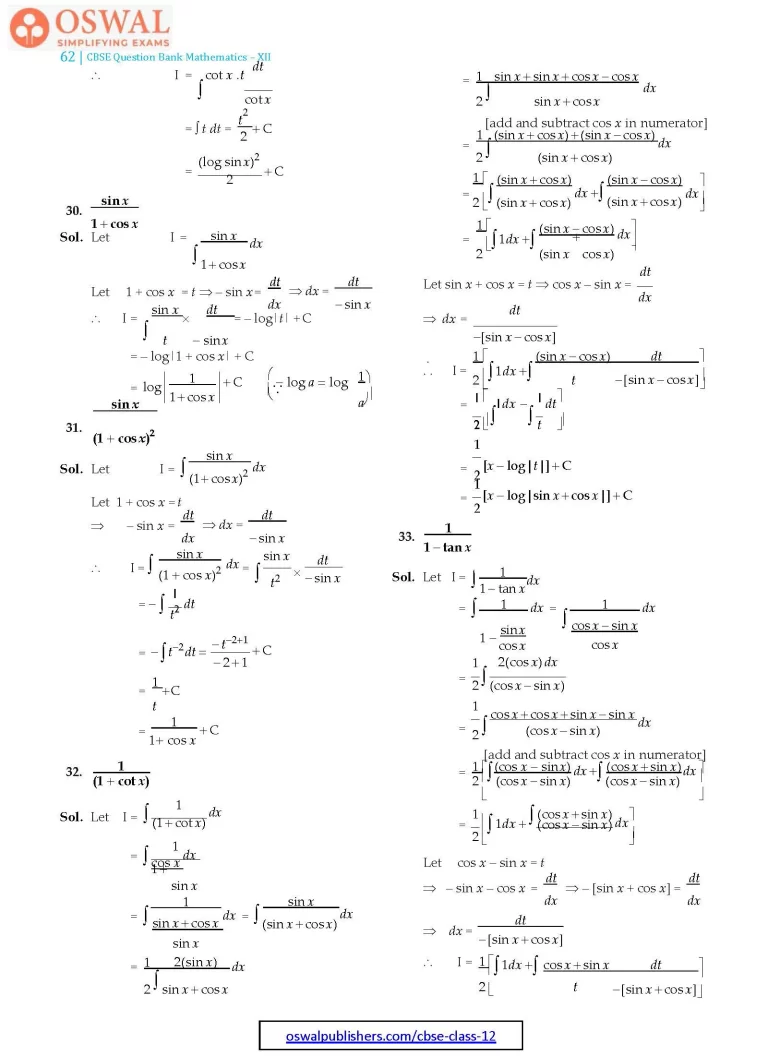
$$\textbf{30.}\space\frac{\textbf{sin x}}{\textbf{1 + cos x}}\\\textbf{Sol.}\space \text{Let}\space \text{I}=\int\frac{\text{sin x}}{\text{1 + cos x}}dx\\\text{Let}\space 1+\text{cos x}=t\\\Rarr\space -\text{sin x}=\frac{dt}{dx}\\\Rarr dx=\frac{dt}{-\text{sin x}}\\\therefore\space \text{I}=\int\frac{sin x}{t}×\frac{dt}{-\text{sin x}}\\=-\text{log}|t|+\text{C}\\=\text{log}\bigg|\frac{1}{1+\text{cos x}}\bigg|+\text{C}$$
$$\bigg(\because\space -\text{log a}=\text{log}\frac{1}{a}\bigg)$$
$$\textbf{31.}\space\frac{\textbf{sin x}}{\textbf{(1 + cos x )}^\textbf{2}}\\\textbf{Sol.}\space \text{Let}\space\text{I}=\int\frac{\text{sin x}}{\text{(1 + cos x)}}dx\\\text{Let 1 + cos x = t}\\\Rarr\space -\text{sin x}=\frac{dt}{dx}\\\Rarr dx=\frac{dt}{-\text{sin x}}\\\therefore\space\text{I}=\int\frac{\text{sin x}}{(1 + \text{cos x})^2}dx\\=\int\frac{\text{sin x}}{t^2}×\frac{dt}{-\text{sin x}}\\=-\int\frac{1}{t^2}\space dt$$
$$=-\int t^{-2}dt=\frac{-t^{-2+1}}{-2+1}\text{C}\\=\frac{1}{t}+\text{C}\\=\frac{1}{\text{1 + cos x}}+\text{C}$$
$$\textbf{32.}\space\frac{\textbf{1}}{\textbf{(1 + cot x)}}dx\\=\int\frac{1}{1 + \frac{\text{cos x}}{\text{sin x}}}dx\\=\int\frac{1}{\frac{\text{sin x + cos x}}{\text{sin x}}}dx\\=\int\frac{\text{sin x}}{\text{(sin x + cos x)}}dx\\=\frac{1}{2}\space\int\frac{\text{2 (sin x)}}{\text{ sin x + cos x}}dx\\=\frac{1}{2}\int\frac{\text{sin x + sin x + cos x - cos x}}{\text{sin x + cos x}}dx\\\text{[add and subtract cos x in numerator]}\\=\frac{1}{2}\int\frac{(\text{sin x + cos x}) + (\text{sin x - cos x})}{\text{(sin x + cos x)}}dx$$
$$=\frac{1}{2}\begin{bmatrix}\int\frac{\text{(sin x + cos x)}}{(\text{sin x + cos x})}dx + \int\frac{(\text{sin x - cos x})}{(\text{sin x + cos })}dx\end{bmatrix}\\=\frac{1}{2}\bigg[\int 1 dx + \int\frac{(\text{sin x - cos x})}{(\text{sin x + cos x})}dx\bigg]\\\text{Let sin x + cos x = t}\\\Rarr\space\text{cos x - sin x}=\frac{dt}{dx}\\\Rarr\space dx = \frac{dt}{-[\text{sin x - cos x}]}\\\therefore\space\text{I}=\\\frac{1}{2}\bigg[\int 1 dx + \int\frac{(\text{sin x - cos x})}{t}\frac{dt}{-[\text{sin x - cos x}]}\bigg]\\=\frac{1}{2}\bigg[\int 1 dx -\int\frac{1}{t}dt\bigg]\\=\frac{1}{2}[x- log|t|] + \text{C}$$
$$=\frac{1}{2}[ x- log|\text{sin x + cos x}|]+\text{C}$$
$$\textbf{33.}\space \frac{\textbf{1}}{\textbf{1 - tan x}}\\\textbf{Sol.}\space \text{Let}\space\text{I}=\int\frac{1}{\text{1 - tan x}}dx\\=\int\frac{1}{1-\frac{sin x}{cos x}}dx=\\\int\frac{1}{\frac{\text{cos x - sin x}}{\text{cos x}}}dx\\=\frac{1}{2}\int\frac{2 (\text{cos x})dx}{\text{(cos x - sin x)}}\\=\frac{1}{2}\int\frac{\text{cos x + cos x + sin x - sin x}}{(cos \space x - sin\space x)}dx\\\text{[add and subtract cos x in numerator]}\\=\frac{1}{2}\bigg[\int\frac{\text{(cos x - sin x)}}{(\text{ cos x - sin x})}dx +\\ \int\frac{(\text{cos x + sin x})}{(\text{ cos x - sin x})}dx\bigg]$$
$$=\frac{1}{2}\bigg[\int 1 dx + \int\frac{(\text{cos x + sin x })}{(\text{cos x - sin x})}dx\bigg]$$
Let cos x – sin x = t
$$\Rarr\space -\text{sin x - cos x}=\frac{dt}{dx}\\\Rarr\space -[\text{sin x + cos x}]=\frac{dt}{dx}\\\Rarr\space dx=\frac{dt}{-[\text{sin x + cos x}]}$$
$$\therefore\space \text{I}=\\\frac{1}{2}\bigg[\int 1 dx + \int\frac{\text{cos x + sin x}}{t}\frac{dt}{-[\text{sin x + cos x }]}\bigg]$$
$$=\frac{1}{2}\bigg[\int 1 dx - \int \frac{1}{t}dt\bigg]\\=\frac{1}{2}[x -\text{log }|t|]+\text{C}\\=\frac{1}{2}[x -\text{log} |\text{cos x - sin x}|]+ \text{C}$$
$$\textbf{34.}\space \frac{\sqrt{\textbf{tan x}}}{\textbf{sin x . cos x}}\textbf{dx}.\\\textbf{Sol.}\space\text{Let}\space \text{I}=\int\frac{\sqrt{\text{tan x}}}{\text{sin x cos x}}dx\\=\int\frac{\sqrt{\text{tan x}}}{\text{sin x cos x}×\frac{\text{cos x}}{\text{cos x}}}dx\\\begin{bmatrix}\text{Multiplying by 1} =\frac{\text{cos x}}{\text{cos x}}\\\text{in denominator}\end{bmatrix}\\=\int\frac{\sqrt{\text{tan x}}}{\text{tan x}}.\text{sec}^2 x dx\\\text{Let tan x = t}\\\Rarr\space\text{sec}^2x=\frac{dt}{dx}\\\Rarr\space dx=\frac{dt}{\text{sec}^2x}$$
$$\therefore\space \text{I}=\int\frac{\sqrt{t}}{t}.\text{sec}^2x\frac{dt}{\text{sec}^2x}\\=\int \frac{1}{\sqrt{t}}dt=\\\int t^{-1/2} dt=\frac{ t^{(-1/2)+1}}{\bigg(-\frac{1}{2}+1\bigg)}+\text{C}\\= \space 2\sqrt{t}+\text{C}\\= 2\sqrt{\text{tan x}}+\text{C}$$
$$\textbf{35.}\space\int \frac{(\textbf{1 + log x})^\textbf{2}}{\textbf{x}}\space \textbf{dx.}\\\textbf{Sol.}\space\text{Let}\space\text{I}=\int\frac{(\text{1 + log x})^2}{x}dx\\\text{Let}\space 1 + \text{log x}= t\\\Rarr\space\frac{1}{t}=\frac{dt}{dx}\\\Rarr\space dx= x\space dt\\\therefore\space \text{I}=\int\frac{t^2}{x} x\space dt=\int t^2dt\\=\frac{t^3}{3}+\text{C}\\=\frac{(\text{1 + log x})^3}{3}+\text{C}$$
$$\textbf{36.}\space \frac{\textbf{(x+1)(x + log x)}^\textbf{2}}{\textbf{x}}\\\textbf{Sol.}\space\text{Let}\space \text{I}=\int\frac{(x+1)(x +log x)^2}{x}dx\\=\int(x + log x)^2\bigg(\frac{x+1}{x}\bigg)dx\\=\int(x + log x)^2\space \bigg(1+\frac{1}{x}\bigg)dx\\\text{Let\space } x + \text{log x}=t \\\Rarr\space \bigg(1+ \frac{1}{x}\bigg)dx=dt\\\therefore\space\text{I}=\int t^2 dt$$
$$=\frac{t^3}{3}+\text{C}\\=\frac{(x + log x)^3}{2}+\text{C}$$
$$\textbf{37.}\space\frac{\textbf{x}^\textbf{3}\space\textbf{sin}(\textbf{tan}^{\normalsize-1}\textbf{x}^{\textbf{4}})}{\textbf{(1 + x}^\textbf{8}\textbf{)}}\textbf{dx}\\\textbf{Sol.}\space\text{Let}\space\text{I}=\int\frac{x^3\text{sin}(\text{tan}^{\normalsize-1}x^{4})}{(1 + x^{8})}dx\\\text{Let}\space \text{tan}^{\normalsize-1}x^{4}=t\\\Rarr\space \frac{1}{1+ x^8}.4x^3=\frac{dt}{dx}\\\Rarr\space dx=\frac{(1 + x^8)}{4x^3}dt\\\therefore\space \text{I}=\int\frac{x^3\text{sin t}}{(1 + x^{8})}.\frac{(1 + x^8)dt}{4x^3}\\=\frac{1}{4}\int\text{sin t dt}=-\frac{1}{4}\text{cos t} + \text{C}$$
$$=-\frac{1}{4}\text{cos}(\text{tan}^{\normalsize-1}x^4)+\text{C}$$
Choose the correct answer.
$$\textbf{38.}\space\int\bigg(\frac{\textbf{10x}^\textbf{9} \textbf{+ 10}^\textbf{x}\space\textbf{log}_e \textbf{10}}{\textbf{x}^{\textbf{10}} \textbf{+ 10}^{\textbf{x}}}\bigg)\space\\\textbf{dx equals.}$$
(a) 10x – x10 + C
(b) 10x + x10 + C
(c) (10x – x10)–1 + C
(d) log|10x + x10|+ C
Sol. (d) log|10x + x10| + C
$$\text{Let}\\\space\text{I}=\int\bigg(\frac{10x^9 + 10^x log_e10}{x^{10} +10^x}\bigg)dx\\\text{Let}\space x^{10} + 10^{x}=t\\\Rarr\space (10x^9 + 10^x log_e 10)dx=dt\\\Rarr\space dx=\frac{dt}{10x^9 + 10^x log_e\space 10}\\\therefore\space \text{I}=\int\frac{10x^9 + 10^x log_e 10}{t}×\\\frac{dt}{10x^9 + 10^x \text{log}_e 10}\\=\int\frac{dt}{t}=\text{log}|t|+\text{C}$$
= log|10x + x10| + C
$$\textbf{39.}\space\int\frac{\textbf{dx}}{\textbf{sin}^\textbf{2}\textbf{x}\textbf{cos}^\textbf{2}\textbf{x}}\space\textbf{is equal to :}$$
(a) tan x + cot x + C
(b) tan x – cot x + C
(c) tan x cot x + C
(d) tan x – cot 2x + C
Sol. (b) tan x – cot x + C
$$\text{Let I}=\int\frac{dx}{\text{sin}^2x\text{cos}^2x}\\=\int\frac{\text{sin}^2x + \text{cos}^2x}{\text{sin}^2x. \text{cos}^2x}\\\lbrack\because\space \text{sin}^2x + \text{cos}^2x=1\rbrack\\=\int\frac{\text{sin}^2x}{\text{sin}^2x.\text{cos}^2x}dx+\int\frac{\text{cos}^2x}{\text{sin}^2x.\text{cos}^2x}dx$$
$$=\int\frac{1}{\text{cos}^2x}dx+\int\frac{1}{\text{sin}^2x}dx$$
= ∫ sec2 x dx + ∫ cosec2 x dx
= tan x – cot x + C
Share page on
