NCERT Solutions for Class 12 Maths Chapter 5 Continuity and Differentiability - Exercise 5.2

Download PDF

Access Exercises of Class 12 Maths Chapter 5 – Continuity and Differentiability

Exercise 5.1 Solutions: 34 Questions (Short Answers)

Exercise 5.2 Solutions: 10 Questions (Short Answers)

Exercise 5.3 Solutions: 15 Questions ( Short Answers)

Exercise 5.4 Solutions: 10 Questions (Short Answers)

Exercise 5.5 Solutions: 18 Questions ( Short Answers)

Exercise 5.6 Solutions: 11 Questions (Short Answers)

Exercise 5.7 Solutions: 17 Questions (Short Answers)

Exercise 5.8 Solutions: 6 Questions (Short Answers)

Miscellaneous Exercise Solutions: 23 Questions (6 Long, 17 Short)

Exercise 5.2

Differentiate the following functions with respect to x.

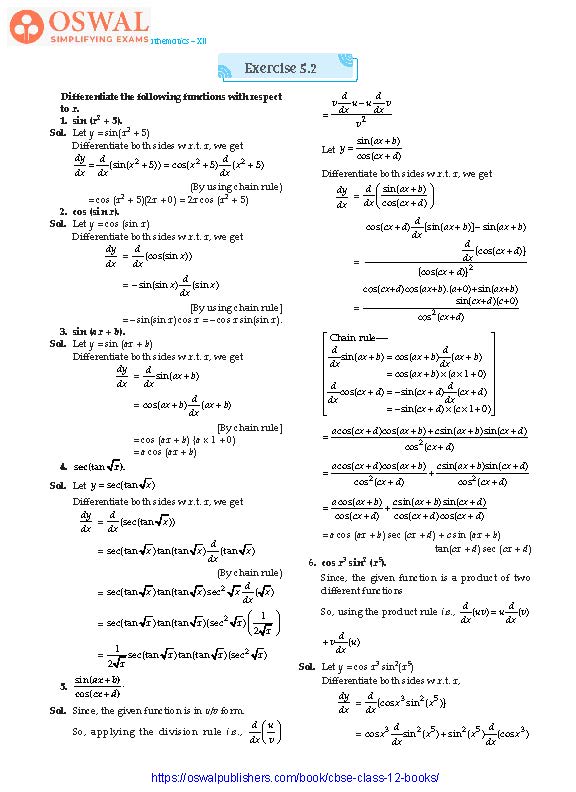
1. sin (x² + 5).

Sol. Let y = sin(x² + 5)

Differentiate both sides w.r.t. x, we get

$$\frac{dy}{dx} =\frac{d}{dx}(\text{sin}(x^{2}+5))=\\\text{cos}(x^{2}+5)\frac{d}{dx}(x^{2}+5)$$

(By using chain rule)

= cos (x² + 5)(2x + 0)

= 2x cos (x² + 5)

2. cos (sin x).

Sol. Let y = cos (sin x)

Differentiate both sides w.r.t. x, we get

$$\frac{dy}{dx} = \frac{d}{dx}(\text{cos(sin x)})\\=-\text{sin(sin x)}\frac{d}{dx}(\text{sin x})$$

[By using chain rule]

= – sin(sin x) cos x = – cos x sin(sin x).

3. sin (ax + b).

Sol. Let y = sin (ax + b)

Differentiate both sides w.r.t. x, we get

$$\frac{dy}{dx}=\frac{d}{dx}\text{sin}(ax+b)\\=\text{cos(ax+b)}\frac{d}{dx}(ax+b)$$

[By chain rule]

= cos (ax + b) {a × 1 + 0)

= a cos (ax + b)

$$\textbf{4.\space sec}(\textbf{tan}\sqrt{\textbf{x}})\textbf{.}\\\textbf{Sol.\space}\text{Let y = }\text{sec(tan}\sqrt{x})$$

Differentiate both sides w.r.t. x, we get

$$\frac{dy}{dx}=\frac{d}{dx}(\text{sec(tan}\sqrt{x}))\\=\text{sec(\text{tan}}\sqrt{x})\text{tan}(\text{tan}\sqrt{x})\\\frac{d}{dx}(\text{tan}\sqrt{x})\\\text{(By chain rule)}\\=\text{sec}(\text{tan}\sqrt{x})\text{tan(tan}\sqrt{x})\\\text{sec}^{2}\sqrt{x}\frac{d}{dx}(\sqrt{x})\\=\text{sec}(\text{tan}\sqrt{x})\text{tan}(\text{tan}\sqrt{x})\\(\text{sec}^{2}\sqrt{x})\bigg(\frac{1}{2\sqrt{x}}\bigg)\\=\frac{1}{2\sqrt{x}}\space\text{sec}(\text{tan}\sqrt{x})\\\text{tan}(tan\sqrt{x})(\text{sec}^{2}\sqrt{x})$$

$$\textbf{5.\space}\frac{\textbf{sin}\textbf{(ax+b)}}{\textbf{cos}\textbf{(cx+d)}}\textbf{.}$$

Sol. Since, the given function is in u/v form.

So, applying the division rule i.e.,

$$\frac{d}{dx}\bigg(\frac{u}{v}\bigg)$$

$$=\frac{v\frac{d}{dx}u-u\frac{d}{dx}v}{v^{2}}\\\text{Let y =}\frac{\text{sin}(ax+b)}{\text{cos}(cx+d)}$$

Differentiate both sides w.r.t. x, we get

$$\frac{dy}{dx} =\frac{d}{dx}\bigg(\frac{\text{sin}(ax+b)}{\text{cos}(cx+d)}\bigg)$$

$$=\\\frac{\text{cos}(cx+d)\frac{d}{dx}\lbrack\text{sin}(ax+b)\rbrack -\text{sin}(ax+b)\frac{d}{dx}\lbrack\text{cos}(cx+d)\rbrack}{\lbrack\text{cos}(cx+d)\rbrack^{2}}$$

$$=\\\frac{\text{cos}(cx+d)\text{cos}(ax+b).(a+0) +\text{sin}(ax+b)\text{sin}(cx+d)(c+0)}{\text{cos}^{2}(cx+d)}$$

$$\begin{bmatrix}\text{Chain rule -}\\\frac{d}{dx}\text{sin}(ax+b) =\text{cos(ax+b)}\frac{d}{dx}(ax+b)\\\text{= cos(ax+b)×(a×1+0)}\\\frac{d}{dx}\text{cos(cx+d)} =-\text{sin}(cx+d)\frac{d}{dx}(cx+d)\\\text{=-sin}(cx+d)×(c×1+0)\end{bmatrix}\\=\\\frac{a\space cos(cx+d)\text{cos}(ax+b)+\\\text{c sin}(ax+b)\text{sin}(cx+d)}{\text{cos}^{2}(cx+d)}\\=\frac{a\text{cos(cx+d)\text{cos}(ax+b)}}{\text{cos}^{2}(cx+d)}+\\\frac{\text{csin(ax+b)sin(cx+d)}}{\text{cos}^{2}(cx+d)}$$

$$=\frac{a\text{cos}(ax+b)}{\text{cos}(cx+d)}+\\\frac{\text{c sin}(ax+b)\text{sin}(cx+d)}{\text{cos}(cx+d)\text{cos}(cx+d)}$$

= a cos (ax + b) sec (cx + d) + c sin (ax + b) tan(cx + d) sec (cx + d)

6. cos x³ sin² (x⁵).

Since, the given function is a product of two different functions

So, using the product rule i.e.,

$$\frac{\textbf{d}}{\textbf{dx}}(\textbf{uv}) \textbf{= u}\frac{\textbf{d}}{\textbf{dx}}\textbf{(v)}\textbf{+ v}\frac{\textbf{d}}{\textbf{dx}}\textbf{(u)}$$

Sol. Let y = cos x³ sin²(x⁵)

Differentiate both sides w.r.t. x,

$$\frac{dy}{dx} =\frac{d}{dx}\lbrace\text{cosx}^{3}\text{sin}^{2}(x^{5})\rbrace\\=\text{cos x}^{3}\frac{d}{dx}\text{sin}^{2}(x^{5})+\\\text{sin}^{2}(x^{5})\frac{d}{dx}(\text{cos}x^{3})$$

$$\begin{bmatrix}\text{Using product rule}\\\frac{d}{dx}(uv) =u\frac{d}{dx}v +u\frac{d}{dx}v\end{bmatrix}\\=(\text{cosx}^{3})(2\text{sin x}^{5})\frac{d}{dx}(\text{sin}x^{5})+\\\text{sin}^{2}(x^{5})(-\text{sin}x^{3})\frac{d}{dx}(x^{3})\\\begin{bmatrix}\text{Using chain rule}\\\frac{d}{dx}f|g(x)| =f'(x)\frac{d}{dx}g(x)\end{bmatrix}\\=\text{(cos x}^{3})(2 sin \space x^{5})(\text{cos x} ^{5})\frac{d}{dx}(x^{5})+\\\text{sin}^{2}(x^{5})(-\text{sin x}^{3})(3x^{2})$$

(Using chain rule)

= (cos x³)(2 sin x⁵)(cos x⁵)(5x⁴)– sin²(x⁵)(sin x³)(3x²)

= 10x⁴(sin x⁵)(cos x⁵)(cos x³) – 3x² sin x³ sin²x⁵

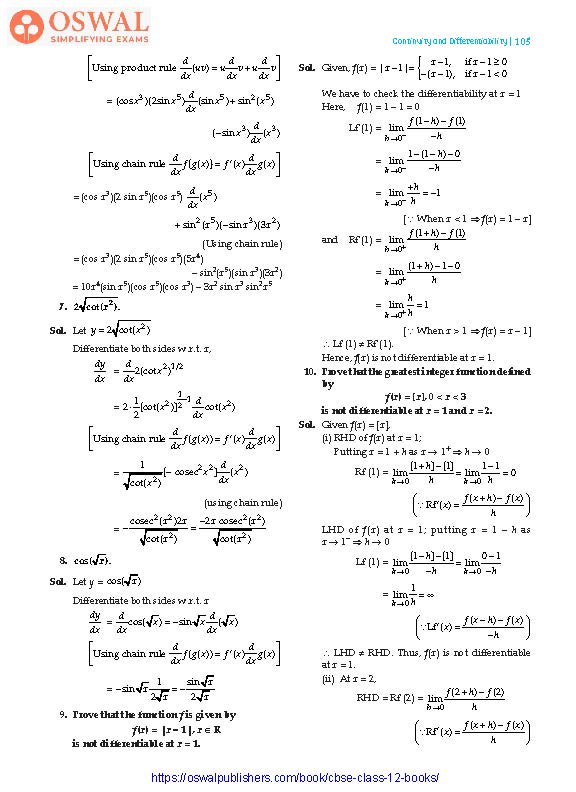
$$\textbf{7.\space 2}\sqrt{\textbf{cot (x}^{\textbf{2}}\textbf{)}.}\\\textbf{Sol.\space}\text{Let y = 2}\sqrt{\text{cot(x}^{2})}$$

Differentiate both sides w.r.t. x,

$$\frac{dy}{dx} =\frac{d}{dx}2(\text{cot x}^{2})^{\frac{1}{2}}\\=2.\frac{1}{2}\lbrack\text{cot}(x^{2})\rbrack^{\frac{1}{2}-1}\frac{d}{dx}\text{cot}(x^{2})\\\begin{bmatrix}\text{Using chain rule}\\\frac{d}{dx}f(g(x)) =f'(x)\frac{d}{dx}g(x)\end{bmatrix}\\=\frac{1}{\sqrt{\text{cot}(x^{2})}}\lbrack-\text{cosec}^{2}x^{2}\rbrack\frac{d}{dx}(x^{2})\\\text{(using chain rule)}\\=-\frac{\text{cosec}^{2}(x^{2})2x}{\sqrt{\text{cot}(x^{2})}}\\=\frac{-\text{2x cosec}^{2}(x^{2})}{\sqrt{\text{cot}(x^{2})}}$$

$$\textbf{8. cos(}\sqrt{x})\textbf{.}\\\textbf{Sol.}\space \text{Let y = cos(}\sqrt{x})\\\text{Differentiate both sides w.r.t. x}\\\frac{dy}{dx} =\frac{d}{dx}\text{cos}(\sqrt{x})=\\-\text{sin}\sqrt{x}\frac{d}{dx}(\sqrt{x})\\\begin{bmatrix}\text{Using chain rule}\\\frac{d}{dx}f(g(x)) =f'(x)\frac{d}{dx}g(x)\end{bmatrix}\\=-\text{sin}\sqrt{x}\frac{1}{2\sqrt{x}}=-\frac{\text{sin}\sqrt{x}}{2\sqrt{x}}$$

9. Prove that the function f is given by

f(x) = |x – 1|, x ∈ R

is not differentiable at x = 1.

$$\textbf{Sol.\space}\text{Given, f(x) =}\\|x-1| =\begin{cases}x-1,\space\text{if x-1}\geq0\\-(x-1),\space\text{if x-1}\lt0\end{cases}$$

We have to check the differentiability at x = 1

Here, f(1) = 1 – 1 = 0

$$\text{Lf'(1) =}\\\lim_{h\to0^{\normalsize-}}\frac{f(1-h)-f(1)}{\normalsize-h}\\=\lim_{h\to0^{\normalsize-}}\frac{1-(1-h)-0}{-h}\\=\lim_{h\to0}\frac{+h}{h}=-1$$

[∵ When x < 1 ⇒ f(x) = 1 – x]

$$\text{and Rf}^′\text{(1) =}\\\lim_{h\to0^{\normalsize+}}\frac{f(1+h)-f(1)}{h}\\=\lim_{h\to0^{\normalsize-}}\frac{(1+h)-1-0}{h}\\=\lim_{h\to0}\frac{h}{h}=1$$

[∵ When x > 1 ⇒ f(x) = x – 1]

∴ Lf′(1) ≠ Rf′(1).

Hence, f(x) is not differentiable at x = 1.

10. Prove that the greatest integer function defined by f(x) = [x], 0 < x < 3 is not differentiable at x = 1 and x = 2.

Sol. Given f(x) = [x],

(i) RHD of f(x) at x = 1;

Putting x = 1 + h as x → 1⁺ ⇒ h → 0

$$\text{Rf'}(1) =\lim_{h\to0}\frac{[1+h]-[1]}{h}\\=\lim_{h\to0}\frac{1-1}{h}=0\\\bigg(\because\space\text{RF'}(x) =\frac{f(x+h)-f(x)}{h}\bigg)$$

LHD of f(x) at x = 1; putting x = 1 – h as

x → 1^– ⇒ h → 0

$$\text{Lf'(1)} =\lim_{h\to0}\frac{[1-h]-[1]}{\normalsize-h}\\=\lim_{h\to0}\frac{0-1}{h}\\=\lim_{h\to0}\frac{1}{h}=\infty\\\bigg(\because\space\text{LF'(x)=}\frac{f(x-h)-f(x)}{\normalsize-h}\bigg)$$

∴ LHD ≠ RHD. Thus, f(x) is not differentiable
at x = 1.

(ii) At x = 2,

$$\text{RHD = Rf}^′\text{(2) =}\\\lim_{h\to0}\frac{f(2+h)-f(2)}{h}\\\bigg(\because\space\text{Rf'(s)} =\frac{f(x+h)-f(x)}{h}\bigg)\\=\lim_{h\to0}\frac{[2+h]-2}{h}\\=\lim_{h\to0}\frac{2-2}{h} = 0\\\text{LHD = Lf'(2) =}\\\lim_{h\to0}\frac{f(2-h)-f(2)}{\normalsize-h}\\\bigg(\because\text{Lf'(x)} =\frac{f(x-h)-f(x)}{\normalsize-h}\bigg)$$

$$=\lim_{h\to0}\frac{[2-h]-[2]}{\normalsize-h}\\=\lim_{h\to0}\frac{1-2}{h}=\infty$$