NCERT Solutions for Class 12 Maths Chapter 5 Continuity and Differentiability - Exercise 5.7


Access Exercises of Class 12 Maths Chapter 5 – Continuity and Differentiability
Exercise 5.1 Solutions: 34 Questions (Short Answers)
Exercise 5.2 Solutions: 10 Questions (Short Answers)
Exercise 5.3 Solutions: 15 Questions ( Short Answers)
Exercise 5.4 Solutions: 10 Questions (Short Answers)
Exercise 5.5 Solutions: 18 Questions ( Short Answers)
Exercise 5.6 Solutions: 11 Questions (Short Answers)
Exercise 5.7 Solutions: 17 Questions (Short Answers)
Exercise 5.8 Solutions: 6 Questions (Short Answers)
Miscellaneous Exercise Solutions: 23 Questions (6 Long, 17 Short)
Exercise 5.7
Find the second order derivative of the given functions.
1. x2 + 3x + 2.
Sol. Let y = x2 + 3x + 2
Differentiating twicely w.r.t. x, we get
$$\frac{dy}{dx} = 2x+3\space\text{and}\space\\\frac{d^{2}y}{dx^{2}} =\frac{d}{dx}(2x+3) = 2$$
2. x20.
Sol. Let y = x20
Differentiating twicely w.r.t. x, we get
$$\frac{dy}{dx} = 20 x^{19}\\\text{and\space}\frac{d^{2}y}{dx^{2}} =\frac{d}{dx}(20x^{19})$$
= 20(19x18) = 380x18
3. x cos x.
Sol. Let y = x cos x
Differentiating twicely w.r.t. x, we get
$$\frac{dy}{dx} = \frac{d}{dx}(x cos x)\\ = x\frac{d}{dx}\text{cos x + cos x}\frac{d}{dx}(x)$$
= x(– sin x) + cos x.1
= – x sin x + cos x
$$\begin{pmatrix}\text{Using product rule}\\\frac{d}{dx}(u.v) =u\frac{dv}{dx} +v\frac{du}{dx}\end{pmatrix}\\\text{and}\space\frac{d^{2}y}{dx^{2}} =\frac{d}{dx}(- sin x + cos x)\\=-\frac{d}{dx}(x sin x) + \frac{d}{dx}(\text{cos x})$$
= – {x cos x + sin x.1} – sin x
= – x cos x – 2 sin x
(Using product rule)
4. log x.
Sol. Let y = log x
Differentiating twicely w.r.t. x, we get
$$\frac{dy}{dx} =\frac{1}{x}\space\text{and}\\\space\frac{d^{2}y}{dx^{2}} =\frac{d}{dx}\bigg(\frac{1}{x}\bigg) =\frac{\normalsize-1}{x^{2}}$$
5. x3 log x.
Sol. Let y = x3 log x. Differentiating twicely w.r.t. x,
we get
$$\frac{dy}{dx} = \frac{d}{dx}(x^{3}\text{log x})\\ = x^{3}\frac{d}{dx}(log \space x) + \text{log x}\frac{d}{dx}(x^{3})\\= x^{3}\bigg(\frac{1}{x}\bigg) + \text{(log x)(3x}^{2})$$
= x2(1 + 3 log x)
(Using product rule)
$$\text{and\space}\frac{d^{2}y}{dx^{2}} = \frac{d}{dx}\lbrack x^{2}(1 + 3 log x)\rbrack\\= x^{2}\bigg(0 +\frac{3}{x}\bigg) +\\(1 + 3 log x)(2x)$$
= 3x + 2x(1 + 3 log x)
= x(5 + 6 log x)
6. ex sin 5x.
Sol. Let y = exsin 5x.
Differentiating twicely w.r.t. x,
we get
$$\frac{dy}{dx} = \frac{d}{dx}(e^{x} \text{sin 5x})\\= e^{x}\frac{d}{dx}(\text{sin 5x}) + sin\space 5x\frac{d}{dx}(e^{x})$$
= ex cos 5x.5 + sin 5x ex
(Using product rule)
= ex (5 cos 5x + sin 5x)
$$\text{and}\space\frac{d^{2}y}{dx^{2}} =\frac{d}{dx}\lbrace e^{x}(5cos5x + sin5x)\rbrace\\= e^{x}\frac{d}{dx}\lbrace5 cos 5x + sin 5x\rbrace + \\(5 cos 5x + sin 5x)\frac{d}{dx}e^{x}$$
= ex{– 5 sin 5x.5 + cos 5x.5} + {5 cos 5x + sin 5x)ex
= ex {10 cos 5x – 24 sin 5x}
= 2ex(5 cos 5x – 12 sin 5x)
7. e6x cos 3x.
Sol. Let y = e6x cos 3x
Differentiating twicely w.r.t. x, we get
$$\frac{dy}{dx} = \frac{d}{dx}\lbrace e^{6x} cos 3x\rbrace\\ = e^{6x}\frac{d}{dx}(cos 3x) + cos 3x\frac{d}{dx}(e)^{6x}$$
= e6x(– sin 3x.3) + (cos 3x) e6x.6
= e6x {– 3 sin 3x + 6 cos 3x}
$$\text{and}\space\frac{d^{2}y}{dx^{2}} =\\\frac{d}{dx}\lbrack e^{6x}(-3 sin \space 3x + 6 cos 3x)\rbrack\\= e^{6x}\frac{d}{dx}(-3 sin 3x + 6 cos\space 3x) + \\(-3 sin \space3x + 6 cos \space3x)\frac{d}{dx}e^{6x}$$
= e6x(– 3 cos 3x.3 – 6 sin 3x.3) + (– 3 sin 3x + 6 cos 3x) e6x.6
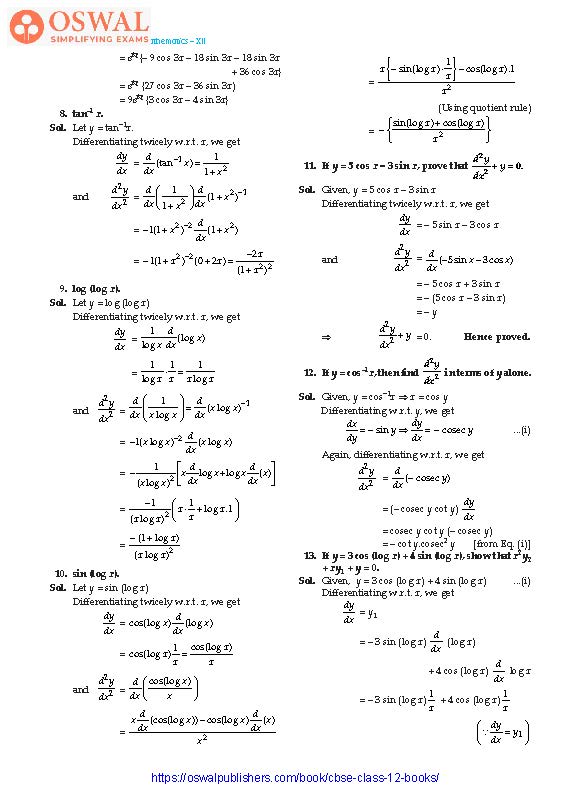
= e6x{– 9 cos 3x – 18 sin 3x – 18 sin 3x + 36 cos 3x}
= e6x {27 cos 3x – 36 sin 3x)
= 9e6x {3 cos 3x – 4 sin 3x}
8. tan–1 x.
Sol. Let y = tan–1x.
Differentiating twicely w.r.t. x, we get
$$\frac{dy}{dx} = \frac{d}{dx}(\text{tan}^{\normalsize-1}x) \\=\frac{1}{\text{1 +x}^{2}}\\\text{and}\space\frac{d^{2}y}{dx^{2}} =\frac{d}{dx}\bigg(\frac{1}{1 +x^{2}}\bigg)\\\frac{d}{dx}(1 +x^{2})^{\normalsize-1}\\=-1(1 + x^{2})^{\normalsize-2}\frac{d}{dx}(1 + x^{2})\\= \normalsize-1(1 +x^{2})^{\normalsize-2}(0 +2x) \\=\frac{-2x}{(1 +x^{2})^{2}}$$
9. log (log x).
Sol. Let y = log (log x)
Differentiating twicely w.r.t. x, we get
$$\frac{dy}{dx} =\frac{1}{log x}\frac{d}{dx}(\text{log x}) \\=\frac{1}{\text{log x}}.\frac{1}{x} = \frac{1}{x log x}\\\text{and}\space \frac{d^{2}y}{dx^{2}} =\frac{d}{dx}\bigg(\frac{1}{\text{x log x}}\bigg)\\=\frac{d}{dx}(x\space log x)^{\normalsize-1}\\=-1(xlog x)^{\normalsize-2}\frac{d}{dx}(x log x)\\ =-\frac{1}{(xlog x)^{2}}\\\bigg[x\frac{d}{dx}log x +log x\frac{d}{dx}(x)\bigg]$$
$$=\frac{\normalsize-1}{(x log x)^{2}}\bigg(x.\frac{1}{x} + log x.1\bigg)\\=\frac{-(1 + log\space x)}{(x \space log x)^{2}}$$
10. sin (log x).
Sol. Let y = sin (log x)
Differentiating twicely w.r.t. x, we get
$$\frac{dy}{dx} =\text{cos(log x)}\frac{d}{dx}(log x)\\=\text{cos(log x)}\frac{1}{x} =\frac{\text{cos(log x)}}{x}\\\text{and}\space\frac{d^{2}y}{dx^{2}} =\frac{d}{dx}\bigg(\frac{\text{cos(log x)}}{x}\bigg)\\= \\\frac{x\frac{d}{dx}(\text{cos}(log x)) -\text{cos(log x)}\frac{d}{dx}(x)}{x^{2}}\\=\\\frac{x\begin{Bmatrix}\text{- sin}(log \space x).\frac{1}{x}\end{Bmatrix} -\text{cos(log x)}.1}{x^{2}}$$
(Using quotient rule)
$$= \\-\begin{Bmatrix}\frac{\text{sin(log x) + cos (log x)}}{x^{2}}\end{Bmatrix}$$
11. If y = 5 cos x – 3 sin x,
$$\textbf{prove that}\space\frac{\textbf{d}^{\textbf{2}}\textbf{y}}{\textbf{dx}^{\textbf{2}}}\textbf{+ y = 0}\textbf{.}$$
Sol. Given, y = 5 cos x – 3 sin x
Differentiating twicely w.r.t. x, we get
$$\frac{dy}{dx} =\text{-5 sin x - 3 cos x}\\\text{and}\space\frac{d^{2}y}{dx^{2}} =\frac{d}{dx}(-5 sin x -3 cos x)$$
= – 5 cos x + 3 sin x
= – (5 cos x – 3 sin x)
= – y
$$\Rarr\space\frac{d^{2}y}{dx^{2}} + y =0$$
Hence proved.
12. If y = cos–1x, then find
$$\frac{\textbf{d}^{\textbf{2}}\textbf{y}}{\textbf{dx}^{\textbf{2}}}\space\textbf{in terms of y alone.}$$
Sol. Given, y = cos–1x ⇒ x = cos y
Differentiating w.r.t. y, we get
$$\frac{dx}{dy} =-\text{sin y}\\\Rarr\space\frac{dy}{dx} =-\text{cosec y}\space\text{...(i)}$$
Again, differentiating w.r.t. x, we get
$$\frac{d^{2}y}{dx^{2}} =\frac{d}{dx}(- cosec \space y)\\=(-\text{cosec y cot y})\frac{dy}{dx}$$
= cosec y cot y (– cosec y)
= – cot y.cosec2 y [from Eq. (i)]
13. If y = 3 cos (log x) + 4 sin (log x),
show that x2y2 + xy1 + y = 0.
Sol. Given, y = 3 cos (log x) + 4 sin (log x) ...(i)
Differentiating w.r.t. x, we get
$$\frac{dy}{dx} = y_{1}\\= -3\space\text{sin(log x)}\frac{d}{dx}(log x) +\\\text{4 cos(log x)}\frac{d}{dx}\space\text{log x}\\=-\text{3 sin(log x)}\frac{1}{x} +\\\text{4 cos(log x)}\frac{1}{x}\\\bigg(\because\space\frac{dy}{dx} = y_{1}\bigg)$$
Multiplying by x, we get
xy1 = – 3 sin (log x) + 4 cos (log x) ...(ii)
Again differentiating w.r.t. x, we obtain
xy2 + y1.1 =
$$\text{-3 cos(log x)}\frac{d}{dx}(log x) -\\\text{4 sin(log x)}\frac{d}{dx}\text{log x}\\=\\\text{- 3 cos(log x)}\frac{1}{x} -\text{4 sin(log x)}\frac{1}{x}$$
Multiplying throughout by x, we have
x2y2 + xy1 = – [3 cos (log x) + 4 sin (log x)]
[from Eq. (i)]
$$\Rarr\space x^{2}y_{2} + xy_{1} =-y\\\Rarr\space x^{2}y_{2} +xy_{1} +y = 0$$
14. If y = Aemx + Benx, show that
$$\frac{\textbf{d}^{\textbf{2}}\textbf{y}}{\textbf{dx}^{\textbf{2}}} \textbf{= (m+n)}\frac{\textbf{dy}}{\textbf{dx}} +\\\textbf{mmy = 0.}$$
Sol. Given, y = A emx + B enx ...(i)
Differentiating twicely w.r.t. x, we get
$$\frac{dy}{dx} = Ae^{mx}\frac{d}{dx}(mx) +\\Be^{nx}\frac{d}{dx}(nx)$$
= Aemx + Benx n ...(ii)
$$\Rarr\space\frac{d^{2}y}{dx^{2}} =m^{2}Ae^{mx} + n^{2} Be^{nx}\\\text{...(iii)}$$
Using equations (i), (ii) and (iii), we have
$$\frac{d^{2}y}{dx^{2}}-(m+n)\frac{dy}{dx} +mny$$
= m2 Aemx + n2 Benx – (m + n) {Amemx + nBenx} + mn {Aemx + Benx}
$$\text{i.e,\space}\frac{d^{2}y}{dx^{2}}-(m+n)\frac{dy}{dx} +mny = 0.$$
Hence proved.
15. If y = 500 e7x + 600 e–7x,
$$\textbf{show that}\space\frac{\textbf{d}^{\textbf{2}}\textbf{y}}{\textbf{dx}^{\textbf{2}}} \textbf{= 49 y.}$$
Sol. Given, y = 500e7x + 600e–7x ...(i)
Differentiating twicely w.r.t. x, we get
$$\frac{dy}{dx} = 500 e^{7x} \frac{d}{dx}(7x) +\\ 600 e^{\normalsize-7x}\frac{d}{dx}(-7x) $$
= 500 e7x.600 e–7x.(– 7)
$$\text{and}\space\frac{d^{2}y}{dx^{2}} =(7×500)e^{7x}.7-\\(7×600) e^{\normalsize-7x}(\normalsize-7)$$
= 49(500 e7x + 600e–7x)
$$\Rarr\space \frac{d^{2}y}{dx^{2}} = 49 y.$$
Hence proved.
16. If ey(x + 1) = 1, show that
$$\frac{\textbf{d}^{\textbf{2}}\textbf{y}}{\textbf{dx}^{\textbf{2}}} =\bigg(\frac{\textbf{dy}}{\textbf{dx}}\bigg)^{\textbf{2}}\textbf{.}$$
Sol. Given, ey (x + 1) = 1 ...(i)
$$\Rarr\space e^{y} =\frac{1}{x+1}$$
Differentiating both sides w.r.t. x, we get
$$e^{y}\frac{dy}{dx} = -\frac{1}{(x+1)^{2}}\\\Rarr\space \bigg(\frac{1}{x+1}\bigg)\frac{dy}{dx} =-\frac{1}{(x+1)^{2}}\\\bigg(\because\space e^{y} =\frac{1}{x+1}\bigg)\\\Rarr\space\frac{dy}{dx} =-\frac{x+1}{(x+1)^{2}} =\\-\frac{1}{x+1}\space\text{...(ii)}$$
Differentiating again w.r.t. x, we get
$$\frac{d^{2}y}{dx^{2}} =\frac{1}{(x+1)^{2}}\\\text{or}\space\frac{d^{2}y}{dx^{2}} =\bigg(-\frac{1}{x+1}\bigg)^{2} \\=\bigg(\frac{dy}{dx}\bigg)^{2}$$
Hence proved.
17. If y = (tan–1x)2, show that (x2 + 1)2 y2 + 2x(x2 + 1) y1 = 2.
Sol. Given, y = (tan–1 x)2
Differentiating w.r.t. x, we get
$$\frac{dy}{dx} = 2\text{tan}^{\normalsize-1}x\frac{d}{dx}(\text{tan}^{\normalsize-1}x)\\= 2(\text{tan}^{\normalsize-1}x)\frac{1}{1 +x^{2}}$$
or (1 + x2)y1 = 2 tan–1x
$$\bigg(\because\space\frac{dy}{dx} = y_{1}\bigg)$$
Again differentiating w.r.t. x, we get
$$(1 + x^{2})\frac{dy_{1}}{dx} + y_{1}\frac{d}{dx}(1 +x^{2}) \\=\frac{2}{1 +x^{2}}\\\Rarr\space (1 +x^{2})y_{2} + y_{1}(0+2x)\\=\frac{2}{1 +x^{2}}\\\Rarr\space (1 +x^{2})^{2}y_{2} + 2x(1 +x^{2})y_{1}=2\\\bigg(\because\space\frac{d}{dx}(y_{1}) =y_{2}\bigg)$$
