NCERT Solutions for Class 12 Maths Chapter 5 Continuity and Differentiability - Exercise 5.6

Access Exercises of Class 12 Maths Chapter 5 – Continuity and Differentiability
Exercise 5.1 Solutions: 34 Questions (Short Answers)
Exercise 5.2 Solutions: 10 Questions (Short Answers)
Exercise 5.3 Solutions: 15 Questions ( Short Answers)
Exercise 5.4 Solutions: 10 Questions (Short Answers)
Exercise 5.5 Solutions: 18 Questions ( Short Answers)
Exercise 5.6 Solutions: 11 Questions (Short Answers)
Exercise 5.7 Solutions: 17 Questions (Short Answers)
Exercise 5.8 Solutions: 6 Questions (Short Answers)
Miscellaneous Exercise Solutions: 23 Questions (6 Long, 17 Short)
Exercise 5.6
$$\textbf{Find}\space\space\frac{\textbf{dy}}{\textbf{dx}}\textbf{,}\space\textbf{if x and y are connected}\\\textbf{parametrically by the equations}$$
given in questions without eliminating the parameter.
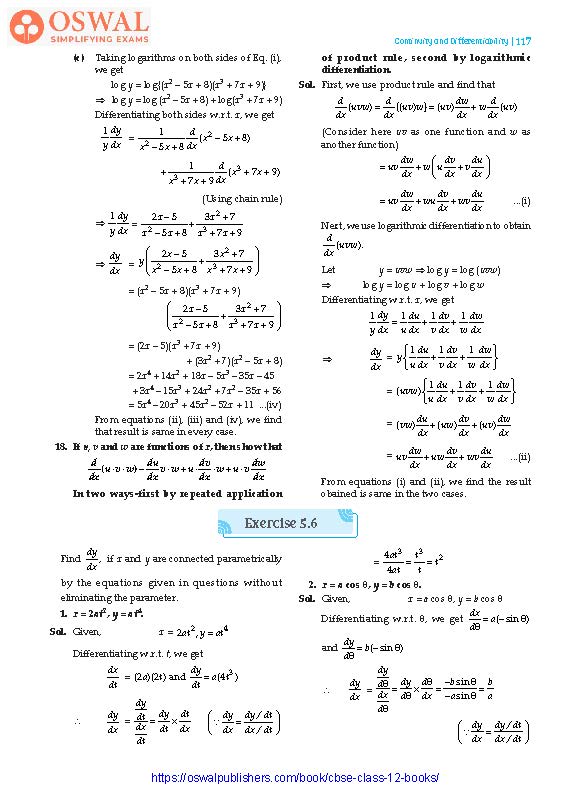
1. x = 2at2, y = at4.
Sol. Given, x = 2at2 , y = at4
Differentiating w.r.t. t, we get
$$\frac{dx}{dt} = (2a)(2t)\text{and}\\\frac{dy}{dt} = a(4t^{3})\\\therefore\space\frac{dy}{dx} = \frac{\frac{dy}{dt}}{\frac{dx}{dt}} = \frac{dy}{dt}×\frac{dt}{dx}$$
$$\begin{pmatrix}\because\space\frac{dy}{dx} =\frac{\frac{dy}{dt}}{\frac{dx}{dt}}\end{pmatrix}\\=\frac{4 at^{3}}{4at} =\frac{t^{3}}{t} =t^{2}$$
2. x = a cos θ, y = b cos θ.
Sol. Given, x = a cos θ, y = b cos θ
Differentiating w.r.t. θ, we get
$$\frac{dx}{d\theta} = a(-\text{sin}\theta)\space\text{and}\\\space\frac{dy}{d\theta} = b(-\text{sin}\theta)\\\therefore\space\frac{dy}{dx} = \frac{\frac{dy}{d\theta}}{\frac{dx}{d\theta}} =\frac{dy}{d\theta}×\frac{d\theta}{dx}\\=\frac{-b\space\text{sin}\space\theta}{\space-a\space\text{sin}\theta} =\frac{b}{a}\\\begin{pmatrix}\because\space\frac{dy}{dx} =\frac{\frac{dy}{dt}}{\frac{dx}{dt}}\end{pmatrix}$$
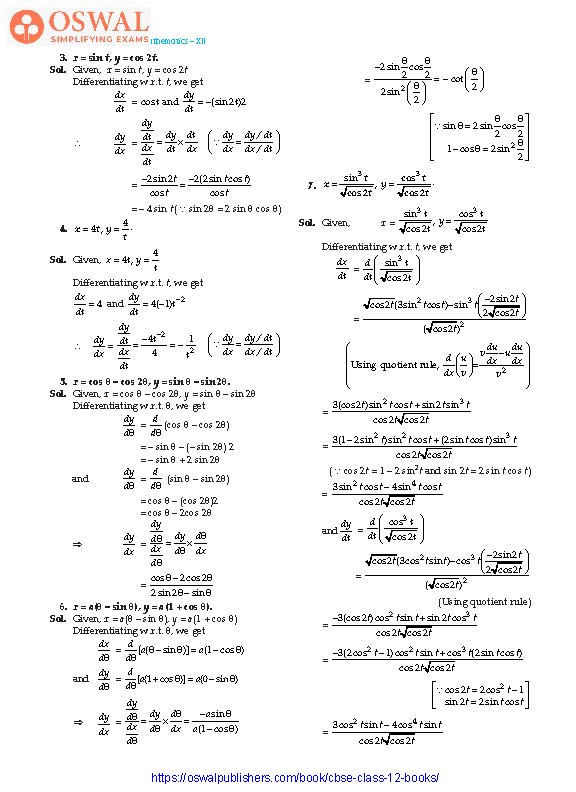
3. x = sin t, y = cos 2t.
Sol. Given, x = sin t, y = cos 2t
Differentiating w.r.t. t, we get
$$\frac{dx}{dt} = \text{cos t and}\\\space\frac{dy}{dt} =-(\text{sin 2t})2\\\therefore\space\frac{dy}{dx} =\frac{\frac{dy}{dt}}{\frac{dx}{dt}}\\=\frac{dy}{dt}×\frac{dt}{dx}\\\begin{pmatrix}\because\space\frac{dy}{dx} =\frac{\frac{dy}{dt}}{\frac{dx}{dt}}\end{pmatrix}\\=\frac{-2\text{sin 2t}}{\text{cos t}} \\=\frac{-2(\text{2 sin t cos t})}{\text{cos t}}$$
= – 4 sin t (∵ sin 2θ = 2 sin θ cos θ)
$$\textbf{4.\space x = 4t, y =}\frac{4}{t}\\\textbf{Sol.\space}\text{Given, \space x = 4t, y} =\frac{4}{t}$$
Differentiating w.r.t. t, we get
$$\frac{dx}{dt} = 4\space\text{and}\space\frac{dy}{dt} = 4(\normalsize-1)t^{\normalsize-2}\\\therefore\space\frac{dy}{dx} =\frac{\frac{dy}{dt}}{\frac{dx}{dt}} =\frac{-4t^{\normalsize-2}}{4}\\=-\frac{1}{t^{2}}\space\begin{pmatrix}\because\space\frac{dy}{dx} =\frac{\frac{dy}{dt}}{\frac{dx}{dt}}\end{pmatrix}$$
5. x = cos θ – cos 2θ, y = sin θ – sin 2θ.
Sol. Given, x = cos θ – cos 2θ, y = sin θ – sin 2θ
Differentiating w.r.t. θ, we get
$$\frac{dy}{d\theta} =\frac{d}{d\theta}(\text{cos}\theta - cos 2\theta)$$
= – sin θ – (– sin 2θ) 2
= – sin θ + 2 sin 2θ
$$\text{and}\space\frac{dy}{d\theta} = \frac{d}{d\theta}(\text{sin}\theta - sin 2\theta)$$
= cos θ – (cos 2θ)2
= cos θ – 2cos 2θ
$$\Rarr\space\frac{dy}{dx} = \frac{\frac{dy}{d\theta}}{\frac{dx}{d\theta}} =\frac{dy}{d\theta}×\frac{d\theta}{dx}\\=\frac{\text{cos}\theta - 2cos\space 2\theta}{\text{2 sin 2}\theta -\text{sin}\space\theta}$$
6. x = a(θ – sin θ), y = a(1 + cos θ).
Sol. Given, x = a(θ – sin θ), y = a(1 + cos θ)
Differentiating w.r.t. θ, we get
$$\frac{dx}{d\theta} = \frac{d}{d\theta}\lbrack a(\theta - sin\theta)\rbrack$$
= (1− cos θ)
$$\text{and}\space\frac{dy}{d\theta} = \frac{d}{d\theta}\lbrack a(1 + cos\space\theta)\rbrack$$
= a(0 − sin θ)
$$\Rarr\space\frac{dy}{dx} =\frac{\frac{dy}{d\theta}}{\frac{dx}{d\theta}}\\=\frac{dy}{d\theta}×\frac{d\theta}{dx} = \frac{\text{-a sin}\theta}{a(1 - cos \space\theta)}\\=\frac{-2 sin\frac{\theta}{2}\text{cos}\frac{\theta}{2}}{2\text{sin}^{2}\bigg(\frac{\theta}{2}\bigg)} =-\text{cot}\bigg(\frac{\theta}{2}\bigg)\\\begin{bmatrix}\because\space\text{sin}\space\theta = 2\text{sin}\frac{\theta}{2}\text{cos}\frac{\theta}{2}\\\text{1 - cos}\theta = 2 \text{sin}^{2}\frac{\theta}{2}\end{bmatrix}$$
$$\textbf{7.\space x =}\space\frac{\textbf{sin}^{\textbf{3}}\textbf{t}}{\sqrt{\textbf{cos 2t}}}\textbf{,}\space\textbf{y =}\frac{\textbf{cos}^{\textbf{3}}\textbf{t}}{\sqrt{\textbf{cos 2t}}}.$$
Sol. Given,
$$x =\frac{\text{sin}^{3}t}{\sqrt{\text{cos 2t}}}, y =\frac{\text{cos}^{3}t}{\sqrt{\text{cos 2t}}}$$
Differentiating w.r.t. t, we get
$$\frac{dx}{dt} = \frac{d}{dt}\begin{pmatrix}\frac{\text{sin}^{3}t}{\sqrt{\text{cos 2t}}}\end{pmatrix}\\=\\\frac{\sqrt{\text{cos}2t}(3 sin^{2}t cos t) - \text{sin}^{3}t\begin{pmatrix}\frac{-2 sin 2t}{2\sqrt{\text{cos 2t}}}\end{pmatrix}}{(\sqrt{ cos 2 t})^{2}}$$
$$\begin{pmatrix}\text{Using quotient rule,}\\\frac{d}{dx}\bigg(\frac{u}{v}\bigg) =\frac{v\frac{du}{dx} -u\frac{du}{dx}}{v^{2}}\end{pmatrix}\\=\\\frac{3(\text{cos 2t})\text{sin}^{2}t\text{cos t + sin 2t sin}^{3}t}{\text{cos 2t}\sqrt{\text{cos 2t}}}\\=\\\frac{3(1 - 2 \text{sin}^{2}t)\text{sin}^{2}t\text{cos t} + (\text{2 sint cos t})sin^{3}t}{\text{cos 2t}\sqrt{\text{cos 2t}}}$$
(∵ cos 2t = 1 – 2 sin2t and sin 2t = 2 sin t cos t)
$$= \frac{3\space\text{sin}^{2}\text{t cos t} - 4\space\text{sin}^{4}tcos t}{\text{cos 2t}\sqrt{cos 2t}}\\\text{and\space}\frac{dy}{dt} =\frac{d}{dt}\begin{pmatrix}\frac{\text{cos}^{3}t}{\sqrt{cos 2t}}\end{pmatrix}\\=\\\frac{\sqrt{cos 2t}(3 cos^{2}t sin t) - \text{cos}^{3}t\bigg(\frac{-\text{2 sin 2t}}{2\sqrt{cos 2t}}\bigg)}{(\sqrt{cos 2t})^{2}}$$
(Using quotient rule)
$$=\\\frac{-3(cos \space 2t)\text{cos}^{2}t sint + sin 2t cos^{3}t}{\text{cos 2t}\sqrt{\text{cos 2t}}}\\=\\\frac{-3(2 cos^{2}t -1)\text{cos}^{2}t sin t + \text{cos}^{3}t(2 sin t cos t)}{\text{cos 2t}\sqrt{\text{cos 2t}}}$$
$$\begin{bmatrix}\because\space \text{cos 2t = 2 cos}^{2}t-1\\\text{sin 2t = 2 sin t cos t}\end{bmatrix}\\=\\\frac{\text{3 cos}^{2}\text{t sint} - 4\text{cos}^{4}t\space\text{sin t}}{\text{cos 2t}\sqrt{\text{cos 2t}}}$$
$$\Rarr\space\frac{dy}{dt} = \frac{\frac{dy}{dt}}{\frac{dx}{dt}} =\frac{dy}{dt}×\frac{dt}{dx}\\=\frac{\text{3 cos}^{2}\text{t sint} - 4\text{cos}^{4}\text{t sint}}{\text{3 sin}^{2}\text{t cos t} - 4\text{sin}^{4}t cos t}\\=\frac{cos^{2}\text{t sin t}(3 -4 \space cos^{2}t)}{\text{sin}^{2}t\text{cos t}(3-4 sin^{2}t)}\\=\frac{\text{cos t}(3 -4 cos^{2}t)}{\text{sin t(3 - 4 sin}^{2}t)}\\=\frac{\text{3 cos t - 4 cos t}^{3}t}{\text{3 sin t - 4 sin}^{2}t}\\=\frac{\text{- cos 3t}}{\text{sin 3t}} =-\text{cot 3t}$$
$$\begin{bmatrix}\because\space\text{cos 3t = 4 cos}^{3}t - 3\text{cos t}\\\text{sin 3t = 3 sint - 4 sin}^{3}t\end{bmatrix}$$
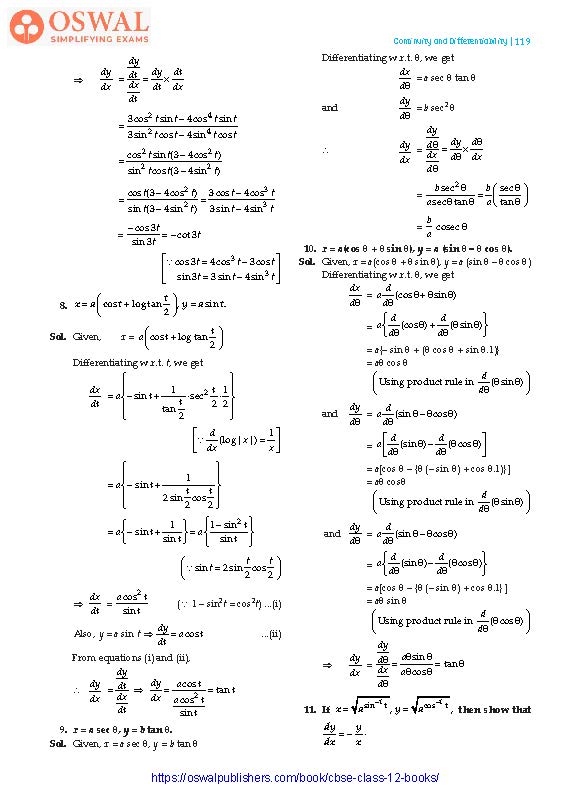
$$\textbf{8.\space x=a}\begin{pmatrix}\textbf{cos t + log tan}\frac{\textbf{t}}{\textbf{2}}\end{pmatrix}\textbf{,}\\\textbf{y = a sin t}\textbf{.}\\\textbf{Sol.\space}\text{Given,}\\\text{\space x = a}\begin{pmatrix}\text{cos t + log tan}\frac{t}{2}\end{pmatrix}$$
Differentiating w.r.t. t, we get
$$\frac{dx}{dt} = a\begin{Bmatrix}-\text{sin t} + \frac{1}{\text{tan}\frac{t}{2}}.\text{sec}^{2}\frac{t}{2}.\frac{1}{2}\end{Bmatrix}\\\begin{bmatrix}\because\space\frac{d}{dx}(\text{log|x|}) =\frac{1}{x}\end{bmatrix}\\ = a\begin{Bmatrix}-\text{sin t} +\frac{1}{\text{2 sin}\frac{t}{2}\text{cos}\frac{t}{2}}\end{Bmatrix}\\ = a\begin{Bmatrix}-\text{sin t} + \frac{1}{\text{sin t}}\end{Bmatrix} \\= a\begin{Bmatrix}\frac{\text{1 - sin}^{2}t}{\text{sin t}}\end{Bmatrix}\\\begin{pmatrix}\because\space\text{sin t = 2 sin}\frac{t}{2}\text{cos}\frac{t}{2}\end{pmatrix}\\\Rarr\space\frac{dx}{dt} =\frac{\text{a cos}^{2}t}{\text{sin t}}$$
(∵ 1 – sin2t = cos2t) ...(i)
$$\text{Also, y = a sint}\\\Rarr\space\frac{dy}{dt} = \text{a cos t}\space\text{...(ii)}$$
From equations (i) and (ii),
$$\therefore\space\frac{dy}{dx} =\frac{\frac{dy}{dt}}{\frac{dx}{dt}}\\\Rarr\space\frac{dy}{dx} =\frac{\text{a cos t}}{\frac{\text{a cos}^{2}t}{\text{sin t}}} =\text{tan t}$$
9. x = a sec θ, y = b tan θ.
Sol. Given, x = a sec θ, y = b tan θ
Differentiating w.r.t. θ, we get
$$\frac{dx}{d\theta} =\text{a sec}\theta\text{tan}\theta\\\text{and}\space\frac{dy}{d\theta} =\text{b sec}^{2}\theta\\\therefore\space\frac{dy}{dx} =\frac{\frac{dy}{d\theta}}{\frac{dx}{d\theta}}\\=\frac{dy}{d\theta}×\frac{d\theta}{dx}\\=\frac{\text{b sec}^{2}\theta}{\text{a sec}\theta \space tan\theta}\\=\frac{b}{a}\bigg(\frac{\text{sec}\theta}{\text{tan}\theta}\bigg)\\=\frac{b}{a}\text{cosec}\space\theta$$
10. x = a(cos θ + θ sin θ), y = a (sin θ – θ cos θ).
Sol. Given, x = a(cos θ + θ sin θ), y = a (sin θ – θ cos θ)
Differentiating w.r.t. θ, we get
$$\frac{dx}{d\theta} = a\frac{d}{d\theta}(\text{cos}\space\theta + \text{sin}\space\theta)\\= a\begin{Bmatrix}\frac{d}{d\theta}(\text{cos}\space\theta) + \frac{d}{d\theta}(\theta sin\space\theta)\end{Bmatrix}$$
= a{– sin θ + (θ cos θ + sin θ.1)}
= aθ cos θ
$$\begin{pmatrix}\text{Using product rule in}\\\frac{d}{d\theta}(\theta sin\theta)\end{pmatrix}$$
$$\text{and}\space\frac{dy}{d\theta} = a\frac{d}{d\theta}(\text{sin}\theta - \theta\text{cos}\theta)\\= a\begin{bmatrix}\frac{d}{d\theta}(\text{sin}\theta) -\frac{d}{d\theta}(\theta cos\theta)\end{bmatrix}$$
= a[cos θ – {θ (– sin θ) + cos θ.1)}]
= aθ cosθ
$$\begin{pmatrix}\text{Using product rule in}\\\frac{d}{d\theta}(\theta sin\theta)\end{pmatrix}\\\text{and}\space\frac{dy}{d\theta} = a\frac{d}{d\theta}(\text{sin}\theta - \theta cos\theta)\\= a\begin{Bmatrix}\frac{d}{d\theta}(\text{sin}\theta) - \frac{d}{d\theta}(\theta cos\theta)\end{Bmatrix}$$
= a[cos θ – {θ (– sin θ) + cos θ.1}]
= aθ sin θ
$$\begin{pmatrix}\text{Using product rule in}\\\space\frac{d}{d\theta}(\theta cos \theta)\end{pmatrix}\\\Rarr\space\frac{dy}{dx} =\frac{\frac{dy}{d\theta}}{\frac{dx}{d\theta}}\\=\frac{a\theta sin\theta}{a \theta\text{cos}\theta} =\text{tan}\space\theta$$
$$\textbf{11. If x =}\sqrt{\textbf{a}^{\textbf{sin}^{\normalsize-1}}t}, \textbf{y =}\sqrt{\textbf{a}^{\textbf{cos}^{\normalsize-1}}t}\textbf{,}$$
then show that
$$\frac{\textbf{dy}}{\textbf{dx}} =\normalsize-\frac{\textbf{y}}{\textbf{x}}\textbf{.}$$
Sol. Given,
$$x =\sqrt{a^{sin^{\normalsize-1}}t},\space y =\sqrt{a^{\text{cos}^{\normalsize-1}}t}\\\text{i.e.\space} x = a^{\frac{1}{2}sin^{\normalsize-1}t}\\\text{and\space} y = a^{\frac{1}{2}\text{cos}^{\normalsize-t}t}$$
[∵ (ab)c = abc]
Differentiating w.r.t. t, we get
$$\frac{dx}{dt} = a^{\frac{1}{2}sin^{\normalsize-1}t}\\\text{log a}\frac{d}{dt}\begin{pmatrix}\frac{1}{2}\text{sin}^{\normalsize-1}t\end{pmatrix}\\\begin{bmatrix}\because\space\frac{d}{dx}a^{x} = a^{x} log a\end{bmatrix}\\= a^{\frac{1}{2}sin^{\normalsize-1}t}\space\text{log a}\begin{pmatrix}\frac{1}{2\sqrt{1 - t^{2}}}\end{pmatrix}\\=\frac{a^{\frac{1}{2}\text{sin}^{\normalsize-1}}t \space log a}{2\sqrt{1 - t^{2}}}\\\text{and}\frac{dy}{dt} = a^{\frac{1}{2}cos^{\normalsize-1}t}\\log \space a\frac{d}{dt}\begin{pmatrix}\frac{1}{2}\text{cos}^{\normalsize-1}t\end{pmatrix}$$
(Using chain rule)
$$= a^{\frac{1}{2}\text{cos}^{\normalsize-1}t}\text{log a}\begin{pmatrix}\frac{\normalsize-1}{2\sqrt{1 - t^{2}}}\end{pmatrix}\\=\frac{-a^{\frac{1}{2}\text{cos}^{\normalsize-1}t} log\space a}{2\sqrt{1 - t^{2}}}\\\Rarr\space\frac{dy}{dx} = \frac{\frac{dy}{dt}}{\frac{dx}{dt}}\\=\frac{-a^{\frac{1}{2}\text{cos}^{\normalsize-1}t}log\space a}{2\sqrt{1 - t^{2}}}\\\Rarr\space\frac{dy}{dt} =\frac{\frac{dy}{dt}}{\frac{dx}{dt}} =\frac{-a^{\frac{1}{2}\text{cos}^{\normalsize-1}t}}{a^{\frac{1}{2}\text{sin}^{\normalsize-1}t}}\\=-\frac{\sqrt{a^{cos^{\normalsize-1}t}}}{\sqrt{a^{sin^{\normalsize-1}t}}} =-\frac{y}{x}$$
Alternate method :
$$x =\sqrt{a^{sin^{\normalsize-1}t}}$$
Taking log on both sides
log x = log(asin-1t) 1/2
$$\Rarr\space\text{log x = }\frac{1}{2}\text{log a}^{\textbf{sin}^{\normalsize-1}t}\\\Rarr\space\text{log x = }\frac{1}{2}\text{sin}^{\normalsize-1}\text{tlog a}\\\Rarr\space\text{log x =}\frac{1}{2}\text{log a sin}^{\normalsize-1}t$$
Differentiating w.r.t. t, we get
$$\frac{1}{x}.\frac{dx}{dt} = \frac{1}{2}\text{log a}\frac{d}{dt}(\text{sin}^{\normalsize-1}t)\\=\frac{1}{2}\text{log a}\frac{1}{\sqrt{1 - t^{2}}}\\\Rarr\space\frac{dx}{dt} = \frac{x log a}{2}×\frac{1}{\sqrt{1 - t^{2}}}\\\text{Again,\space} y =\sqrt{a^{cos^{\normalsize-1}t}}$$
Taking log on both sides,
$$\text{log y = log}(a^{cos^{\normalsize-1}t})^{\frac{1}{2}}\\\Rarr\space\text{log y} =\frac{1}{2}\text{log a}^{cos^{\normalsize-1}t}\\\Rarr\space\text{log y =}\frac{1}{2}\text{cos}^{\normalsize-1}t\text{log a}$$
Differentiating w.r.t. t, we get
$$\frac{1}{y}.\frac{dy}{dt} =\\\frac{1}{2}\text{log a}×\begin{bmatrix}\frac{\normalsize-1}{\sqrt{1 - t^{2}}}\end{bmatrix}\\\Rarr\space \frac{dy}{dt} =-\frac{ylog a}{2}×\frac{1}{\sqrt{1 - t^{2}}}$$
We know that
$$\frac{dy}{dx} = \frac{dy}{dt}×\frac{dt}{dx}\\\Rarr\space\frac{dy}{dx} =\frac{-ylog a}{2}×\frac{1}{\sqrt{1 - t^{2}}}\\×\frac{2×\sqrt{1 - t^{2}}}{x log a}\\\Rarr\space\frac{dy}{dx} = \frac{-y}{x}$$
Another method
$$x =\sqrt{a^{sin^{\normalsize-1}t}}\space\text{...(i)}\\\text{and}\space y =\sqrt{a^{cos^{\normalsize-1}t}}\space\text{...(ii)}$$
Multiplying equations (i) and (ii), we get
$$xy =\sqrt{a^{sin^{\normalsize-1}t}}×\\\sqrt{a^{cos{\normalsize-1 \space t}}}\\\Rarr\space xy =\\\sqrt{a^{sin^{\normalsize-1}t}.a^{cos^{\normalsize-1}t}}\\\Rarr\space xy =\sqrt{a^{sin^{\normalsize-1}t} + \text{cos}^{\normalsize-1t}}\\\Rarr\space xy =\sqrt{a^{sin^{\normalsize-1\space t} + cos^{\normalsize-1}t}}\\\begin{bmatrix}\because\space\text{sin}^{-1}x + \text{cos}^{-1}\space x =\frac{\pi}{2}\end{bmatrix}\\\Rarr\space xy =\sqrt{a^{\frac{\pi}{2}}}$$
Differentiating w.r.t. x, we get
$$x\frac{dy}{dx} +y =0\\\Rarr\space x\frac{dy}{dx} =-y\\\begin{pmatrix}\frac{d}{dx}\text{(constant) =0}\end{pmatrix}\\\Rarr\space\frac{dy}{dx} =\frac{-y}{x}$$
