NCERT Solutions for Class 12 Maths Chapter 5 Continuity and Differentiability - Exercise 5.3
Access Exercises of Class 12 Maths Chapter 5 – Continuity and Differentiability
Exercise 5.1 Solutions: 34 Questions (Short Answers)
Exercise 5.2 Solutions: 10 Questions (Short Answers)
Exercise 5.3 Solutions: 15 Questions ( Short Answers)
Exercise 5.4 Solutions: 10 Questions (Short Answers)
Exercise 5.5 Solutions: 18 Questions ( Short Answers)
Exercise 5.6 Solutions: 11 Questions (Short Answers)
Exercise 5.7 Solutions: 17 Questions (Short Answers)
Exercise 5.8 Solutions: 6 Questions (Short Answers)
Miscellaneous Exercise Solutions: 23 Questions (6 Long, 17 Short)
Exercise 5.3
$$\textbf{Find\space}\frac{\textbf{dy}}{\textbf{dx}}\space\textbf{in the following questions :}$$
1. 2x + 3y = sin x.
Sol. Given, 2x + 3y = sin x
Differentiating both sides w.r.t. x, we get
$$\frac{d}{dx}(2x+3y) =\frac{d}{dx}(\text{sinx})\\2+3\frac{dy}{dx} =\text{cos x}\\\Rarr\space 3\frac{dy}{dx} =\text{cos x-2}\\\Rarr\space\frac{dy}{dx} =\frac{\text{cos x-2}}{3}$$
2. 2x + 3y = sin y.
Sol. Given, 2x + 3y = sin y
Differentiating both sides w.r.t. x, we get
$$\frac{d}{dx}(2x+3y) =\frac{d}{dx}(\text{sin y})\\\Rarr\space 2+3\frac{dy}{dx} =\text{cos y}\frac{dy}{dx}\\\Rarr\space3\frac{dy}{dx} -\text{cos y}\frac{dy}{dx}=-2\\\Rarr\space(3-cos y)\frac{dy}{dx}=\normalsize-2\\\Rarr\space\frac{dy}{dx} =\frac{2}{\text{cos y-3}}$$
3. ax + by2 = cos y.
Sol. Given, ax + by2 = cos y
Differentiating both sides w.r.t. x, we get
$$\frac{d}{dx}(ax+by^{2}) =\frac{d}{dx}(\text{cos y})\\\text{a+2by}\frac{dy}{dx}=\\-\text{sin y}\frac{dy}{dx}\\\Rarr\space\text{2by}\frac{dy}{dx} +\text{sin y}\frac{dy}{dx}=-a\\\Rarr\space\frac{dy}{dx}(2by + \text{sin y})=-a\\\Rarr\space\frac{dy}{dx} =\frac{\normalsize-a}{\text{2by +sin y}}$$
4. xy + y2 = tan x + y.
Sol. Given, xy + y2 = tan x + y
Differentiating both sides w.r.t. x, we get
$$\frac{d}{dx}(xy+y^{2}) =\\\frac{d}{dx}(\text{tan x +y})\\\frac{d}{dx}(xy) +\frac{d}{dx}(y^{2}) =\\\text{sec}^{2}x + \frac{dy}{dx}\\\Rarr\space x\frac{dy}{dx} +y.1+2y\frac{dy}{dx}\\=\text{sec}^{2}x +\frac{dy}{dx}\\\begin{pmatrix}\text{Using product rule}\\\frac{d}{dx}(u.v) = u\frac{d}{dx}v +v\frac{d}{dx}u\end{pmatrix}\\\Rarr\space x\frac{dy}{dx} +2y\frac{dy}{dx}-\frac{dy}{dx}$$
= sec2 x – y
$$\Rarr\space(x+2y-1)\frac{dy}{dx}=\\\text{sec}^{2}x-y\\\Rarr\space\frac{dy}{dx} =\frac{\text{sec}^{2}x-y}{x+2y-1}$$
5. x2 + xy + y2 = 100.
Sol. Given, x2 + xy + y2 = 100
Differentiating both sides w.r.t. x, we get
$$\frac{d}{dx}(x^{2} +xy+y^{2}) =0\\\Rarr\space 2x+\bigg(x\frac{dy}{dx}+y.1\bigg)+\\2y\frac{dy}{dx} = 0$$
$$\begin{pmatrix}\text{Using product rule}\\\frac{d}{dx}(u.v) =u\frac{d}{dx}v + v\frac{d}{dx}u\end{pmatrix}\\\Rarr\space\frac{dy}{dx}(x+2y) =\\-2x+y\\\Rarr\space\frac{dy}{dx} =-\frac{(2x+y)}{x+2y}$$
6. x3 + x2 y + xy2 + y3 = 81.
Sol. Given, x3 + x2y + xy2 + y3 = 81.
Differentiating both sides w.r.t. x, we get
$$\frac{d}{dx}(x^{3} +x^{2}y+xy^{2}+y^{2})\\=\frac{d}{dx}(81)\\\Rarr\space 3x^{2} +\frac{d}{dx}(x^{2}y) +\\\frac{d}{dx}(xy^{2}) +3y^{2}\frac{dy}{dx} = 0\\\Rarr\space 3x^{2} +x^{2}\frac{dy}{dx} +y(2x) +\\x\bigg(2y\frac{dy}{dx}\bigg) +y^{2}.1 +3y^{2}\frac{dy}{dx} = 0$$
$$\begin{pmatrix}\text{Using product rule}\\\frac{d}{dx}(u.v) =u\frac{d}{dx}v + v\frac{d}{dx}u\end{pmatrix}\\\Rarr\space(x^{2} +2xy +3y^{2})\frac{dy}{dx}\\=-3x^{2}-2xy-y^{2}\\\Rarr\space\frac{dy}{dx} =-\frac{(3x^{2} +2xy+y^{2})}{x^{2}+2xy+3y^{2}}$$
7. sin2 y + cos xy = k.
Sol. Given, sin2 y + cos xy = k
Differentiating both sides w.r.t. x, we get
$$\frac{d}{dx}(\text{sin}^{2}y +\text{cos xy}) =\frac{d}{dx}(k)\\\Rarr\space\frac{d}{dx}(\text{sin}^{2}y) +\frac{d}{dx}(\text{cos xy}) =0$$
$$\Rarr\space\text{2 siny cos y}\frac{dy}{dx} +\\(-\text{sin xy})\frac{d}{dx}(xy) =0\\\begin{pmatrix}\text{Using chain rule}\\\frac{d}{dx}f(g(x)) =f'(x)\frac{d}{dx}g(x)\end{pmatrix}\\\Rarr\space 2y\frac{dy}{dx} -\text{sin xy}\bigg(x\frac{dy}{dx} +y.1\bigg) =0$$
(∵ sin 2x = 2 sin x.cos x)
$$\Rarr\space\text{sin 2y}\frac{dy}{dx} -\text{x sin xy}\frac{dy}{dx}$$
= y sin xy
$$\Rarr\\\frac{dy}{dx} =\frac{y\space\text{sin(xy)}}{\text{sin 2y - x sin}(xy)}$$
8. sin2 x + cos2 y = 1.
Sol. Given, sin2x + cos2 y = 1
Differentiating both sides w.r.t. x, we get
$$\frac{d}{dx}(\text{sin}^{2}x +\text{cos}^{2}y)=\frac{d}{dx}(1)\\\Rarr\space\text{2 sin x cos x 2 cos y}\\\bigg(-\text{sin y}\frac{dy}{dx}\bigg) = 0$$
$$\begin{pmatrix}\text{Using chain rule}\\\frac{d}{dx}f(g(x)) = f'(x)\frac{d}{dx}g(x)\end{pmatrix}\\\Rarr\space -\text{2 sin ycos y}\frac{dy}{dx} =\\\text{- 2 sin x cos x}\\\Rarr\space\frac{dy}{dx} =\frac{-\text{sin 2x}}{-\text{sin 2y}}\\=\frac{\text{sin 2x}}{\text{sin 2y}}$$
(∵ sin 2x = 2 sin x.cos x)
$$\textbf{9.\space y = sin}^{\normalsize-1}\bigg(\frac{\textbf{2x}}{\textbf{1+x}^{\textbf{2}}}\bigg)\\\textbf{Sol.\space}Given,\\\space y =\text{sin}^{\normalsize-1}\bigg(\frac{2x}{1+x^{2}}\bigg)$$
Putting θ = tan–1 x i.e., x = tan θ
$$\text{Then, y = sin}^{\normalsize-1}\bigg(\frac{\text {2 tan}\space\theta}{\text{1 + tan}^{2}\theta}\bigg)$$
= sin–1 (sin 2θ) = 2θ = 2 tan–1x
$$\bigg[\because\space\text{sin 2}\theta =\frac{2\text{tan}\space\theta}{\text{1 + tan}^{2}\theta}\bigg]$$
Differentiating both sides w.r.t. x, we get
$$\frac{dy}{dx} = \frac{d}{dx}(2\space\text{tan}^{\normalsize-1}x)\\\frac{dy}{dx} = 2.\frac{1}{1+x^{2}}=\frac{2}{1 + x^{2}}\\\bigg[\because\space\frac{d}{dx}\text{tan}^{\normalsize-1}x=\frac{1}{1+x^{2}}\bigg]$$
$$\textbf{10.\space y = tan}^{\normalsize-1}\bigg(\frac{\textbf{3x-x}^{\textbf{3}}}{\textbf{1-3x}^{\textbf{2}}}\bigg)\textbf{,}\\\textbf{-}\frac{\textbf{1}}{\sqrt{\textbf{3}}}\lt\textbf{x}\lt\frac{\textbf{1}}{\sqrt{\textbf{3}}}.$$
Sol. Substitute tan–1x = θ i.e., x = tan θ,
$$\therefore\space\text{y = tan}^{\normalsize-1}\bigg(\frac{3x-x^{2}}{1-3x^{2}+}\bigg)\\=\text{tan}^{\normalsize-1}\bigg(\frac{3\text{tan}\theta - \text{tan}^{3}\theta}{\text{1 - 3 tan}^{2}\theta}\bigg)\\\begin{pmatrix}\because\space\text{tan 3}\theta =\\\frac{3\text{tan}\theta -\text{tan}^{3}\theta}{\text{1 -3 atan}^{2}\theta}\end{pmatrix}$$
$$\Rarr\space\text{y = tan}^{\normalsize-1}(\text{tan}\space3\theta)\\=3\theta = 3\text{tan}^{\normalsize-1}x$$
Differentiating both sides w.r.t. x, we get
$$\Rarr\space\frac{dy}{dx} = 3\frac{d}{dx}(\text{tan}^{\normalsize-1}x) =\frac{3}{\text{1 +x}^{2}}\\\bigg(\because\space\frac{d}{dx}(\text{tan}^{\normalsize-1}x) =\frac{1}{1 +x^{2}}\bigg)$$
$$\textbf{11. y = cos}^{\normalsize-1}\bigg(\frac{\textbf{1-x}^{\textbf{2}}}{\textbf{1 +x}^{\textbf{2}}}\bigg)\textbf{,}\\\textbf{0}\lt \textbf{x}\lt\textbf{1.}$$
Sol. Let tan–1x = θ i.e., x = tan θ
$$\therefore\space\text{y = cos}^{\normalsize-1}\bigg(\frac{\text{1-x}^{2}}{\text{1+x}^{2}}\bigg)\\=\text{cos}^{\normalsize-1}\bigg(\frac{\text{1-tan}^{2}\theta}{\text{1 +tan}^{2}\theta}\bigg)\\\bigg(\because\space\frac{\text{1-tan}^{2}\theta}{\text{1 + tan}^{2}\theta} =\text{cos 2}\theta\bigg)$$
$$\Rarr\space\text{y = cos}^{\normalsize-1}(\text{cos}2\theta)\\=2\theta = \text{2 tan}^{\normalsize-1}x$$
Differentiating both sides w.r.t. x, we get
$$\frac{dy}{dx}= 2\frac{d}{dx}(\text{tan}^{\normalsize-1}x)=\frac{2}{1 +x^{2}}\\\bigg(\because\space\frac{d}{dx}\text{tan}^{\normalsize-1}x =\frac{1}{1+x^{2}}\bigg)$$
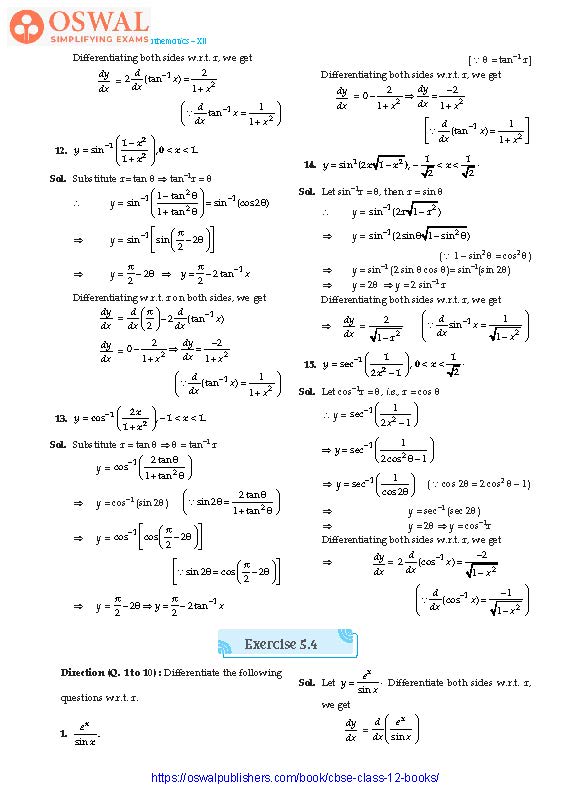
$$\textbf{12.\space y = sin}^{\normalsize-1}\bigg(\frac{\text{1-x}^{2}}{\text{1+x}^{2}}\bigg)\textbf{,}\\\textbf{0}\lt \textbf{x} \lt \textbf{1.}$$
Sol. Substitute x= tan θ
⇒ tan–1x = θ
$$\therefore\space\text{y = sin}^{\normalsize-1}\bigg(\frac{\text{1 - tan}^{2}\theta}{\text{1 +tan}^{2}\theta}\bigg)\\=\text{sin}^{\normalsize-1}(\text{cos}\space 2\theta)\\\Rarr\space y =\text{sin}^{\normalsize-1}\bigg[\text{sin}\bigg(\frac{\pi}{2} - 2\theta\bigg)\bigg]\\\Rarr\space y =\frac{\pi}{2} - 2\theta\\\Rarr\space y =\frac{\pi}{2}- 2\text{tan}^{\normalsize-1}x$$
Differentiating w.r.t. x on both sides, we get
$$\frac{dy}{dx} =\frac{d}{dx}\bigg(\frac{\pi}{2}\bigg)-\\2\frac{d}{dx}(\text{tan}^{\normalsize-1}x)\\\frac{dy}{dx} =0 -\frac{2}{1 +x^{2}}\\\Rarr\space\frac{dy}{dx} =\frac{\normalsize-2}{1+x^{2}}\\\begin{pmatrix}\because\space\frac{d}{dx}\begin{pmatrix}\text{tan}^{\normalsize-1}x\end{pmatrix} =\frac{1}{1+x^{2}}\end{pmatrix}$$
$$\textbf{13.\space y = cos}^{\normalsize-1}\bigg(\frac{2x}{\text{1 +x}^{2}}\bigg)\textbf{,}\\\textbf{\normalsize-1}\lt \textbf{x}\lt \textbf{1.}$$
Sol. Substitute x = tan θ
⇒ θ = tan–1 x
$$y =\text{cos}^{\normalsize-1}\bigg(\frac{2\text{tan}\theta}{\text{1 + tan}^{2}\theta}\bigg)\\\Rarr\space\text{y = cos}^{\normalsize-1}(\text{sin 2}\theta)\\\bigg(\because\space\text{sin 2}\theta =\frac{\text{2 tan}\theta}{\text{1 + tan}^{2}\theta}\bigg)\\\Rarr\space y =\text{cos}^{\normalsize-1}\bigg[\text{cos}\bigg(\frac{\pi}{2}-2 \theta\bigg)\bigg]\\\bigg[\because\space\text{sin 2}\theta = cos\bigg(\frac{\pi}{2}-2\theta\bigg)\bigg]\\\Rarr y =\frac{\pi}{2}-2\theta\\\Rarr\space y =\frac{\pi}{2} -2\text{tan}^{\normalsize-1}x$$
[∵ θ = tan–1 x]
Differentiating both sides w.r.t. x, we get
$$\frac{dy}{dx} =0-\frac{2}{\text{1+x}^{2}}\\\Rarr\space\frac{dy}{dx} =\frac{\normalsize-2}{\text{1 +x}^{2}}\\\bigg[\because\space\frac{d}{dx}(\text{tan}^{\normalsize-1} x) =\frac{1}{1 +x^{2}}\bigg]$$
$$\textbf{14.\space y = sin}^{\textbf{1}}(\textbf{2x}\sqrt{\textbf{1-x}^{\textbf{2}}})\textbf{,}\\\textbf{-}\frac{\textbf{1}}{\sqrt{\textbf{2}}}\lt x \lt\frac{\textbf{1}}{\sqrt{\textbf{2}}}\textbf{.}$$
Sol. Let sin–1x = θ, then x = sin θ
$$\therefore\space y =\text{sin}^{\normalsize-1}(2x\sqrt{1 - x^{2}})\\\Rarr\space\text{y = sin}^{\normalsize-1}\\(2\text{sin}\theta\sqrt{1- \text{sin}^{2}\theta})\\(\because\space 1 -\text{sin}^{2}\theta = \text{cos}^{2}\theta)\\\Rarr\space y =\text{sin}^{\normalsize-1}(2\text{sin}\theta\space cos\theta)\\=\text{sin}^{\normalsize-1}(\text{sin 2}\theta)\\\Rarr\space y = 2\theta\space\\\Rarr\space y = 2\text{sin}^{\normalsize-1 }x$$
Differentiating both sides w.r.t. x, we get
$$\Rarr\space\frac{dy}{dx} =\frac{2}{\sqrt{1 - x^{2}}}\\\bigg(\because\space\frac{d}{dx}\text{sin}^{\normalsize-1}x =\frac{1}{\sqrt{1 - x^{2}}}\bigg)$$
$$\textbf{15.\space y = sec}^{\normalsize-1}\bigg(\frac{\textbf{1}}{\textbf{2x}^{\textbf{2}}\textbf{- 1}}\bigg)\textbf{,}\\\textbf{0}\lt \textbf{x}\lt\frac{\textbf{1}}{\sqrt{\textbf{2}}}\textbf{.}$$
Sol. Let cos–1x = θ, i.e., x = cos θ
$$\therefore\space\text{y = sec}^{\normalsize-1}\bigg(\frac{1}{2x^{2}-1}\bigg)\\\Rarr\space y = \text{sec}^{\normalsize-1}\bigg(\frac{1}{\text{2 cos}^{2}\theta-1}\bigg)\\\Rarr\space y =\text{sec}^{\normalsize-1}\bigg(\frac{1}{\text{cos 2}\theta}\bigg)$$
(∵ cos 2θ = 2 cos2 θ – 1)
$$\Rarr\space y =\text{sec}^{\normalsize-1}(\text{sec}\space 2\theta)\\\Rarr\space y = 2\theta\space\\\Rarr\space y = \text{cos}^{\normalsize-1}x$$
Differentiating both sides w.r.t. x, we get
$$\Rarr\space\frac{dy}{dx} = 2\frac{d}{dx}(\text{cos}^{\normalsize-1}x)\\=\frac{\normalsize-2}{\sqrt{1 - x^{2}}}\\\bigg(\because\space\frac{d}{dx}(\text{cos}^{\normalsize-1}x) =\frac{\normalsize-1}{\sqrt{1 - x^{2}}}\bigg)$$
