NCERT Solutions for Class 12 Maths Chapter 5 Continuity and Differentiability - Exercise 5.8
Access Exercises of Class 12 Maths Chapter 5 – Continuity and Differentiability
Exercise 5.1 Solutions: 34 Questions (Short Answers)
Exercise 5.2 Solutions: 10 Questions (Short Answers)
Exercise 5.3 Solutions: 15 Questions ( Short Answers)
Exercise 5.4 Solutions: 10 Questions (Short Answers)
Exercise 5.5 Solutions: 18 Questions ( Short Answers)
Exercise 5.6 Solutions: 11 Questions (Short Answers)
Exercise 5.7 Solutions: 17 Questions (Short Answers)
Exercise 5.8 Solutions: 6 Questions (Short Answers)
Miscellaneous Exercise Solutions: 23 Questions (6 Long, 17 Short)
Exercise 5.8
1. Verify Rolle’s theorem for the function
f(x) = x2 + 2x – 8, x ∈ [– 4, 2].
Sol. Conditions for Rolle’s theorem.
(i) f(x) is continuous in [a, b]
(ii) f(x) is differentiable in (a, b) and
(iii) f(a) = f(b)
Then, there will be atleast one value of c ∈ (a, b) such that f ′(c) = 0
Given function is f(x) = x2 + 2x – 8, x ∈ [– 4, 2].
Since, a polynomial function is continuous and derivable on R, therefore
(i) f(x) is continuous on [– 4, 2].
(ii) f(x) is derivable on (–4, 2).
Also, f(– 4) = (– 4)2 + 2(– 4) – 8 = 0
[∵ f(x) = x2 + 2x – 8]
and f(2) = 22 + 2 × 2 – 8 = 0
⇒ f(– 4) = f(2)
This means that all the conditions of Rolle’s theorem are satisfied by f(x) in [– 4, 2].
Therefore, it exists atleast one real c ∈ (– 4, 2) such that f ′(c) = 0.
Now, f(x) = x2 + 2x –8
$$\Rarr\space f'(x) =\frac{d}{dx}(x^{2} +2x-8)$$
= 2x + 2
Putting f ′(c) = 0 ⇒ 2c + 2 = 0 ⇒ c = – 1.
Thus, f ′(– 1) = 0 and – 1 ∈ (– 4, 2).
∴ Rolle’s theorem is verified with c = – 1.
2. Examine if Rolle’s theorem is applicable to any of the following functions. Can you say someting about the converse of Rolle’s theorem from these example?
(i) f(x) = [x] for x ∈ [5, 9]
(ii) f(x) = [x] for x ∈ [– 2, 2]
(iii) f(x) = x2 – 1 for x ∈ [1, 2].
Sol. (i) Given function is f(x) = [x], x ∈ [5, 9]
Since, f(x) is neither continuous nor derivable at integral point i.e., at 5, 6, 7, 8 and 9.
Thus, f(x) is not continuous on [5, 9]. So, f(x) is not derivable on (5, 9).
Using property, if f(x) is not continuous then it is not differentiable.
Moreover, f(5) = [5] = 5 and f(9) = [9] = 9 i.e., f(5) ≠ f(9).
Hence, Rolle’s theorem is not applicable to f(x) in the [5, 9].
It may be noted that f ′(x) = 0 for all nonintegral points in (5, 9).
This shows that converse of Rolle’s theorem is not true.
(ii) Given function is f(x) = [x], x ∈ [– 2, 2]
Since f(x) is neither continuous nor differentiable at integral points i.e., – 2, 0, 1, 2.
Thus, f(x) is not continuous on [– 2, 2].
So, f is not derivable on (–2, 2).
Moreover, f(– 2) = [– 2] = – 2 ≠ 2 = f(2) i.e., f(– 2) ≠ f(2).
Hence, Rolle’s theorem is not applicable to f(x) in the given interval.
It may be noted that f ′(x) = 0 for all nonintegral points in (– 2, 2).
(iii) Given, f(x) = x2 – 1, which is a polynomial function. It is continuous and derivable at all x ∈ R.
In particular, f(x) is continuous on [1, 2] and derivable on (1, 2). But f(1) = 12 – 1 = 0 and f(2) = 22 – 1 = 3 i.e., f(1) ≠ f(2).
∴ Rolle’s theorem is not applicable to given function in the given interval. Note that f ′(x) = 2x ≠ 0 for any x in (1, 2).
Conclusion : From the above examples, we conclude that the converse of Rolle’s theorem does not hold. This means that
if conditions of Rolle’s theorem are not satisfied by a function f(x) on [a, b], then f ′(x) may or may not vanish at some point in (a, b).
3. If f : [– 5, 5] → R is a differentiable function and if f ′(x) does not vanish anywhere, then prove that f(– 5) ≠ f(5).
Sol. If we suppose that f(– 5) = f(5), then f would satisfy all the conditions of Rolle’s theorem on [– 5, 5].
(∵ Differentiable function is always continuous) Hence, there would exist atleast, one c ∈ (– 5, 5) such that f ′(c) = 0.
But f ′(x) does not vanish anywhere, therefore, our supposition is wrong and f(5) ≠ f(– 5).
4. Verify Mean Value Theorem if f(x) = x2 – 4x – 3 in the inteval [a, b], where a = 1 and b = 4.
Sol. Condition for Mean Value Theorem (MVT)
(i) f(x) is continuous in [a, b]
(ii) f(x) is differentiable in (a, b) and there will be atleast one value of c ∈ (a, b) such that
$$f'(c) =\frac{\text{f(b) - f(a)}}{b-a}$$
Here, f(x) = x2 – 4x – 3, x ∈ [1, 4] which is a polynomial function, so it is continuous and derivable at all x ∈ R, therefore
(i) f(x) is continuous on [1, 4]
(ii) f(x) is derivable on (1, 4).
∴ Conditions of Lagrange’s theorem are satisfied on [1, 4].
Hence, there is atleast one real number, c ∈ [1, 4] such that
$$f'(c) =\frac{f(4) -f(1)}{4-1}\\\bigg(\because\space f'(c)-\frac{\text{f(b)-f(a)}}{b-a}\bigg)\\\Rarr\space 2c-4 =\\\frac{(4^{2}-4×4-3) -(1^{2}-4×1-3)}{4-1} = 1$$
$$\begin{pmatrix}\because\space f'(x) =\frac{d}{dx}(x^{2}-4x-3)\\ 2x-4\end{pmatrix}\\\Rarr\space 2c-4 = 1\\\Rarr\space c =\frac{5}{2}\epsilon(1,4)$$
∴ MVT is verified for f(x) in [1, 4].
5. Verify Mean Value Theorem if f(x) = x3 – 5x2 – 3x in the interval [a, b], where a = 1 and b = 3.
Find all c = (1, 3) for which f ′(c) = 0.
Sol. Given, f(x) = x3 – 5x2 – 3x, x ∈ (1, 3), which is a polynomial function. Since, a polynomial f unction is continuous and derivable at all x ∈ R, therefore
(i) f(x) is continuous on [1, 3].
(ii) f(x) is derivable on (1, 3).
∴ Condition of Lagrange’s MVT are satisfied on [1, 3].
Hence, there exists atleast one real c ∈ (1, 3).
$$\text{Such that f'(c)} =\frac{f(3) - f(1)}{3-1}\\\Rarr\space 3c^{2} - 10 c -3 =\\\frac{(3^{3} - 5×3^{2} - 3×3) -(1 -5-3)}{3-1}\\\bigg(\because\space\text{f'(c)} -\frac{f(b) -f(a)}{b-a}\bigg)$$
$$\Rarr\space 3c^{2} - 10 c - 3 = -10\\\Rarr\space 3c^{2} -10c +7 = 0\\\Rarr\space c = \frac{10\pm\sqrt{100-84}}{6}\\=\frac{10\pm4}{6} = 1,\frac{7}{3}\\\text{Note that}\frac{7}{3}\epsilon(1,3).$$
Hence, Lagrange’s MVT is satisfied for the given function in the given interval.
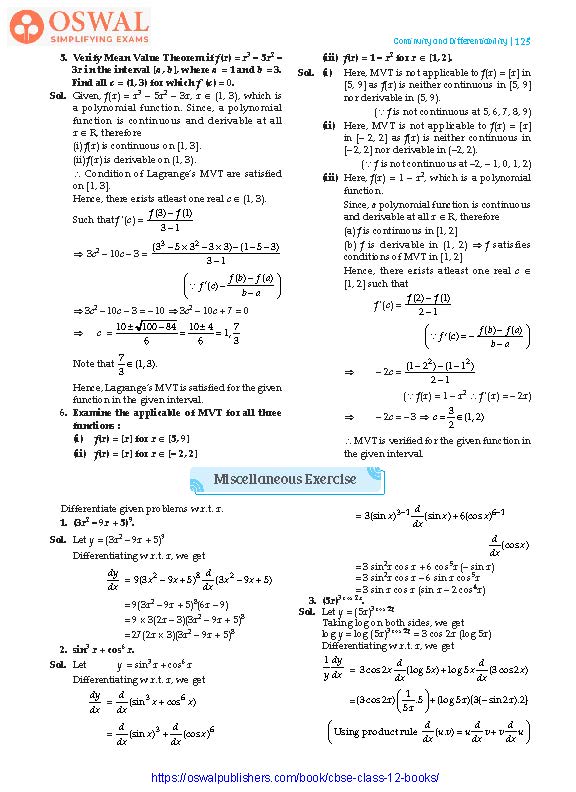
6. Examine the applicable of MVT for all three functions :
(i) f(x) = [x] for x ∈ [5, 9]
(ii) f(x) = [x] for x ∈ [– 2, 2]
(iii) f(x) = 1 – x2 for x ∈ [1, 2].
Sol. (i) Here, MVT is not applicable to f(x) = [x] in [5, 9] as f(x) is neither continuous in [5, 9] nor derivable in (5, 9).
(∵ f is not continuous at 5, 6, 7, 8, 9)
(ii) Here, MVT is not applicable to f(x) = [x] in [– 2, 2] as f(x) is neither continuous in [– 2, 2] nor derivable in (–2, 2).
(∵ f is not continuous at –2, – 1, 0, 1, 2)
(iii) Here, f(x) = 1 – x2, which is a polynomial function.
Since, a polynomial function is continuous and derivable at all x ∈ R, therefore
(a) f is continuous in [1, 2]
(b) f is derivable in (1, 2) ⇒ f satisfies conditions of MVT in [1, 2]
Hence, there exists atleast one real c ∈ [1, 2] such that
$$f'(c) =\frac{\text{f(2) - f(1)}}{2-1}\\\bigg(\because\space f'(c) =-\frac{f(b) -f(a)}{b-a}\bigg)\\\Rarr\space -2c =\\\frac{(1 - 2^{2}) -(1 - 1^{2})}{2-1}$$
(∵ f(x) = 1 – x2 ∴ f ′(x) = – 2x)
$$\Rarr\space -2c = -3\\\Rarr\space c =\frac{3}{2}\epsilon(1,2)$$
∴ MVT is verified for the given function in the given interval.
