NCERT Solutions for Class 12 Maths Chapter 5 Continuity and Differentiability - Miscellaneous Exercise
Access Exercises of Class 12 Maths Chapter 5 – Continuity and Differentiability
Exercise 5.1 Solutions: 34 Questions (Short Answers)
Exercise 5.2 Solutions: 10 Questions (Short Answers)
Exercise 5.3 Solutions: 15 Questions ( Short Answers)
Exercise 5.4 Solutions: 10 Questions (Short Answers)
Exercise 5.5 Solutions: 18 Questions ( Short Answers)
Exercise 5.6 Solutions: 11 Questions (Short Answers)
Exercise 5.7 Solutions: 17 Questions (Short Answers)
Exercise 5.8 Solutions: 6 Questions (Short Answers)
Miscellaneous Exercise Solutions: 23 Questions (6 Long, 17 Short)
Miscellaneous Exercise
Differentiate given problems w.r.t. x.
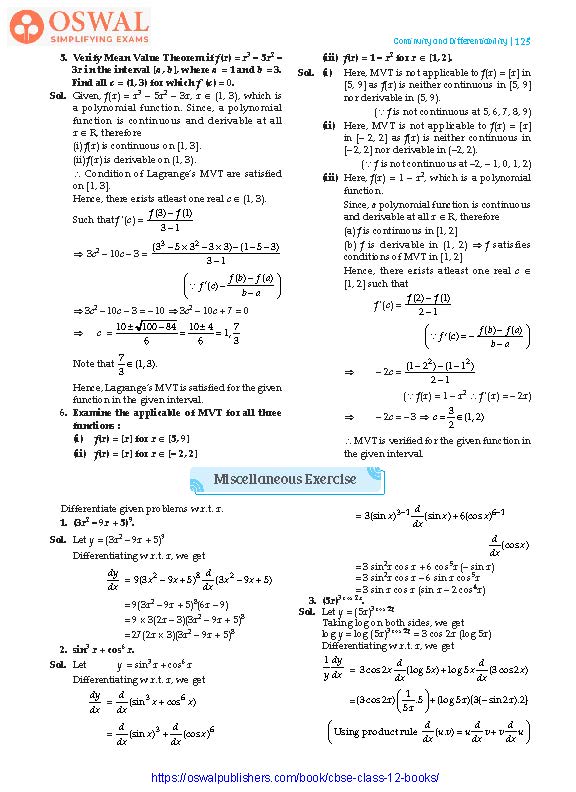
1. (3x2 – 9x + 5)9.
Sol. Let y = (3x2 – 9x + 5)9
Differentiating w.r.t. x, we get
$$\frac{dy}{dx} = 9(3x^{2} -9x +5)^{8}\\\frac{d}{dx}(3x^{2} -9x+5)$$
= 9(3x2 – 9x + 5)8(6x – 9)
= 9 × 3(2x – 3)(3x2 – 9x + 5)8
= 27(2x × 3)(3x2 – 9x + 5)8
2. sin3 x + cos6 x.
Sol. Let y = sin3 x + cos6 x
Differentiating w.r.t. x, we get
$$\frac{dy}{dx} = \frac{d}{dx}(\text{sin}^{3}x + \text{cos}^{6}x)\\=\frac{d}{dx}(\text{sin x})^{3} + \frac{d}{dx}(\text{cos x})^{6}\\= 3(\text{sin x})^{3-1}\frac{d}{dx}(sin \space x) +\\6(\text{cos x})^{6-1}\frac{d}{dx}(\text{cos x})$$
= 3 sin2x cos x + 6 cos5x (– sin x)
= 3 sin2x cos x – 6 sin x cos5x
= 3 sin x cos x (sin x – 2 cos4x)
3. (5x)3 cos 2x.
Sol. Let y = (5x)3 cos 2x
Taking log on both sides, we get
log y = log (5x)3 cos 2x = 3 cos 2x (log 5x)
Differentiating w.r.t. x, we get
$$\frac{1}{y}\frac{dy}{dx} = \text{3 cos 2x}\frac{d}{dx}(log \space 5x) +\\\text{log 5x}\frac{d}{dx}(3 cos 2x)\\ = (3 \space cos 2x)\bigg(\frac{1}{5x}.5\bigg) +\\\text{(log 5x)}\lbrace3(\normalsize- sin \space 2x).2\rbrace$$
$$\begin{pmatrix}\text{Using product rule}\\\frac{d}{dx}(u.v) = u\frac{d}{dx}v + v\frac{d}{dx}u\end{pmatrix}\\\Rarr\space\frac{dy}{dx} = y\begin{Bmatrix}\frac{\text{3 cos 2x}}{x} -\\\text{6(sin 2x)(log 5x)}\end{Bmatrix}\\\Rarr\space \frac{dy}{dx} = (5x)^{\text{3 cos 2x}}\\\begin{Bmatrix}\frac{\text{3 cos 2x}}{x} -\\\text{6 sin 2x log 5x}\end{Bmatrix}$$
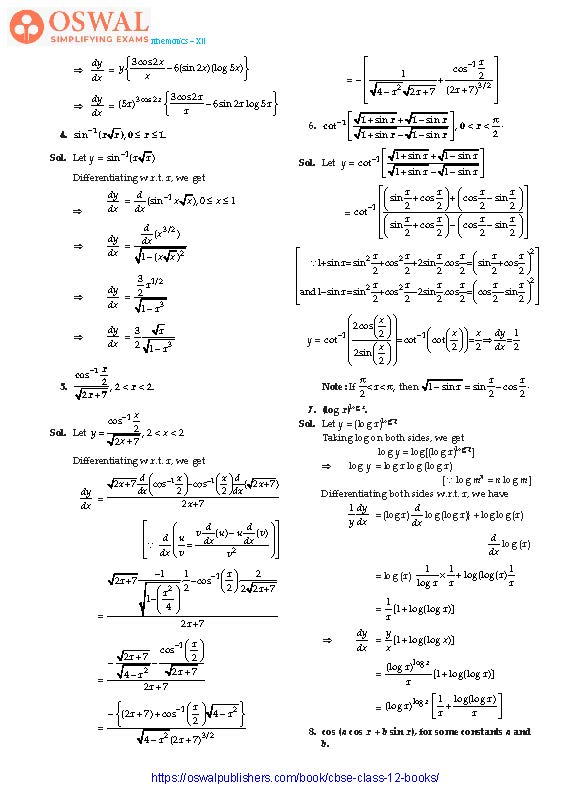
$$\textbf{4. sin}^{\normalsize-1}\textbf{(x}\sqrt{\textbf{x}}), \textbf{0}\leq \textbf{x} \leq \textbf{1.}\\\textbf{Sol.\space}\text{Let y = sin}^{\normalsize-1}(x\sqrt{x})$$
Differentiating w.r.t. x, we get
$$\Rarr\space \frac{dy}{dx} = \frac{d}{dx}(\text{sin}^{\normalsize-1}\space x\sqrt{x}),\\0\leq x\leq1\\\Rarr\space\frac{dy}{dx} = \frac{\frac{d}{dx}(x^{3/2})}{\sqrt{1 -(x\sqrt{x})^{2}}}\\\Rarr\space\frac{dy}{dx} =\frac{\frac{3}{2}x^{\frac{1}{2}}}{\sqrt{1 - x^{3}}}\\\Rarr\space \frac{dy}{dx} =\frac{3}{2}\frac{\sqrt{x}}{\sqrt{1- x^{3}}}$$
$$\textbf{5.\space}\frac{\textbf{cos}^{\normalsize-1}\frac{\textbf{x}}{\textbf{2}}}{\sqrt{\textbf{2x+7}}}\textbf{,} \textbf{2}\lt \textbf{x}\lt \textbf{2.}\\\textbf{Sol.\space} \text{Let y =}\frac{\text{cos}^{\normalsize-1}\frac{x}{2}}{\sqrt{2x+7}},\\ 2\lt x\lt 2$$
Differentiating w.r.t. x, we get
$$\frac{dy}{dx} = \\\frac{\sqrt{2x+7}\frac{d}{dx}\bigg(\text{cos}^{\normalsize-1}\frac{x}{2}\bigg) -\text{cos}^{\normalsize-1}\bigg(\frac{x}{2}\bigg)\frac{d}{dx}(\sqrt{2x +7})}{2x+7}$$
$$\begin{bmatrix}\because\space\frac{d}{dx}\begin{pmatrix}\frac{u}{v} =\frac{v\frac{d}{dx}(u) -u\frac{d}{dx}(v)}{v^{2}}\end{pmatrix}\end{bmatrix}\\ =\\\frac{\sqrt{2x+7}\frac{\normalsize-1}{\sqrt{1 -\bigg(\frac{x^{2}}{4}\bigg)}}.\frac{1}{2} -\text{cos}^{-1}\bigg(\frac{x}{2}\bigg)\frac{2}{2\sqrt{2x+7}}}{2x+7}$$
$$=\\\frac{\frac{\sqrt{2x+7}}{\sqrt{4-x^{2}}} -\frac{\text{cos}^{\normalsize-1}\bigg(\frac{x}{2}\bigg)}{\sqrt{2x+7}}}{2x+7}\\=\\\frac{-\begin{Bmatrix}(2x+7) + \text{cos}^{\normalsize-1}\bigg(\frac{x}{2}\bigg)\sqrt{4 - x^{2}}\end{Bmatrix}}{\sqrt{4 - x^{2}}(2x + 7)^{\frac{3}{2}}}\\= - \begin{bmatrix}\frac{1}{\sqrt{4 - x^{2}}\sqrt{2x+7}} + \frac{\text{cos}^{\normalsize-1}\frac{x}{2}}{(2x+7)^{\frac{3}{2}}}\end{bmatrix}$$
$$\textbf{6.\space cot}^{\normalsize-1}\begin{bmatrix}\frac{\sqrt{\textbf{1 + sin x}} + \sqrt{\textbf{1 - sin \space x}}}{\sqrt{\textbf{1 + sin x}} -\sqrt{\textbf{1 - sin x}}}\end{bmatrix}\textbf{,}\\\textbf{0}\lt \textbf{x}\lt\frac{\pi}{\textbf{2}}\textbf{.}\\\textbf{Sol.\space}\text{Let y = cot}^{\normalsize-1}\\\begin{bmatrix}\frac{\sqrt{1 + sin \space x} + \sqrt{\text{1 - sin x}}}{\sqrt{1 + sin x} - \sqrt{1 - sin x}}\end{bmatrix}\\ = \text{cot}^{\normalsize-1}\\\begin{bmatrix}\frac{\bigg(\text{sin}\frac{x}{2} +\text{cos}\frac{x}{2}\bigg) + \bigg(\text{cos}\frac{x}{2} -\text{sin}\frac{x}{2}\bigg)}{\bigg(\text{sin}\frac{x}{2} + \text{cos}\frac{x}{2}\bigg) -\bigg(\text{cos}\frac{x}{2} -\text{sin}\frac{x}{2}\bigg)}\end{bmatrix}$$
$$\begin{bmatrix}\because\space \text{1 + sin x = sin}^{2}\frac{x}{2} +\text{cos}^{2}\frac{x}{2} +\\\text{2 sin}\frac{x}{2}\text{cos}\frac{x}{2} =\bigg(\text{sin}\frac{x}{2} + \text{cos}\frac{x}{2}\bigg)^{2}\\\text{and 1 - sin x - sin}^{2}\frac{x}{2} + \text{cos}^{2}\frac{x}{2}-\\\text{2 sin}\frac{x}{2}.\text{cos}\frac{x}{2} =\bigg(\text{cos}\frac{x}{2} -\text{sin}\frac{x}{2}\bigg)\end{bmatrix}\\ y =\text{cot}^{\normalsize-1}\begin{pmatrix}\frac{\text{2 cos}\bigg(\frac{x}{2}\bigg)}{\text{2 sin}\bigg(\frac{x}{2}\bigg)}\end{pmatrix}\\=\text{cot}^{\normalsize-1}\begin{pmatrix}\text{cot}\bigg(\frac{x}{2}\bigg)\end{pmatrix} =\frac{x}{2}\\\Rarr\space \frac{dy}{dx} =\frac{1}{2}$$
$$\textbf{Note :}\space\text{If}\space\frac{\pi}{2}\lt x \lt\pi,\\\text{then}\space\sqrt{\text{1 - sin x}}$$
$$ =\text{sin}\frac{x}{2} -\text{cos}\frac{x}{2}.$$
7. (log x)log x.
Sol. Let y = (log x)log x
Taking log on both sides, we get
log y = log[(log x)log x]
⇒ log y = log x log (log x)
[∵ log mn = n log m]
Differentiating both sides w.r.t. x, we have
$$\frac{1}{y}\frac{dy}{dx} =\lbrace(log x)\frac{d}{dx}\text{log}(log x)\rbrace +\\\text{log log(x)}\frac{d}{dx}\space\text{log(x)}\\=\text{log(x)}\frac{1}{\text{log x}}×\frac{1}{x} + \\\text{log(log(x))}\frac{1}{x}\\=\frac{1}{x}\lbrack 1 + \text{log(log x)}\rbrack\\\Rarr\space\frac{dy}{dx} =\frac{y}{x}\lbrack 1+ \text{log(log x)}\rbrack\\=\space\frac{(log \space x)^{\text{log x}}}{x}\lbrack 1 +\text{log(log x)}\rbrack\\=\space \text{(log x)}^{\text{log x}}\begin{bmatrix}\frac{1}{x} + \frac{\text{log(log x)}}{x}\end{bmatrix}$$
8. cos (a cos x + b sin x), for some constants a and b.
Sol. Let y = cos (a cos x + b sin x)]
Differentiating w.r.t. x, we get
$$\frac{d}{dx}\lbrack\text{cos}(a cos x + b sin x)\rbrack\\= -\text{sin (a cos x + b sin x)}\frac{d}{dx}\\(\text{a cos x + b \space sin x})$$
= – sin (a cos x + b sin x){– a sin x + b cos x}
= a sin x – b cos x} sin(a cos x + b sin x)
$$\textbf{9.\space}\textbf{(sin x - cos x)}^{\textbf{(sin x - cos x)}}\textbf{,}\\\frac{\pi}{\textbf{4}}\lt x \lt \frac{\textbf{3}\pi}{\textbf{4}}\textbf{.}$$
Sol. Let y = (sin x – cos x)(sin x – cos x)
Taking log on both sides w.r.t. x, we get
log y = log {(sin x – cos x)(sin x – cos x)}
or log y = (sin x – cos x) log(sin x – cos x)
(∵ log mn = n log m)
Differentiating both sides w.r.t. x, we obtain
$$\frac{1}{y}\frac{dy}{dx} =(\text{sin x - cos x}).\\\frac{\text{cos x + sin x}}{\text{sin x - cos x}} + \\\lbrace \text{log}(\text{sin \space x - cos x)(cos x + sin x})\rbrace\\\Rarr\space \frac{dy}{dx} = y(cos x + sin x)\\\lbrace 1 + \text{log(sin x - cos x)}\rbrace$$
= (cos x + sin x)(sin x – cos x)(sin x – cos x)
{1 + log (sin x – cos x)}
10. xx + xa + ax + aa, for some fixed a > 0 and x > 0.
$$\textbf{Sol.\space}\frac{d}{dx}(x^{x} + x^{a} + x^{x} + a^{a})\\\Rarr\space \frac{d}{dx}(x^{x}) +\frac{d}{dx}(x^{a}) +\\\frac{d}{dx}(a^{x}) +\frac{d}{dx}(a^{a})\\\space = x^{x}\frac{d}{dx}(\text{x \space log x}) + ax^{a-1} +\\ a^{x}\space\text{log a + 0}\\\bigg(\because\space\frac{d}{dx}(u^{v}) = u^{v}\frac{d}{dx}(v\space log u)\bigg)\\= x^{x}\bigg(x\frac{1}{x} + \text{log x.1}\bigg) +\\\text{ax}^{a-1} + a^{x}\space\text{log a}$$
= xx(1 + log x) + axa –1 + ax log a
Alternative method :
Let y = xx + xa + ax + aa
Let u = xx ⇒ y = u + xa + ax + aa
$$\Rarr\space \frac{dy}{dx} = \frac{du}{dx} + \frac{d}{dx}x^{a} + \\\frac{d}{dx}a^{x} +\frac{d}{dx}a^{a}\\\Rarr\space\frac{dy}{dx} = \frac{du}{dx} +\\\text{ax}^{a-1} + a^{x}\space\text{log a}\\\text{...(i)}$$
Now, u = xx
Taking log on both sides,
⇒ log u = log xx = x log x
[∵ log mn = n log m]
Differentiating both sides w.r.t. x, we get
$$\Rarr\space \frac{1}{u}\frac{du}{dx} =\space\text{log x + x}\bigg(\frac{1}{x}\bigg)$$
= 1 + log x
$$\Rarr\space \frac{du}{dx} = u(1 + log x)$$
= xx(1 + log x)
$$\text{Putting}\space\frac{du}{dx}\space\text{in eq. (i). we get}\\\frac{dy}{dx} = x^{x}(1 + log x) +\\ ax^{a-1} + a^{x}\text{log a}$$
11. xx2 −3 + (x − 3)x2 for x > 3.
Sol. Let y = xx2 −3 + ( − 3)x2
Again let u = xx2-3 and v = (x-3)x2
⇒ y = u + v
Differentiating both sides w.r.t. x, we get
$$\frac{dy}{dx} = \frac{du}{dx} + \frac{dv}{dx}\space\\\text{...(i)}$$
Now, u = xx2 −3
Taking log on both sides,
log u = log(x) x2 −3 = (x2 - 3)log x
Differentiating both sides w.r.t. x, we get
$$\frac{1}{u}.\frac{du}{dx} =(x^{2}-3).\frac{1}{x} +\\\text{2x log x}\\\Rarr\space \frac{du}{dx} =u\begin{bmatrix}\frac{x^{2}-3}{x} +\text{2x log x}\end{bmatrix}\\\Rarr\space \frac{du}{dx} = x^{x^{2}-3}\\\begin{bmatrix}\frac{x^{2}-3}{x} +\\\text{2 x log x}\end{bmatrix}$$
Now, v = (x − 3)x2
Taking log on both sides,
log v = log(x − 3)x2 = x2 log(x – 3)
(∵ log mn = n log m)
Differentiating both sides w.r.t. x, we get
$$\frac{1}{v}\frac{dv}{dx} = x^{2}\frac{d}{dx}\text{log}(x-3) +\\\text{log}(x-3)\frac{d}{dx}x^{2}\\ = x^{2}.\frac{1}{x-3} + \text{2x\space log}(x-3)\\\frac{dv}{dx} = v\begin{bmatrix}\frac{x^{2}}{x-3} + \text{2x . log(x-3)}\end{bmatrix}\\=\frac{dv}{dx} =(x-3)^{x^{2}}\\\begin{bmatrix}\frac{x^{2}}{\text{x-3}} + \text{2x .log(x-3)}\end{bmatrix}\\\text{Putting the values of}\\\frac{du}{dx}\space\text{and}\space\frac{dv}{dx}\space\text{in Eq. (i), we get}$$
$$\frac{dy}{dx} = x^{x^{2}}-3\begin{bmatrix}\frac{x^{2}-3}{x} +\text{2x log x}\end{bmatrix}+\\\text{(x-3)}^{x^{2}}\begin{bmatrix}\frac{x^{2}}{x-3} +\\\text{2x log(x-3)}\end{bmatrix}$$
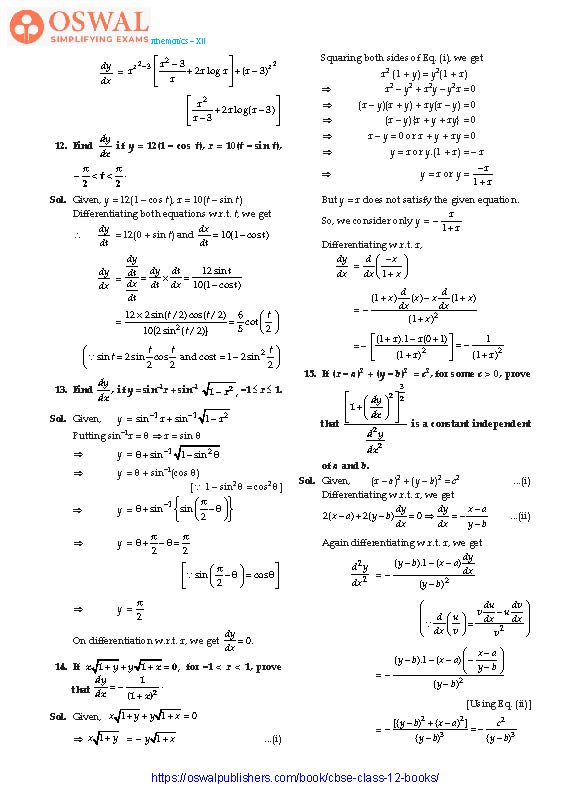
$$\textbf{12. Find}\space\frac{\textbf{dy}}{\textbf{dx}}\space \textbf{if y = 12(1 - cos t)}\textbf{,}\\\textbf{x = 10(t - sin t)}\textbf{,}\\\textbf{-}\frac{\pi}{\textbf{2}}\lt \textbf{t}\lt\frac{\pi}{\textbf{2}}\textbf{.}$$
Sol. Given, y = 12(1 – cos t), x = 10(t – sin t)
Differentiating both equations w.r.t. t, we get
$$\therefore\space\frac{dy}{dt} = 12(0 + sin \space t)\space\text{and}\\\frac{dx}{dt} = \text{10(1 - cost)}\\\frac{dy}{dx} =\frac{\frac{dy}{dt}}{\frac{dx}{dt}} =\frac{dy}{dt}×\frac{dt}{dx}\\=\frac{\text{12 sint}}{10(\text{1 - cos t})}\\= \frac{12 × 2 \text{sin}(\frac{t}{2})\text{cos}(\frac{t}{2})}{10\lbrack\text{2 sin}^{2}(\frac{t}{2})\rbrack}\\=\frac{6}{5}\text{cot}\bigg(\frac{t}{2}\bigg)$$
$$\begin{pmatrix}\because\space\text{sin t = 2 sin}\frac{t}{2}\text{cos}\frac{t}{2}\\\text{and cos t = 1 - 2 sin}^{2}\frac{t}{2}\end{pmatrix}$$
$$\textbf{13.\space Find}\space\frac{\textbf{dy}}{\textbf{dx}}\textbf{,}\space\textbf{if y = sin}^{\normalsize-1}\textbf{x +}\\\textbf{sin}^{\normalsize-1}\textbf{x}\space\sqrt{\textbf{1 - x}^{\textbf{2}}}\textbf{,}\space \normalsize\textbf{-1}\leq \textbf{x} \leq \textbf{1.}\\\textbf{Sol.\space}\text{Given,\space y = sin}^{\normalsize-1}x +\\\text{sin}^{\normalsize-1}\sqrt{1 - x^{2}}$$
Putting sin–1x = θ ⇒ x = sin θ
$$\Rarr\space\text{y =}\space \theta + \text{sin}^{\normalsize-1}\sqrt{1 - sin^{2}\theta}$$
$$\Rarr\space y =\theta + \text{sin}^{\normalsize-1}(\text{cos}\space\theta)\\\lbrack\because\space 1 - \text{sin}^{2}\theta =\text{cos}^{2}\theta\rbrack\\\Rarr\space\text{y =}\space\theta +\text{sin}^{\normalsize-1}\\\begin{Bmatrix}\text{sin}\bigg(\frac{\pi}{2} -\theta\bigg)\end{Bmatrix}\\\Rarr\space y = \theta +\frac{\pi}{2}-\theta =\frac{\pi}{2}\\\begin{bmatrix}\because\space\text{sin}\bigg(\frac{\pi}{2}-\theta\bigg) =\text{cos}\space\theta\end{bmatrix}\\\Rarr\space y =\frac{\pi}{2}$$
On differentiation w.r.t. x,
$$\text{we get}\space\frac{dy}{dx} = 0.$$
$$\textbf{14.\space If x}\sqrt{\textbf{1 + y}} \textbf{+ y}\sqrt{\textbf{1 +x}} \textbf{= 0,}$$
for –1 < x < 1, prove that
$$\frac{\textbf{dy}}{\textbf{dx}} \textbf{=-}\frac{\textbf{1}}{\textbf{(1 +x)}^{\textbf{2}}}\textbf{.}\\\textbf{Sol.\space}\text{Given,\space}\\ x\sqrt{1 +y}\sqrt{1 +x} = 0\\\Rarr\space x\sqrt{1 + y} =-y\sqrt{1 +x}\\\text{...(i)}$$
Squaring both sides of Eq. (i), we get
x2 (1 + y) = y2(1 + x)
$$\Rarr\space x^{2} - y^{2} + x^{2}y-y^{2}x = 0\\\Rarr\space (x-y)(x+y) +xy(x-y) = 0\\\Rarr\space (x-y)\lbrace x +y +xy\rbrace = 0\\\Rarr\space x-y =0 \space \text{or} \space x+y+xy = 0\\\Rarr\space y = x\space\text{or y.}(1 +x) = -x\\\Rarr\space\text{y = x or y} = \frac{\normalsize-x}{1 +x}$$
But y = x does not satisfy the given equation.
So, we consider only
$$y = -\frac{x}{\text{1 + x}}$$
Differentiating w.r.t. x,
$$\frac{dy}{dx} = \frac{d}{dx}\bigg(\frac{\normalsize-x}{1 + x}\bigg)\\=-\frac{(1 + x)\frac{d}{dx}(x) - x\frac{d}{dx}(1 + x)}{(1 +x)^{2}}\\=-\begin{bmatrix}\frac{(1 + x).1 - x(0+1)}{(1 + x)^{2}}\end{bmatrix}\\=-\frac{1}{(1 + x)^{2}}$$
15. If (x – a)2 + (y – b)2 = c2, for some c > 0, prove that
$$\frac{\bigg[\textbf{1 +} \bigg(\frac{\textbf{dy}}{\textbf{dx}}\bigg)^{\textbf{2}}\bigg]^{\frac{\textbf{3}}{\textbf{2}}}}{\frac{\textbf{d}^{\textbf{2}}\textbf{y}}{\textbf{dx}^{\textbf{2}}}}\space\textbf{is a constant}$$
independent of a and b.
Sol. Given, (x – a)2 + (y – b)2 = c2 ...(i)
Differentiating w.r.t. x, we get
$$\text{2(x-a)} + 2(y-b)\frac{dy}{dx} =0\\\Rarr\space\frac{dy}{dx} =-\frac{x-a}{y-b}\\\text{...(ii)}$$
Again differentiating w.r.t. x, we get
$$\frac{d^{2}y}{dx^{2}} =-\frac{(y-b).1 -(x-a)\frac{dy}{dx}}{(y-b)^{2}}\\\begin{pmatrix}\because\space\frac{d}{dx}\bigg(\frac{u}{v}\bigg) =\frac{v\frac{d}{dx} - u\frac{dv}{dx}}{v^{2}}\end{pmatrix}\\=\\ -\frac{(y-b).1 -(x-a)\bigg(-\frac{x-a}{y-b}\bigg)}{(y-b)^{2}}$$
[Using Eq. (ii)]
$$= -\frac{\lbrack(y-b)^{2} + (x-a)^{2}\rbrack}{(y-b)^{3}}\\=-\frac{c^{2}}{(y-b)^{3}}\\\text{Now,\space}\frac{\begin{bmatrix}1 + \bigg(\frac{dy}{dx}\bigg)^{2}\end{bmatrix}^{\frac{3}{2}}}{\frac{d^{2}y}{dx^{2}}}\\=\frac{\begin{bmatrix}1 + \bigg(\frac{x-a}{y-b}\bigg)^{2}\end{bmatrix}^{\frac{3}{2}}}{-\frac{c^{2}}{(y-b)^{3}}}$$
$$=\frac{\lbrace(y-b)^{2} + (x-a)^{2}\rbrace^{\frac{3}{2}}}{\lbrace(y-b)^{2}\rbrace^{\frac{3}{2}}\frac{(\normalsize-c^{2})}{(y-b)^{3}}}\\=\frac{(c^{2})^{\frac{3}{2}}}{-c^{2}} = \frac{c^{3}}{-c^{2}} = -c.$$
which is independent of a and b.
Hence proved.
16. If cos y = x cos (a + y), with cos a ≠ ±1, prove that
$$\frac{\textbf{dy}}{\textbf{dx}} \textbf{=}\frac{\textbf{cos}^{2}\textbf{(a+y)}}{\textbf{sin a}}\textbf{.} $$
Sol. Given, cos y = x cos (a + y)
$$\Rarr\space x =\frac{\text{cos y}}{\text{cos(a + y)}}$$
Differentiating both sides w.r.t. y, we get
$$\frac{dx}{dy} =\frac{d}{dy}\begin{Bmatrix}\frac{\text{cos y}}{\text{cos}(a+y)}\end{Bmatrix}\\=\\\frac{\text{cos}(a+y)(-sin\space y) - cos \space y(-\text{sin a + y}).1)}{\text{cos}^{2}(a+y)}$$
$$\begin{pmatrix}\text{Using quotient rule,}\\\frac{d}{dy}\bigg(\frac{u}{v}\bigg) =\frac{v\frac{d}{dy}u - u\frac{d}{dy}v}{v^{2}}\end{pmatrix}\\=\frac{\text{sin}(a+y)cos\space y - cos(a+y)sin \space y}{\text{cos}^{2}(a+y)}\\=\frac{\text{sin}(a + y-y)}{\text{cos}^{2}(a+y)} =\frac{\text{sin a}}{\text{cos}^{2}(a+y)}$$
(∵ sin A – B) = sin A cos B – cos A sin B
$$\therefore\space \frac{dy}{dx} =\frac{1}{\frac{dx}{dy}} =\frac{\text{cos}^{2}(a+y)}{sin \space a}.$$
17. If x = a(cos t + t sin t) and y = a(sin t – t cos t), $$\textbf{find}\space\frac{\textbf{d}^{\textbf{2}}\textbf{y}}{\textbf{dx}^{\textbf{2}}}\textbf{.}$$
Sol. Given, x = a(cos t + t sin t) and y = a(sin t – t cos t)
Differentiating w.r.t. t, we get
$$\frac{dx}{dt} = a\begin{bmatrix}\frac{d}{dt}\text{cos t} + \\\bigg(t\frac{d}{dt}(\text{sin \space t}) + \text{sin t}\frac{d}{dt}(t)\bigg)\end{bmatrix}$$
$$\therefore\space\frac{dx}{dt} = a\\\lbrack-\text{sin t + (t cos t + sin t.1)}\rbrack\\=\text{at\space cos t}\\\text{and\space}\frac{dy}{dt} = a\\\begin{bmatrix}\frac{d}{dt}\text{sin t} -\\\bigg(t\frac{d}{dt}\text{cos t + cos t.1}\bigg)\end{bmatrix}$$
$$\Rarr\space \frac{dy}{dt} = a\lbrace\text{cos t - (t(- sin t))+}\\\text{cos t.1}\rbrace = \text{at sin t}\\\Rarr\space\frac{dy}{dx} =\frac{\frac{dy}{dt}}{\frac{dx}{dt}}\\=\frac{\text{at sin t}}{\text{at cos t}}$$
= tan t
$$\text{i.e.,\space}\frac{dy}{dx} =\text{tan t}\space\text{...(i)}$$
Differentiating again w.r.t. x, we get
$$\frac{d^{2}y}{dx^{2}} =\frac{d}{dx}(\text{tan t})\\=\frac{d}{dt}(\text{tan t})\frac{dt}{dx}\\=\frac{\text{sec}^{2}t}{\text{at cos t}}\\\begin{Bmatrix}\because\space\frac{dx}{dt} =\text{at cos t}\end{Bmatrix}\\=\frac{1}{at}\text{sec}^{3}t$$
The result is valid for all real t except 0 and odd multiplies of
$$\frac{\pi}{2}.$$
18. If f(x) = |x|3, show that f ′′(x) exists for all real x and find it.
Sol. Here, f(x) = |x|3
When x ≥ 0, f(x) = |x|3 = x3
Differentiating both sides w.r.t. x, we have
$$\frac{d}{dx}\lbrack f(x)\rbrack =\frac{d}{dx}(3x^{2})\\\Rarr\space f'(x) = 3x^{2}$$
Again differentiating both sides w.r.t. x, we have
$$\frac{d}{dx}\lbrack f'(x)\rbrack = \frac{d}{dx}(3x^{2})\\\Rarr\space f''(x) = 6x$$
When x < 0.
f(x) = |x|3 = – x3
Differentiating both sides w.r.t. x, we have
$$\frac{d}{dx}\lbrack f(x)\rbrack =\frac{d}{dx}(-x^{3})\\\Rarr\space f'(x) = -3x^{2}$$
Again differentiating both sides w.r.t. x, we get
$$\frac{d}{dx}\lbrack f'(x)\rbrack =\frac{d}{dx}(-3x^{2}) =-6x$$
f ′′(x) = – 6x.
$$\text{Hence, f'(x) =}\begin{cases}6x,\space x\geq0\\-6x,\space x\lt 0\end{cases}$$
19. Using mathematical induction prove that
$$\frac{\textbf{d}}{\textbf{dx}}(\textbf{x}^{\textbf{n}}) = \pi \textbf{x}^{\textbf{n-1}}\space$$
for all positive integers n.
$$\textbf{Sol.\space}\text{Let P(n) =}\frac{d}{dx}(x^{n}) = nx^{n-1}\\\text{Now\space P(1) is}\frac{d}{dx}(x^{1}) = 1.x^{1-1}\\\text{i.e.,}\space\frac{d}{dx}(x)=1,\space\text{which is true.}$$
∴ P(1) is true. Let P(m) be true, m ∈ N
$$\Rarr\space\frac{d}{dx}(x^{m}) = mx^{m-1}\\\text{...(i)}\\\Rarr\space\frac{d}{dx}(x^{m+1}) =\frac{d}{dx}(x^{m}x)\\= x^{m}\frac{d}{dx}(x) + x\frac{d}{dx}(x^{m}) $$
(by product rule)
= xm.1 + x(mxm-1) = (m+1)xm
[using Eq. (i)]
$$\Rarr\space\frac{d}{dx}(x^{m+1}) = (m+1)x^{(m+1)-1}$$
⇒ P(m + 1) is true. Thus, P(1) is true and P(m)
⇒ P(m + 1), m ∈ N.
Hence, by induction P(n) is true for all n ∈ N.
20. Using the fact that sin (A + B) = sin A cos B + cos A sin B and the differentiation, obtain the sum formula for cosines.
Sol. Using sin (A + B) = sin A cos B + cos A sin B, we have on differentiating w.r.t. x, regard B as constant and consider A as a formula for x, we have
$$\text{cos(A +B)}\frac{dA}{dx} =\text{cos B. cos A}\frac{dA}{dx} -\\\bigg(\text{sin A}\frac{dA}{dx}\bigg)\text{sin B}\\\Rarr\space \text{cos(A + B)}\frac{dA}{dx} =\\\text{(cos A cos B - sin A sin B)}\\\frac{dA}{dx}$$
$$\Rarr\space\text{cos(A + B)} =\\\space\text{cos A cos B - sin A sin B}\\\bigg(\because\space\frac{dA}{dx}\neq 0\bigg)$$
21. Does there exist a function which is continuous every where but not differentiable at exactly two points? Justify your answer.
Sol. Yes, we have the continuous function f(x) = |x – 1| + |x – 2|, which is continuous at all x ∈ R but differentiable at all x except 1, 2.
Here, f(x) = |x – 1| + |x – 2|
$$=\begin{Bmatrix}-(x-1)-(x-2),\space \text{if x}\lt 1\\ x-1-(x-2),\space \text{if\space 1}\leq x\leq 2\\ x-1 +x-2,\space \text{if}\space x\gt 2\end{Bmatrix}$$
$$\text{i.e.\space f(x)} =\begin{cases}-2x+3,\space\text{if x}\lt 1\\1,\space \text{if 1}\leq x \leq 2\\2x-3,\space\text{if x}\gt 2\end{cases}$$
Since, polynomial function is continuous, so it is clear that f is continuous at all except possible at 1, 2.
Now, we have to check the continuity at 1, 2
At x = 1 f(1) = 1
$$\text{LHL =}\lim_{x\xrightarrow{}1^{\normalsize-}}\space f(x) =\\\lim_{x\xrightarrow{} 1^{\normalsize-}}(-2x+3)$$
= – 2 + 3 = 1
$$\text{RHL = }\lim_{x\xrightarrow{}1^{\normalsize+}}\space f(x) =\\\lim_{x\xrightarrow{}1^{\normalsize+}} 1 = 1\\\Rarr\space f(1) =\lim_{x\xrightarrow{}1^{\normalsize-}}\space f(x) =\\\lim_{x\xrightarrow{}1^{\normalsize+}} f(x)\\\Rarr\space\text{f is continuous at x = 1}$$
At x = 2 f(2) = 1
$$\text{LHL =}\lim_{x\xrightarrow{}2^{\normalsize-}}\space f(x) =\\\lim_{x\xrightarrow{}2^{\normalsize-}}\space f(1)= 1\\\text{RHL =}\lim_{x\xrightarrow{}2^{\normalsize+}}\space f(x) =\lim_{x\xrightarrow{}2^{\normalsize-}}(2x-3)\\\Rarr\space f(x) =\lim_{x\xrightarrow{}2^{0}}\space f(x) =\\\lim_{x\xrightarrow{} 2^{\normalsize+}}\space f(x)$$
So, f is continuous at x = 2. Thus, f is continuous at all x ∈ R.
$$\text{Also,\space f'(x)} =\begin{cases}-2,\space\text{if x}\lt 1\\0,\space\text{if 1}\lt x\lt 2\\2,\space\text{if x}\gt 2\end{cases}$$
We check the differentiability at 1, 2
$$\text{Lf'(1)} =\\\lim_{h\to0^{\normalsize-}}\frac{f(1 - h) -f(1)}{-h}\\=\lim_{h\to0^{\normalsize-}}\frac{-2(1 - h) + 3-1}{\normalsize-h} =-2$$
$$\text{and\space}\text{Rf'(1) =}\\\lim_{h\to0^{\normalsize-}}\frac{f(1 +h) -f(1)}{h}\\=\lim_{h\to0^{\normalsize+}}\frac{1-1}{h} = 0$$
∴ Lf′(1) ≠ Rf′(1) ⇒ f is not differentiable at 1. Further,
$$\Rarr\space\text{Lf'(2)} =\\\lim_{h\to0^{\normalsize-}}\frac{\text{f(2-h) -f(2)}}{\normalsize-h}\\=\lim_{h\to0^{\normalsize+}}\frac{1-1}{-h} = 0\\\text{and Rf'(2)} =\\\lim_{h\to0^{\normalsize+}}\frac{f(2+h)-f(2)}{h}\\=\lim_{h\to0^{\normalsize+}}\frac{2(2+h)-3-1}{h} = 2$$
∴ Lf′(2) ≠ Rf′(2)
⇒ f is not differentiable at 2.
Thus, we see that f(x) = |x – 1|+ |x – 2| is continuous everywhere and differentiable also at all x ∈ R except at 1, 2.
$$\textbf{22.\space If y =}\begin{vmatrix}\textbf{f(x)} &\textbf{g(x)} &\textbf{h(x)}\\\textbf{l} &\textbf{m} &\textbf{n}\\\textbf{a} &\textbf{b} &\textbf{c}\end{vmatrix}\textbf{,}\\\textbf{prove that}\\\frac{\textbf{dy}}{\textbf{dx}} =\begin{vmatrix}\textbf{f'(x)} &\textbf{g'(x)} &\textbf{h}'(x)\\\textbf{l} &\textbf{m} &\textbf{n}\\\textbf{a} &\textbf{b} &\textbf{c}\end{vmatrix}\\\textbf{Sol.\space}\text{Given,}\\{\text y =}\begin{vmatrix}f(x) &g(x) &h(x)\\l &m &n\\a &b &c\end{vmatrix}$$
Expand along R1
⇒ y = (mc – nb)f(x) + (na – lc)g(x) + (lb – ma)h(x)
Differentiating w.r.t. x,
$$\Rarr\space \frac{dy}{dx} =(mc - nb)f'(x) +\\ (na - lc) h(x) +\\\text{(lb - ma)}h'(x)\\\therefore\space\frac{dy}{dx} =\begin{vmatrix}f'(x) &g'(x) &h'(x)\\l &m &n\\a &b &c\end{vmatrix} $$
$$\textbf{23.\space If y =}\space \textbf{e}^{\textbf{a cos}^{\normalsize-1}}\textbf{x}\textbf{,}\\\space \textbf{\normalsize-1}\leq \textbf{x} \leq \textbf{1}\space\textbf{show that}\\\textbf{(1 - x}^{\textbf{2}})\frac{\textbf{d}^{\textbf{2}}\textbf{y}}{\textbf{dx}^{\textbf{2}}} \textbf{- x}\frac{\textbf{dy}}{\textbf{dx}} \textbf{- a}^{\textbf{2}}\textbf{y} \textbf{= 0.}$$
Sol. Given, y = ea cos−1 x ...(i)
Differentiating w.r.t. x, we get
$$\Rarr\space \frac{dy}{dx} = e^{a cos^{\normalsize-1}}x\frac{d}{dx}\text{a cos}^{\normalsize-1}x\\ = e^{cos^{\normalsize-1}x}.\frac{\normalsize-a}{\sqrt{1 - x^{2}}}\\\Rarr\space\frac{dy}{dx} =\frac{-ay}{\sqrt{1 - x^{2}}}\\\bigg(\because\space e^{a cos^{\normalsize-1}x} = y\bigg)\\\Rarr\space\sqrt{1 - x^{2}}\frac{dy}{dx} =-ay\\\text{...(ii)}$$
Again differentiating w.r.t. x, we get
$$\sqrt{1 - x^{2}}\frac{d^{2}y}{dx^{2}} +\frac{dy}{dx}.\\\frac{-2x}{2\sqrt{1-x^{2}}} =-a\frac{dy}{dx}\\\Rarr\space\sqrt{1 - x^{2}}\frac{d^{2}y}{dx^{2}} + \\\bigg(\frac{\normalsize-x}{\sqrt{1 - x^{2}}}\bigg)\frac{dy}{dx} =\frac{-a(-ay)}{\sqrt{1 - x^{2}}}$$
[Using Eq. (ii)]
Multiplying throughout by
$$\sqrt{1 - x^{2},}\space\text{we get}\\(1 - x^{2})\frac{d^{2}y}{dx^{2}}-x\frac{dy}{dx}-a^{2}y = 0$$
